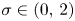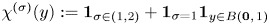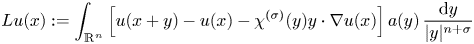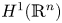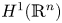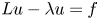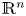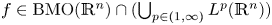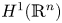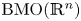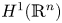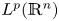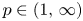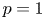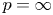1. Introduction
Let $n\ge 1$![]() , $\sigma \in (0,\,2)$
, $\sigma \in (0,\,2)$![]() , $\chi ^{(\sigma )}(y):={\mathbf 1}_{\sigma \in (1,2)}+{\mathbf 1}_{\sigma =1} {\mathbf 1}_{y\in B(\mathbf {0},\,1)}$
, $\chi ^{(\sigma )}(y):={\mathbf 1}_{\sigma \in (1,2)}+{\mathbf 1}_{\sigma =1} {\mathbf 1}_{y\in B(\mathbf {0},\,1)}$![]() , where $\mathbf {0}$
, where $\mathbf {0}$![]() denotes the origin of $\mathbb {R}^n$
denotes the origin of $\mathbb {R}^n$![]() , and $a$
, and $a$![]() be a non-negative and bounded measurable function on ${\mathbb {R}^n}$
be a non-negative and bounded measurable function on ${\mathbb {R}^n}$![]() . In this paper, we first consider the boundedness of the non-local elliptic operator
. In this paper, we first consider the boundedness of the non-local elliptic operator
from the Sobolev space based on $\mathrm {BMO}(\mathbb {R}^n)\cap (\bigcup _{p\in (1,\infty )}L^p(\mathbb {R}^n))$![]() to the BMO (bounded mean oscillation) space $\mathrm {BMO}(\mathbb {R}^n)$
to the BMO (bounded mean oscillation) space $\mathrm {BMO}(\mathbb {R}^n)$![]() , and from the Sobolev space based on the Hardy space $H^1(\mathbb {R}^n)$
, and from the Sobolev space based on the Hardy space $H^1(\mathbb {R}^n)$![]() to $H^1(\mathbb {R}^n)$
to $H^1(\mathbb {R}^n)$![]() . Assume further that $\lambda \in (0,\,\infty )$
. Assume further that $\lambda \in (0,\,\infty )$![]() , $p\in (1,\,\infty )$
, $p\in (1,\,\infty )$![]() , and $f$
, and $f$![]() belongs to $L^p({\mathbb {R}^n})\cap \mathrm {BMO}({\mathbb {R}^n})$
belongs to $L^p({\mathbb {R}^n})\cap \mathrm {BMO}({\mathbb {R}^n})$![]() or the Hardy space $H^1({\mathbb {R}^n})$
or the Hardy space $H^1({\mathbb {R}^n})$![]() , we also investigate the unique solvability of the non-local elliptic equation
, we also investigate the unique solvability of the non-local elliptic equation
in the Sobolev space based on $\mathrm {BMO}(\mathbb {R}^n)$![]() or $H^1(\mathbb {R}^n)$
or $H^1(\mathbb {R}^n)$![]() . The results obtained in this paper are further devolvement of the corresponding results in the scale of the Lebesgue space $L^p(\mathbb {R}^n)$
. The results obtained in this paper are further devolvement of the corresponding results in the scale of the Lebesgue space $L^p(\mathbb {R}^n)$![]() with $p\in (1,\,\infty )$
with $p\in (1,\,\infty )$![]() established by Dong and Kim [Reference Dong and Kim7] in the endpoint cases of $p=1$
established by Dong and Kim [Reference Dong and Kim7] in the endpoint cases of $p=1$![]() and $p=\infty$
and $p=\infty$![]() .
.
In particular, when $a$![]() is a fixed appropriate constant, the corresponding operator $L$
is a fixed appropriate constant, the corresponding operator $L$![]() is just the fractional Laplacian $-(-\Delta )^{\sigma /2}$
is just the fractional Laplacian $-(-\Delta )^{\sigma /2}$![]() . It is said that the function $u$
. It is said that the function $u$![]() is a solution of the equation (1.2), if (1.2) holds true in the sense of almost everywhere.
is a solution of the equation (1.2), if (1.2) holds true in the sense of almost everywhere.
Denote by ${\mathcal {S}}({\mathbb {R}^n})$![]() the classical Schwartz function space, that is, the set of all infinitely differentiable functions satisfying that all derivatives decrease rapidly at infinity, and by ${\mathcal {S}}'({\mathbb {R}^n})$
the classical Schwartz function space, that is, the set of all infinitely differentiable functions satisfying that all derivatives decrease rapidly at infinity, and by ${\mathcal {S}}'({\mathbb {R}^n})$![]() its dual space (namely, the space of all tempered distributions).
its dual space (namely, the space of all tempered distributions).
Recall that, for any given $\alpha \in (0,\,\infty )$![]() , the Bessel potential operator $J_\alpha$
, the Bessel potential operator $J_\alpha$![]() on ${\mathcal {S}}'({\mathbb {R}^n})$
on ${\mathcal {S}}'({\mathbb {R}^n})$![]() is defined by, for any $f\in {\mathcal {S}}'({\mathbb {R}^n})$
is defined by, for any $f\in {\mathcal {S}}'({\mathbb {R}^n})$![]() and $\xi \in {\mathbb {R}^n}$
and $\xi \in {\mathbb {R}^n}$![]() ,
,
(see, for instance, [Reference Grafakos14, definition 1.2.4]). Here and hereafter, ${\mathcal {F}}$![]() and ${\mathcal {F}}^{-1}$
and ${\mathcal {F}}^{-1}$![]() , respectively, denote the Fourier transform and the inverse Fourier transform. Moreover, for any given $\alpha \in (0,\,\infty )$
, respectively, denote the Fourier transform and the inverse Fourier transform. Moreover, for any given $\alpha \in (0,\,\infty )$![]() , the Riesz potential operator $I_\alpha$
, the Riesz potential operator $I_\alpha$![]() on ${\mathcal {S}}'({\mathbb {R}^n})$
on ${\mathcal {S}}'({\mathbb {R}^n})$![]() is defined by, for any $f\in {\mathcal {S}}'({\mathbb {R}^n})$
is defined by, for any $f\in {\mathcal {S}}'({\mathbb {R}^n})$![]() and $\xi \in {\mathbb {R}^n}$
and $\xi \in {\mathbb {R}^n}$![]() ,
,
(see, for instance, [Reference Grafakos14, definition 1.2.1]). It is worth pointing out that, when $\alpha \in (0,\,\infty )$![]() , $|\cdot |^{-\alpha }$
, $|\cdot |^{-\alpha }$![]() has singularity at the origin. Therefore, $I_\alpha$
has singularity at the origin. Therefore, $I_\alpha$![]() can only be defined on the space of tempered distributions modulo polynomials. Moreover, for any $\alpha \in (0,\,\infty )$
can only be defined on the space of tempered distributions modulo polynomials. Moreover, for any $\alpha \in (0,\,\infty )$![]() and $u\in {\mathcal {S}}'({\mathbb {R}^n})$
and $u\in {\mathcal {S}}'({\mathbb {R}^n})$![]() , the fractional derivative of $u$
, the fractional derivative of $u$![]() with order $\alpha$
with order $\alpha$![]() is defined by
is defined by
Furthermore, for any given $\alpha \in (0,\,2)$![]() and $u\in {\mathcal {S}}({\mathbb {R}^n})$
and $u\in {\mathcal {S}}({\mathbb {R}^n})$![]() , the fractional derivative of $u$
, the fractional derivative of $u$![]() with order $\alpha$
with order $\alpha$![]() has the equivalent definition
has the equivalent definition

where
$\Gamma$![]() is the Gamma function, and $\mathrm {P.V.}$
is the Gamma function, and $\mathrm {P.V.}$![]() denotes the integral is taken according to the Cauchy principal value sense. It is worth pointing out that (1.3) is well defined for any $u\in C^2_b({\mathbb {R}^n})$
denotes the integral is taken according to the Cauchy principal value sense. It is worth pointing out that (1.3) is well defined for any $u\in C^2_b({\mathbb {R}^n})$![]() (the set of all $2$
(the set of all $2$![]() -times continuously differentiable bounded functions) (see, for instance, [Reference Garofalo13]).
-times continuously differentiable bounded functions) (see, for instance, [Reference Garofalo13]).
For any given $\alpha \in (0,\,\infty )$![]() and function space $X$
and function space $X$![]() on ${\mathbb {R}^n}$
on ${\mathbb {R}^n}$![]() , the Sobolev spaces based on $X$
, the Sobolev spaces based on $X$![]() , $J_\alpha (X)$
, $J_\alpha (X)$![]() and $I_\alpha (X)$
and $I_\alpha (X)$![]() , are defined by the image of $X$
, are defined by the image of $X$![]() under $J_\alpha$
under $J_\alpha$![]() and $I_\alpha$
and $I_\alpha$![]() , respectively. Furthermore, for any $u\in J_\alpha (X)$
, respectively. Furthermore, for any $u\in J_\alpha (X)$![]() [or $u\in I_\alpha (X)$
[or $u\in I_\alpha (X)$![]() ], the (quasi-)norm of $u$
], the (quasi-)norm of $u$![]() is given by $\|u\|_{J_\alpha (X)}:=\|J_\alpha (u)\|_X$
is given by $\|u\|_{J_\alpha (X)}:=\|J_\alpha (u)\|_X$![]() [or $\|u\|_{I_\alpha (X)}:=\|I_\alpha (u)\|_X$
[or $\|u\|_{I_\alpha (X)}:=\|I_\alpha (u)\|_X$![]() ]. By this, we find that, for any function $u\in I_\alpha (X)$
]. By this, we find that, for any function $u\in I_\alpha (X)$![]() , the fractional derivative $\partial ^\alpha u\in X$
, the fractional derivative $\partial ^\alpha u\in X$![]() .
.
Moreover, recall that the Riesz transform $R_j$![]() , for any given $j\in \{1,\,\ldots,\,n\}$
, for any given $j\in \{1,\,\ldots,\,n\}$![]() , is defined by, for any $f\in {\mathcal {S}}({\mathbb {R}^n})$
, is defined by, for any $f\in {\mathcal {S}}({\mathbb {R}^n})$![]() ,
,
where $c_n:=\Gamma (\frac {n+1}{2})\pi ^{-\frac {n+1}{2}}$![]() (see, for instance, [Reference Stein26, Reference Stein27]). When $n=1$
(see, for instance, [Reference Stein26, Reference Stein27]). When $n=1$![]() , the corresponding operator is known as the Hilbert transform.
, the corresponding operator is known as the Hilbert transform.
The classical Hardy space $H^1({\mathbb {R}^n})$![]() is defined to be the set of all $f\in L^1({\mathbb {R}^n})$
is defined to be the set of all $f\in L^1({\mathbb {R}^n})$![]() such that $R_jf\in L^1({\mathbb {R}^n})$
such that $R_jf\in L^1({\mathbb {R}^n})$![]() for any $j\in \{1,\,\ldots,\,n\}$
for any $j\in \{1,\,\ldots,\,n\}$![]() , with the norm
, with the norm

(see, for instance, [Reference Stein27]). Furthermore, denote by $L^1_{\mathop{\mathrm {loc}}}({\mathbb {R}^n})$![]() the set of all locally integrable functions on ${\mathbb {R}^n}$
the set of all locally integrable functions on ${\mathbb {R}^n}$![]() . Let $f\in L^1_{\mathop{\mathrm {loc}}}({\mathbb {R}^n})$
. Let $f\in L^1_{\mathop{\mathrm {loc}}}({\mathbb {R}^n})$![]() . It is said that $f$
. It is said that $f$![]() belongs to the $\mathrm {BMO}$
belongs to the $\mathrm {BMO}$![]() (bounded mean oscillation) space ${\mathop {\mathrm {BMO}}}({\mathbb {R}^n})$
(bounded mean oscillation) space ${\mathop {\mathrm {BMO}}}({\mathbb {R}^n})$![]() , if
, if
where the supremum is taken over all balls $B$![]() of ${\mathbb {R}^n}$
of ${\mathbb {R}^n}$![]() and $f_B:=\frac {1}{|B|}\int _Bf(y)\,{\rm d}y$
and $f_B:=\frac {1}{|B|}\int _Bf(y)\,{\rm d}y$![]() (see, for instance, [Reference Grafakos14, Reference John and Nirenberg15, Reference Stein27]). Recall that $\|\cdot \|_{{\mathop {\mathrm {BMO}}}({\mathbb {R}^n})}$
(see, for instance, [Reference Grafakos14, Reference John and Nirenberg15, Reference Stein27]). Recall that $\|\cdot \|_{{\mathop {\mathrm {BMO}}}({\mathbb {R}^n})}$![]() is only a semi-norm and ${\mathop {\mathrm {BMO}}}({\mathbb {R}^n})$
is only a semi-norm and ${\mathop {\mathrm {BMO}}}({\mathbb {R}^n})$![]() modulo constants is a Banach space. To make ${\mathop {\mathrm {BMO}}}({\mathbb {R}^n})$
modulo constants is a Banach space. To make ${\mathop {\mathrm {BMO}}}({\mathbb {R}^n})$![]() itself a Banach space, for $f\in {\mathop {\mathrm {BMO}}}({\mathbb {R}^n})$
itself a Banach space, for $f\in {\mathop {\mathrm {BMO}}}({\mathbb {R}^n})$![]() , we may consider the norm
, we may consider the norm

which is useful to consider the pointwise multipliers of ${\mathop {\mathrm {BMO}}}({\mathbb {R}^n})$![]() , where $B_1(\mathbf {0})$
, where $B_1(\mathbf {0})$![]() denotes the ball with the centre $\mathbf {0}$
denotes the ball with the centre $\mathbf {0}$![]() and the radius $1$
and the radius $1$![]() . It is known that the Hardy space $H^1({\mathbb {R}^n})$
. It is known that the Hardy space $H^1({\mathbb {R}^n})$![]() and the ${\mathop {\mathrm {BMO}}}$
and the ${\mathop {\mathrm {BMO}}}$![]() space ${\mathop {\mathrm {BMO}}}({\mathbb {R}^n})$
space ${\mathop {\mathrm {BMO}}}({\mathbb {R}^n})$![]() , respectively, are appropriate substitutes of the Lebesgue spaces $L^1({\mathbb {R}^n})$
, respectively, are appropriate substitutes of the Lebesgue spaces $L^1({\mathbb {R}^n})$![]() and $L^\infty ({\mathbb {R}^n})$
and $L^\infty ({\mathbb {R}^n})$![]() when studying the boundedness of some linear operators (see, for instance, [Reference Grafakos14, Reference Stein27–Reference Strichartz29]). Moreover, it is well known that the space ${\mathop {\mathrm {BMO}}}({\mathbb {R}^n})$
when studying the boundedness of some linear operators (see, for instance, [Reference Grafakos14, Reference Stein27–Reference Strichartz29]). Moreover, it is well known that the space ${\mathop {\mathrm {BMO}}}({\mathbb {R}^n})$![]() is the dual space of the Hardy space $H^1({\mathbb {R}^n})$
is the dual space of the Hardy space $H^1({\mathbb {R}^n})$![]() (see, for instance, [Reference Grafakos14, Reference Stein27]).
(see, for instance, [Reference Grafakos14, Reference Stein27]).
Non-local equations have aroused extensive research interest in recent years. The non-local equations of the form (1.2) naturally arise in the study of jump Lévy processes; they have extensive applications in many fields, such as, economics, physics and probability theory (see, for instance, [Reference Caffarelli and Silvestre3, Reference Cont and Tankov5, Reference Garofalo13, Reference Ros-Oton24]), and have been extensively studied (see, for instance, [Reference Caffarelli and Silvestre3, Reference Caffarelli and Silvestre4, Reference Dong, Jung and Kim6–Reference Dong and Liu11, Reference Karlsen, Petitta and Ulusoy16, Reference Mengesha, Schikorra and Yeepo18–Reference Mikulevičius and Pragarauskas20]).
The study of the boundedness of the non-local elliptic operator $L$![]() defined as in (1.1) can be founded in many existing literatures. In particular, if the kernel function $a$
defined as in (1.1) can be founded in many existing literatures. In particular, if the kernel function $a$![]() satisfies the lower and upper bounds condition, and also satisfies the cancellation condition when $\sigma =1$
satisfies the lower and upper bounds condition, and also satisfies the cancellation condition when $\sigma =1$![]() , Dong and Kim [Reference Dong and Kim7, Reference Dong and Kim8] obtained the boundedness of the operator $L$
, Dong and Kim [Reference Dong and Kim7, Reference Dong and Kim8] obtained the boundedness of the operator $L$![]() from the Sobolev space $J_\sigma (L^p({\mathbb {R}^n}))$
from the Sobolev space $J_\sigma (L^p({\mathbb {R}^n}))$![]() with $p\in (1,\,\infty )$
with $p\in (1,\,\infty )$![]() to $L^p({\mathbb {R}^n})$
to $L^p({\mathbb {R}^n})$![]() , and from the Lipschitz space $\Lambda ^{\alpha +\sigma }({\mathbb {R}^n})$
, and from the Lipschitz space $\Lambda ^{\alpha +\sigma }({\mathbb {R}^n})$![]() to $\Lambda ^{\alpha }({\mathbb {R}^n})$
to $\Lambda ^{\alpha }({\mathbb {R}^n})$![]() for any given $\alpha \in (0,\,\infty )$
for any given $\alpha \in (0,\,\infty )$![]() (see, for instance, [Reference Stein26, Reference Stein27] or § 2 below for the definition of the Lipschitz space). Afterwards, for the non-local operator associated with the $x$
(see, for instance, [Reference Stein26, Reference Stein27] or § 2 below for the definition of the Lipschitz space). Afterwards, for the non-local operator associated with the $x$![]() -dependent kernel $a(x,\,\cdot )$
-dependent kernel $a(x,\,\cdot )$![]() imposed on the Hölder continuity of $x$
imposed on the Hölder continuity of $x$![]() , by using the boundedness of the singular integral of convolution type on Lebesgue spaces $L^p({\mathbb {R}^n})$
, by using the boundedness of the singular integral of convolution type on Lebesgue spaces $L^p({\mathbb {R}^n})$![]() and the partition of unity argument, Mikulevičius and Pragarauskas [Reference Mikulevičius and Pragarauskas20] obtained the boundedness of the operator $L$
and the partition of unity argument, Mikulevičius and Pragarauskas [Reference Mikulevičius and Pragarauskas20] obtained the boundedness of the operator $L$![]() from the Sobolev space $J_\sigma (L^p({\mathbb {R}^n}))$
from the Sobolev space $J_\sigma (L^p({\mathbb {R}^n}))$![]() to $L^p({\mathbb {R}^n})$
to $L^p({\mathbb {R}^n})$![]() when $p\in (1,\,\infty )$
when $p\in (1,\,\infty )$![]() is sufficiently large. Recently, Dong et al. [Reference Dong, Jung and Kim6] removed the restriction on $p$
is sufficiently large. Recently, Dong et al. [Reference Dong, Jung and Kim6] removed the restriction on $p$![]() and extended the result established by Mikulevičius and Pragarauskas [Reference Mikulevičius and Pragarauskas20] to the weighted Lebesgue spaces $L^p_\omega({\mathbb {R}^n})$
and extended the result established by Mikulevičius and Pragarauskas [Reference Mikulevičius and Pragarauskas20] to the weighted Lebesgue spaces $L^p_\omega({\mathbb {R}^n})$![]() for any $p\in (1,\,\infty )$
for any $p\in (1,\,\infty )$![]() and $\omega \in A_p({\mathbb {R}^n})$
and $\omega \in A_p({\mathbb {R}^n})$![]() (the Muckenhoupt weight class). Furthermore, when the kernel also depends on the temporal variable, the boundedness of parabolic operators with local or non-local time derivatives was also considered in the existing literatures (see, for instance, [Reference Dong, Jung and Kim6, Reference Dong and Kim9–Reference Dong and Liu11, Reference Mikulevičius and Pragarauskas19, Reference Mikulevičius and Pragarauskas20]).
(the Muckenhoupt weight class). Furthermore, when the kernel also depends on the temporal variable, the boundedness of parabolic operators with local or non-local time derivatives was also considered in the existing literatures (see, for instance, [Reference Dong, Jung and Kim6, Reference Dong and Kim9–Reference Dong and Liu11, Reference Mikulevičius and Pragarauskas19, Reference Mikulevičius and Pragarauskas20]).
The research on the solvability and regularity of the solutions of non-local equations is even richer. In particular, for the fraction Laplacian problem $(-\Delta )^su=f$![]() in ${\mathbb {R}^n}$
in ${\mathbb {R}^n}$![]() , with $s\in (\frac 12,\,1)$
, with $s\in (\frac 12,\,1)$![]() and $f\in L^1({\mathbb {R}^n})$
and $f\in L^1({\mathbb {R}^n})$![]() , Karlsen et al. [Reference Karlsen, Petitta and Ulusoy16] proved the unique existence by a dual method, and the solution belonging to the local fractional Sobolev space $W^{1-(2-2s)/q,\,q}_{\mathop{\mathrm {loc}}}({\mathbb {R}^n})$
, Karlsen et al. [Reference Karlsen, Petitta and Ulusoy16] proved the unique existence by a dual method, and the solution belonging to the local fractional Sobolev space $W^{1-(2-2s)/q,\,q}_{\mathop{\mathrm {loc}}}({\mathbb {R}^n})$![]() with $q\in (1,\,\frac {n+2-2s}{n+1-2s})$
with $q\in (1,\,\frac {n+2-2s}{n+1-2s})$![]() . For the fractional Laplacian equation with $L^p({\mathbb {R}^n})$
. For the fractional Laplacian equation with $L^p({\mathbb {R}^n})$![]() -data, the existence and regularity of the solution can be obtained by the classical theory of pseudo-differential operators. However, for the general kernel $a$
-data, the existence and regularity of the solution can be obtained by the classical theory of pseudo-differential operators. However, for the general kernel $a$![]() , the theory of pseudo-differential operators is no longer effective. In [Reference Dong and Kim7], by using the boundedness of the non-local operator $L$
, the theory of pseudo-differential operators is no longer effective. In [Reference Dong and Kim7], by using the boundedness of the non-local operator $L$![]() as in (1.1) from $J_\sigma (L^p({\mathbb {R}^n}))$
as in (1.1) from $J_\sigma (L^p({\mathbb {R}^n}))$![]() to $L^p({\mathbb {R}^n})$
to $L^p({\mathbb {R}^n})$![]() with $p\in (1,\,\infty )$
with $p\in (1,\,\infty )$![]() , Dong and Kim proved that the solution of the non-local elliptic equation (1.2) with $f\in L^p({\mathbb {R}^n})\,(p\in (1,\,\infty ))$
, Dong and Kim proved that the solution of the non-local elliptic equation (1.2) with $f\in L^p({\mathbb {R}^n})\,(p\in (1,\,\infty ))$![]() exists and belongs to the Bessel potential space $J_\sigma (L^p({\mathbb {R}^n}))$
exists and belongs to the Bessel potential space $J_\sigma (L^p({\mathbb {R}^n}))$![]() . Moreover, in [Reference Dong and Kim8], by using the boundedness of the non-local operator $L$
. Moreover, in [Reference Dong and Kim8], by using the boundedness of the non-local operator $L$![]() from the Lipschitz space $\Lambda ^{\alpha +\sigma }({\mathbb {R}^n})$
from the Lipschitz space $\Lambda ^{\alpha +\sigma }({\mathbb {R}^n})$![]() to $\Lambda ^{\alpha }({\mathbb {R}^n})$
to $\Lambda ^{\alpha }({\mathbb {R}^n})$![]() with any given $\alpha \in (0,\,\infty )$
with any given $\alpha \in (0,\,\infty )$![]() and the method of continuity, Dong and Kim established the unique solvability of the equation (1.2) with any given $f\in \Lambda ^\alpha ({\mathbb {R}^n})$
and the method of continuity, Dong and Kim established the unique solvability of the equation (1.2) with any given $f\in \Lambda ^\alpha ({\mathbb {R}^n})$![]() , and also proved that the corresponding solution belongs to $\Lambda ^{\alpha +\sigma }({\mathbb {R}^n})$
, and also proved that the corresponding solution belongs to $\Lambda ^{\alpha +\sigma }({\mathbb {R}^n})$![]() . In the same paper [Reference Dong and Kim8], the solvability of the equation (1.2), with the kernel being $x$
. In the same paper [Reference Dong and Kim8], the solvability of the equation (1.2), with the kernel being $x$![]() -dependent, was also established. Furthermore, the solvability of the non-local parabolic equation, the Dirichlet problem of the non-local equation on domains and the semi-linear non-local equations also have been extensively studied in the existing literatures (see, for instance, [Reference Abdellaoui, Fernández, Leonori and Younes1, Reference Dong, Jung and Kim6, Reference Dong and Liu11, Reference Nowak22–Reference Ros-Oton and Serra25, Reference Wu, Huang and Zhou31]).
-dependent, was also established. Furthermore, the solvability of the non-local parabolic equation, the Dirichlet problem of the non-local equation on domains and the semi-linear non-local equations also have been extensively studied in the existing literatures (see, for instance, [Reference Abdellaoui, Fernández, Leonori and Younes1, Reference Dong, Jung and Kim6, Reference Dong and Liu11, Reference Nowak22–Reference Ros-Oton and Serra25, Reference Wu, Huang and Zhou31]).
Throughout this paper, we always assume that the kernel function $a$![]() satisfies the following assumption.
satisfies the following assumption.
Assumption 1.1 Let $\sigma \in (0,\,2)$![]() and $a$
and $a$![]() be a non-negative measurable function on ${\mathbb {R}^n}$
be a non-negative measurable function on ${\mathbb {R}^n}$![]() .
.
(i) There are positive constants $\mu$
 and $\Lambda$
and $\Lambda$ such that, for any $y\in {\mathbb {R}^n}$
such that, for any $y\in {\mathbb {R}^n}$ ,
\[ (2-\sigma)\mu\le a(y)\le(2-\sigma)\Lambda. \]
,
\[ (2-\sigma)\mu\le a(y)\le(2-\sigma)\Lambda. \]
(ii) If $\sigma =1$
 , then, for any $0< r< R$
, then, for any $0< r< R$ ,
\[ \displaystyle\int_{r\le|y|\le R}ya(y)\,\frac{{\rm d}y}{|y|^{n+1}}=0. \]
,
\[ \displaystyle\int_{r\le|y|\le R}ya(y)\,\frac{{\rm d}y}{|y|^{n+1}}=0. \]
Now, we give the main results of this paper.
Theorem 1.2 Let $n\ge 1$![]() , $\sigma \in (0,\,2)$
, $\sigma \in (0,\,2)$![]() , $p\in (1,\,\infty )$
, $p\in (1,\,\infty )$![]() , and the kernel function $a$
, and the kernel function $a$![]() satisfy assumption 1.1. Then the following two assertions hold true.
satisfy assumption 1.1. Then the following two assertions hold true.
(i) The operator $L$
 defined as in (1.1) is a continuous operator from $J_\sigma (L^p({\mathbb {R}^n}))\cap J_\sigma ({\mathop {\mathrm {BMO}}}({\mathbb {R}^n}))$
defined as in (1.1) is a continuous operator from $J_\sigma (L^p({\mathbb {R}^n}))\cap J_\sigma ({\mathop {\mathrm {BMO}}}({\mathbb {R}^n}))$ to ${\mathop {\mathrm {BMO}}}({\mathbb {R}^n}),$
to ${\mathop {\mathrm {BMO}}}({\mathbb {R}^n}),$ moreover, there exists a positive constant $C,$
moreover, there exists a positive constant $C,$ depending only on $n,$
depending only on $n,$ $\sigma,$
$\sigma,$ $\mu$
$\mu$ and $\Lambda,$
and $\Lambda,$ such that, for any $u\in J_\sigma (L^p({\mathbb {R}^n}))\cap J_\sigma ({\mathop {\mathrm {BMO}}}({\mathbb {R}^n})),$
such that, for any $u\in J_\sigma (L^p({\mathbb {R}^n}))\cap J_\sigma ({\mathop {\mathrm {BMO}}}({\mathbb {R}^n})),$ (1.6)\begin{equation} \|Lu\|_{{\mathop{\mathrm {BMO}}}({{{\mathbb{R}}}^n})}\le C\left\|\partial^\sigma u\right\|_{{\mathop{\mathrm {BMO}}}+({{{\mathbb{R}}}^n})}, \end{equation}where, for a function $f\in {\mathop {\mathrm {BMO}}}({\mathbb {R}^n}),$
(1.6)\begin{equation} \|Lu\|_{{\mathop{\mathrm {BMO}}}({{{\mathbb{R}}}^n})}\le C\left\|\partial^\sigma u\right\|_{{\mathop{\mathrm {BMO}}}+({{{\mathbb{R}}}^n})}, \end{equation}where, for a function $f\in {\mathop {\mathrm {BMO}}}({\mathbb {R}^n}),$
 $\|f\|_{{\mathop {\mathrm {BMO}}}+({\mathbb {R}^n})}$
$\|f\|_{{\mathop {\mathrm {BMO}}}+({\mathbb {R}^n})}$ is defined as in (1.5).
is defined as in (1.5).(ii) The operator $L$
 defined as in (1.1) is a continuous operator from $J_\sigma (H^1({\mathbb {R}^n}))$
defined as in (1.1) is a continuous operator from $J_\sigma (H^1({\mathbb {R}^n}))$ to $H^1({\mathbb {R}^n}),$
to $H^1({\mathbb {R}^n}),$ moreover, there exists a positive constant $C,$
moreover, there exists a positive constant $C,$ depending only on $n,$
depending only on $n,$ $\sigma,$
$\sigma,$ $\mu$
$\mu$ and $\Lambda,$
and $\Lambda,$ such that, for any $u\in J_\sigma (H^1({\mathbb {R}^n})),$
such that, for any $u\in J_\sigma (H^1({\mathbb {R}^n})),$ (1.7)\begin{equation} \|Lu\|_{H^1({{{\mathbb{R}}}^n})}\le C\left\|\partial^\sigma u\right\|_{H^1({{{\mathbb{R}}}^n})}. \end{equation}
(1.7)\begin{equation} \|Lu\|_{H^1({{{\mathbb{R}}}^n})}\le C\left\|\partial^\sigma u\right\|_{H^1({{{\mathbb{R}}}^n})}. \end{equation}
Remark 1.3 In theorem 1.2(i), we need a constraint that $u\in J_\sigma (L^p({\mathbb {R}^n}))$![]() for some $p\in (1,\,\infty )$
for some $p\in (1,\,\infty )$![]() to obtain (1.6). This additional condition is due to our proof method (see (3.20) and (3.21) below for the details). Precisely, let $\lambda \in (0,\,\infty )$
to obtain (1.6). This additional condition is due to our proof method (see (3.20) and (3.21) below for the details). Precisely, let $\lambda \in (0,\,\infty )$![]() be a constant and $f:=-(-\Delta )^{\sigma /2}u-\lambda u$
be a constant and $f:=-(-\Delta )^{\sigma /2}u-\lambda u$![]() . To guarantee that there exists a unique solution for the equation $Lw-\lambda w=f$
. To guarantee that there exists a unique solution for the equation $Lw-\lambda w=f$![]() which is important in the proof of theorem 1.2(i), we need to assume that $f\in L^p({\mathbb {R}^n})$
which is important in the proof of theorem 1.2(i), we need to assume that $f\in L^p({\mathbb {R}^n})$![]() for some $p\in (1,\,\infty )$
for some $p\in (1,\,\infty )$![]() . This leads to the constraint that $u\in J_\sigma (L^p({\mathbb {R}^n}))$
. This leads to the constraint that $u\in J_\sigma (L^p({\mathbb {R}^n}))$![]() for some $p\in (1,\,\infty )$
for some $p\in (1,\,\infty )$![]() . Meanwhile, because of the lack of the density of $L^p({\mathbb {R}^n})\cap {\mathop {\mathrm {BMO}}}({\mathbb {R}^n})$
. Meanwhile, because of the lack of the density of $L^p({\mathbb {R}^n})\cap {\mathop {\mathrm {BMO}}}({\mathbb {R}^n})$![]() in ${\mathop {\mathrm {BMO}}}({\mathbb {R}^n})$
in ${\mathop {\mathrm {BMO}}}({\mathbb {R}^n})$![]() , we could not replace the condition $u\in J_\sigma (L^p({\mathbb {R}^n}))\cap J_\sigma ({\mathop {\mathrm {BMO}}}({\mathbb {R}^n}))$
, we could not replace the condition $u\in J_\sigma (L^p({\mathbb {R}^n}))\cap J_\sigma ({\mathop {\mathrm {BMO}}}({\mathbb {R}^n}))$![]() with $u\in J_\sigma ({\mathop {\mathrm {BMO}}}({\mathbb {R}^n}))$
with $u\in J_\sigma ({\mathop {\mathrm {BMO}}}({\mathbb {R}^n}))$![]() by the method used in the proof of theorem 1.2(i).
by the method used in the proof of theorem 1.2(i).
Next, we show via a counterexample that, for any given $p\in (1,\,\infty )$![]() , $L^p({\mathbb {R}^n})\cap {\mathop {\mathrm {BMO}}}({\mathbb {R}^n})$
, $L^p({\mathbb {R}^n})\cap {\mathop {\mathrm {BMO}}}({\mathbb {R}^n})$![]() is not dense in ${\mathop {\mathrm {BMO}}}({\mathbb {R}^n})$
is not dense in ${\mathop {\mathrm {BMO}}}({\mathbb {R}^n})$![]() with respect to $\|\cdot \|_{{\mathop {\mathrm {BMO}}}({\mathbb {R}^n})}$
with respect to $\|\cdot \|_{{\mathop {\mathrm {BMO}}}({\mathbb {R}^n})}$![]() . Indeed, let $n=1$
. Indeed, let $n=1$![]() and $f_0(x):=\sin x$
and $f_0(x):=\sin x$![]() for any $x\in {\mathbb {R}}$
for any $x\in {\mathbb {R}}$![]() . Then $f_0\in L^\infty ({\mathbb {R}^n})$
. Then $f_0\in L^\infty ({\mathbb {R}^n})$![]() and hence $f_0\in {\mathop {\mathrm {BMO}}}({\mathbb {R}^n})$
and hence $f_0\in {\mathop {\mathrm {BMO}}}({\mathbb {R}^n})$![]() . Let $p\in (1,\,\infty )$
. Let $p\in (1,\,\infty )$![]() . Now, we prove that, for any $g\in L^p({\mathbb {R}^n})\cap {\mathop {\mathrm {BMO}}}({\mathbb {R}^n})$
. Now, we prove that, for any $g\in L^p({\mathbb {R}^n})\cap {\mathop {\mathrm {BMO}}}({\mathbb {R}^n})$![]() , $\|f_0-g\|_{{\mathop {\mathrm {BMO}}}({\mathbb {R}^n})}\ge \frac {2}{\pi }$
, $\|f_0-g\|_{{\mathop {\mathrm {BMO}}}({\mathbb {R}^n})}\ge \frac {2}{\pi }$![]() , which implies that $L^p({\mathbb {R}^n})\cap {\mathop {\mathrm {BMO}}}({\mathbb {R}^n})$
, which implies that $L^p({\mathbb {R}^n})\cap {\mathop {\mathrm {BMO}}}({\mathbb {R}^n})$![]() is not dense in ${\mathop {\mathrm {BMO}}}({\mathbb {R}^n})$
is not dense in ${\mathop {\mathrm {BMO}}}({\mathbb {R}^n})$![]() with respect to $\|\cdot \|_{{\mathop {\mathrm {BMO}}}({\mathbb {R}^n})}$
with respect to $\|\cdot \|_{{\mathop {\mathrm {BMO}}}({\mathbb {R}^n})}$![]() . For any $k\in {\mathbb {N}}$
. For any $k\in {\mathbb {N}}$![]() , let $I_k:=(k\pi,\,(k+2)\pi )$
, let $I_k:=(k\pi,\,(k+2)\pi )$![]() . Then, for any $k\in {\mathbb {N}}$
. Then, for any $k\in {\mathbb {N}}$![]() ,
,

Moreover, by $g\in L^p({\mathbb {R}})$![]() , we conclude that $\lim _{k\to \infty }\int _{k\pi }^{(k+2)\pi }|g|\,{\rm d}x=0$
, we conclude that $\lim _{k\to \infty }\int _{k\pi }^{(k+2)\pi }|g|\,{\rm d}x=0$![]() . Thus, letting $k\to \infty$
. Thus, letting $k\to \infty$![]() in (1.8), we find that, for any $g\in L^p({\mathbb {R}^n})\cap {\mathop {\mathrm {BMO}}}({\mathbb {R}^n})$
in (1.8), we find that, for any $g\in L^p({\mathbb {R}^n})\cap {\mathop {\mathrm {BMO}}}({\mathbb {R}^n})$![]() , $\|f_0-g\|_{{\mathop {\mathrm {BMO}}}({\mathbb {R}^n})}\ge~\frac {2}{\pi }$
, $\|f_0-g\|_{{\mathop {\mathrm {BMO}}}({\mathbb {R}^n})}\ge~\frac {2}{\pi }$![]() .
.
Theorem 1.4 Let $n\ge 1,$![]() $\lambda \in (0,\,\infty ),$
$\lambda \in (0,\,\infty ),$![]() $\sigma \in (0,\,2),$
$\sigma \in (0,\,2),$![]() $p\in (1,\,\infty ),$
$p\in (1,\,\infty ),$![]() and the kernel function $a$
and the kernel function $a$![]() satisfy assumption 1.1. Then the following two assertions hold true.
satisfy assumption 1.1. Then the following two assertions hold true.
(i) For any given $f\in {\mathop {\mathrm {BMO}}}({\mathbb {R}^n})\cap L^p({\mathbb {R}^n}),$
 the solution $u$
the solution $u$ of the equation (1.2) uniquely exists and, moreover, $u\in J_\sigma ({\mathop {\mathrm {BMO}}}({\mathbb {R}^n}))$
of the equation (1.2) uniquely exists and, moreover, $u\in J_\sigma ({\mathop {\mathrm {BMO}}}({\mathbb {R}^n}))$ and there exists a positive constant $C,$
and there exists a positive constant $C,$ depending only on $n,$
depending only on $n,$ $\sigma,$
$\sigma,$ $\mu$
$\mu$ and $\Lambda,$
and $\Lambda,$ such that
(1.9)\begin{equation} \lambda\|u\|_{{\mathop{\mathrm {BMO}}}({{{\mathbb{R}}}^n})}+\left\|\partial^\sigma u\right\|_{{\mathop{\mathrm {BMO}}}({{{\mathbb{R}}}^n})}\le C\|f\|_{{\mathop{\mathrm {BMO}}}+({{{\mathbb{R}}}^n})}, \end{equation}where, for any $f\in {\mathop {\mathrm {BMO}}}({\mathbb {R}^n}),$
such that
(1.9)\begin{equation} \lambda\|u\|_{{\mathop{\mathrm {BMO}}}({{{\mathbb{R}}}^n})}+\left\|\partial^\sigma u\right\|_{{\mathop{\mathrm {BMO}}}({{{\mathbb{R}}}^n})}\le C\|f\|_{{\mathop{\mathrm {BMO}}}+({{{\mathbb{R}}}^n})}, \end{equation}where, for any $f\in {\mathop {\mathrm {BMO}}}({\mathbb {R}^n}),$
 $\|f\|_{{\mathop {\mathrm {BMO}}}+({\mathbb {R}^n})}$
$\|f\|_{{\mathop {\mathrm {BMO}}}+({\mathbb {R}^n})}$ is defined as in (1.5).
is defined as in (1.5).(ii) For any given $f\in H^1({\mathbb {R}^n}),$
 the solution $u$
the solution $u$ of the equation (1.2) uniquely exists and, moreover, $u\in J_\sigma (H^1({\mathbb {R}^n}))$
of the equation (1.2) uniquely exists and, moreover, $u\in J_\sigma (H^1({\mathbb {R}^n}))$ and there exists a positive constant $C,$
and there exists a positive constant $C,$ depending only on $n,$
depending only on $n,$ $\sigma,$
$\sigma,$ $\mu$
$\mu$ and $\Lambda,$
and $\Lambda,$ such that
(1.10)\begin{equation} \lambda\|u\|_{H^1({{{\mathbb{R}}}^n})}+\left\|\partial^\sigma u\right\|_{H^1({{{\mathbb{R}}}^n})}\le C\|f\|_{H^1({{{\mathbb{R}}}^n})}. \end{equation}
such that
(1.10)\begin{equation} \lambda\|u\|_{H^1({{{\mathbb{R}}}^n})}+\left\|\partial^\sigma u\right\|_{H^1({{{\mathbb{R}}}^n})}\le C\|f\|_{H^1({{{\mathbb{R}}}^n})}. \end{equation}
Remark 1.5 (i) Let $\lambda \in (0,\,\infty )$![]() , $\sigma \in (0,\,2)$
, $\sigma \in (0,\,2)$![]() , $f\in L^\infty ({\mathbb {R}^n})\cap L^p({\mathbb {R}^n})$
, $f\in L^\infty ({\mathbb {R}^n})\cap L^p({\mathbb {R}^n})$![]() with some $p\in (1,\,\infty )$
with some $p\in (1,\,\infty )$![]() , and $u$
, and $u$![]() be the solution of the equation (1.2). By the maximum principle, it was proved in [Reference Dong and Kim8, theorem 1.1] that $\lambda \|u\|_{L^\infty ({\mathbb {R}^n})}\le \|f\|_{L^\infty ({\mathbb {R}^n})}$
be the solution of the equation (1.2). By the maximum principle, it was proved in [Reference Dong and Kim8, theorem 1.1] that $\lambda \|u\|_{L^\infty ({\mathbb {R}^n})}\le \|f\|_{L^\infty ({\mathbb {R}^n})}$![]() . From this and theorem 1.4, it follows that
. From this and theorem 1.4, it follows that
where $C$![]() is a positive constant depending only on $n$
is a positive constant depending only on $n$![]() , $\sigma$
, $\sigma$![]() , $\mu$
, $\mu$![]() and $\Lambda$
and $\Lambda$![]() .
.
(ii) When $\lambda =0$![]() in theorem 1.4, we could give a priori estimate for the equation $Lu=f$
in theorem 1.4, we could give a priori estimate for the equation $Lu=f$![]() in ${\mathbb {R}^n}$
in ${\mathbb {R}^n}$![]() . Indeed, if $u\in {\mathop {\mathrm {BMO}}}({\mathbb {R}^n})\cap L^p({\mathbb {R}^n})$
. Indeed, if $u\in {\mathop {\mathrm {BMO}}}({\mathbb {R}^n})\cap L^p({\mathbb {R}^n})$![]() is a solution of the equation $Lu=f$
is a solution of the equation $Lu=f$![]() with $f\in {\mathop {\mathrm {BMO}}}({\mathbb {R}^n})\cap L^p({\mathbb {R}^n})$
with $f\in {\mathop {\mathrm {BMO}}}({\mathbb {R}^n})\cap L^p({\mathbb {R}^n})$![]() for some $p\in (1,\,\infty )$
for some $p\in (1,\,\infty )$![]() , then, for any $\lambda \in (0,\,\infty )$
, then, for any $\lambda \in (0,\,\infty )$![]() , we have $Lu-\lambda u=f-\lambda u$
, we have $Lu-\lambda u=f-\lambda u$![]() . Since the constant $C$
. Since the constant $C$![]() in (1.9) is independent of $\lambda$
in (1.9) is independent of $\lambda$![]() , $u$
, $u$![]() and $f$
and $f$![]() , by taking $\lambda \to 0^+$
, by taking $\lambda \to 0^+$![]() , it follows that (1.9) holds true with $\lambda =0$
, it follows that (1.9) holds true with $\lambda =0$![]() . Similarly, if $u\in H^1({\mathbb {R}^n})$
. Similarly, if $u\in H^1({\mathbb {R}^n})$![]() is a solution of the equation $Lu=f$
is a solution of the equation $Lu=f$![]() with $f\in H^1({\mathbb {R}^n})$
with $f\in H^1({\mathbb {R}^n})$![]() , we also obtain that (1.10) holds true with $\lambda =0$
, we also obtain that (1.10) holds true with $\lambda =0$![]() .
.
(iii) The methods used in this paper to show theorems 1.2 and 1.4 are not effective to deal with the general case that the kernel function $a$![]() depends on both the variables $x$
depends on both the variables $x$![]() and $y$
and $y$![]() , considered as [Reference Dong, Jung and Kim6, Reference Dong and Liu11, Reference Mikulevičius and Pragarauskas20]. Indeed, in the proofs of theorems 1.2 and 1.4, we use the exchangeability that $(-\Delta )^{\sigma /2}L=L(-\Delta )^{\sigma /2}$
, considered as [Reference Dong, Jung and Kim6, Reference Dong and Liu11, Reference Mikulevičius and Pragarauskas20]. Indeed, in the proofs of theorems 1.2 and 1.4, we use the exchangeability that $(-\Delta )^{\sigma /2}L=L(-\Delta )^{\sigma /2}$![]() and $R_jL=LR_j$
and $R_jL=LR_j$![]() which plays a key role in the proofs of theorems 1.2 and 1.4, where the operator $L$
which plays a key role in the proofs of theorems 1.2 and 1.4, where the operator $L$![]() is as in (1.1) and $R_j$
is as in (1.1) and $R_j$![]() with $j\in \{1,\,\ldots,\,n\}$
with $j\in \{1,\,\ldots,\,n\}$![]() denotes the Riesz transform. However, these exchangeable properties may not hold true for the operator $L$
denotes the Riesz transform. However, these exchangeable properties may not hold true for the operator $L$![]() when the kernel function $a$
when the kernel function $a$![]() depends on both the variables $x$
depends on both the variables $x$![]() and $y$
and $y$![]() .
.
The remainder of this paper is organized as follows. In § 2, we recall the notions of the Bessel potential space and the Riesz potential space based on $L^p({\mathbb {R}^n})$![]() , $H^1({\mathbb {R}^n})$
, $H^1({\mathbb {R}^n})$![]() or ${\mathop {\mathrm {BMO}}}({\mathbb {R}^n})$
or ${\mathop {\mathrm {BMO}}}({\mathbb {R}^n})$![]() , and the Lipschitz–Zygmund space. Moreover, we also present the boundedness result of the singular integral operator on the Hardy space $H^1({\mathbb {R}^n})$
, and the Lipschitz–Zygmund space. Moreover, we also present the boundedness result of the singular integral operator on the Hardy space $H^1({\mathbb {R}^n})$![]() , and some important results established by Dong and Kim [Reference Dong and Kim7, Reference Dong and Kim8]. In § 3, we prove theorems 1.2 and 1.4. To prove theorem 1.2(i), the key step is to establish the mean oscillation estimates. This method was originated in [Reference Krylov17] and used to treat second-order elliptic and parabolic equations with $\mathrm {VMO}$
, and some important results established by Dong and Kim [Reference Dong and Kim7, Reference Dong and Kim8]. In § 3, we prove theorems 1.2 and 1.4. To prove theorem 1.2(i), the key step is to establish the mean oscillation estimates. This method was originated in [Reference Krylov17] and used to treat second-order elliptic and parabolic equations with $\mathrm {VMO}$![]() coefficients. Moreover, in [Reference Dong and Kim7, Reference Dong and Kim8, Reference Dong and Liu11], this method was further developed to treat non-local elliptic and parabolic equations. To show theorem 1.2(ii), we use the boundedness of the singular integral operator on the Hardy space $H^1({\mathbb {R}^n})$
coefficients. Moreover, in [Reference Dong and Kim7, Reference Dong and Kim8, Reference Dong and Liu11], this method was further developed to treat non-local elliptic and parabolic equations. To show theorem 1.2(ii), we use the boundedness of the singular integral operator on the Hardy space $H^1({\mathbb {R}^n})$![]() , which is motivated by [Reference Dong, Jung and Kim6]. Meanwhile, to prove theorem 1.4, we also use the method of mean oscillation estimates. Moreover, a duality argument is also used.
, which is motivated by [Reference Dong, Jung and Kim6]. Meanwhile, to prove theorem 1.4, we also use the method of mean oscillation estimates. Moreover, a duality argument is also used.
Finally, we make some conventions on notations. Throughout the whole paper, we always denote by $C$![]() a positive constant which is independent of the main parameters, but it may vary from line to line. The symbol $f\lesssim g$
a positive constant which is independent of the main parameters, but it may vary from line to line. The symbol $f\lesssim g$![]() means that $f\le Cg$
means that $f\le Cg$![]() . For any $x\in {\mathbb {R}^n}$
. For any $x\in {\mathbb {R}^n}$![]() and $r\in (0,\,\infty )$
and $r\in (0,\,\infty )$![]() , let $B_r(x)$
, let $B_r(x)$![]() be a ball with centre $x$
be a ball with centre $x$![]() and radius $r$
and radius $r$![]() . In particular, when $x={\mathbf 0}$
. In particular, when $x={\mathbf 0}$![]() (the origin of ${\mathbb {R}^n}$
(the origin of ${\mathbb {R}^n}$![]() ), we let $B_r:=B_r({\mathbf 0})$
), we let $B_r:=B_r({\mathbf 0})$![]() . We denote by ${\mathbb {N}}$
. We denote by ${\mathbb {N}}$![]() the set of all positive integers. Moreover, for an open set $\Omega \subset {\mathbb {R}^n}$
the set of all positive integers. Moreover, for an open set $\Omega \subset {\mathbb {R}^n}$![]() , we denote by $C^\infty _{\rm c}(\Omega )$
, we denote by $C^\infty _{\rm c}(\Omega )$![]() the set of all infinitely differentiable functions with compact supports on $\Omega$
the set of all infinitely differentiable functions with compact supports on $\Omega$![]() and by $C^\infty _{b}(\Omega )$
and by $C^\infty _{b}(\Omega )$![]() the set of all infinitely differentiable functions with bound derivatives on $\Omega$
the set of all infinitely differentiable functions with bound derivatives on $\Omega$![]() . For a multiindex $\gamma :=(\gamma _1,\,\ldots,\,\gamma _n)$
. For a multiindex $\gamma :=(\gamma _1,\,\ldots,\,\gamma _n)$![]() with each component $\gamma _i$
with each component $\gamma _i$![]() being a nonnegative integer, let $|\gamma |=\gamma _1+\cdots +\gamma _n$
being a nonnegative integer, let $|\gamma |=\gamma _1+\cdots +\gamma _n$![]() and, for any $|\gamma |$
and, for any $|\gamma |$![]() -th differentiable function $u$
-th differentiable function $u$![]() , set $D^\gamma u(x):=\frac {\partial ^{|\gamma |}u(x)}{\partial x_1^{\gamma _1}\cdots \partial x_n^{\gamma _n}}$
, set $D^\gamma u(x):=\frac {\partial ^{|\gamma |}u(x)}{\partial x_1^{\gamma _1}\cdots \partial x_n^{\gamma _n}}$![]() .
.
2. Preliminaries
In this section, we recall the notions of some function spaces, such as, the Bessel potential space and the Riesz potential space based on $L^p({\mathbb {R}^n})$![]() , $H^1({\mathbb {R}^n})$
, $H^1({\mathbb {R}^n})$![]() or ${\mathop {\mathrm {BMO}}}({\mathbb {R}^n})$
or ${\mathop {\mathrm {BMO}}}({\mathbb {R}^n})$![]() , and the Lipschitz–Zygmund space. Moreover, we also present the boundedness result of the singular integral operator on $H^1({\mathbb {R}^n})$
, and the Lipschitz–Zygmund space. Moreover, we also present the boundedness result of the singular integral operator on $H^1({\mathbb {R}^n})$![]() , and some important results established in [Reference Dong and Kim7, Reference Dong and Kim8].
, and some important results established in [Reference Dong and Kim7, Reference Dong and Kim8].
When $X$![]() is one of $L^p({\mathbb {R}^n})$
is one of $L^p({\mathbb {R}^n})$![]() , $H^1({\mathbb {R}^n})$
, $H^1({\mathbb {R}^n})$![]() or ${\mathop {\mathrm {BMO}}}({\mathbb {R}^n})$
or ${\mathop {\mathrm {BMO}}}({\mathbb {R}^n})$![]() , we recall the relations of the Sobolev spaces $J_\alpha (X)$
, we recall the relations of the Sobolev spaces $J_\alpha (X)$![]() and $I_\alpha (X)$
and $I_\alpha (X)$![]() as follows (see, for instance, [Reference Stein26, Reference Strichartz28, Reference Strichartz29]).
as follows (see, for instance, [Reference Stein26, Reference Strichartz28, Reference Strichartz29]).
Proposition 2.1 Let $\alpha \in (0,\,\infty )$![]() . Then the following properties hold true.
. Then the following properties hold true.
(i) For any $p\in (1,\,\infty ),$
 $J_\alpha (L^p({\mathbb {R}^n}))=L^p({\mathbb {R}^n})\cap I_\alpha (L^p({\mathbb {R}^n}))$
$J_\alpha (L^p({\mathbb {R}^n}))=L^p({\mathbb {R}^n})\cap I_\alpha (L^p({\mathbb {R}^n}))$ .
.(ii) $J_\alpha (H^1({\mathbb {R}^n}))=H^1({\mathbb {R}^n})\cap I_\alpha (H^1({\mathbb {R}^n}))$
 .
.(iii) $J_\alpha ({\mathop {\mathrm {BMO}}}({\mathbb {R}^n}))={\mathop {\mathrm {BMO}}}({\mathbb {R}^n})\cap I_\alpha ({\mathop {\mathrm {BMO}}}({\mathbb {R}^n}))$
 .
.
Let $u\in L^\infty ({\mathbb {R}^n})$![]() . We recall that the harmonic extension of $u$
. We recall that the harmonic extension of $u$![]() to ${\mathbb {R}}^{n+1}_+:={\mathbb {R}^n}\times (0,\,\infty )$
to ${\mathbb {R}}^{n+1}_+:={\mathbb {R}^n}\times (0,\,\infty )$![]() is defined by the convolution
is defined by the convolution
for any $y\in (0,\,\infty )$![]() , where $p(\cdot,\,y)$
, where $p(\cdot,\,y)$![]() is the classical Poisson kernel on ${\mathbb {R}}^{n+1}_+$
is the classical Poisson kernel on ${\mathbb {R}}^{n+1}_+$![]() . Let $\alpha \in (0,\,\infty )$
. Let $\alpha \in (0,\,\infty )$![]() and $\ell$
and $\ell$![]() be the smallest integer greater that $\alpha$
be the smallest integer greater that $\alpha$![]() . The Lipschitz–Zygmund space $\Lambda ^\alpha ({\mathbb {R}^n})$
. The Lipschitz–Zygmund space $\Lambda ^\alpha ({\mathbb {R}^n})$![]() is defined by
is defined by

where $D^\ell _y$![]() denotes the $\ell$
denotes the $\ell$![]() -th derivative with respect to $y$
-th derivative with respect to $y$![]() , which is equipped with the norm
, which is equipped with the norm
Let $\Omega \subset {\mathbb {R}^n}$![]() be an open set, $\alpha \in (0,\,\infty )$
be an open set, $\alpha \in (0,\,\infty )$![]() be a non-integer and $\ell$
be a non-integer and $\ell$![]() the largest integer smaller than $\alpha$
the largest integer smaller than $\alpha$![]() . Denote by $C^\alpha (\Omega )$
. Denote by $C^\alpha (\Omega )$![]() the set of all bounded continuous functions on $\Omega$
the set of all bounded continuous functions on $\Omega$![]() , with satisfying that
, with satisfying that
where $[\cdot ]_{C^{\alpha -\ell }(\Omega )}$![]() denotes the Hölder semi-norm, namely, for a function $g$
denotes the Hölder semi-norm, namely, for a function $g$![]() on $\Omega$
on $\Omega$![]() ,
,

Then we have the following properties of the Lipschitz–Zygmund space (see, for instance, [Reference Stein26, chapter V] and [Reference Stein27, chapter VI]).
Proposition 2.2 Let $\alpha \in (0,\,\infty )$![]() and $\Lambda ^\alpha ({\mathbb {R}^n})$
and $\Lambda ^\alpha ({\mathbb {R}^n})$![]() be the Lipschitz–Zygmund space on ${\mathbb {R}^n}$
be the Lipschitz–Zygmund space on ${\mathbb {R}^n}$![]() .
.
(i) For any $0<\alpha _1<\alpha _2<\infty,$
 $\Lambda ^{\alpha _2}({\mathbb {R}^n})\subsetneq \Lambda ^{\alpha _1}({\mathbb {R}^n})$
$\Lambda ^{\alpha _2}({\mathbb {R}^n})\subsetneq \Lambda ^{\alpha _1}({\mathbb {R}^n})$ .
.(ii) If $\alpha$
 is a non-integer, then $\Lambda ^\alpha ({\mathbb {R}^n})=C^\alpha ({\mathbb {R}^n})$
is a non-integer, then $\Lambda ^\alpha ({\mathbb {R}^n})=C^\alpha ({\mathbb {R}^n})$ .
.(iii) If $\alpha \in (0,\,2),$
 then
\[ \|u\|_{\Lambda^\alpha({{{\mathbb{R}}}^n})}=\|u\|_{L^\infty({{{\mathbb{R}}}^n})} +\sup_{|h|>0}|h|^{-\alpha}\|u({\cdot}{+}h)+u({\cdot}{-}h)-2u({\cdot})\|_{L^\infty({{{\mathbb{R}}}^n})}. \]
then
\[ \|u\|_{\Lambda^\alpha({{{\mathbb{R}}}^n})}=\|u\|_{L^\infty({{{\mathbb{R}}}^n})} +\sup_{|h|>0}|h|^{-\alpha}\|u({\cdot}{+}h)+u({\cdot}{-}h)-2u({\cdot})\|_{L^\infty({{{\mathbb{R}}}^n})}. \]
The following is the known result of the boundless of the singular integral operator on $H^1({\mathbb {R}^n})$![]() (see, for instance, [Reference Stein27, chapter III, theorem 3]).
(see, for instance, [Reference Stein27, chapter III, theorem 3]).
Lemma 2.3 Let $T$![]() be a singular integral operator on ${\mathbb {R}^n}$
be a singular integral operator on ${\mathbb {R}^n}$![]() . Assume that there exists a kernel function $K$
. Assume that there exists a kernel function $K$![]() such that, for any $f\in L^2({\mathbb {R}^n})$
such that, for any $f\in L^2({\mathbb {R}^n})$![]() with compact support,
with compact support,
holds true for any $x$![]() outside the support of $f$
outside the support of $f$![]() . Assume further that there exists a positive constant $A$
. Assume further that there exists a positive constant $A$![]() such that, for any $y\neq 0,$
such that, for any $y\neq 0,$![]()
and, for any $f\in L^2({\mathbb {R}^n}),$![]()
Then there exists a positive constant $C$![]() depending only on the constant $A$
depending only on the constant $A$![]() such that, for any $f\in H^1({\mathbb {R}^n}),$
such that, for any $f\in H^1({\mathbb {R}^n}),$![]()
The following conclusions were established in [Reference Dong and Kim7, Reference Dong and Kim8].
Lemma 2.4 [Reference Dong and Kim7, theorem 2.1]
Let $p\in (1,\,\infty ),$![]() $\lambda \in [0,\,\infty ),$
$\lambda \in [0,\,\infty ),$![]() $\sigma \in (0,\,2),$
$\sigma \in (0,\,2),$![]() and the kernel function $a$
and the kernel function $a$![]() satisfy assumption 1.1. Then the operator $L$
satisfy assumption 1.1. Then the operator $L$![]() defined as in (1.1) is a continuous operator from $J_\sigma (L^p({\mathbb {R}^n}))$
defined as in (1.1) is a continuous operator from $J_\sigma (L^p({\mathbb {R}^n}))$![]() to $L^p({\mathbb {R}^n})$
to $L^p({\mathbb {R}^n})$![]() and there exists a positive constant $C,$
and there exists a positive constant $C,$![]() depending only on $n,$
depending only on $n,$![]() $p,$
$p,$![]() $\sigma,$
$\sigma,$![]() $\mu$
$\mu$![]() and $\Lambda,$
and $\Lambda,$![]() such that
such that
Moreover, for any $\lambda \in (0,\,\infty )$![]() and $f\in L^p({\mathbb {R}^n}),$
and $f\in L^p({\mathbb {R}^n}),$![]() there exists a unique solution $u\in J_\sigma (L^p({\mathbb {R}^n}))$
there exists a unique solution $u\in J_\sigma (L^p({\mathbb {R}^n}))$![]() for the equation (1.2) , and there exists a positive constant $C,$
for the equation (1.2) , and there exists a positive constant $C,$![]() depending only on $n,$
depending only on $n,$![]() $p,$
$p,$![]() $\sigma,$
$\sigma,$![]() $\mu$
$\mu$![]() and $\Lambda,$
and $\Lambda,$![]() such that
such that
Lemma 2.5 [Reference Dong and Kim8, theorem 1.3]
Let $\alpha \in (0,\,\infty ),$![]() $\lambda \in (0,\,\infty ),$
$\lambda \in (0,\,\infty ),$![]() $\sigma \in (0,\,2),$
$\sigma \in (0,\,2),$![]() and the kernel function $a$
and the kernel function $a$![]() satisfy assumption 1.1. Then the operator $L-\lambda$
satisfy assumption 1.1. Then the operator $L-\lambda$![]() is a continuous operator from $\Lambda ^{\alpha +\sigma }({\mathbb {R}^n})$
is a continuous operator from $\Lambda ^{\alpha +\sigma }({\mathbb {R}^n})$![]() to $\Lambda ^\alpha ({\mathbb {R}^n}),$
to $\Lambda ^\alpha ({\mathbb {R}^n}),$![]() where $L$
where $L$![]() is as in (1.1). Moreover, for any $f\in \Lambda ^\alpha ({\mathbb {R}^n}),$
is as in (1.1). Moreover, for any $f\in \Lambda ^\alpha ({\mathbb {R}^n}),$![]() there exists a unique solution $u\in \Lambda ^{\alpha +\sigma }({\mathbb {R}^n})$
there exists a unique solution $u\in \Lambda ^{\alpha +\sigma }({\mathbb {R}^n})$![]() for the equation (1.2), and there exists a positive constant $C,$
for the equation (1.2), and there exists a positive constant $C,$![]() depending only on $n,$
depending only on $n,$![]() $\sigma,$
$\sigma,$![]() $\mu,$
$\mu,$![]() $\Lambda,$
$\Lambda,$![]() $\lambda$
$\lambda$![]() and $\alpha,$
and $\alpha,$![]() such that
such that
3. Proofs of theorems 1.2 and 1.4
In this section, we prove theorems 1.2 and 1.4. Assume that $\sigma \in (0,\,2)$![]() . Throughout this paper, we always assume that $\omega (x):=\frac {1}{1+|x|^{n+\sigma }}$
. Throughout this paper, we always assume that $\omega (x):=\frac {1}{1+|x|^{n+\sigma }}$![]() for any $x\in {\mathbb {R}^n}$
for any $x\in {\mathbb {R}^n}$![]() and
and
Moreover, for an open set $\Omega \subset {\mathbb {R}^n}$![]() , it is said that a function $f\in C^2_{\mathop{\mathrm {loc}}}(\Omega )$
, it is said that a function $f\in C^2_{\mathop{\mathrm {loc}}}(\Omega )$![]() , if, for any $\phi \in C^\infty _{\rm c}(\Omega )$
, if, for any $\phi \in C^\infty _{\rm c}(\Omega )$![]() , $\phi f\in C^2_{\rm c}(\Omega )$
, $\phi f\in C^2_{\rm c}(\Omega )$![]() (the set of all 2-th continuous differentiable functions with compact supports).
(the set of all 2-th continuous differentiable functions with compact supports).
We first recall the following property of the space ${\mathop {\mathrm {BMO}}}({\mathbb {R}^n})$![]() (see, for instance, [Reference Grafakos14, proposition 3.1.5]).
(see, for instance, [Reference Grafakos14, proposition 3.1.5]).
Proposition 3.1 Let $f\in {\mathop {\mathrm {BMO}}}({\mathbb {R}^n})$![]() . Then, for any $\delta \in (0,\,\infty ),$
. Then, for any $\delta \in (0,\,\infty ),$![]() there exists a positive constant $C,$
there exists a positive constant $C,$![]() depending only on $n$
depending only on $n$![]() and $\delta,$
and $\delta,$![]() such that, for any $x_0\in {\mathbb {R}^n}$
such that, for any $x_0\in {\mathbb {R}^n}$![]() and $R\in (0,\,\infty ),$
and $R\in (0,\,\infty ),$![]()
Now, we need the following lemma 3.2, which was established in [Reference Dong and Kim7, corollary 4.3].
Lemma 3.2 Let $\lambda \in [0,\,\infty ),$![]() $\sigma \in (0,\,2),$
$\sigma \in (0,\,2),$![]() $f\in L^\infty (B_1),$
$f\in L^\infty (B_1),$![]() and $u\in C^2_{\mathop{\mathrm {loc}}}(B_1)\cap L^1({\mathbb {R}^n},\,\omega )$
and $u\in C^2_{\mathop{\mathrm {loc}}}(B_1)\cap L^1({\mathbb {R}^n},\,\omega )$![]() be a solution of
be a solution of
in $B_1,$![]() where the operator $L$
where the operator $L$![]() is as in (1.1) and the kernel function $a$
is as in (1.1) and the kernel function $a$![]() satisfies assumption 1.1. Then, for any $\alpha \in (0,\,\min \{1,\,\sigma \}),$
satisfies assumption 1.1. Then, for any $\alpha \in (0,\,\min \{1,\,\sigma \}),$![]() there exists a positive constant $C,$
there exists a positive constant $C,$![]() depending only $n,$
depending only $n,$![]() $\sigma,$
$\sigma,$![]() $\mu,$
$\mu,$![]() $\Lambda,$
$\Lambda,$![]() and $\alpha,$
and $\alpha,$![]() such that
such that
where $\mathrm {osc}_{B_1} f:=\sup _{x,\,y\in B_1}|f(x)-f(y)|$![]() .
.
Moreover, as a corollary of lemma 3.2, we have the following lemma 3.3, which was obtained in [Reference Dong and Kim8, proposition 1].
Lemma 3.3 Let $\lambda \in [0,\,\infty ),$![]() $\sigma \in (0,\,2),$
$\sigma \in (0,\,2),$![]() $f\in L^\infty (B_1)$
$f\in L^\infty (B_1)$![]() and $u\in C^2_{\mathop{\mathrm {loc}}}(B_1)\cap L^\infty ({\mathbb {R}^n})$
and $u\in C^2_{\mathop{\mathrm {loc}}}(B_1)\cap L^\infty ({\mathbb {R}^n})$![]() be a solution of
be a solution of
in $B_1,$![]() where $L$
where $L$![]() is as in lemma 3.2. Then, for any $\alpha \in (0,\,\min \{1,\,\sigma \}),$
is as in lemma 3.2. Then, for any $\alpha \in (0,\,\min \{1,\,\sigma \}),$![]() there exists a positive constant $C,$
there exists a positive constant $C,$![]() depending only $n,$
depending only $n,$![]() $\sigma,$
$\sigma,$![]() $\mu,$
$\mu,$![]() $\Lambda$
$\Lambda$![]() and $\alpha,$
and $\alpha,$![]() such that
such that
where $(u)_{B_1}:=\frac {1}{|B_1|}\int _{B_1}u(x)\,{\rm d}x$![]() .
.
Lemma 3.4 Let $\lambda \in [0,\,\infty )$![]() , $\sigma \in (0,\,2),$
, $\sigma \in (0,\,2),$![]() $f\in C^\infty _{\mathop{\mathrm {loc}}}({\mathbb {R}^n})\cap {\mathop {\mathrm {BMO}}}({\mathbb {R}^n})$
$f\in C^\infty _{\mathop{\mathrm {loc}}}({\mathbb {R}^n})\cap {\mathop {\mathrm {BMO}}}({\mathbb {R}^n})$![]() satisfy that $f=0$
satisfy that $f=0$![]() in $B_2$
in $B_2$![]() , and $u\in J_\sigma (L^2({\mathbb {R}^n}))\cap C^\infty _b({\mathbb {R}^n})$
, and $u\in J_\sigma (L^2({\mathbb {R}^n}))\cap C^\infty _b({\mathbb {R}^n})$![]() be a solution of
be a solution of
in ${\mathbb {R}^n},$![]() where $L$
where $L$![]() is as in lemma 3.2. Then, for any $\alpha \in (0,\,\min \{1,\,\sigma \}),$
is as in lemma 3.2. Then, for any $\alpha \in (0,\,\min \{1,\,\sigma \}),$![]() there exists a positive constant $C,$
there exists a positive constant $C,$![]() depending only on $n,$
depending only on $n,$![]() $\sigma,$
$\sigma,$![]() $\mu,$
$\mu,$![]() $\Lambda$
$\Lambda$![]() and $\alpha,$
and $\alpha,$![]() such that
such that
and

Proof. By lemma 3.3 and the assumption that $f=0$![]() in $B_2$
in $B_2$![]() , we find that (3.4) holds true. Now, we show (3.5). Applying $(-\Delta )^{\sigma /2}$
, we find that (3.4) holds true. Now, we show (3.5). Applying $(-\Delta )^{\sigma /2}$![]() to both sides of (3.3), we conclude that
to both sides of (3.3), we conclude that
For any $x\in B_1$![]() , we have $f(x)=0$
, we have $f(x)=0$![]() and, if $y\in B_{1/2}$
and, if $y\in B_{1/2}$![]() , then $f(x+y)=0$
, then $f(x+y)=0$![]() . By this, proposition 3.1, and the fact that, for any $x\in B_1$
. By this, proposition 3.1, and the fact that, for any $x\in B_1$![]() , $(f)_{B_{1/2}(x)}=0$
, $(f)_{B_{1/2}(x)}=0$![]() , we find that, for any $x\in B_1$
, we find that, for any $x\in B_1$![]() ,
,

which, combined with the fact that $\mathrm {osc}_{B_1}(-\Delta )^{\sigma /2}f\le 2\|(-\Delta )^{\sigma /2}f\|_{L^\infty (B_1)}$![]() and lemma 3.3, further implies that (3.5) holds true. This finishes the proof of lemma 3.4.
and lemma 3.3, further implies that (3.5) holds true. This finishes the proof of lemma 3.4.
Then, by lemma 3.4 and a scaling and shifting the coordinates argument, we obtain the following lemma.
Lemma 3.5 Let $\lambda \in [0,\,\infty ),$![]() $\sigma \in (0,\,2),$
$\sigma \in (0,\,2),$![]() $k\in [2,\,\infty ),$
$k\in [2,\,\infty ),$![]() $f\in C^\infty _{\mathop{\mathrm {loc}}}({\mathbb {R}^n})\cap {\mathop {\mathrm {BMO}}}({\mathbb {R}^n})$
$f\in C^\infty _{\mathop{\mathrm {loc}}}({\mathbb {R}^n})\cap {\mathop {\mathrm {BMO}}}({\mathbb {R}^n})$![]() satisfy that $f=0$
satisfy that $f=0$![]() in $B_{2kr}(x_0)$
in $B_{2kr}(x_0)$![]() for some $x_0\in {\mathbb {R}^n}$
for some $x_0\in {\mathbb {R}^n}$![]() and $r\in (0,\,\infty ),$
and $r\in (0,\,\infty ),$![]() and $u\in J_\sigma (L^2({\mathbb {R}^n}))\cap C^\infty _b({\mathbb {R}^n})$
and $u\in J_\sigma (L^2({\mathbb {R}^n}))\cap C^\infty _b({\mathbb {R}^n})$![]() be a solution of
be a solution of
in ${\mathbb {R}^n},$![]() where $L$
where $L$![]() is as in lemma 3.2. Then, for any $\alpha \in (0,\,\min \{1,\,\sigma \}),$
is as in lemma 3.2. Then, for any $\alpha \in (0,\,\min \{1,\,\sigma \}),$![]() there exists a positive constant $C,$
there exists a positive constant $C,$![]() depending only on $n,$
depending only on $n,$![]() $\sigma,$
$\sigma,$![]() $\mu,$
$\mu,$![]() $\Lambda$
$\Lambda$![]() and $\alpha,$
and $\alpha,$![]() such that
such that
and

Proof. Let $R:=kr$![]() , $U(x):=u(Rx+x_0)$
, $U(x):=u(Rx+x_0)$![]() , and $F(x):=R^\sigma f(Rx+x_0)$
, and $F(x):=R^\sigma f(Rx+x_0)$![]() . Then, we conclude that $U$
. Then, we conclude that $U$![]() satisfies the equation
satisfies the equation
in ${\mathbb {R}^n}$![]() , where $F(x)=0$
, where $F(x)=0$![]() in $B_2$
in $B_2$![]() and $L_1$
and $L_1$![]() is the nonlocal operator with the coefficient $a_1(\cdot )=a(R\cdot )$
is the nonlocal operator with the coefficient $a_1(\cdot )=a(R\cdot )$![]() . Moreover, it is easy to find that $a_1$
. Moreover, it is easy to find that $a_1$![]() also satisfies assumption 1.1.
also satisfies assumption 1.1.
Therefore, from lemma 3.4 and a change of variables, it follows that
and

In addition, for any $k\in [2,\,\infty )$![]() and any function $g\in C^\alpha (B_{kr/2}(x_0))$
and any function $g\in C^\alpha (B_{kr/2}(x_0))$![]() , we have
, we have

which, together with (3.8) and (3.9), further implies that (3.6) and (3.7) hold true. This finishes the proof of lemma 3.5.
Lemma 3.6 Let $\sigma \in (0,\,2),$![]() $\lambda \in (0,\,\infty ),$
$\lambda \in (0,\,\infty ),$![]() $k\in [2,\,\infty ),$
$k\in [2,\,\infty ),$![]() $f\in C^\infty _{\mathop{\mathrm {loc}}}({\mathbb {R}^n})\cap {\mathop {\mathrm {BMO}}}({\mathbb {R}^n})$
$f\in C^\infty _{\mathop{\mathrm {loc}}}({\mathbb {R}^n})\cap {\mathop {\mathrm {BMO}}}({\mathbb {R}^n})$![]() and $u\in J_\sigma (L^2({\mathbb {R}^n}))\cap J_\sigma ({\mathop {\mathrm {BMO}}}({\mathbb {R}^n}))\cap C^\infty _b({\mathbb {R}^n})$
and $u\in J_\sigma (L^2({\mathbb {R}^n}))\cap J_\sigma ({\mathop {\mathrm {BMO}}}({\mathbb {R}^n}))\cap C^\infty _b({\mathbb {R}^n})$![]() be a solution of
be a solution of
in ${\mathbb {R}^n},$![]() where $L$
where $L$![]() is as in lemma 3.2. Then, for any $\alpha \in (0,\,\min \{1,\,\sigma \}),$
is as in lemma 3.2. Then, for any $\alpha \in (0,\,\min \{1,\,\sigma \}),$![]() $x_0\in {\mathbb {R}^n},$
$x_0\in {\mathbb {R}^n},$![]() and $r\in (0,\,\infty ),$
and $r\in (0,\,\infty ),$![]() there exists a positive constant $C,$
there exists a positive constant $C,$![]() depending only $n,$
depending only $n,$![]() $\sigma,$
$\sigma,$![]() $\mu,$
$\mu,$![]() $\Lambda$
$\Lambda$![]() and $\alpha,$
and $\alpha,$![]() such that
such that

Proof. Let $x_0\in {\mathbb {R}^n}$![]() and $r\in (0,\,\infty )$
and $r\in (0,\,\infty )$![]() . Take $\eta \in C_{\rm c}^\infty ({\mathbb {R}^n})$
. Take $\eta \in C_{\rm c}^\infty ({\mathbb {R}^n})$![]() such that $\eta \equiv 1$
such that $\eta \equiv 1$![]() on $B_{2kr}(x_0)$
on $B_{2kr}(x_0)$![]() , $0\le \eta \le 1$
, $0\le \eta \le 1$![]() , and $\mathop{\mathrm {\,supp\,}}(\eta )\subset B_{4kr}(x_0)$
, and $\mathop{\mathrm {\,supp\,}}(\eta )\subset B_{4kr}(x_0)$![]() . Then, we have $\eta [f-(f)_{B_{4kr}(x_0)}]\in C_{\rm c}^\infty (B_{4kr}(x_0))$
. Then, we have $\eta [f-(f)_{B_{4kr}(x_0)}]\in C_{\rm c}^\infty (B_{4kr}(x_0))$![]() . By this, we find that $\eta [f-(f)_{B_{4kr}(x_0)}]\in L^p({\mathbb {R}^n})\cap C^s({\mathbb {R}^n})$
. By this, we find that $\eta [f-(f)_{B_{4kr}(x_0)}]\in L^p({\mathbb {R}^n})\cap C^s({\mathbb {R}^n})$![]() for any $p\in (1,\,\infty )$
for any $p\in (1,\,\infty )$![]() and $s\in (0,\,1)$
and $s\in (0,\,1)$![]() . From lemmas 2.4 and 2.5, we deduce that there exists a unique solution $w\in J_\sigma (\cap _{p\in (1,\infty )}L^p({\mathbb {R}^n}))\cap \Lambda ^{\sigma +s}({\mathbb {R}^n})$
. From lemmas 2.4 and 2.5, we deduce that there exists a unique solution $w\in J_\sigma (\cap _{p\in (1,\infty )}L^p({\mathbb {R}^n}))\cap \Lambda ^{\sigma +s}({\mathbb {R}^n})$![]() for the equation (1.2) with $f$
for the equation (1.2) with $f$![]() replaced by $\eta [f-(f)_{B_{4kr}(x_0)}]$
replaced by $\eta [f-(f)_{B_{4kr}(x_0)}]$![]() , and, for any $p\in (1,\,\infty )$
, and, for any $p\in (1,\,\infty )$![]() , $w$
, $w$![]() satisfies that
satisfies that
where $C$![]() is a positive constant independent of $\lambda$
is a positive constant independent of $\lambda$![]() , $\eta$
, $\eta$![]() , $f$
, $f$![]() and $w$
and $w$![]() . Furthermore, by proposition 2.2(iii) and taking $s\in (0,\,1)$
. Furthermore, by proposition 2.2(iii) and taking $s\in (0,\,1)$![]() small enough such that $\sigma +s\in (0,\,2)$
small enough such that $\sigma +s\in (0,\,2)$![]() , we conclude that $w\in L^{\infty }({\mathbb {R}^n})$
, we conclude that $w\in L^{\infty }({\mathbb {R}^n})$![]() and, for any $x\in {\mathbb {R}^n}$
and, for any $x\in {\mathbb {R}^n}$![]() ,
,

Thus, $w\in J_\sigma ({\mathop {\mathrm {BMO}}}({\mathbb {R}^n}))$![]() . In addition, from the classical theory of the Fourier transform (see, for instance, [Reference Abels2, remark 2.2]), it follows that $w\in C^\infty _b({\mathbb {R}^n})$
. In addition, from the classical theory of the Fourier transform (see, for instance, [Reference Abels2, remark 2.2]), it follows that $w\in C^\infty _b({\mathbb {R}^n})$![]() .
.
Let $v:=u-w$![]() . Then, we have $v\in J_\sigma ({\mathop {\mathrm {BMO}}}({\mathbb {R}^n})) \cap J_\sigma (L^2({\mathbb {R}^n}))\cap C_b^\infty ({\mathbb {R}^n})$
. Then, we have $v\in J_\sigma ({\mathop {\mathrm {BMO}}}({\mathbb {R}^n})) \cap J_\sigma (L^2({\mathbb {R}^n}))\cap C_b^\infty ({\mathbb {R}^n})$![]() and
and
By the fact that $(1-\eta )[f-(f)_{B_{4kr}(x_0)}]+(f)_{B_{4kr}(x_0)}$![]() is a constant in $B_{2kr}(x_0)$
is a constant in $B_{2kr}(x_0)$![]() , similarly to the proof of lemma 3.5, we find that
, similarly to the proof of lemma 3.5, we find that
Applying $(-\Delta )^{\sigma /2}$![]() to both sides of (3.11), we conclude that
to both sides of (3.11), we conclude that
For any $x\in B_{kr}(x_0)$![]() , we have $(1-\eta )\left (x\right )=0$
, we have $(1-\eta )\left (x\right )=0$![]() and, if $y\in B_{kr/2}(x)$
and, if $y\in B_{kr/2}(x)$![]() , then $(1-\eta) \left (x+y\right )=0$
, then $(1-\eta) \left (x+y\right )=0$![]() . By this, proposition 3.1, and the fact that, for any $x\in B_{kr}(x_0)$
. By this, proposition 3.1, and the fact that, for any $x\in B_{kr}(x_0)$![]() and $y\notin B_{kr/2}(x)$
and $y\notin B_{kr/2}(x)$![]() , $|y-x|\gtrsim |y-x_0|$
, $|y-x|\gtrsim |y-x_0|$![]() , we find that, for any $x\in B_{kr}(x_0)$
, we find that, for any $x\in B_{kr}(x_0)$![]() ,
,

This, together with lemma 3.3 and the scaling and shifting the coordinates argument as in lemma 3.5, implies that

From (3.13), we deduce that

Moreover, by (3.10) and the equivalent characterization of $\|f\|_{{\mathop {\mathrm {BMO}}}({\mathbb {R}^n})}$![]() (see, for instance, [Reference Grafakos14, corollary 3.1.9]), we conclude that, for any $p\in (1,\,\infty )$
(see, for instance, [Reference Grafakos14, corollary 3.1.9]), we conclude that, for any $p\in (1,\,\infty )$![]() and $R\in [r,\,\infty )$
and $R\in [r,\,\infty )$![]() ,
,

Furthermore, take $p\in (1,\,\infty )$![]() small enough such that $n/p+\sigma >1$
small enough such that $n/p+\sigma >1$![]() . Then, from (3.15), it follows that
. Then, from (3.15), it follows that

which, together with (3.14), (3.15) and proposition 3.1, further implies that

Similarly, by (3.10), (3.12) and proposition 3.1, we find that

which, combined with (3.17), further implies that lemma 3.6 holds true. This finishes the proof of lemma 3.6.
Let $\phi$![]() be a non-negative, real-valued function in $C_{\rm c}^\infty ({\mathbb {R}^n})$
be a non-negative, real-valued function in $C_{\rm c}^\infty ({\mathbb {R}^n})$![]() with the property that $\int _{\mathbb {R}^n}\phi (x)\,{\rm d}x=1$
with the property that $\int _{\mathbb {R}^n}\phi (x)\,{\rm d}x=1$![]() and $\mathop{\mathrm {\,supp\,}}(\phi )\subset B_1$
and $\mathop{\mathrm {\,supp\,}}(\phi )\subset B_1$![]() . For any $\varepsilon \in (0,\,\infty )$
. For any $\varepsilon \in (0,\,\infty )$![]() , let $\phi _\varepsilon (\cdot ): =\frac {1}{\varepsilon ^{n}}\phi (\frac {\cdot }{\varepsilon })$
, let $\phi _\varepsilon (\cdot ): =\frac {1}{\varepsilon ^{n}}\phi (\frac {\cdot }{\varepsilon })$![]() . Let $u\in L^p({\mathbb {R}^n})\cap {\mathop {\mathrm {BMO}}}({\mathbb {R}^n})$
. Let $u\in L^p({\mathbb {R}^n})\cap {\mathop {\mathrm {BMO}}}({\mathbb {R}^n})$![]() for some $p\in (1,\,\infty )$
for some $p\in (1,\,\infty )$![]() . The mollification $u_\varepsilon$
. The mollification $u_\varepsilon$![]() of $u$
of $u$![]() is defined by, for any $x\in {\mathbb {R}^n}$
is defined by, for any $x\in {\mathbb {R}^n}$![]() ,
,
Then, we have the following well-known properties of $u_\varepsilon$![]() (see, for instance, [Reference Ziemer32, theorem 1.6.1]).
(see, for instance, [Reference Ziemer32, theorem 1.6.1]).
Lemma 3.7 Let $p\in (1,\,\infty ),$![]() $u\in L^p({\mathbb {R}^n})\cap L^\infty ({\mathbb {R}^n})$
$u\in L^p({\mathbb {R}^n})\cap L^\infty ({\mathbb {R}^n})$![]() and $u_\varepsilon$
and $u_\varepsilon$![]() be the mollification of $u$
be the mollification of $u$![]() . Then the following properties hold true.
. Then the following properties hold true.
(i) For any $\varepsilon \in (0,\,\infty ),$
 $u_\varepsilon \in C^\infty ({\mathbb {R}^n})$
$u_\varepsilon \in C^\infty ({\mathbb {R}^n})$ .
.(ii) For any $\varepsilon \in (0,\,\infty ),$
 $u_\varepsilon \in L^p({\mathbb {R}^n})$
$u_\varepsilon \in L^p({\mathbb {R}^n})$ and $\lim _{\varepsilon \to 0}\|u-u_\varepsilon \|_{L^p({\mathbb {R}^n})}=0$
and $\lim _{\varepsilon \to 0}\|u-u_\varepsilon \|_{L^p({\mathbb {R}^n})}=0$ .
.(iii) For any $\varepsilon \in (0,\,\infty ),$
 $\|u_\varepsilon \|_{L^\infty ({\mathbb {R}^n})}\le \|u\|_{L^\infty ({\mathbb {R}^n})}$
$\|u_\varepsilon \|_{L^\infty ({\mathbb {R}^n})}\le \|u\|_{L^\infty ({\mathbb {R}^n})}$ .
.
In addition, when $u\in {\mathop {\mathrm {BMO}}}({\mathbb {R}^n})$![]() , we have the following property of $u_{\varepsilon }$
, we have the following property of $u_{\varepsilon }$![]() .
.
Lemma 3.8 Let $u\in {\mathop {\mathrm {BMO}}}({\mathbb {R}^n})$![]() and $u_\varepsilon$
and $u_\varepsilon$![]() be the mollification of $u$
be the mollification of $u$![]() . Then, for any $\varepsilon \in (0,\,\infty ),$
. Then, for any $\varepsilon \in (0,\,\infty ),$![]() $u_\varepsilon \in {\mathop {\mathrm {BMO}}}({\mathbb {R}^n})$
$u_\varepsilon \in {\mathop {\mathrm {BMO}}}({\mathbb {R}^n})$![]() and
and
where $C$![]() is a positive constant independent of $\varepsilon$
is a positive constant independent of $\varepsilon$![]() and $u$
and $u$![]() .
.
Proof. Let $\varepsilon \in (0,\,\infty )$![]() and $B_r(x_0)\subset {\mathbb {R}^n}$
and $B_r(x_0)\subset {\mathbb {R}^n}$![]() be a ball. By the equivalent characterization of $\|u_\varepsilon \|_{{\mathop {\mathrm {BMO}}}({\mathbb {R}^n})}$
be a ball. By the equivalent characterization of $\|u_\varepsilon \|_{{\mathop {\mathrm {BMO}}}({\mathbb {R}^n})}$![]() (see, for instance, [Reference Grafakos14, proposition 3.1.2(4)]), to show lemma 3.8, we only need to prove that, for any $B_r(x_0)\subset {\mathbb {R}^n}$
(see, for instance, [Reference Grafakos14, proposition 3.1.2(4)]), to show lemma 3.8, we only need to prove that, for any $B_r(x_0)\subset {\mathbb {R}^n}$![]() , there exists a constant $c$
, there exists a constant $c$![]() such that
such that
We first assume that $r\le \varepsilon$![]() . In this case, let $c:=(u)_{B_{3\varepsilon }(x_0)}$
. In this case, let $c:=(u)_{B_{3\varepsilon }(x_0)}$![]() . Then, by the fact that, for any $x\in B_r(x_0)$
. Then, by the fact that, for any $x\in B_r(x_0)$![]() with $r\le \varepsilon$
with $r\le \varepsilon$![]() and $y\in B_\varepsilon (x)$
and $y\in B_\varepsilon (x)$![]() , $y\in B_{3\varepsilon }(x_0)$
, $y\in B_{3\varepsilon }(x_0)$![]() , we have
, we have

Now, we assume that $r\ge \varepsilon$![]() . In this case, let $c:=(u)_{B_{2r}(x_0)}$
. In this case, let $c:=(u)_{B_{2r}(x_0)}$![]() . Then, from the fact that, for any $y\in B_{\varepsilon }$
. Then, from the fact that, for any $y\in B_{\varepsilon }$![]() and $x\in B_r(x_0-y)$
and $x\in B_r(x_0-y)$![]() with $r\ge \varepsilon$
with $r\ge \varepsilon$![]() , $x\in B_{2r}(x_0)$
, $x\in B_{2r}(x_0)$![]() , it follows that
, it follows that

which, together with (3.19), further implies that (3.18) holds true. This finishes the proof of lemma 3.8.
To prove theorems 1.2 and 1.4, we also need the following convergence lemma on the space ${\mathop {\mathrm {BMO}}}({\mathbb {R}^n})$![]() .
.
Lemma 3.9 Let $p\in (1,\,\infty ),$![]() $\{f_k\}_{k\in {\mathbb {N}}}\subset {\mathop {\mathrm {BMO}}}({\mathbb {R}^n})\cap L^p({\mathbb {R}^n})$
$\{f_k\}_{k\in {\mathbb {N}}}\subset {\mathop {\mathrm {BMO}}}({\mathbb {R}^n})\cap L^p({\mathbb {R}^n})$![]() be a sequence of functions and $f\in L^p({\mathbb {R}^n})$
be a sequence of functions and $f\in L^p({\mathbb {R}^n})$![]() . Assume that $\lim _{k\to \infty }\|f-f_k\|_{L^p({\mathbb {R}^n})}=0$
. Assume that $\lim _{k\to \infty }\|f-f_k\|_{L^p({\mathbb {R}^n})}=0$![]() and $\lim _{k\to \infty }f_k=f$
and $\lim _{k\to \infty }f_k=f$![]() in the sense of almost everywhere. Then, $f\in {\mathop {\mathrm {BMO}}}({\mathbb {R}^n})$
in the sense of almost everywhere. Then, $f\in {\mathop {\mathrm {BMO}}}({\mathbb {R}^n})$![]() and
and
Proof. Let $B\subset {\mathbb {R}^n}$![]() be a ball. Then, by the Hölder inequality, we conclude that, for any $k\in {\mathbb {N}}$
be a ball. Then, by the Hölder inequality, we conclude that, for any $k\in {\mathbb {N}}$![]() ,
,

Furthermore, from the assumptions that $\lim _{k\to \infty }\|f-f_k\|_{L^p({\mathbb {R}^n})}=0$![]() and $\lim _{k\to \infty }f_k=f$
and $\lim _{k\to \infty }f_k=f$![]() in the sense of almost everywhere, we deduce that
in the sense of almost everywhere, we deduce that
which, together with the Fatou lemma, further implies that
Since the ball $B$![]() is arbitrary, it follows that
is arbitrary, it follows that
This finishes the proof of lemma 3.9.
Now, we prove theorem 1.2 by using lemmas 2.3, 2.4, 2.5, 3.3, 3.6, 3.7 and 3.9.
Proof of theorem 1.2. We first show (i). Let $\lambda \in (0,\,\infty )$![]() , $u\in J_ \sigma (L^p({\mathbb {R}^n}))\cap J_\sigma ({\mathop {\mathrm {BMO}}}({\mathbb {R}^n}))$
, $u\in J_ \sigma (L^p({\mathbb {R}^n}))\cap J_\sigma ({\mathop {\mathrm {BMO}}}({\mathbb {R}^n}))$![]() and $f=-(-\Delta )^{\sigma /2}u-\lambda u$
and $f=-(-\Delta )^{\sigma /2}u-\lambda u$![]() . Then, we have $f\in L^p({\mathbb {R}^n})\cap {\mathop {\mathrm {BMO}}}({\mathbb {R}^n})$
. Then, we have $f\in L^p({\mathbb {R}^n})\cap {\mathop {\mathrm {BMO}}}({\mathbb {R}^n})$![]() . Let $f_\varepsilon$
. Let $f_\varepsilon$![]() be the mollification of $f$
be the mollification of $f$![]() . Then, by lemmas 3.7 and 3.8, we find that, for any $\varepsilon \in (0,\,\infty )$
. Then, by lemmas 3.7 and 3.8, we find that, for any $\varepsilon \in (0,\,\infty )$![]() , $f_\varepsilon \in L^p({\mathbb {R}^n})\cap {\mathop {\mathrm {BMO}}}({\mathbb {R}^n})$
, $f_\varepsilon \in L^p({\mathbb {R}^n})\cap {\mathop {\mathrm {BMO}}}({\mathbb {R}^n})$![]() . From lemma 2.4, it follows that there exists a $u_\varepsilon \in J_\sigma (L^p({\mathbb {R}^n}))$
. From lemma 2.4, it follows that there exists a $u_\varepsilon \in J_\sigma (L^p({\mathbb {R}^n}))$![]() such that
such that
moreover, there exists a positive constant $C$![]() , independent of $f$
, independent of $f$![]() , $f_\varepsilon$
, $f_\varepsilon$![]() , $u$
, $u$![]() , $u_\varepsilon$
, $u_\varepsilon$![]() and $\lambda$
and $\lambda$![]() , such that
, such that
Let $\{\chi _j\}_{j\in {\mathbb {N}}}$![]() be a sequence of smooth functions satisfying that $\chi _j=1$
be a sequence of smooth functions satisfying that $\chi _j=1$![]() on the ball $B_j$
on the ball $B_j$![]() , $\mathop{\mathrm {\,supp\,}}(\chi _j)\subset B_{j+1}$
, $\mathop{\mathrm {\,supp\,}}(\chi _j)\subset B_{j+1}$![]() , and $0\le \chi _j\le 1$
, and $0\le \chi _j\le 1$![]() , where, for any $j\in {\mathbb {N}}$
, where, for any $j\in {\mathbb {N}}$![]() , $B_j:=B(\mathbf {0},\,j)$
, $B_j:=B(\mathbf {0},\,j)$![]() . Then, we have $\chi _jf_\varepsilon \in C_{\rm c}^\infty ({\mathbb {R}^n})$
. Then, we have $\chi _jf_\varepsilon \in C_{\rm c}^\infty ({\mathbb {R}^n})$![]() for any $j\in {\mathbb {N}}$
for any $j\in {\mathbb {N}}$![]() and
and
Moreover, from lemma 2.4 and the theory of Fourier transform (see, for instance, [Reference Abels2, remark 2.2]), we deduce that there exists a unique $u_{\varepsilon,j}\in C^\infty _b({\mathbb {R}^n})\cap J_\sigma (L^2({\mathbb {R}^n}))$![]() such that
such that
meanwhile, there exists a positive constant $C$![]() , independent of $f_\varepsilon$
, independent of $f_\varepsilon$![]() , $u_\varepsilon$
, $u_\varepsilon$![]() , $u_{\varepsilon,j}$
, $u_{\varepsilon,j}$![]() , $\chi _j$
, $\chi _j$![]() and $\lambda$
and $\lambda$![]() , such that
, such that
Take $\eta \in C_{\rm c}^\infty ({\mathbb {R}^n})$![]() such that $\eta \equiv 1$
such that $\eta \equiv 1$![]() on $B_2$
on $B_2$![]() , $\mathop{\mathrm {\,supp\,}}(\eta )\subset B_4$
, $\mathop{\mathrm {\,supp\,}}(\eta )\subset B_4$![]() and $0\le \eta \le 1$
and $0\le \eta \le 1$![]() . Then, we have $\eta \chi _jf_\varepsilon \subset C_{\rm c}^\infty ({\mathbb {R}^n})$
. Then, we have $\eta \chi _jf_\varepsilon \subset C_{\rm c}^\infty ({\mathbb {R}^n})$![]() . By using lemma 2.4 and the theory of Fourier transform again, we find that there exists a unique $w_{\varepsilon,j}\in J_\sigma (\cap _{q\in (1,\infty )}L^q({\mathbb {R}^n})) \cap C^\infty _b({\mathbb {R}^n})$
. By using lemma 2.4 and the theory of Fourier transform again, we find that there exists a unique $w_{\varepsilon,j}\in J_\sigma (\cap _{q\in (1,\infty )}L^q({\mathbb {R}^n})) \cap C^\infty _b({\mathbb {R}^n})$![]() such that
such that
and, for any $q\in (1,\,\infty )$![]() ,
,
where $C$![]() is a positive constant independent of $w_{\varepsilon,j}$
is a positive constant independent of $w_{\varepsilon,j}$![]() , $\eta$
, $\eta$![]() , $\chi _j$
, $\chi _j$![]() , $f_\varepsilon$
, $f_\varepsilon$![]() and $\lambda$
and $\lambda$![]() .
.
Let $v_{\varepsilon,j}:=u_{\varepsilon,j}-w_{\varepsilon,j}\in J_\sigma (L^2({\mathbb {R}^n}))\cap C^\infty _b({\mathbb {R}^n})$![]() . Then
. Then
By applying $L$![]() to both sides of (3.26), we conclude that
to both sides of (3.26), we conclude that
From the fact that $v_{\varepsilon,j}\in C^\infty _b({\mathbb {R}^n})\subset \Lambda ^s({\mathbb {R}^n})$![]() for any $s\in (0,\,\infty )$
for any $s\in (0,\,\infty )$![]() and lemma 2.5, we deduce that $Lv_{\varepsilon,j}\in \Lambda ^s({\mathbb {R}^n})$
and lemma 2.5, we deduce that $Lv_{\varepsilon,j}\in \Lambda ^s({\mathbb {R}^n})$![]() for any $s\in (0,\,\infty )$
for any $s\in (0,\,\infty )$![]() . Then, by proposition 2.2, we find that $Lv_{\varepsilon,j}\in L^\infty ({\mathbb {R}^n})\cap C^2({\mathbb {R}^n})$
. Then, by proposition 2.2, we find that $Lv_{\varepsilon,j}\in L^\infty ({\mathbb {R}^n})\cap C^2({\mathbb {R}^n})$![]() , which, together with lemma 3.3, further implies that there exists $\alpha \in (0,\,\min \{1,\,\sigma \})$
, which, together with lemma 3.3, further implies that there exists $\alpha \in (0,\,\min \{1,\,\sigma \})$![]() such that
such that
where $C$![]() is a positive constant independent of $v_{\varepsilon,j}$
is a positive constant independent of $v_{\varepsilon,j}$![]() , $\eta$
, $\eta$![]() , $\chi _j$
, $\chi _j$![]() , $f_\varepsilon$
, $f_\varepsilon$![]() and $\lambda$
and $\lambda$![]() . For any $x\in B_1$
. For any $x\in B_1$![]() , we have that $(1-\eta )\chi _jf_\varepsilon (x)=0$
, we have that $(1-\eta )\chi _jf_\varepsilon (x)=0$![]() , and if $y\in B_{1/2}$
, and if $y\in B_{1/2}$![]() , then $(1-\eta )\chi _jf_\varepsilon (x+y)=0$
, then $(1-\eta )\chi _jf_\varepsilon (x+y)=0$![]() . Meanwhile, by $f\in {\mathop {\mathrm {BMO}}}({\mathbb {R}^n})$
. Meanwhile, by $f\in {\mathop {\mathrm {BMO}}}({\mathbb {R}^n})$![]() and lemma 3.8, we find that, for any $\varepsilon \in (0,\,\infty )$
and lemma 3.8, we find that, for any $\varepsilon \in (0,\,\infty )$![]() , $f_\varepsilon \in {\mathop {\mathrm {BMO}}}({\mathbb {R}^n})$
, $f_\varepsilon \in {\mathop {\mathrm {BMO}}}({\mathbb {R}^n})$![]() , which, combined with the characterization of pointwise multipliers for functions of bounded mean oscillation (see, for instance, [Reference Nakai and Yabuta21, theorem 1]), implies that, for any $j\in {\mathbb {N}}$
, which, combined with the characterization of pointwise multipliers for functions of bounded mean oscillation (see, for instance, [Reference Nakai and Yabuta21, theorem 1]), implies that, for any $j\in {\mathbb {N}}$![]() and $\varepsilon \in (0,\,\infty )$
and $\varepsilon \in (0,\,\infty )$![]() , $(1-\eta )\chi _jf_\varepsilon \in {\mathop {\mathrm {BMO}}}({\mathbb {R}^n})$
, $(1-\eta )\chi _jf_\varepsilon \in {\mathop {\mathrm {BMO}}}({\mathbb {R}^n})$![]() . Moreover, from [Reference Nakai and Yabuta21, lemmas 3.1 and 3.3] and the proof of [Reference Nakai and Yabuta21, theorem 1] (see [Reference Nakai and Yabuta21, pp. 215-216]), we deduce that
. Moreover, from [Reference Nakai and Yabuta21, lemmas 3.1 and 3.3] and the proof of [Reference Nakai and Yabuta21, theorem 1] (see [Reference Nakai and Yabuta21, pp. 215-216]), we deduce that
which, together with the fact that, for any $x\in B_1$![]() , $((1-\eta )\chi _jf_\varepsilon )_{B_{1/2}(x)} =0$
, $((1-\eta )\chi _jf_\varepsilon )_{B_{1/2}(x)} =0$![]() , proposition 3.1, and lemma 3.8, further implies that, for any $x\in B_1$
, proposition 3.1, and lemma 3.8, further implies that, for any $x\in B_1$![]() ,
,

where $C$![]() is a positive constant independent of $\eta$
is a positive constant independent of $\eta$![]() , $\chi _j$
, $\chi _j$![]() , $f_\varepsilon$
, $f_\varepsilon$![]() and $\lambda$
and $\lambda$![]() . By this and (3.27), we conclude that
. By this and (3.27), we conclude that
Then, similarly to the proofs of lemmas 3.5 and 3.6, by (3.25), (3.28), and a scaling and shifting the coordinates argument, we conclude that, for any $k\in [2,\,\infty )$![]() ,
,

where $C$![]() is a positive constant independent of $x_0$
is a positive constant independent of $x_0$![]() , $r$
, $r$![]() , $k$
, $k$![]() , $u_{\varepsilon,j}$
, $u_{\varepsilon,j}$![]() , $f$
, $f$![]() and $\lambda$
and $\lambda$![]() . Since $x_0$
. Since $x_0$![]() and $r$
and $r$![]() are arbitrary, it follows that, by taking a sufficient large $k$
are arbitrary, it follows that, by taking a sufficient large $k$![]() such that $Ck^{-\alpha }\le \frac 12$
such that $Ck^{-\alpha }\le \frac 12$![]() , we have
, we have
where $C$![]() is a positive constant independent of $f$
is a positive constant independent of $f$![]() , $u_{\varepsilon,j}$
, $u_{\varepsilon,j}$![]() and $\lambda$
and $\lambda$![]() .
.
Furthermore, by (3.22) and (3.24), we find that there exists a subsequence of $\{Lu_{\varepsilon,j}\}_{j\in {\mathbb {N}}}$![]() , still denoted by $\{Lu_{\varepsilon,j}\}_{j\in {\mathbb {N}}}$
, still denoted by $\{Lu_{\varepsilon,j}\}_{j\in {\mathbb {N}}}$![]() , such that
, such that
in the sense of almost everywhere, which, together with lemma 3.9 and (3.29), further implies that
Similarly, from (3.30), (3.21) and lemmas 3.7 and 3.9, we deduce that

Since the constant $C$![]() in (3.31) is independent of $\lambda$
in (3.31) is independent of $\lambda$![]() , by taking $\lambda \to 0^+$
, by taking $\lambda \to 0^+$![]() , we obtain (i). This finishes the proof of (i).
, we obtain (i). This finishes the proof of (i).
Next, we prove (ii) by borrowing some ideas from [Reference Dong, Jung and Kim6] (see also [Reference Mikulevičius and Pragarauskas20]). We first assume that $\sigma \in (0,\,1)$![]() . From the proof of [Reference Dong, Jung and Kim6, proposition 4.1], it follows that
. From the proof of [Reference Dong, Jung and Kim6, proposition 4.1], it follows that

where $C_0:=\frac {\Gamma ((n-\alpha )/2)}{2^\alpha \pi ^{n/2}\Gamma (\alpha /2)}$![]() , $\varepsilon \in (0,\,1)$
, $\varepsilon \in (0,\,1)$![]() , $a_\varepsilon (y):=a(y)\mathbf {1}_{\varepsilon \le 1\le \frac {1}{\varepsilon }}$
, $a_\varepsilon (y):=a(y)\mathbf {1}_{\varepsilon \le 1\le \frac {1}{\varepsilon }}$![]() , and $k^\sigma (z,\,y):=|z+y|^{-n+\sigma }-|z|^{-n+\sigma }$
, and $k^\sigma (z,\,y):=|z+y|^{-n+\sigma }-|z|^{-n+\sigma }$![]() .
.
Let
Then, we have
where $T^\varepsilon$![]() denotes the singular integral operator associated with the kernel $k_\varepsilon$
denotes the singular integral operator associated with the kernel $k_\varepsilon$![]() . By [Reference Dong, Jung and Kim6, lemmas 4.4 and 4.5], we conclude that the assumptions in lemma 2.3 are satisfied. Thus, from lemma 2.3 and the Fatou lemma, we deduce that
. By [Reference Dong, Jung and Kim6, lemmas 4.4 and 4.5], we conclude that the assumptions in lemma 2.3 are satisfied. Thus, from lemma 2.3 and the Fatou lemma, we deduce that
For the case $\sigma =1$![]() and $\sigma \in (1,\,2)$
and $\sigma \in (1,\,2)$![]() , (1.7) also holds true. Indeed, if $\sigma \in (1,\,2)$
, (1.7) also holds true. Indeed, if $\sigma \in (1,\,2)$![]() , $L_\varepsilon u$
, $L_\varepsilon u$![]() can be written as
can be written as

where
and $L^{(a_\varepsilon )_i}$![]() denotes the non-local elliptic operator defined by
denotes the non-local elliptic operator defined by
Then, by (3.32) and the boundedness of the Riesz transform on $H^1({\mathbb {R}^n})$![]() (see, for instance, [Reference Stein27, chapter III, theorem 4]), we conclude that
(see, for instance, [Reference Stein27, chapter III, theorem 4]), we conclude that

Thus, (1.7) holds true in the case of $\sigma \in (1,\,2)$![]() .
.
If $\sigma =1$![]() , via using assumption 1.1(ii) and an argument used in [Reference Dong, Jung and Kim6, p. 18], we find that
, via using assumption 1.1(ii) and an argument used in [Reference Dong, Jung and Kim6, p. 18], we find that

where, for any $\varepsilon \in (0,\,\infty )$![]() and $z\in {\mathbb {R}^n}$
and $z\in {\mathbb {R}^n}$![]() with $z\neq \mathbf {0}$
with $z\neq \mathbf {0}$![]() ,
,

satisfies that there exists a positive constant $C$![]() , depending only on $n$
, depending only on $n$![]() , such that $|m_\varepsilon (z)|\le C$
, such that $|m_\varepsilon (z)|\le C$![]() . Since $\sigma -\frac 12\in (\frac 14,\,\frac 34)$
. Since $\sigma -\frac 12\in (\frac 14,\,\frac 34)$![]() and $|m_\varepsilon (z)|\lesssim 1$
and $|m_\varepsilon (z)|\lesssim 1$![]() for any $\varepsilon \in (0,\,\infty )$
for any $\varepsilon \in (0,\,\infty )$![]() and $z\in {\mathbb {R}^n}$
and $z\in {\mathbb {R}^n}$![]() with $z\neq \mathbf {0}$
with $z\neq \mathbf {0}$![]() , similar to the proof of (3.32), it follows that
, similar to the proof of (3.32), it follows that
This, together with (3.32) and (3.33), implies that
holds true for any $\sigma \in (0,\,2)$![]() .
.
Furthermore, it is known that $J_\sigma (L^2({\mathbb {R}^n}))\cap I_\sigma (H^1({\mathbb {R}^n}))$![]() is dense in $I_\sigma (H^1({\mathbb {R}^n}))$
is dense in $I_\sigma (H^1({\mathbb {R}^n}))$![]() (see, for instance, [Reference Triebel30, chapter 5]). Therefore, for any $u\in J_\sigma (H^1({\mathbb {R}^n}))$
(see, for instance, [Reference Triebel30, chapter 5]). Therefore, for any $u\in J_\sigma (H^1({\mathbb {R}^n}))$![]() , there exists a Cauchy sequence $\{u_k\}_{k\in {\mathbb {N}}}\subset J_\sigma (L^2({\mathbb {R}^n}))\cap I_\sigma (H^1({\mathbb {R}^n}))$
, there exists a Cauchy sequence $\{u_k\}_{k\in {\mathbb {N}}}\subset J_\sigma (L^2({\mathbb {R}^n}))\cap I_\sigma (H^1({\mathbb {R}^n}))$![]() such that $u_k$
such that $u_k$![]() converges to $u$
converges to $u$![]() in $I_\sigma (H^1({\mathbb {R}^n}))$
in $I_\sigma (H^1({\mathbb {R}^n}))$![]() . By lemma 2.4 and (3.34), we find that, for any $k\in {\mathbb {N}}$
. By lemma 2.4 and (3.34), we find that, for any $k\in {\mathbb {N}}$![]() , $Lu_k\in L^2({\mathbb {R}^n})\cap L^1({\mathbb {R}^n})$
, $Lu_k\in L^2({\mathbb {R}^n})\cap L^1({\mathbb {R}^n})$![]() and
and
Moreover, from the boundedness of the Riesz transform $R_j$![]() on $H^1({\mathbb {R}^n})$
on $H^1({\mathbb {R}^n})$![]() and (3.34), we deduce that, for any $k\in {\mathbb {N}}$
and (3.34), we deduce that, for any $k\in {\mathbb {N}}$![]() ,
,

which, combined with (3.35), further implies that, for any $k\in {\mathbb {N}}$![]() ,
,
By this estimate and the density of $J_\sigma (L^2({\mathbb {R}^n}))\cap I_\sigma (H^1({\mathbb {R}^n}))$![]() in $I_\sigma (H^1({\mathbb {R}^n}))$
in $I_\sigma (H^1({\mathbb {R}^n}))$![]() , we conclude that (1.7) holds true. Therefore, this finishes the proof of (ii) and hence of theorem 1.2.
, we conclude that (1.7) holds true. Therefore, this finishes the proof of (ii) and hence of theorem 1.2.
Next, we prove theorem 1.4 by using lemmas 2.4, 2.5, 3.6, 3.7 and 3.9, and theorem 1.2.
Proof of theorem 1.4. We first show (i). Let $p\in (1,\,\infty )$![]() , $f\in L^p({\mathbb {R}^n})\cap {\mathop {\mathrm {BMO}}}({\mathbb {R}^n})$
, $f\in L^p({\mathbb {R}^n})\cap {\mathop {\mathrm {BMO}}}({\mathbb {R}^n})$![]() and $f_\varepsilon$
and $f_\varepsilon$![]() be the mollification of $f$
be the mollification of $f$![]() . Then, for any $\varepsilon \in (0,\,\infty )$
. Then, for any $\varepsilon \in (0,\,\infty )$![]() , $f_\varepsilon \in L^p({\mathbb {R}^n})\cap {\mathop {\mathrm {BMO}}}({\mathbb {R}^n})\cap C^\infty ({\mathbb {R}^n})$
, $f_\varepsilon \in L^p({\mathbb {R}^n})\cap {\mathop {\mathrm {BMO}}}({\mathbb {R}^n})\cap C^\infty ({\mathbb {R}^n})$![]() . From lemma 2.4, it follows that there exist solutions $u,\,u_\varepsilon \in J_\sigma (L^p({\mathbb {R}^n}))$
. From lemma 2.4, it follows that there exist solutions $u,\,u_\varepsilon \in J_\sigma (L^p({\mathbb {R}^n}))$![]() for the equation (1.1) with respect to $f$
for the equation (1.1) with respect to $f$![]() and $f_\varepsilon$
and $f_\varepsilon$![]() , respectively, with satisfying that
, respectively, with satisfying that

and
where $C$![]() is a positive constant independent of $u$
is a positive constant independent of $u$![]() , $f$
, $f$![]() , $u_\varepsilon$
, $u_\varepsilon$![]() , $f_\varepsilon$
, $f_\varepsilon$![]() and $\lambda$
and $\lambda$![]() .
.
Let $\{\eta _j\}_{j\in {\mathbb {N}}}$![]() be a sequence of smooth functions satisfying that $\eta _j=1$
be a sequence of smooth functions satisfying that $\eta _j=1$![]() on the ball $B_j$
on the ball $B_j$![]() , $\mathop{\mathrm {\,supp\,}}({\eta _j})\subset B_{j+1}$
, $\mathop{\mathrm {\,supp\,}}({\eta _j})\subset B_{j+1}$![]() and $0\le \eta _j\le 1$
and $0\le \eta _j\le 1$![]() , where, for any $j\in {\mathbb {N}}$
, where, for any $j\in {\mathbb {N}}$![]() , $B_j:=B(\mathbf 0,\,j)$
, $B_j:=B(\mathbf 0,\,j)$![]() . For any $j\in {\mathbb {N}}$
. For any $j\in {\mathbb {N}}$![]() , we have $\eta _j f_\varepsilon \in C_{\rm c}^\infty ({\mathbb {R}^n})\cap L^2({\mathbb {R}^n})\cap L^p({\mathbb {R}^n})\cap {\mathop {\mathrm {BMO}}}({\mathbb {R}^n})$
, we have $\eta _j f_\varepsilon \in C_{\rm c}^\infty ({\mathbb {R}^n})\cap L^2({\mathbb {R}^n})\cap L^p({\mathbb {R}^n})\cap {\mathop {\mathrm {BMO}}}({\mathbb {R}^n})$![]() and
and
By lemma 2.4, we find that there exists a unique solution $u_{\varepsilon,j}\in J_\sigma (L^2({\mathbb {R}^n}))\cap J_\sigma (L^p({\mathbb {R}^n}))$![]() for the equation (1.1) with $f$
for the equation (1.1) with $f$![]() replaced by $\eta _jf_\varepsilon$
replaced by $\eta _jf_\varepsilon$![]() , moreover, there exists a positive constant $C$
, moreover, there exists a positive constant $C$![]() , independent of $u_\varepsilon$
, independent of $u_\varepsilon$![]() , $f_\varepsilon$
, $f_\varepsilon$![]() , $u_{\varepsilon,j}$
, $u_{\varepsilon,j}$![]() , $\eta _j$
, $\eta _j$![]() and $\lambda$
and $\lambda$![]() , such that
, such that
Since $\eta _jf_\varepsilon \in C_{\rm c}^\infty ({\mathbb {R}^n})$![]() , it follows that $\eta _jf_\varepsilon \in C^s({\mathbb {R}^n})$
, it follows that $\eta _jf_\varepsilon \in C^s({\mathbb {R}^n})$![]() for any $s\in (0,\,1)$
for any $s\in (0,\,1)$![]() . Then, by lemma 2.5, we conclude that $u_{\varepsilon,j}\in \Lambda ^{s+\sigma }({\mathbb {R}^n})$
. Then, by lemma 2.5, we conclude that $u_{\varepsilon,j}\in \Lambda ^{s+\sigma }({\mathbb {R}^n})$![]() , which, together with proposition 2.2(iii), further implies that $u_{\varepsilon,j}$
, which, together with proposition 2.2(iii), further implies that $u_{\varepsilon,j}$![]() and $\partial ^\sigma u_{\varepsilon,j}$
and $\partial ^\sigma u_{\varepsilon,j}$![]() belong to $L^\infty ({\mathbb {R}^n})$
belong to $L^\infty ({\mathbb {R}^n})$![]() . Thus, $u_{\varepsilon,j}\in J_\sigma ({\mathop {\mathrm {BMO}}})({\mathbb {R}^n})$
. Thus, $u_{\varepsilon,j}\in J_\sigma ({\mathop {\mathrm {BMO}}})({\mathbb {R}^n})$![]() . From the fact that $\eta _jf_\varepsilon \in C_{\rm c}^\infty ({\mathbb {R}^n})$
. From the fact that $\eta _jf_\varepsilon \in C_{\rm c}^\infty ({\mathbb {R}^n})$![]() and the theory of Fourier transform, we deduce that $u_{\varepsilon,j}\in C_b^\infty ({\mathbb {R}^n})$
and the theory of Fourier transform, we deduce that $u_{\varepsilon,j}\in C_b^\infty ({\mathbb {R}^n})$![]() . Then, by lemma 3.6, we find that
. Then, by lemma 3.6, we find that

where $C$![]() is a positive constant independent of $u_{\varepsilon,j}$
is a positive constant independent of $u_{\varepsilon,j}$![]() , $f_{\varepsilon }$
, $f_{\varepsilon }$![]() , $\eta _j$
, $\eta _j$![]() , $x_0$
, $x_0$![]() , $r$
, $r$![]() , $k$
, $k$![]() and $\lambda$
and $\lambda$![]() . Since $x_0\in {\mathbb {R}^n}$
. Since $x_0\in {\mathbb {R}^n}$![]() and $r\in (0,\,\infty )$
and $r\in (0,\,\infty )$![]() are arbitrary, it follows that
are arbitrary, it follows that

Via taking a sufficient large $k$![]() such that $Ck^{-\alpha }\le \frac 12$
such that $Ck^{-\alpha }\le \frac 12$![]() , we then obtain that
, we then obtain that
By the characterization of pointwise multipliers for functions of bounded mean oscillation (see, for instance, [Reference Nakai and Yabuta21]) and lemmas 3.8, we conclude that
Moreover, from (3.37) and (3.38), we deduce that there exists a subsequence of $\{u_{\varepsilon,j}\}_{j\in {\mathbb {N}}}$![]() , still denoted by $\{u_{\varepsilon,j}\}_{j\in {\mathbb {N}}}$
, still denoted by $\{u_{\varepsilon,j}\}_{j\in {\mathbb {N}}}$![]() , such that
, such that
and
in the sense of almost everywhere, which, combined with (3.39) and lemma 3.9, further implies that
Similarly, by (3.36), lemma 3.7(ii), (3.40) and lemma 3.9, we find that (1.9) holds true. This finishes the proof of (i).
Next, we prove (ii). We first assume that $f\in H^1({\mathbb {R}^n})\cap L^2({\mathbb {R}^n})$![]() . Let $L^\ast$
. Let $L^\ast$![]() be the non-local operator associated with the kernel $a(-\cdot )$
be the non-local operator associated with the kernel $a(-\cdot )$![]() . Then, we observe that $a(-\cdot )$
. Then, we observe that $a(-\cdot )$![]() also satisfies assumption 1.1. For any $g\in L^\infty ({\mathbb {R}^n})\cap L^2({\mathbb {R}^n})$
also satisfies assumption 1.1. For any $g\in L^\infty ({\mathbb {R}^n})\cap L^2({\mathbb {R}^n})$![]() , by (i) and lemma 2.4, we conclude that there exists a unique $u\in J_\sigma ({\mathop {\mathrm {BMO}}}({\mathbb {R}^n}))\cap J_\sigma (L^2({\mathbb {R}^n}))$
, by (i) and lemma 2.4, we conclude that there exists a unique $u\in J_\sigma ({\mathop {\mathrm {BMO}}}({\mathbb {R}^n}))\cap J_\sigma (L^2({\mathbb {R}^n}))$![]() such that
such that
moreover, there exists a positive constant $C$![]() , independent of $u$
, independent of $u$![]() , $g$
, $g$![]() and $\lambda$
and $\lambda$![]() , such that
, such that
Furthermore, from lemma 2.4, it follows that there exists a unique $v\in J_\sigma (L^2({\mathbb {R}^n}))$![]() such that
such that
Then, we find that
which, together with (3.41) and the characterization of the norm of $L^1({\mathbb {R}^n})$![]() (see, for instance, [Reference Folland12, theorem 6.14]) and the fact that ${\mathop {\mathrm {BMO}}}({\mathbb {R}^n})$
(see, for instance, [Reference Folland12, theorem 6.14]) and the fact that ${\mathop {\mathrm {BMO}}}({\mathbb {R}^n})$![]() is the dual space of $H^1({\mathbb {R}^n})$
is the dual space of $H^1({\mathbb {R}^n})$![]() (see, for instance, [Reference Grafakos14, theorem 3.2.2] and [Reference Stein27, p. 142, theorem 1]), further implies that
(see, for instance, [Reference Grafakos14, theorem 3.2.2] and [Reference Stein27, p. 142, theorem 1]), further implies that

Similarly, for $(-\Delta )^{\sigma /2}v$![]() , we have
, we have

which, combined with (3.41) and the characterization of the norm of $L^1({\mathbb {R}^n})$![]() , implies that
, implies that

By applying the Riesz transform $R_j$![]() to the two sides of (3.42), we obtain that
to the two sides of (3.42), we obtain that
Since the Riesz transform $R_j$![]() is bounded on both $L^2({\mathbb {R}^n})$
is bounded on both $L^2({\mathbb {R}^n})$![]() and $H^1({\mathbb {R}^n})$
and $H^1({\mathbb {R}^n})$![]() , it follows that $R_jf\in L^2({\mathbb {R}^n})\cap H^1({\mathbb {R}^n})$
, it follows that $R_jf\in L^2({\mathbb {R}^n})\cap H^1({\mathbb {R}^n})$![]() . Then, by using an argument similar to that used in (3.43) and (3.44), we conclude that
. Then, by using an argument similar to that used in (3.43) and (3.44), we conclude that

which, together with (3.43), (3.44) and (1.4), further implies that
Finally, for any $f\in H^1({\mathbb {R}^n})$![]() , it is known that there exists a Cauchy sequence $\{f_k\}_{k\in {\mathbb {N}}}\subset L^2({\mathbb {R}^n})\cap H^1({\mathbb {R}^n})$
, it is known that there exists a Cauchy sequence $\{f_k\}_{k\in {\mathbb {N}}}\subset L^2({\mathbb {R}^n})\cap H^1({\mathbb {R}^n})$![]() such that $f_k$
such that $f_k$![]() converges to $f$
converges to $f$![]() in $H^1({\mathbb {R}^n})$
in $H^1({\mathbb {R}^n})$![]() (see, for instance, [Reference Grafakos14, proposition 2.1.7] and [Reference Stein27]). Then, from (3.45) and lemma 2.4, we deduce that, for $f_k$
(see, for instance, [Reference Grafakos14, proposition 2.1.7] and [Reference Stein27]). Then, from (3.45) and lemma 2.4, we deduce that, for $f_k$![]() and $f_m$
and $f_m$![]() with $k$
with $k$![]() , $m\in {\mathbb {N}}$
, $m\in {\mathbb {N}}$![]() , there exist $v_k,\,v_m\in J_\sigma (L^2({\mathbb {R}^n}))\cap J_\sigma (H^1({\mathbb {R}^n}))$
, there exist $v_k,\,v_m\in J_\sigma (L^2({\mathbb {R}^n}))\cap J_\sigma (H^1({\mathbb {R}^n}))$![]() such that
such that
and
moreover, we have
and
Therefore, $\{v_k\}_{k\in {\mathbb {N}}}$![]() is a Cauchy sequence in $J_\sigma (H^1({\mathbb {R}^n}))$
is a Cauchy sequence in $J_\sigma (H^1({\mathbb {R}^n}))$![]() , and there exists a $v\in J_\sigma (H^1({\mathbb {R}^n}))$
, and there exists a $v\in J_\sigma (H^1({\mathbb {R}^n}))$![]() such that $v_k$
such that $v_k$![]() converges to $v$
converges to $v$![]() in $J_\sigma (H^1({\mathbb {R}^n}))$
in $J_\sigma (H^1({\mathbb {R}^n}))$![]() . Then, by theorem 1.2(ii), we conclude that
. Then, by theorem 1.2(ii), we conclude that
and $Lv_k$![]() converges to $Lv$
converges to $Lv$![]() in $L^1({\mathbb {R}^n})$
in $L^1({\mathbb {R}^n})$![]() . Furthermore, $v$
. Furthermore, $v$![]() is a solution of $Lv-\lambda v=f$
is a solution of $Lv-\lambda v=f$![]() and
and
Meanwhile, the uniqueness follows from the above estimate. This finishes the proof of theorem 1.4.
Acknowledgments
The authors would like to thank the referee for her/his many valuable comments which essentially improve the presentation of this paper and, particularly, motivate the authors to improve the conclusions of theorems 1.2(ii) and 1.4(i). The authors would also like to thank Yangyang Zhang very much for some helpful discussions on the counterexample for that $L^p({\mathbb {R}^n})\cap {\mathop {\mathrm {BMO}}}({\mathbb {R}^n})$![]() with $p\in (1,\,\infty )$
with $p\in (1,\,\infty )$![]() is not dense in ${\mathop {\mathrm {BMO}}}({\mathbb {R}^n})$
is not dense in ${\mathop {\mathrm {BMO}}}({\mathbb {R}^n})$![]() .
.
This project is partially supported by the National Natural Science Foundation of China (Grant Nos. 11871254 and 12071431) and the Fundamental Research Funds for the Central Universities (Grant No. lzujbky-2021-ey18).