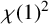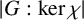1 Introduction
In this paper, all groups under consideration are finite and all characters are complex. Notation is standard and taken from [Reference Isaacs5]. Let G be a finite group and
![]() $\chi \in \textrm {Irr}(G)$
, where
$\chi \in \textrm {Irr}(G)$
, where
![]() $\textrm {Irr}(G)$
denotes the set of all irreducible complex characters of G. We say that
$\textrm {Irr}(G)$
denotes the set of all irreducible complex characters of G. We say that
![]() $\chi $
is a monolithic character if
$\chi $
is a monolithic character if
![]() $G/\textrm {ker}\,\chi $
has a unique minimal normal subgroup. These characters play an important role in understanding the structure of finite groups (for more details, see [Reference Berkovich and Zhmud2]).
$G/\textrm {ker}\,\chi $
has a unique minimal normal subgroup. These characters play an important role in understanding the structure of finite groups (for more details, see [Reference Berkovich and Zhmud2]).
A monolithic character
![]() $\chi $
of G is said to be a strongly monolithic character if either
$\chi $
of G is said to be a strongly monolithic character if either
![]() $Z(\chi )=\textrm {ker}\,\chi $
or
$Z(\chi )=\textrm {ker}\,\chi $
or
![]() $G/\textrm {ker}\,\chi $
is a p-group whose commutator subgroup is its unique minimal normal subgroup. The strongly monolithic characters of a group were first studied in [Reference Erkoç, Güngör and Özkan3]. It is proven in [Reference Erkoç, Güngör and Özkan3] that linear characters of a group are not strongly monolithic and every nonabelian group has at least one strongly monolithic character. Moreover, by [Reference Isaacs5, Lemma 12.3], the nonlinear irreducible characters whose kernels are maximal among the kernels of all nonlinear irreducible characters of a nonabelian solvable group are monomial and strongly monolithic. Thus, every nonabelian solvable group has at least one monomial strongly monolithic character. We use the notation
$G/\textrm {ker}\,\chi $
is a p-group whose commutator subgroup is its unique minimal normal subgroup. The strongly monolithic characters of a group were first studied in [Reference Erkoç, Güngör and Özkan3]. It is proven in [Reference Erkoç, Güngör and Özkan3] that linear characters of a group are not strongly monolithic and every nonabelian group has at least one strongly monolithic character. Moreover, by [Reference Isaacs5, Lemma 12.3], the nonlinear irreducible characters whose kernels are maximal among the kernels of all nonlinear irreducible characters of a nonabelian solvable group are monomial and strongly monolithic. Thus, every nonabelian solvable group has at least one monomial strongly monolithic character. We use the notation
![]() $\textrm {cd}_{\textrm {sm}}(G)$
and
$\textrm {cd}_{\textrm {sm}}(G)$
and
![]() $\textrm {ker}_{\textrm {msm}}(G)$
to denote the set of all strongly monolithic character degrees of G and the set of all monomial strongly monolithic character kernels of G, respectively. Given
$\textrm {ker}_{\textrm {msm}}(G)$
to denote the set of all strongly monolithic character degrees of G and the set of all monomial strongly monolithic character kernels of G, respectively. Given
![]() $N\vartriangleleft G$
, we write
$N\vartriangleleft G$
, we write
![]() $\textrm {cd}(G|N)$
to denote the set of all character degrees of
$\textrm {cd}(G|N)$
to denote the set of all character degrees of
![]() $\textrm {Irr}(G|N)$
, where the set
$\textrm {Irr}(G|N)$
, where the set
![]() $\textrm {Irr}(G|N)=\textrm {Irr}(G)-\textrm {Irr}(G/N)$
.
$\textrm {Irr}(G|N)=\textrm {Irr}(G)-\textrm {Irr}(G/N)$
.
Berkovich proved in [Reference Berkovich1] that a finite group G is solvable whenever
![]() $\lvert \textrm {cd}_{\textrm {m}}(G)\rvert \leq 3$
, where
$\lvert \textrm {cd}_{\textrm {m}}(G)\rvert \leq 3$
, where
![]() $\textrm {cd}_{\textrm {m}}(G)$
is the set of all monolithic character degrees of G. Gagola and Lewis proved in [Reference Gagola and Lewis4] that a group G is nilpotent if and only if
$\textrm {cd}_{\textrm {m}}(G)$
is the set of all monolithic character degrees of G. Gagola and Lewis proved in [Reference Gagola and Lewis4] that a group G is nilpotent if and only if
![]() $\chi (1)^{2}$
divides
$\chi (1)^{2}$
divides
![]() $\lvert G:\textrm {ker}\,\chi \rvert $
for every irreducible character
$\lvert G:\textrm {ker}\,\chi \rvert $
for every irreducible character
![]() $\chi $
of G. Later, Lu et al. [Reference Lu, Qin and Liu10] generalised this theorem for monolithic characters. Motivated by these papers, we give some criteria for solvability and nilpotency of finite groups by their strongly monolithic characters.
$\chi $
of G. Later, Lu et al. [Reference Lu, Qin and Liu10] generalised this theorem for monolithic characters. Motivated by these papers, we give some criteria for solvability and nilpotency of finite groups by their strongly monolithic characters.
Theorem 1.1. Let G be a nonabelian group. Then G is solvable if one of the following conditions holds:
-
(i)
 $\lvert \mathrm{cd}_{\mathrm{sm}}(G)\rvert \leq 2$
;
$\lvert \mathrm{cd}_{\mathrm{sm}}(G)\rvert \leq 2$
; -
(ii)
 $\chi (1)$
is a prime for every strongly monolithic character
$\chi (1)$
is a prime for every strongly monolithic character
 $\chi $
of G;
$\chi $
of G; -
(iii) every strongly monolithic character of G is monomial.
The next result generalises the theorem of Gagola and Lewis in [Reference Gagola and Lewis4] by considering only strongly monolithic characters.
Theorem 1.2. Let G be a nonabelian group.
-
(i) G is nilpotent if and only if
 $\chi (1)^{2}$
divides
$\chi (1)^{2}$
divides
 $\lvert G:\mathrm{ker}\,\chi \rvert $
for every strongly monolithic character
$\lvert G:\mathrm{ker}\,\chi \rvert $
for every strongly monolithic character
 $\chi $
of G.
$\chi $
of G. -
(ii) If G is solvable, then G is nilpotent if and only if
 $\chi (1)^{2}$
divides
$\chi (1)^{2}$
divides
 $\lvert G:\mathrm{ker}\,\chi \rvert $
for every monomial strongly monolithic character
$\lvert G:\mathrm{ker}\,\chi \rvert $
for every monomial strongly monolithic character
 $\chi $
of G.
$\chi $
of G.
Theorem 1.3. Let G be a solvable group.
-
(i) If
 $\lvert \mathrm{ker}_{\mathrm{msm}}(G)\rvert =1$
, then
$\lvert \mathrm{ker}_{\mathrm{msm}}(G)\rvert =1$
, then
 $G'$
is nilpotent.
$G'$
is nilpotent. -
(ii) If
 $\lvert \mathrm{ker}_{\mathrm{msm}}(G)\rvert =2$
, then
$\lvert \mathrm{ker}_{\mathrm{msm}}(G)\rvert =2$
, then
 $G'$
is meta-nilpotent.
$G'$
is meta-nilpotent.
2 Proofs of the main theorems
Let G be a group,
![]() $N\vartriangleleft G$
and
$N\vartriangleleft G$
and
![]() $\chi \in \textrm {Irr}(G)$
such that
$\chi \in \textrm {Irr}(G)$
such that
![]() $N\subseteq \textrm {ker}\,\chi $
. Since there exists a one-to-one correspondence between irreducible characters of
$N\subseteq \textrm {ker}\,\chi $
. Since there exists a one-to-one correspondence between irreducible characters of
![]() $G/N$
and irreducible characters of G with kernel containing N, it follows that
$G/N$
and irreducible characters of G with kernel containing N, it follows that
![]() $\chi $
is a strongly monolithic character of G if and only if it is a strongly monolithic character of
$\chi $
is a strongly monolithic character of G if and only if it is a strongly monolithic character of
![]() $G/N$
. We use this fact in the proofs.
$G/N$
. We use this fact in the proofs.
Proof of Theorem 1.1
Let G be a minimal counterexample for all the situations. Suppose that G has two distinct minimal normal subgroups
![]() $N_{1}$
and
$N_{1}$
and
![]() $N_{2}$
. Then, both
$N_{2}$
. Then, both
![]() $G/N_{1}$
and
$G/N_{1}$
and
![]() $G/N_{2}$
are solvable groups by induction. However, G is isomorphic to a subgroup of
$G/N_{2}$
are solvable groups by induction. However, G is isomorphic to a subgroup of
![]() $G/N_{1}\times G/N_{2}$
, in contrast to the assumption that G is not solvable. Thus, G has a unique minimal normal subgroup in every situation of Theorem 1.1 and so it has at least one faithful irreducible character. Let N be the unique minimal normal subgroup of G. It follows that N is not solvable and
$G/N_{1}\times G/N_{2}$
, in contrast to the assumption that G is not solvable. Thus, G has a unique minimal normal subgroup in every situation of Theorem 1.1 and so it has at least one faithful irreducible character. Let N be the unique minimal normal subgroup of G. It follows that N is not solvable and
![]() $N'=N$
because
$N'=N$
because
![]() $G/N$
is a solvable group by induction. Moreover, all faithful irreducible characters of G are strongly monolithic since
$G/N$
is a solvable group by induction. Moreover, all faithful irreducible characters of G are strongly monolithic since
![]() $Z(G)=1$
.
$Z(G)=1$
.
Suppose that
![]() $\lvert \textrm {cd}_{\textrm {sm}}(G)\rvert \leq 2$
. Then
$\lvert \textrm {cd}_{\textrm {sm}}(G)\rvert \leq 2$
. Then
![]() $\lvert \textrm {cd}(G|N)\rvert \leq 2$
since
$\lvert \textrm {cd}(G|N)\rvert \leq 2$
since
![]() $\textrm {cd}(G|N)\subseteq \textrm {cd}_{\textrm {sm}}(G)$
. It follows from [Reference Isaacs and Knutson8, Theorem B] that N is solvable. This contradiction completes the proof of (i).
$\textrm {cd}(G|N)\subseteq \textrm {cd}_{\textrm {sm}}(G)$
. It follows from [Reference Isaacs and Knutson8, Theorem B] that N is solvable. This contradiction completes the proof of (i).
Now, let
![]() $\nu \in \textrm {Irr}(N)$
and
$\nu \in \textrm {Irr}(N)$
and
![]() $\nu (1)>1$
. Then, there exists an irreducible character
$\nu (1)>1$
. Then, there exists an irreducible character
![]() $\psi $
of G such that
$\psi $
of G such that
![]() $[\psi ,\nu ^{G}]\ne 0$
. Thus,
$[\psi ,\nu ^{G}]\ne 0$
. Thus,
![]() $\textrm {ker}\,\psi _{N}=N\cap \textrm {ker}\,\psi \leq \textrm {ker}\,\nu <N$
because
$\textrm {ker}\,\psi _{N}=N\cap \textrm {ker}\,\psi \leq \textrm {ker}\,\nu <N$
because
![]() $[\psi _{N},\nu ]\neq 0$
by Frobenius reciprocity. Hence,
$[\psi _{N},\nu ]\neq 0$
by Frobenius reciprocity. Hence,
![]() $\textrm {ker}\,\psi =1$
since N is the unique minimal normal subgroup of G and
$\textrm {ker}\,\psi =1$
since N is the unique minimal normal subgroup of G and
![]() $\textrm {ker}\,\psi _{N}<N$
. Therefore,
$\textrm {ker}\,\psi _{N}<N$
. Therefore,
![]() $\psi $
is a strongly monolithic character of G. Also, from Clifford’s theorem,
$\psi $
is a strongly monolithic character of G. Also, from Clifford’s theorem,
![]() $\nu (1)\mid \psi (1)$
. In the case (ii),
$\nu (1)\mid \psi (1)$
. In the case (ii),
![]() $\nu (1)=\psi (1)$
and the degrees of all nonlinear irreducible characters of N are prime numbers. It follows from [Reference Isaacs and Passman9] that N is solvable, which is a contradiction. This completes the proof of (ii).
$\nu (1)=\psi (1)$
and the degrees of all nonlinear irreducible characters of N are prime numbers. It follows from [Reference Isaacs and Passman9] that N is solvable, which is a contradiction. This completes the proof of (ii).
In the case (iii), N must be a proper subgroup of G. Otherwise, all nonlinear irreducible characters of G would be faithful and so strongly monolithic. However, this is a contradiction since all M-groups are solvable. Let
![]() $\chi $
be a faithful irreducible character of G of least degree. Then
$\chi $
be a faithful irreducible character of G of least degree. Then
![]() $\chi $
is a strongly monolithic character and so monomial by hypothesis. So there exists a linear character
$\chi $
is a strongly monolithic character and so monomial by hypothesis. So there exists a linear character
![]() $\lambda $
of a subgroup H of G such that
$\lambda $
of a subgroup H of G such that
![]() $\lambda ^{G}=\chi $
. Since
$\lambda ^{G}=\chi $
. Since
![]() $[G:H]=\chi (1)>1$
, it follows that
$[G:H]=\chi (1)>1$
, it follows that
![]() $H<G$
and
$H<G$
and
![]() $(1_{H})^{G}$
is reducible. Let
$(1_{H})^{G}$
is reducible. Let
![]() $\theta $
be an irreducible constituent of
$\theta $
be an irreducible constituent of
![]() $(1_{H})^{G}$
, so that
$(1_{H})^{G}$
, so that
![]() $\theta (1)<\chi (1)$
. By the choice of
$\theta (1)<\chi (1)$
. By the choice of
![]() $\chi $
, we deduce that
$\chi $
, we deduce that
![]() $N\leq \textrm {ker}\,\theta $
. Moreover,
$N\leq \textrm {ker}\,\theta $
. Moreover,
![]() $N\leq \textrm {ker}\,(1_{H})^{G}\leq H$
because
$N\leq \textrm {ker}\,(1_{H})^{G}\leq H$
because
![]() $N\leq \textrm {ker}\,\theta $
for every irreducible constituent
$N\leq \textrm {ker}\,\theta $
for every irreducible constituent
![]() $\theta $
of
$\theta $
of
![]() $(1_{H})^{G}$
. It follows that
$(1_{H})^{G}$
. It follows that
![]() $N\leq \textrm {ker}\,\lambda $
since
$N\leq \textrm {ker}\,\lambda $
since
![]() $N=N'$
and
$N=N'$
and
![]() $\lambda $
is a linear character of H. Furthermore,
$\lambda $
is a linear character of H. Furthermore,
![]() $N\leq (\textrm {ker}\,\lambda )^{g}$
for every
$N\leq (\textrm {ker}\,\lambda )^{g}$
for every
![]() $g\in G$
, since
$g\in G$
, since
![]() $N\vartriangleleft G$
. This gives the contradiction that
$N\vartriangleleft G$
. This gives the contradiction that
![]() $N\leq \textrm {ker}(\lambda ^{G})=\textrm {ker}\chi =1$
. This contradiction completes the proof of (iii).
$N\leq \textrm {ker}(\lambda ^{G})=\textrm {ker}\chi =1$
. This contradiction completes the proof of (iii).
A group G may not be solvable when
![]() $\lvert \textrm {cd}_{\textrm {sm}}(G)\rvert>2$
. For instance,
$\lvert \textrm {cd}_{\textrm {sm}}(G)\rvert>2$
. For instance,
![]() $\lvert \textrm {cd}_{\textrm {sm}}(S_{5})\rvert =3$
, where
$\lvert \textrm {cd}_{\textrm {sm}}(S_{5})\rvert =3$
, where
![]() $S_{5}$
is the symmetric group on five letters. Additionally, a strongly monolithic character of a solvable group may not be monomial. For example, the semidirect product
$S_{5}$
is the symmetric group on five letters. Additionally, a strongly monolithic character of a solvable group may not be monomial. For example, the semidirect product
![]() $\textrm {He}_{3}\rtimes \textrm {SD}_{16}$
(SmallGroup(432,520) in GAP), where the semidihedral group
$\textrm {He}_{3}\rtimes \textrm {SD}_{16}$
(SmallGroup(432,520) in GAP), where the semidihedral group
![]() $\textrm {SD}_{16}$
acts faithfully on the Heisenberg group
$\textrm {SD}_{16}$
acts faithfully on the Heisenberg group
![]() $\textrm {He}_{3}$
, has some strongly monolithic characters which are not monomial.
$\textrm {He}_{3}$
, has some strongly monolithic characters which are not monomial.
Proof of Theorem 1.2
If G is a nilpotent group, then by [Reference Gagola and Lewis4, Theorem A],
![]() $\chi (1)^{2}$
divides
$\chi (1)^{2}$
divides
![]() $\lvert G:\textrm {ker}\,\chi \rvert $
for every
$\lvert G:\textrm {ker}\,\chi \rvert $
for every
![]() $\chi \in \textrm {Irr}(G)$
. Thus, it is clear that the necessary conditions in both cases of Theorem 1.2 hold.
$\chi \in \textrm {Irr}(G)$
. Thus, it is clear that the necessary conditions in both cases of Theorem 1.2 hold.
Let G be a minimal counterexample for the sufficient conditions in both cases of the theorem. Since the hypotheses of Theorem 1.2 are inherited by
![]() $G/N$
for all
$G/N$
for all
![]() $N\vartriangleleft G$
, it follows that G has a unique minimal normal subgroup M and therefore G has at least one faithful irreducible character. Moreover,
$N\vartriangleleft G$
, it follows that G has a unique minimal normal subgroup M and therefore G has at least one faithful irreducible character. Moreover,
![]() $Z(G)=1=\Phi (G)$
since G is a minimal counterexample. Thus all faithful irreducible characters of G are strongly monolithic. By the hypothesis of case (i) in the theorem,
$Z(G)=1=\Phi (G)$
since G is a minimal counterexample. Thus all faithful irreducible characters of G are strongly monolithic. By the hypothesis of case (i) in the theorem,
![]() $\theta (1)^{2}\mid \lvert G:\textrm {ker}\,\theta \rvert $
for every faithful irreducible character
$\theta (1)^{2}\mid \lvert G:\textrm {ker}\,\theta \rvert $
for every faithful irreducible character
![]() $\theta $
of G. Moreover,
$\theta $
of G. Moreover,
![]() $\chi (1)^{2}\mid \lvert G:\textrm {ker}\,\chi \rvert $
for every
$\chi (1)^{2}\mid \lvert G:\textrm {ker}\,\chi \rvert $
for every
![]() $\chi \in \textrm {Irr}(G)$
, because
$\chi \in \textrm {Irr}(G)$
, because
![]() $G/M$
is nilpotent by induction. Thus, by [Reference Gagola and Lewis4, Theorem A], G is nilpotent, which is a contradiction. This contradiction completes the proof of (i).
$G/M$
is nilpotent by induction. Thus, by [Reference Gagola and Lewis4, Theorem A], G is nilpotent, which is a contradiction. This contradiction completes the proof of (i).
Since
![]() $\Phi (G)=1$
, there exists a subgroup H of G such that
$\Phi (G)=1$
, there exists a subgroup H of G such that
![]() $G=MH$
and
$G=MH$
and
![]() $M\cap H=1$
. Then H is nilpotent by induction. Using Gaschütz’s theorem,
$M\cap H=1$
. Then H is nilpotent by induction. Using Gaschütz’s theorem,
![]() $(\lvert M\rvert ,\lvert H\rvert )=1$
. It follows from [Reference Isaacs6, Theorem B] that there exists a
$(\lvert M\rvert ,\lvert H\rvert )=1$
. It follows from [Reference Isaacs6, Theorem B] that there exists a
![]() $\lambda \in \textrm {Irr}(M)$
with
$\lambda \in \textrm {Irr}(M)$
with
![]() $\lambda \neq 1$
such that
$\lambda \neq 1$
such that
![]() $\lvert I_{H}(\lambda )\rvert \leq (\lvert {H\rvert }/{q})^{1/q}$
, where
$\lvert I_{H}(\lambda )\rvert \leq (\lvert {H\rvert }/{q})^{1/q}$
, where
![]() $I_{H}(\lambda )$
is the inertia group of
$I_{H}(\lambda )$
is the inertia group of
![]() $\lambda $
in H and q is the smallest prime divisor of
$\lambda $
in H and q is the smallest prime divisor of
![]() $\lvert H\rvert $
. This gives the inequality:
$\lvert H\rvert $
. This gives the inequality:
 $$ \begin{align*} \lvert I_{H}(\lambda)\rvert \leq\bigg(\frac{\lvert H\rvert}{q}\bigg)^{1/q}\leq\bigg(\frac{\lvert H\rvert}{2}\bigg)^{1/2}<\lvert H\rvert^{1/2}. \end{align*} $$
$$ \begin{align*} \lvert I_{H}(\lambda)\rvert \leq\bigg(\frac{\lvert H\rvert}{q}\bigg)^{1/q}\leq\bigg(\frac{\lvert H\rvert}{2}\bigg)^{1/2}<\lvert H\rvert^{1/2}. \end{align*} $$
From [Reference Isaacs5, Problem 6.18], there exists a linear character
![]() $\psi \in \textrm {Irr}(I_{G}(\lambda ))$
such that
$\psi \in \textrm {Irr}(I_{G}(\lambda ))$
such that
![]() $\psi _{M} = \lambda $
, where
$\psi _{M} = \lambda $
, where
![]() $I_{G}(\lambda )$
is the inertia group of
$I_{G}(\lambda )$
is the inertia group of
![]() $\lambda $
in G. This implies that
$\lambda $
in G. This implies that
![]() $\psi ^{G}$
is a monomial irreducible character of G. Let
$\psi ^{G}$
is a monomial irreducible character of G. Let
![]() $\chi =\psi ^{G}$
. Then
$\chi =\psi ^{G}$
. Then
![]() $\chi $
is a faithful irreducible character of G. Otherwise,
$\chi $
is a faithful irreducible character of G. Otherwise,
![]() $M\leq \textrm {ker}\,\chi ={\bigcap }_{g\in G}(\textrm {ker}\,\psi )^{g}\leq \textrm {ker}\,\psi $
. However, this is a contradiction since
$M\leq \textrm {ker}\,\chi ={\bigcap }_{g\in G}(\textrm {ker}\,\psi )^{g}\leq \textrm {ker}\,\psi $
. However, this is a contradiction since
![]() $\psi _{M}=\lambda \neq 1$
. Therefore,
$\psi _{M}=\lambda \neq 1$
. Therefore,
![]() $\chi $
is a monomial strongly monolithic character of G. By the hypothesis of (ii),
$\chi $
is a monomial strongly monolithic character of G. By the hypothesis of (ii),
![]() $\chi (1)^{2}\mid \lvert G\rvert $
. Also
$\chi (1)^{2}\mid \lvert G\rvert $
. Also
![]() $\chi (1)\mid \lvert H\rvert $
by Ito’s theorem (see [Reference Isaacs5]). Then,
$\chi (1)\mid \lvert H\rvert $
by Ito’s theorem (see [Reference Isaacs5]). Then,
![]() $\lvert G\rvert =\lvert M\rvert \lvert I_{H}(\lambda )\rvert\kern2pt \chi (1)<\lvert M\rvert \lvert H\rvert =\lvert G\rvert $
, which is a contradiction. This completes the proof of (ii).
$\lvert G\rvert =\lvert M\rvert \lvert I_{H}(\lambda )\rvert\kern2pt \chi (1)<\lvert M\rvert \lvert H\rvert =\lvert G\rvert $
, which is a contradiction. This completes the proof of (ii).
We note that the commutator subgroup
![]() $G'$
of a solvable group G may not be nilpotent when G has only one maximal kernel among the kernels of the strongly monolithic characters of G. For example, all nonlinear irreducible characters of the symmetric group
$G'$
of a solvable group G may not be nilpotent when G has only one maximal kernel among the kernels of the strongly monolithic characters of G. For example, all nonlinear irreducible characters of the symmetric group
![]() $S_{4}$
are strongly monolithic and there is only one maximal kernel among the kernels of these characters. However, the alternating group
$S_{4}$
are strongly monolithic and there is only one maximal kernel among the kernels of these characters. However, the alternating group
![]() $A_{4}$
is not nilpotent. Also the commutator subgroup
$A_{4}$
is not nilpotent. Also the commutator subgroup
![]() $G'$
of a solvable group G may not be abelian when
$G'$
of a solvable group G may not be abelian when
![]() $\lvert \textrm {ker}_{\textrm {msm}}(G)\rvert =1$
. For instance,
$\lvert \textrm {ker}_{\textrm {msm}}(G)\rvert =1$
. For instance,
![]() $\textrm {SL}(2,3)$
has only one (monomial) strongly monolithic character and its commutator subgroup is not abelian.
$\textrm {SL}(2,3)$
has only one (monomial) strongly monolithic character and its commutator subgroup is not abelian.
Proof of Theorem 1.3
Let G be a minimal counterexample to the case (i). Assume that G has two distinct minimal normal subgroups, say
![]() $N_{1}$
and
$N_{1}$
and
![]() $N_{2}$
. It follows that
$N_{2}$
. It follows that
![]() $\lvert \textrm {ker}_{\textrm {msm}}(G/N_{i})\rvert \leq 1$
for
$\lvert \textrm {ker}_{\textrm {msm}}(G/N_{i})\rvert \leq 1$
for
![]() $i=1,2$
. Since a solvable group does not have any monomial strongly monolithic characters if and only if it is abelian, the commutator subgroups of
$i=1,2$
. Since a solvable group does not have any monomial strongly monolithic characters if and only if it is abelian, the commutator subgroups of
![]() $G/N_{1}$
and
$G/N_{1}$
and
![]() $G/N_{2}$
are nilpotent by induction. Thus,
$G/N_{2}$
are nilpotent by induction. Thus,
![]() $G'$
is nilpotent since G is isomorphic to a subgroup of
$G'$
is nilpotent since G is isomorphic to a subgroup of
![]() $G/N_{1}\times G/N_{2}$
. This contradiction shows that G has a unique minimal normal subgroup and it has at least one faithful irreducible character.
$G/N_{1}\times G/N_{2}$
. This contradiction shows that G has a unique minimal normal subgroup and it has at least one faithful irreducible character.
Let N be the unique minimal normal subgroup of G. Assume that
![]() $Z(G)>1$
. Then
$Z(G)>1$
. Then
![]() $G'Z(G)/Z(G)$
, the commutator subgroup of
$G'Z(G)/Z(G)$
, the commutator subgroup of
![]() $G/Z(G)$
, is nilpotent by induction. Therefore,
$G/Z(G)$
, is nilpotent by induction. Therefore,
![]() $G'$
is also nilpotent, which is a contradiction. Thus,
$G'$
is also nilpotent, which is a contradiction. Thus,
![]() $Z(G)$
must be trivial and so all faithful irreducible characters of G must be strongly monolithic. However,
$Z(G)$
must be trivial and so all faithful irreducible characters of G must be strongly monolithic. However,
![]() $\Phi (G)=1$
. Otherwise,
$\Phi (G)=1$
. Otherwise,
![]() $G'\Phi (G)/\Phi (G)$
would be nilpotent by induction. Thus,
$G'\Phi (G)/\Phi (G)$
would be nilpotent by induction. Thus,
![]() $G'\Phi (G)$
would be nilpotent by [Reference Isaacs7, Theorem 8.24], which is a contradiction. Since
$G'\Phi (G)$
would be nilpotent by [Reference Isaacs7, Theorem 8.24], which is a contradiction. Since
![]() $\Phi (G)=1$
, there is a subgroup H of G such that
$\Phi (G)=1$
, there is a subgroup H of G such that
![]() $G=NH$
and
$G=NH$
and
![]() $N\cap H=1$
. Let
$N\cap H=1$
. Let
![]() $1_{N}\neq \lambda \in \textrm {Irr}(N)$
. From [Reference Isaacs5, Problem 6.18], there exists a linear character
$1_{N}\neq \lambda \in \textrm {Irr}(N)$
. From [Reference Isaacs5, Problem 6.18], there exists a linear character
![]() $\theta \in \textrm {Irr}(I_{G}(\lambda ))$
such that
$\theta \in \textrm {Irr}(I_{G}(\lambda ))$
such that
![]() $\theta _{N} = \lambda $
, where
$\theta _{N} = \lambda $
, where
![]() $I_{G}(\lambda )$
is the inertia group of
$I_{G}(\lambda )$
is the inertia group of
![]() $\lambda $
in G. This implies that
$\lambda $
in G. This implies that
![]() $\theta ^{G}\in \textrm {Irr}(G)$
is a faithful monomial strongly monolithic character of G since
$\theta ^{G}\in \textrm {Irr}(G)$
is a faithful monomial strongly monolithic character of G since
![]() $\textrm {ker}\,\theta ^{G}=1$
. Since
$\textrm {ker}\,\theta ^{G}=1$
. Since
![]() $\lvert \textrm {ker}_{\textrm {msm}}(G)\rvert =1$
, the maximal kernel among the kernels of all nonlinear irreducible characters of G must be trivial. It follows that all nonlinear irreducible characters of G are faithful. Thus,
$\lvert \textrm {ker}_{\textrm {msm}}(G)\rvert =1$
, the maximal kernel among the kernels of all nonlinear irreducible characters of G must be trivial. It follows that all nonlinear irreducible characters of G are faithful. Thus,
![]() $G'$
is the unique minimal normal subgroup of G, which is a contradiction. This completes the proof of (i).
$G'$
is the unique minimal normal subgroup of G, which is a contradiction. This completes the proof of (i).
To prove (ii), let G again be a minimal counterexample. Then G has a unique minimal normal subgroup N. Assume that
![]() $Z(G)>1$
. Then
$Z(G)>1$
. Then
![]() $G'Z(G)/Z(G)$
is meta-nilpotent by induction. Thus, there is a normal subgroup A of
$G'Z(G)/Z(G)$
is meta-nilpotent by induction. Thus, there is a normal subgroup A of
![]() $G'Z(G)$
such that both
$G'Z(G)$
such that both
![]() $G'Z(G)/A$
and
$G'Z(G)/A$
and
![]() $A/Z(G)$
are nilpotent. Since
$A/Z(G)$
are nilpotent. Since
![]() $A/Z(G)$
is nilpotent, A is nilpotent and so
$A/Z(G)$
is nilpotent, A is nilpotent and so
![]() $A\cap G'$
is nilpotent. Moreover,
$A\cap G'$
is nilpotent. Moreover,
![]() $G'/(A\cap G')$
is nilpotent because
$G'/(A\cap G')$
is nilpotent because
![]() $G'/(A\cap G')\cong G'Z(G)/A$
. Then
$G'/(A\cap G')\cong G'Z(G)/A$
. Then
![]() $G'$
is also meta-nilpotent, which is a contradiction. Hence,
$G'$
is also meta-nilpotent, which is a contradiction. Hence,
![]() $Z(G)=1$
. Similarly, it is clear that
$Z(G)=1$
. Similarly, it is clear that
![]() $\Phi (G)=1$
. Therefore, G has a faithful monomial strongly monolithic character
$\Phi (G)=1$
. Therefore, G has a faithful monomial strongly monolithic character
![]() $\chi $
as in the proof of (i). Since
$\chi $
as in the proof of (i). Since
![]() $\lvert \textrm {ker}_{\textrm {msm}}(G)\rvert =2$
, we see that
$\lvert \textrm {ker}_{\textrm {msm}}(G)\rvert =2$
, we see that
![]() $\lvert \textrm {ker}_{\textrm {msm}}(G/N)\rvert =1$
. Thus,
$\lvert \textrm {ker}_{\textrm {msm}}(G/N)\rvert =1$
. Thus,
![]() $G'/N$
is nilpotent by (i) of the theorem, that is,
$G'/N$
is nilpotent by (i) of the theorem, that is,
![]() $G'$
is meta-nilpotent. This contradiction completes the proof of (ii).
$G'$
is meta-nilpotent. This contradiction completes the proof of (ii).