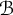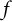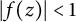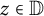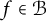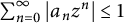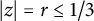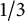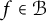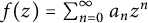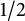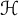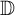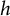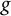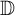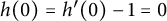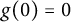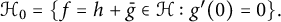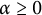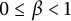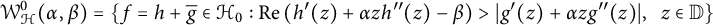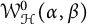1 Introduction
Harmonic mappings play the natural role in parameterizing minimal surfaces in the context of differential geometry. Planner harmonic mappings have application not only in the differential geometry but also in the various field of engineering, physics, operations research, and other intriguing aspects of applied mathematics. The theory of harmonic functions has been used to study and solve fluid flow problems (see [Reference Aleman and Constantin10]). The theory of univalent harmonic functions having prominent geometric properties like starlikeness, convexity, and close-to-convexity appear naturally while dealing with planner fluid dynamical problems. For instance, the fluid flow problem on a convex domain satisfying an interesting geometric property has been extensively studied by Aleman and Constantin [Reference Aleman and Constantin10]. With the help of geometric properties of harmonic mappings, Constantin and Martin [Reference Constantin and Martin19] have obtained a complete solution of classifying all two-dimensional fluid flows.
In this paper, our purpose is to investigate several Bohr-type inequalities which will be harmonic analog of the inequalities for bounded analytic functions. Below, we first recall the classical result of Harald Bohr discovered in
![]() $ 1914 $
, which generates intensive research activity, what is called Bohr phenomenon.
$ 1914 $
, which generates intensive research activity, what is called Bohr phenomenon.
Let
![]() $ \mathcal {B} $
denote the class of analytic functions in the unit disk
$ \mathcal {B} $
denote the class of analytic functions in the unit disk
![]() $ \mathbb {D}:=\{z\in \mathbb {C} : |z|<1\} $
of the form
$ \mathbb {D}:=\{z\in \mathbb {C} : |z|<1\} $
of the form
![]() $ f(z)=\sum _{n=0}^{\infty }a_nz^n $
such that
$ f(z)=\sum _{n=0}^{\infty }a_nz^n $
such that
![]() $ |f(z)|<1 $
in
$ |f(z)|<1 $
in
![]() $ \mathbb {D} $
. In the study of Dirichlet series, in
$ \mathbb {D} $
. In the study of Dirichlet series, in
![]() $ 1914 $
, Bohr [Reference Bohr17] discovered the following interesting phenomenon that if
$ 1914 $
, Bohr [Reference Bohr17] discovered the following interesting phenomenon that if
![]() $ f\in \mathcal {B} $
, then its associated majorant series:
$ f\in \mathcal {B} $
, then its associated majorant series:
 $$ \begin{align*} M_f(r):=\sum_{n=0}^{\infty}|a_n|r^n\leq 1\;\;\mbox{for}\;\; |z|=r\leq \frac{1}{3}. \end{align*} $$
$$ \begin{align*} M_f(r):=\sum_{n=0}^{\infty}|a_n|r^n\leq 1\;\;\mbox{for}\;\; |z|=r\leq \frac{1}{3}. \end{align*} $$
The constant
![]() $ 1/3 $
, known as the Bohr radius for the class
$ 1/3 $
, known as the Bohr radius for the class
![]() $ \mathcal {B} $
, is the best possible. Furthermore, for
$ \mathcal {B} $
, is the best possible. Furthermore, for
![]() $ \psi _a(z)={(a-z)}/{(1-az)},\; a\in [0,1), $
it follows easily that
$ \psi _a(z)={(a-z)}/{(1-az)},\; a\in [0,1), $
it follows easily that
![]() $ {M_{\psi _a}(r)>1 }$
if, and only if,
$ {M_{\psi _a}(r)>1 }$
if, and only if,
![]() $ r>1/(1+2a) $
, and hence the radius
$ r>1/(1+2a) $
, and hence the radius
![]() $ 1/3 $
is optimal as
$ 1/3 $
is optimal as
![]() $ a\rightarrow 1 $
. For the recent advancements of the Bohr-type inequalities, we refer to the articles [Reference Huang, Liu and Ponnusamy26, Reference Kumar35, Reference Liu38, Reference Ponnusamy, Vijayakumar and Wirths44, Reference Ponnusamy, Vijayakumar and Wirths45] and references therein.
$ a\rightarrow 1 $
. For the recent advancements of the Bohr-type inequalities, we refer to the articles [Reference Huang, Liu and Ponnusamy26, Reference Kumar35, Reference Liu38, Reference Ponnusamy, Vijayakumar and Wirths44, Reference Ponnusamy, Vijayakumar and Wirths45] and references therein.
The Bohr inequality can be written in the following distance formulation:
 $$ \begin{align} d\left(\sum_{n=0}^{\infty}|a_nz^n|,|a_0|\right)=\sum_{n=1}^{\infty}|a_nz^n|\leq 1- |f(0)|=d(f(0),\partial \mathbb{D}), \end{align} $$
$$ \begin{align} d\left(\sum_{n=0}^{\infty}|a_nz^n|,|a_0|\right)=\sum_{n=1}^{\infty}|a_nz^n|\leq 1- |f(0)|=d(f(0),\partial \mathbb{D}), \end{align} $$
for
![]() $ |z|=r\leq 1/3 $
and the constant
$ |z|=r\leq 1/3 $
and the constant
![]() $ 1/3 $
is independent on the coefficients of the Taylor series of
$ 1/3 $
is independent on the coefficients of the Taylor series of
![]() $ f $
, where
$ f $
, where
![]() $ d $
is the Euclidean distance and
$ d $
is the Euclidean distance and
![]() $ \partial f(\mathbb {D}) $
is the boundary of
$ \partial f(\mathbb {D}) $
is the boundary of
![]() $ f(\mathbb {D}) $
. In view of the distance form (1.1), the notion of the Bohr phenomenon can be generalized to a class
$ f(\mathbb {D}) $
. In view of the distance form (1.1), the notion of the Bohr phenomenon can be generalized to a class
![]() $ \mathcal {G} $
consisting of analytic functions
$ \mathcal {G} $
consisting of analytic functions
![]() $ f $
in
$ f $
in
![]() $ \mathbb {D} $
which take values in a given domain
$ \mathbb {D} $
which take values in a given domain
![]() $ \Omega \subseteq \mathbb {C} $
such that
$ \Omega \subseteq \mathbb {C} $
such that
![]() $ f(\mathbb {D})\subset \Omega $
and the class
$ f(\mathbb {D})\subset \Omega $
and the class
![]() $ \mathcal {G} $
is said to satisfy the Bohr phenomenon if there exists largest radius
$ \mathcal {G} $
is said to satisfy the Bohr phenomenon if there exists largest radius
![]() $ r_{\Omega }\in (0,1) $
such that the inequality (1.1) holds for
$ r_{\Omega }\in (0,1) $
such that the inequality (1.1) holds for
![]() $ |z|=r\leq r_{\Omega } $
and for all functions
$ |z|=r\leq r_{\Omega } $
and for all functions
![]() $ f\in \mathcal {G} $
. The largest radius
$ f\in \mathcal {G} $
. The largest radius
![]() $ r_{\Omega } $
is called the Bohr radius for the class
$ r_{\Omega } $
is called the Bohr radius for the class
![]() $ \mathcal {G} $
.
$ \mathcal {G} $
.
A complex-valued function
![]() $ f(z)=u(x,y)+iv(x,y) $
is called harmonic in
$ f(z)=u(x,y)+iv(x,y) $
is called harmonic in
![]() $ \mathbb {D} $
if both
$ \mathbb {D} $
if both
![]() $ u $
and
$ u $
and
![]() $ v $
satisfy the Laplace’s equation
$ v $
satisfy the Laplace’s equation
![]() $ \bigtriangledown ^2 u=0 $
and
$ \bigtriangledown ^2 u=0 $
and
![]() $ \bigtriangledown ^2 v=0 $
, where
$ \bigtriangledown ^2 v=0 $
, where
 $$ \begin{align*} \bigtriangledown^2:=\frac{\partial^2}{\partial x^2}+\frac{\partial^2}{\partial y^2}. \end{align*} $$
$$ \begin{align*} \bigtriangledown^2:=\frac{\partial^2}{\partial x^2}+\frac{\partial^2}{\partial y^2}. \end{align*} $$
It is well known that under the assumption
![]() $ g(0)=0 $
, the harmonic function
$ g(0)=0 $
, the harmonic function
![]() $ f $
has the unique canonical representation
$ f $
has the unique canonical representation
![]() $ f=h+\overline {g} $
, where
$ f=h+\overline {g} $
, where
![]() $ h $
and
$ h $
and
![]() $ g $
are analytic functions in
$ g $
are analytic functions in
![]() $ \mathbb {D} $
, respectively called, analytic and co-analytic parts of
$ \mathbb {D} $
, respectively called, analytic and co-analytic parts of
![]() $ f $
. If, in addition,
$ f $
. If, in addition,
![]() $ f $
is univalent, then we say that
$ f $
is univalent, then we say that
![]() $ f $
is univalent harmonic on a domain
$ f $
is univalent harmonic on a domain
![]() $ \Omega $
. A locally univalent harmonic mapping
$ \Omega $
. A locally univalent harmonic mapping
![]() $ f=h+\overline {g} $
is sense-preserving whenever its Jacobian
$ f=h+\overline {g} $
is sense-preserving whenever its Jacobian
![]() $ J_f(z):=|f_{z}(z)|^2-|f_{\bar {z}}(z)|=|h^{\prime }(z)|^2-|g^{\prime }(z)|^2>0 $
for
$ J_f(z):=|f_{z}(z)|^2-|f_{\bar {z}}(z)|=|h^{\prime }(z)|^2-|g^{\prime }(z)|^2>0 $
for
![]() $ z\in \mathbb {D} $
.
$ z\in \mathbb {D} $
.
Let
![]() $ \mathcal {H} $
be the class of all complex-valued harmonic functions
$ \mathcal {H} $
be the class of all complex-valued harmonic functions
![]() $ f=h+\bar {g} $
defined on the unit disk
$ f=h+\bar {g} $
defined on the unit disk
![]() $ \mathbb {D} $
, where
$ \mathbb {D} $
, where
![]() $ h $
and
$ h $
and
![]() $ g $
are analytic
$ g $
are analytic
![]() $ \mathbb {D} $
with the normalization
$ \mathbb {D} $
with the normalization
![]() $ h(0)=h^{\prime }(0)-1=0 $
and
$ h(0)=h^{\prime }(0)-1=0 $
and
![]() $ g(0)=0 $
. Let
$ g(0)=0 $
. Let
![]() $ \mathcal {H}_0 $
be defined by
$ \mathcal {H}_0 $
be defined by
![]() $ \mathcal {H}_0=\{f=h+\bar {g}\in \mathcal {H} : g^{\prime }(0)=0\}. $
Then each
$ \mathcal {H}_0=\{f=h+\bar {g}\in \mathcal {H} : g^{\prime }(0)=0\}. $
Then each
![]() $ f=h+\bar {g}\in \mathcal {H}_0 $
has the following form:
$ f=h+\bar {g}\in \mathcal {H}_0 $
has the following form:
 $$ \begin{align} h(z)=z+\sum_{n=2}^{\infty}a_nz^n\quad\mbox{and}\quad g(z)=\sum_{n=1}^{\infty}b_nz^n. \end{align} $$
$$ \begin{align} h(z)=z+\sum_{n=2}^{\infty}a_nz^n\quad\mbox{and}\quad g(z)=\sum_{n=1}^{\infty}b_nz^n. \end{align} $$
In 2013, Ponnusamy et al. [Reference Ponnusamy, Yamamoto and Yanagihara46] considered the following class of harmonic mappings:
and motivated by the class
![]() $\mathcal {P}_{\mathcal {H}}^{0}$
, Li and Ponnusamy [Reference Li and Ponnusamy36] have studied the class
$\mathcal {P}_{\mathcal {H}}^{0}$
, Li and Ponnusamy [Reference Li and Ponnusamy36] have studied the class
![]() $\mathcal {P}_{\mathcal {H}}^{0}(\alpha )$
defined by
$\mathcal {P}_{\mathcal {H}}^{0}(\alpha )$
defined by
It is easy to see that the class
![]() $ \mathcal {H} $
reduces to the class
$ \mathcal {H} $
reduces to the class
![]() $ \mathcal {A} $
of normalized analytic functions if the co-analytic part of
$ \mathcal {A} $
of normalized analytic functions if the co-analytic part of
![]() $ f $
is zero. A function
$ f $
is zero. A function
![]() $ h\in \mathcal {A} $
is called close-to-convex in
$ h\in \mathcal {A} $
is called close-to-convex in
![]() $ \mathbb {D} $
if the complement of
$ \mathbb {D} $
if the complement of
![]() $ h(\mathbb {D}) $
can be written as the union of nonintersecting half lines. A function
$ h(\mathbb {D}) $
can be written as the union of nonintersecting half lines. A function
![]() $ h\in \mathcal {A} $
is said to be close-to-convex function of order
$ h\in \mathcal {A} $
is said to be close-to-convex function of order
![]() $ \beta \; (0\leq \beta <1) $
if
$ \beta \; (0\leq \beta <1) $
if
![]() $ \mathrm {Re}\left (h^{\prime }(z)\right )>\beta $
for
$ \mathrm {Re}\left (h^{\prime }(z)\right )>\beta $
for
![]() $ z\in \mathbb {D} $
(see [Reference Rajbala and Prajapat47]). For
$ z\in \mathbb {D} $
(see [Reference Rajbala and Prajapat47]). For
![]() $ \alpha \geq 0 $
and
$ \alpha \geq 0 $
and
![]() $ 0\leq \beta <1 $
, let
$ 0\leq \beta <1 $
, let
The class
![]() $ \mathcal {W}(\alpha , \beta ) $
has been studied by Gao and Zhou [Reference Gao and Zhou23] for
$ \mathcal {W}(\alpha , \beta ) $
has been studied by Gao and Zhou [Reference Gao and Zhou23] for
![]() $ \beta <1 $
and
$ \beta <1 $
and
![]() $ \alpha>1 $
.
$ \alpha>1 $
.
In
![]() $ 1977 $
, Chichra [Reference Chichra18] introduced the following class
$ 1977 $
, Chichra [Reference Chichra18] introduced the following class
![]() $ \mathcal {W}(\alpha ) $
for
$ \mathcal {W}(\alpha ) $
for
![]() $ \alpha \geq 0 $
:
$ \alpha \geq 0 $
:
Moreover, Chichra [Reference Chichra18] has proved that functions in the class
![]() $ \mathcal {W}(\alpha ) $
constitute a subclass of close-to-convex functions in
$ \mathcal {W}(\alpha ) $
constitute a subclass of close-to-convex functions in
![]() $ \mathbb {D} $
. In
$ \mathbb {D} $
. In
![]() $ 2014 $
, Nagpal and Ravichandran [Reference Nagpal and Ravinchandran42] studied a new subclass
$ 2014 $
, Nagpal and Ravichandran [Reference Nagpal and Ravinchandran42] studied a new subclass
![]() $ \mathcal {W}^{0}_{\mathcal {H}} $
of univalent harmonic mappings and obtained the coefficient bounds for functions in the class
$ \mathcal {W}^{0}_{\mathcal {H}} $
of univalent harmonic mappings and obtained the coefficient bounds for functions in the class
![]() $ \mathcal {W}^{0}_{\mathcal {H}} $
, where
$ \mathcal {W}^{0}_{\mathcal {H}} $
, where
In
![]() $ 2019 $
, Ghosh and Allu [Reference Ghosh and Allu24] studied the class
$ 2019 $
, Ghosh and Allu [Reference Ghosh and Allu24] studied the class
![]() $ \mathcal {W}^{0}_{\mathcal {H}}(\alpha ) $
, where
$ \mathcal {W}^{0}_{\mathcal {H}}(\alpha ) $
, where
Chichra [Reference Chichra18] has shown that if
![]() $ 0\leq \beta <\alpha $
, then
$ 0\leq \beta <\alpha $
, then
![]() $ \mathcal {W}(\alpha )\subset \mathcal {W}(\beta ) $
. Hence, it is easy to see that
$ \mathcal {W}(\alpha )\subset \mathcal {W}(\beta ) $
. Hence, it is easy to see that
![]() $ \mathcal {W}^{0}_{\mathcal {H}}(\alpha )\subset \mathcal {W}^{0}_{\mathcal {H}}(\beta ) $
if
$ \mathcal {W}^{0}_{\mathcal {H}}(\alpha )\subset \mathcal {W}^{0}_{\mathcal {H}}(\beta ) $
if
![]() $ 0\leq \beta <\alpha $
. Therefore, for
$ 0\leq \beta <\alpha $
. Therefore, for
![]() $ \alpha \geq 1 $
,
$ \alpha \geq 1 $
,
![]() $\mathcal {W}^{0}_{\mathcal {H}}(\alpha )\subseteq \mathcal {W}^{0}_{\mathcal {H}}(1)$
. Since functions in
$\mathcal {W}^{0}_{\mathcal {H}}(\alpha )\subseteq \mathcal {W}^{0}_{\mathcal {H}}(1)$
. Since functions in
![]() $ \mathcal {W}^{0}_{\mathcal {H}}(1) $
are starlike, it follows that functions in
$ \mathcal {W}^{0}_{\mathcal {H}}(1) $
are starlike, it follows that functions in
![]() $ \mathcal {W}^{0}_{\mathcal {H}}(\alpha ) $
are starlike for
$ \mathcal {W}^{0}_{\mathcal {H}}(\alpha ) $
are starlike for
![]() $ \alpha \geq 1 $
. For
$ \alpha \geq 1 $
. For
![]() $ \alpha \geq 0 $
and
$ \alpha \geq 0 $
and
![]() $ 0\leq \beta <1 $
, let
$ 0\leq \beta <1 $
, let
It is known that functions in
![]() $ \mathcal {W}^{0}_{\mathcal {H}}(\alpha , \beta ) $
are close-to-convex harmonic mappings (see [Reference Rajbala and Prajapat47]).
$ \mathcal {W}^{0}_{\mathcal {H}}(\alpha , \beta ) $
are close-to-convex harmonic mappings (see [Reference Rajbala and Prajapat47]).
Remark 1.1 The class
![]() $ \mathcal {W}^{0}_{\mathcal {H}}(\alpha , \beta ) $
generalizes some of the well-known classes of harmonic mappings. For example,
$ \mathcal {W}^{0}_{\mathcal {H}}(\alpha , \beta ) $
generalizes some of the well-known classes of harmonic mappings. For example,
![]() $ \mathcal {W}^{0}_{\mathcal {H}}(\alpha , 0)=\mathcal {W}^0_{\mathcal {H}}(\alpha ) $
,
$ \mathcal {W}^{0}_{\mathcal {H}}(\alpha , 0)=\mathcal {W}^0_{\mathcal {H}}(\alpha ) $
,
![]() $ \mathcal {W}^{0}_{\mathcal {H}}(0, \beta )=\mathcal {P}^0_{\mathcal {H}}(\beta ) $
,
$ \mathcal {W}^{0}_{\mathcal {H}}(0, \beta )=\mathcal {P}^0_{\mathcal {H}}(\beta ) $
,
![]() $ \mathcal {W}^{0}_{\mathcal {H}}(1, 0)=\mathcal {W}^0_{\mathcal {H}} $
, and
$ \mathcal {W}^{0}_{\mathcal {H}}(1, 0)=\mathcal {W}^0_{\mathcal {H}} $
, and
![]() $ \mathcal {W}^{0}_{\mathcal {H}}(0, 0)=\mathcal {P}^0_{\mathcal {H}} $
.
$ \mathcal {W}^{0}_{\mathcal {H}}(0, 0)=\mathcal {P}^0_{\mathcal {H}} $
.
From the following results, it is easy to see that functions in the class
![]() $ \mathcal {W}^{0}_{\mathcal {H}}(\alpha , \beta ) $
are univalent for
$ \mathcal {W}^{0}_{\mathcal {H}}(\alpha , \beta ) $
are univalent for
![]() $ \alpha \geq 0 $
and
$ \alpha \geq 0 $
and
![]() $ 0\leq \beta <1 $
, and they are closely related to functions in
$ 0\leq \beta <1 $
, and they are closely related to functions in
![]() $ \mathcal {W}(\alpha ,\beta ) $
.
$ \mathcal {W}(\alpha ,\beta ) $
.
Lemma 1.1 [Reference Rajbala and Prajapat47]
The harmonic mapping
![]() $ f=h+\bar {g} $
belongs to
$ f=h+\bar {g} $
belongs to
![]() $ \mathcal {W}^{0}_{\mathcal {H}}(\alpha , \beta ) $
if, and only if, the analytic function
$ \mathcal {W}^{0}_{\mathcal {H}}(\alpha , \beta ) $
if, and only if, the analytic function
![]() $ F_{\epsilon }=h+\epsilon g $
belongs to
$ F_{\epsilon }=h+\epsilon g $
belongs to
![]() $ \mathcal {W}(\alpha , \beta ) $
for each
$ \mathcal {W}(\alpha , \beta ) $
for each
![]() $ |\epsilon |=1. $
$ |\epsilon |=1. $
The sharp coefficient bounds and the sharp growth estimates for functions in the class
![]() $ \mathcal {W}^{0}_{\mathcal {H}}(\alpha , \beta ) $
have been studied in [Reference Rajbala and Prajapat47].
$ \mathcal {W}^{0}_{\mathcal {H}}(\alpha , \beta ) $
have been studied in [Reference Rajbala and Prajapat47].
Lemma 1.2 [Reference Rajbala and Prajapat47]
Let
![]() $ f\in \mathcal {W}^{0}_{\mathcal {H}}(\alpha , \beta ) $
for
$ f\in \mathcal {W}^{0}_{\mathcal {H}}(\alpha , \beta ) $
for
![]() $ \alpha \geq 0 $
,
$ \alpha \geq 0 $
,
![]() $ 0\leq \beta <1 $
and be of the form (1.2). Then, for any
$ 0\leq \beta <1 $
and be of the form (1.2). Then, for any
![]() $ n\geq 2 $
,
$ n\geq 2 $
,
-
(i)
 $ |a_n|+|b_n|\leq \displaystyle \frac {2(1-\beta )}{n\left (1+\alpha (n-1)\right )} $
;
$ |a_n|+|b_n|\leq \displaystyle \frac {2(1-\beta )}{n\left (1+\alpha (n-1)\right )} $
; -
(ii)
 $ ||a_n|-|b_n||\leq \displaystyle \frac {2(1-\beta )}{n\left (1+\alpha (n-1)\right )} $
;
$ ||a_n|-|b_n||\leq \displaystyle \frac {2(1-\beta )}{n\left (1+\alpha (n-1)\right )} $
; -
(iii)
 $ |a_n|\leq \displaystyle \frac {2(1-\beta )}{n\left (1+\alpha (n-1)\right )} $
.
$ |a_n|\leq \displaystyle \frac {2(1-\beta )}{n\left (1+\alpha (n-1)\right )} $
.
All these inequalities are sharp for the function
![]() $ f=f_{\alpha , \beta } $
given by
$ f=f_{\alpha , \beta } $
given by
 $$ \begin{align} f_{\alpha, \beta}(z)=z+\sum_{n=2}^{\infty}\frac{2(1-\beta){\bar{z}}^n}{n\left(1+\alpha(n-1)\right)}. \end{align} $$
$$ \begin{align} f_{\alpha, \beta}(z)=z+\sum_{n=2}^{\infty}\frac{2(1-\beta){\bar{z}}^n}{n\left(1+\alpha(n-1)\right)}. \end{align} $$
Lemma 1.3 [Reference Rajbala and Prajapat47]
Let
![]() $ f\in \mathcal {W}^{0}_{\mathcal {H}}(\alpha , \beta ) $
and be of the form (1.2) with
$ f\in \mathcal {W}^{0}_{\mathcal {H}}(\alpha , \beta ) $
and be of the form (1.2) with
![]() $ 0<\alpha \leq 1 $
and
$ 0<\alpha \leq 1 $
and
![]() $ {0\leq \beta <1 }$
. Then
$ {0\leq \beta <1 }$
. Then
 $$ \begin{align} |z|+\sum_{n=2}^{\infty}\frac{2(1-\beta)(-1)^{n-1}|z|^n}{n\left(1+\alpha(n-1)\right)}\leq |f(z)|\leq |z|+\sum_{n=2}^{\infty}\frac{2(1-\beta)|z|^n}{n\left(1+\alpha(n-1)\right)}. \end{align} $$
$$ \begin{align} |z|+\sum_{n=2}^{\infty}\frac{2(1-\beta)(-1)^{n-1}|z|^n}{n\left(1+\alpha(n-1)\right)}\leq |f(z)|\leq |z|+\sum_{n=2}^{\infty}\frac{2(1-\beta)|z|^n}{n\left(1+\alpha(n-1)\right)}. \end{align} $$
Both the inequalities are sharp for the function
![]() $ f=f_{\alpha , \beta } $
given by (1.3).
$ f=f_{\alpha , \beta } $
given by (1.3).
Bohr–Rogosinski radius is an analogous to the Bohr radius which has been defined (see [Reference Rogosinski48]) as follows: If
![]() $ f\in \mathcal {B} $
is given by
$ f\in \mathcal {B} $
is given by
![]() $ f(z)=\sum _{n=0}^{\infty }a_nz^n $
, then for an integer
$ f(z)=\sum _{n=0}^{\infty }a_nz^n $
, then for an integer
![]() $ N\geq 1 $
, we have
$ N\geq 1 $
, we have
![]() $ |S_N(z)|<1 $
in the disk
$ |S_N(z)|<1 $
in the disk
![]() $ \{z\in \mathbb {C} : |z|<1/2\} $
and the radius
$ \{z\in \mathbb {C} : |z|<1/2\} $
and the radius
![]() $ 1/2 $
is the best possible, where
$ 1/2 $
is the best possible, where
![]() $ S_N(z)=\sum _{n=0}^{N}a_nz^n $
denotes the
$ S_N(z)=\sum _{n=0}^{N}a_nz^n $
denotes the
![]() $ N $
th partial sum of
$ N $
th partial sum of
![]() $ f $
. The radius
$ f $
. The radius
![]() $ r=1/2 $
is called the Rogosinski radius. For
$ r=1/2 $
is called the Rogosinski radius. For
![]() $ f\in \mathcal {B} $
is given by
$ f\in \mathcal {B} $
is given by
![]() $ f(z)=\sum _{n=0}^{\infty }a_nz^n $
, the Bohr–Rogosinski sum
$ f(z)=\sum _{n=0}^{\infty }a_nz^n $
, the Bohr–Rogosinski sum
![]() $ R^f_N(z) $
of
$ R^f_N(z) $
of
![]() $ f $
defined by
$ f $
defined by
 $$ \begin{align} R^f_N(z):=|f(z)|+\sum_{n=N}^{\infty}|a_n|r^n,\;\; |z|=r. \end{align} $$
$$ \begin{align} R^f_N(z):=|f(z)|+\sum_{n=N}^{\infty}|a_n|r^n,\;\; |z|=r. \end{align} $$
It is worth to notice that for
![]() $ N=1$
, the quantity (1.5) is related to the classical Bohr sum in which
$ N=1$
, the quantity (1.5) is related to the classical Bohr sum in which
![]() $ |f(0)| $
is replaced by
$ |f(0)| $
is replaced by
![]() $ |f(z)| $
. The inequality
$ |f(z)| $
. The inequality
![]() $ R^f_N(z)\leq 1 $
is called Bohr–Rogosinski inequality. If
$ R^f_N(z)\leq 1 $
is called Bohr–Rogosinski inequality. If
![]() $ B $
and
$ B $
and
![]() $ R $
denote the Bohr radius and Bohr–Rogosinski radius, respectively, then
$ R $
denote the Bohr radius and Bohr–Rogosinski radius, respectively, then
![]() $ B\leq R $
because
$ B\leq R $
because
![]() $ |S_N(z)|\leq \sum _{n=0}^{N}|a_n||z|^n\leq \sum _{n=0}^{\infty }|a_n||z|^n $
.
$ |S_N(z)|\leq \sum _{n=0}^{N}|a_n||z|^n\leq \sum _{n=0}^{\infty }|a_n||z|^n $
.
In
![]() $ 2005 $
, Aizenberg et al. [Reference Aizenberg, Elin and Shoikhet8] generalized the Bohr–Rogosinski inequality for the holomorphic mappings of the open unit ball into an arbitrary convex domain as well as studied the multidimensional analog of Rogosinski’s theorem with some applications. In
$ 2005 $
, Aizenberg et al. [Reference Aizenberg, Elin and Shoikhet8] generalized the Bohr–Rogosinski inequality for the holomorphic mappings of the open unit ball into an arbitrary convex domain as well as studied the multidimensional analog of Rogosinski’s theorem with some applications. In
![]() $ 2009 $
, Aizenberg [Reference Aizenberg, Gotliv and Vidras9] proved that the abscissas of Bohr and Rogosinski for ordinary Dirichlet series, mapping the right half-plane into the bounded convex domain
$ 2009 $
, Aizenberg [Reference Aizenberg, Gotliv and Vidras9] proved that the abscissas of Bohr and Rogosinski for ordinary Dirichlet series, mapping the right half-plane into the bounded convex domain
![]() $ G\subset \mathbb {C} $
are independent of the domain. In
$ G\subset \mathbb {C} $
are independent of the domain. In
![]() $ 2012 $
, Aizenberg [Reference Aizenberg7] studied Bohr and Rogosinski radii for Hardy classes
$ 2012 $
, Aizenberg [Reference Aizenberg7] studied Bohr and Rogosinski radii for Hardy classes
![]() $ H^p $
of holomorphic functions in the unit disk
$ H^p $
of holomorphic functions in the unit disk
![]() $ \mathbb {D} $
as well as the Bohr and Rogosinski radii for the mappings of Reinhradt domains in
$ \mathbb {D} $
as well as the Bohr and Rogosinski radii for the mappings of Reinhradt domains in
![]() $ \mathbb {C}^n $
into Reinhardt domains in
$ \mathbb {C}^n $
into Reinhardt domains in
![]() $ \mathbb {C}^n $
. In
$ \mathbb {C}^n $
. In
![]() $ 2020 $
, Alkhaleefah et al. [Reference Alkhaleefah, Kayumov and Ponnusamy12] obtained Bohr–Rogosinski radius for analytic functions
$ 2020 $
, Alkhaleefah et al. [Reference Alkhaleefah, Kayumov and Ponnusamy12] obtained Bohr–Rogosinski radius for analytic functions
![]() $ f(z)=\sum _{n=0}^{\infty }a_nz^n $
in unit disk
$ f(z)=\sum _{n=0}^{\infty }a_nz^n $
in unit disk
![]() $ \mathbb {D} $
replacing the coefficient
$ \mathbb {D} $
replacing the coefficient
![]() $ a_n $
of the power series by the derivatives
$ a_n $
of the power series by the derivatives
![]() $ f^{(n)}(z)/n! $
. Recently, Huang et al. [Reference Huang, Liu and Ponnusamy25] have generalized and improved some refined versions of Bohr–Rogosinski inequalities which have been studied by Liu et al. [Reference Liu, Liu and Ponnusamy39]. In
$ f^{(n)}(z)/n! $
. Recently, Huang et al. [Reference Huang, Liu and Ponnusamy25] have generalized and improved some refined versions of Bohr–Rogosinski inequalities which have been studied by Liu et al. [Reference Liu, Liu and Ponnusamy39]. In
![]() $ 2021 $
, Kayumov et al. [Reference Kayumov, Khammatova and Ponnusamy29] investigated Bohr–Rogosinski phenomenon for analytic functions defined on
$ 2021 $
, Kayumov et al. [Reference Kayumov, Khammatova and Ponnusamy29] investigated Bohr–Rogosinski phenomenon for analytic functions defined on
![]() $ \mathbb {D} $
in a general setting and derived Bohr–Rogosinski radii for Cesáro operators on the space of bounded analytic functions. For the extensive study of Bohr phenomenon including recent developments, we refer the reader to the articles [Reference Abu Muhanna1–Reference Ahamed, Allu and Halder5, Reference Alkhaleefah, Kayumov and Ponnusamy11, Reference Allu and Halder14–Reference Boas and Khavinson16, Reference Das20–Reference Evdoridis, Ponnusamy and Rasila22, Reference Huang, Liu and Ponnusamy27, Reference Ismagilov, Kayumov and Ponnusamy28, Reference Kayumov and Ponnusamy30–Reference Kayumov and Ponnusamy32, Reference Kayumov, Ponnusamy and Shakirov34, Reference Lin, Liu and Ponnusamy37, Reference Liu and Ponnusamy40, Reference Liu and Ponnusamy41, Reference Paulsen and Singh43].
$ \mathbb {D} $
in a general setting and derived Bohr–Rogosinski radii for Cesáro operators on the space of bounded analytic functions. For the extensive study of Bohr phenomenon including recent developments, we refer the reader to the articles [Reference Abu Muhanna1–Reference Ahamed, Allu and Halder5, Reference Alkhaleefah, Kayumov and Ponnusamy11, Reference Allu and Halder14–Reference Boas and Khavinson16, Reference Das20–Reference Evdoridis, Ponnusamy and Rasila22, Reference Huang, Liu and Ponnusamy27, Reference Ismagilov, Kayumov and Ponnusamy28, Reference Kayumov and Ponnusamy30–Reference Kayumov and Ponnusamy32, Reference Kayumov, Ponnusamy and Shakirov34, Reference Lin, Liu and Ponnusamy37, Reference Liu and Ponnusamy40, Reference Liu and Ponnusamy41, Reference Paulsen and Singh43].
In
![]() $ 2017 $
, Kayumov and Ponnusamy [Reference Kayumov and Ponnusamy33] obtained the following result on the Bohr–Rogosinski radius for the analytic functions in the unit disk
$ 2017 $
, Kayumov and Ponnusamy [Reference Kayumov and Ponnusamy33] obtained the following result on the Bohr–Rogosinski radius for the analytic functions in the unit disk
![]() $ \mathbb {D} $
.
$ \mathbb {D} $
.
Theorem 1.1 [Reference Kayumov and Ponnusamy33]
Suppose that
![]() $ f(z)=\sum _{n=0}^{\infty }a_nz^n $
is analytic in the unit disk
$ f(z)=\sum _{n=0}^{\infty }a_nz^n $
is analytic in the unit disk
![]() $ \mathbb {D} $
and
$ \mathbb {D} $
and
![]() $ |f(z)|<1 $
in
$ |f(z)|<1 $
in
![]() $ \mathbb {D} $
. Then
$ \mathbb {D} $
. Then
 $$ \begin{align*} |f(z)|+\sum_{n=N}^{\infty}|a_n|r^n\leq 1\;\;\mbox{for}\;\; r\leq R_N, \end{align*} $$
$$ \begin{align*} |f(z)|+\sum_{n=N}^{\infty}|a_n|r^n\leq 1\;\;\mbox{for}\;\; r\leq R_N, \end{align*} $$
where
![]() $ R_N $
is the positive root of the equation
$ R_N $
is the positive root of the equation
![]() $ 2(1+r)r^N-(1-r^2)=0 $
. The radius
$ 2(1+r)r^N-(1-r^2)=0 $
. The radius
![]() $ R_N $
is the best possible. Moreover,
$ R_N $
is the best possible. Moreover,
 $$ \begin{align*} |f(z)|^2+\sum_{n=N}^{\infty}|a_n|r^n\leq 1\;\;\mbox{for}\;\; r\leq R^{\prime}_N, \end{align*} $$
$$ \begin{align*} |f(z)|^2+\sum_{n=N}^{\infty}|a_n|r^n\leq 1\;\;\mbox{for}\;\; r\leq R^{\prime}_N, \end{align*} $$
where
![]() $ R^{\prime }_N $
is the positive root of the equation
$ R^{\prime }_N $
is the positive root of the equation
![]() $ (1+r)r^N-(1-r^2)=0 $
. The radius
$ (1+r)r^N-(1-r^2)=0 $
. The radius
![]() $ R^{\prime }_N $
is the best possible.
$ R^{\prime }_N $
is the best possible.
Recently, Kayumov and Ponnusamy [Reference Kayumov and Ponnusamy33] have obtained another interesting result concerning Bohr–Rogosinski radius considering some power of
![]() $ z $
in
$ z $
in
![]() $ f(z) $
as the following.
$ f(z) $
as the following.
Theorem 1.2 [Reference Kayumov and Ponnusamy33]
Suppose that
![]() $ f(z)=\sum _{n=0}^{\infty }a_nz^n $
is analytic in
$ f(z)=\sum _{n=0}^{\infty }a_nz^n $
is analytic in
![]() $ \mathbb {D} $
such that
$ \mathbb {D} $
such that
![]() $ |f(z)|\leq 1 $
in
$ |f(z)|\leq 1 $
in
![]() $ \mathbb {D} $
. Then, for each
$ \mathbb {D} $
. Then, for each
![]() $ m, N\in \mathbb {N} $
,
$ m, N\in \mathbb {N} $
,
 $$ \begin{align*} |f(z^m)|+\sum_{n=N}^{\infty}|a_n|r^n\leq 1\;\; \mbox{for}\;\; r\leq R_{m,N}, \end{align*} $$
$$ \begin{align*} |f(z^m)|+\sum_{n=N}^{\infty}|a_n|r^n\leq 1\;\; \mbox{for}\;\; r\leq R_{m,N}, \end{align*} $$
where
![]() $ R_{m,N} $
is the positive root of of the equation
$ R_{m,N} $
is the positive root of of the equation
![]() $ {2r^N\left (1+r^m\right )-(1-r)\left (1-r^m\right )=0,} $
and the number
$ {2r^N\left (1+r^m\right )-(1-r)\left (1-r^m\right )=0,} $
and the number
![]() $ R_{m,N} $
cannot be improved. Moreover,
$ R_{m,N} $
cannot be improved. Moreover,
![]() $ \lim _{N\rightarrow \infty }R_{m,N}=1 $
and
$ \lim _{N\rightarrow \infty }R_{m,N}=1 $
and
![]() $\lim _{m\rightarrow \infty }R_{m,N}=A_N, $
where
$\lim _{m\rightarrow \infty }R_{m,N}=A_N, $
where
![]() $ A_N $
is the positive root of the equation
$ A_N $
is the positive root of the equation
![]() $ 2r^N=1-r $
. Also,
$ 2r^N=1-r $
. Also,
![]() $ A_1=1/3 $
and
$ A_1=1/3 $
and
![]() $ A_2=1/2 $
.
$ A_2=1/2 $
.
Study of sharp refine Bohr–Rogosinski inequalities for functions in the class
![]() $ \mathcal {B} $
is an important topic in the geometric function theory. The refine Bohr–Rogosinski inequality for the class
$ \mathcal {B} $
is an important topic in the geometric function theory. The refine Bohr–Rogosinski inequality for the class
![]() $ \mathcal {B} $
is extensively studied by Liu et al. [Reference Liu, Liu and Ponnusamy39] and Ponnusamy et al. [Reference Ponnusamy, Vijayakumar and Wirths44]. For
$ \mathcal {B} $
is extensively studied by Liu et al. [Reference Liu, Liu and Ponnusamy39] and Ponnusamy et al. [Reference Ponnusamy, Vijayakumar and Wirths44]. For
![]() $ N=1 $
, it is easy to see that
$ N=1 $
, it is easy to see that
![]() $ R_1=\sqrt {5}-2 $
and
$ R_1=\sqrt {5}-2 $
and
![]() $ R^{\prime }_1=1/3 $
. However, recently, Liu et al. [Reference Liu, Liu and Ponnusamy39] proved the following interesting result showing that the two constants
$ R^{\prime }_1=1/3 $
. However, recently, Liu et al. [Reference Liu, Liu and Ponnusamy39] proved the following interesting result showing that the two constants
![]() $ R_1 $
and
$ R_1 $
and
![]() $ R^{\prime }_1 $
can be improved for any individual function in
$ R^{\prime }_1 $
can be improved for any individual function in
![]() $ \mathcal {B} $
(in the context of Theorem 1.1 with
$ \mathcal {B} $
(in the context of Theorem 1.1 with
![]() $ N=1 $
).
$ N=1 $
).
Theorem 1.3 [Reference Liu, Liu and Ponnusamy39]
Suppose that
![]() $ f\in \mathcal {B} $
and
$ f\in \mathcal {B} $
and
![]() $ f(z)=\sum _{n=0}^{\infty }a_nz^n $
. Then
$ f(z)=\sum _{n=0}^{\infty }a_nz^n $
. Then
 $$ \begin{align*} A(z):=|f(z)|+\sum_{n=1}^{\infty}|a_n|r^n+\left(\frac{1}{1+|a_1|}+\frac{r}{1-r}\right)\sum_{n=1}^{\infty}|a_n|^2r^{2n}\leq 1 \end{align*} $$
$$ \begin{align*} A(z):=|f(z)|+\sum_{n=1}^{\infty}|a_n|r^n+\left(\frac{1}{1+|a_1|}+\frac{r}{1-r}\right)\sum_{n=1}^{\infty}|a_n|^2r^{2n}\leq 1 \end{align*} $$
for
![]() $ |z|=r\leq r_{a_0}=2/\left (3+|a_0|+\sqrt {5}\left (1+|a_0|\right )\right ) $
. The radius
$ |z|=r\leq r_{a_0}=2/\left (3+|a_0|+\sqrt {5}\left (1+|a_0|\right )\right ) $
. The radius
![]() $ r_{a_0} $
is best possible and
$ r_{a_0} $
is best possible and
![]() $ {r_{a_0}>\sqrt {5}-2} $
. Moreover,
$ {r_{a_0}>\sqrt {5}-2} $
. Moreover,
 $$ \begin{align*} B(z):=|f(z)|^2+\sum_{n=1}^{\infty}|a_n|r^n+\left(\frac{1}{1+|a_1|}+\frac{r}{1-r}\right)\sum_{n=1}^{\infty}|a_n|^2r^{2n}\leq 1 \end{align*} $$
$$ \begin{align*} B(z):=|f(z)|^2+\sum_{n=1}^{\infty}|a_n|r^n+\left(\frac{1}{1+|a_1|}+\frac{r}{1-r}\right)\sum_{n=1}^{\infty}|a_n|^2r^{2n}\leq 1 \end{align*} $$
for
![]() $ |z|=r\leq r^{\prime }_{a_0} $
, where
$ |z|=r\leq r^{\prime }_{a_0} $
, where
![]() $ r^{\prime }_{a_0} $
is the unique positive root of the equation
$ r^{\prime }_{a_0} $
is the unique positive root of the equation
The radius
![]() $ r^{\prime }_{a_0} $
is best possible and
$ r^{\prime }_{a_0} $
is best possible and
![]() $ 1/3<r^{\prime }_{a_0}<1/(2+|a_0|) $
.
$ 1/3<r^{\prime }_{a_0}<1/(2+|a_0|) $
.
Motivated from the papers [Reference Ahamed, Allu and Halder6, Reference Allu and Halder13], the main objective of this paper is to find the sharp Bohr–Rogosinski radius which are the harmonic analog of Theorems 1.1–1.3 for the class
![]() $ \mathcal {W}^0_{\mathcal {H}}(\alpha , \beta ) $
for
$ \mathcal {W}^0_{\mathcal {H}}(\alpha , \beta ) $
for
![]() $ \alpha \geq 0 $
and
$ \alpha \geq 0 $
and
![]() $ 0\leq \beta <1 $
. In Section 2, we state the main results of the paper and the proof of the main results will be discussed in Section 3.
$ 0\leq \beta <1 $
. In Section 2, we state the main results of the paper and the proof of the main results will be discussed in Section 3.
2 Main results
Using Lemmas 1.2 and 1.3, we obtain the following sharp Bohr–Rogosinski inequality and sharp Bohr–Rogosinski radius for the class
![]() $ \mathcal {W}^0_{\mathcal {H}}(\alpha , \beta ) $
.
$ \mathcal {W}^0_{\mathcal {H}}(\alpha , \beta ) $
.
Theorem 2.1 Let
![]() $ f=h+\overline {g}\in \mathcal {W}^0_{\mathcal {H}}(\alpha , \beta ) $
for
$ f=h+\overline {g}\in \mathcal {W}^0_{\mathcal {H}}(\alpha , \beta ) $
for
![]() $ \alpha \geq 0 $
and
$ \alpha \geq 0 $
and
![]() $ 0\leq \beta <1 $
, be of the form (1.2) with
$ 0\leq \beta <1 $
, be of the form (1.2) with
![]() $ b_1=0 $
. Then, for
$ b_1=0 $
. Then, for
![]() $ N\geq 2 $
,
$ N\geq 2 $
,
-
(i)
 $ |f(z)|+\displaystyle \sum _{n=N}^{\infty }\left (|a_n|+|b_n|\right )|z|^n\leq d(f(0),\partial f(\mathbb {D})) $
for
$ |f(z)|+\displaystyle \sum _{n=N}^{\infty }\left (|a_n|+|b_n|\right )|z|^n\leq d(f(0),\partial f(\mathbb {D})) $
for
 $ |z|=r\leq R_N(\alpha ,\beta ) $
, where
$ |z|=r\leq R_N(\alpha ,\beta ) $
, where
 $ R_N(\alpha ,\beta ) $
is the unique root of
$ R_N(\alpha ,\beta ) $
is the unique root of  $$ \begin{align*} r+\sum_{n=2}^{\infty}\frac{2(1-\beta)r^n}{n\left(1+\alpha(n-1)\right)}+\sum_{n=N}^{\infty}\frac{2(1-\beta)r^n}{n\left(1+\alpha(n-1)\right)}-1-\sum_{n=2}^{\infty}\frac{2(1-\beta)(-1)^{n-1}}{n\left(1+\alpha(n-1)\right)}=0. \end{align*} $$
$$ \begin{align*} r+\sum_{n=2}^{\infty}\frac{2(1-\beta)r^n}{n\left(1+\alpha(n-1)\right)}+\sum_{n=N}^{\infty}\frac{2(1-\beta)r^n}{n\left(1+\alpha(n-1)\right)}-1-\sum_{n=2}^{\infty}\frac{2(1-\beta)(-1)^{n-1}}{n\left(1+\alpha(n-1)\right)}=0. \end{align*} $$
Here,
 $ R_N(\alpha ,\beta ) $
is the best possible.
$ R_N(\alpha ,\beta ) $
is the best possible. -
(ii)
 $ \displaystyle |f(z)|^2+\sum _{n=N}^{\infty }\left (|a_n|+|b_n|\right )|z|^n\leq d(f(0),\partial f(\mathbb {D})) $
for
$ \displaystyle |f(z)|^2+\sum _{n=N}^{\infty }\left (|a_n|+|b_n|\right )|z|^n\leq d(f(0),\partial f(\mathbb {D})) $
for
 $ |z|=r\leq R^{\prime }_N(\alpha ,\beta ) $
, where
$ |z|=r\leq R^{\prime }_N(\alpha ,\beta ) $
, where
 $ R^{\prime }_N(\alpha ,\beta ) $
is the unique root of
$ R^{\prime }_N(\alpha ,\beta ) $
is the unique root of  $$ \begin{align*} \left(\kern-1.2pt r+\sum_{n=2}^{\infty}\frac{2(1-\beta)r^n}{n\left(1+\alpha(n-1)\right)}\kern-1.2pt\right)^{\kern-1pt2}{+}\kern1pt\sum_{n=N}^{\infty}\frac{2(1-\beta)r^n}{n\left(1+\alpha(n-1)\right)}\kern1pt{-}\kern1pt1\kern1pt{-}\kern1pt\sum_{n=2}^{\infty}\frac{2(1-\beta)(-1)^{n-1}}{n\left(1+\alpha(n-1)\right)}\kern1pt{=}\kern1.2pt0. \end{align*} $$
$$ \begin{align*} \left(\kern-1.2pt r+\sum_{n=2}^{\infty}\frac{2(1-\beta)r^n}{n\left(1+\alpha(n-1)\right)}\kern-1.2pt\right)^{\kern-1pt2}{+}\kern1pt\sum_{n=N}^{\infty}\frac{2(1-\beta)r^n}{n\left(1+\alpha(n-1)\right)}\kern1pt{-}\kern1pt1\kern1pt{-}\kern1pt\sum_{n=2}^{\infty}\frac{2(1-\beta)(-1)^{n-1}}{n\left(1+\alpha(n-1)\right)}\kern1pt{=}\kern1.2pt0. \end{align*} $$
Here,
 $ R^{\prime }_N(\alpha ,\beta ) $
is best possible.
$ R^{\prime }_N(\alpha ,\beta ) $
is best possible.
Remark 2.1 For some particular values of
![]() $ \alpha $
and
$ \alpha $
and
![]() $ \beta $
, a simple computation gives the following Bohr–Rogosinski radii (Figure 1):
$ \beta $
, a simple computation gives the following Bohr–Rogosinski radii (Figure 1):
-
(i)
 $ R_2(0.5, 0)\approx 0.347966 $
,
$ R_2(0.5, 0)\approx 0.347966 $
,
 $ R_2(0.5, 0.2)\approx 0.408906 $
,
$ R_2(0.5, 0.2)\approx 0.408906 $
,
 $ R_2(0.5, 0.3)\approx 0.442732 $
,
$ R_2(0.5, 0.3)\approx 0.442732 $
,
 $ R_2(0.5, 0.7)\approx 0.625477 $
,
$ R_2(0.5, 0.7)\approx 0.625477 $
,
 $ R_2(0.5, 0.9)\approx 0.797766 $
,
$ R_2(0.5, 0.9)\approx 0.797766 $
,
 $ R_5(0.5, 0)\approx 0.404549 $
,
$ R_5(0.5, 0)\approx 0.404549 $
,
 $ R_5(0.5, 0.3)\approx 0.509745 $
,
$ R_5(0.5, 0.3)\approx 0.509745 $
,
 $ R_5(0.5, 0.6)\approx 0.609243 $
,
$ R_5(0.5, 0.6)\approx 0.609243 $
,
 $ R_5(0.5, 0.9)\approx 0.844344 $
.
$ R_5(0.5, 0.9)\approx 0.844344 $
. -
(ii)
 $ R^{\prime }_2(0.5, 0)\approx 0.44221 $
,
$ R^{\prime }_2(0.5, 0)\approx 0.44221 $
,
 $ R^{\prime }_2(0.5, 0.3)\approx 0.526991 $
,
$ R^{\prime }_2(0.5, 0.3)\approx 0.526991 $
,
 $ R^{\prime }_2(0.5, 0.5)\approx 0.596714 $
,
$ R^{\prime }_2(0.5, 0.5)\approx 0.596714 $
,
 $ R^{\prime }_2(0.5, 0.7)\approx 0.688696 $
,
$ R^{\prime }_2(0.5, 0.7)\approx 0.688696 $
,
 $ R^{\prime }_2(0.5, 0.9)\approx 0.837519 $
,
$ R^{\prime }_2(0.5, 0.9)\approx 0.837519 $
,
 $ R^{\prime }_5(0.5, 0)\approx 0.503019 $
,
$ R^{\prime }_5(0.5, 0)\approx 0.503019 $
,
 $ R^{\prime }_5(0.5, 0.2)\approx 0.555796 $
,
$ R^{\prime }_5(0.5, 0.2)\approx 0.555796 $
,
 $ R^{\prime }_5(0.5, 0.4)\approx 0.616078 $
,
$ R^{\prime }_5(0.5, 0.4)\approx 0.616078 $
,
 $ R^{\prime }_5(0.5, 0.6)\approx 0.68937 $
,
$ R^{\prime }_5(0.5, 0.6)\approx 0.68937 $
,
 $ R^{\prime }_5(0.5, 0.98)\approx 0.959913 $
.
$ R^{\prime }_5(0.5, 0.98)\approx 0.959913 $
.
We obtain the next result which is the harmonic analog of Theorem 1.2 for the class
![]() $ \mathcal {W}^0_{\mathcal {H}}(\alpha , \beta ) $
.
$ \mathcal {W}^0_{\mathcal {H}}(\alpha , \beta ) $
.

Figure 1 The pictorial representation of the radii in Remark 2.1.
Theorem 2.2 Let
![]() $ f=h+\overline {g}\in \mathcal {W}^0_{\mathcal {H}}(\alpha , \beta ) $
for
$ f=h+\overline {g}\in \mathcal {W}^0_{\mathcal {H}}(\alpha , \beta ) $
for
![]() $ \alpha \geq 0 $
and
$ \alpha \geq 0 $
and
![]() $ 0\leq \beta <1 $
, be of the form (1.2) with
$ 0\leq \beta <1 $
, be of the form (1.2) with
![]() $ b_1=0 $
. Then, for
$ b_1=0 $
. Then, for
![]() $ N\geq 2 $
, we have
$ N\geq 2 $
, we have
 $$ \begin{align*} |f(z^m)|+\sum_{n=N}^{\infty}\left(|a_n|+|b_n|\right)|z|^n\leq d(f(0),\partial f(\mathbb{D})) \end{align*} $$
$$ \begin{align*} |f(z^m)|+\sum_{n=N}^{\infty}\left(|a_n|+|b_n|\right)|z|^n\leq d(f(0),\partial f(\mathbb{D})) \end{align*} $$
for
![]() $ |z|=r\leq R_{m,N}(\alpha ,\beta ) $
, where
$ |z|=r\leq R_{m,N}(\alpha ,\beta ) $
, where
![]() $ R_{m,N}(\alpha ,\beta ) $
is the unique root of
$ R_{m,N}(\alpha ,\beta ) $
is the unique root of
 $$ \begin{align*} r^m+\sum_{n=2}^{\infty}\frac{2(1-\beta)r^{mn}}{n\left(1+\alpha(n-1)\right)}\kern-1pt+\sum_{n=N}^{\infty}\frac{2(1-\beta)r^n}{n\left(1+\alpha(n-1)\right)}-1-\sum_{n=2}^{\infty}\frac{2(1-\beta)(-1)^{n-1}}{n\left(1+\alpha(n-1)\right)}=0. \end{align*} $$
$$ \begin{align*} r^m+\sum_{n=2}^{\infty}\frac{2(1-\beta)r^{mn}}{n\left(1+\alpha(n-1)\right)}\kern-1pt+\sum_{n=N}^{\infty}\frac{2(1-\beta)r^n}{n\left(1+\alpha(n-1)\right)}-1-\sum_{n=2}^{\infty}\frac{2(1-\beta)(-1)^{n-1}}{n\left(1+\alpha(n-1)\right)}=0. \end{align*} $$
Here,
![]() $ R_{m,N}(\alpha ,\beta ) $
is best possible.
$ R_{m,N}(\alpha ,\beta ) $
is best possible.
Remark 2.2 For particular values of
![]() $ \alpha $
and
$ \alpha $
and
![]() $ \beta $
, a simple computation gives the Bohr–Rogosinski radii
$ \beta $
, a simple computation gives the Bohr–Rogosinski radii
![]() $ R_{2,2}(0.5, 0)\approx 0.507868 $
,
$ R_{2,2}(0.5, 0)\approx 0.507868 $
,
![]() $ R_{2,2}(0.5, 5)\approx 0.669204$
,
$ R_{2,2}(0.5, 5)\approx 0.669204$
,
![]() $ R_{2,2}(0.5, 0.9)\approx 0.880059 $
,
$ R_{2,2}(0.5, 0.9)\approx 0.880059 $
,
![]() $ R_{5,2}(0.5, 0)\approx 0.643089 $
,
$ R_{5,2}(0.5, 0)\approx 0.643089 $
,
![]() $ R_{5,2}(0.5, 0,5)\approx 0.801547$
,
$ R_{5,2}(0.5, 0,5)\approx 0.801547$
,
![]() $ R_{5,2}(0.5, 0.9)\approx 0.943465$
,
$ R_{5,2}(0.5, 0.9)\approx 0.943465$
,
![]() $ R_{7,5}(0.5, 0)\approx 0. 844912 $
,
$ R_{7,5}(0.5, 0)\approx 0. 844912 $
,
![]() $ R_{7,5}(0.5, 0.4)\approx 0.895644 $
,
$ R_{7,5}(0.5, 0.4)\approx 0.895644 $
,
![]() $ R_{7,5}(0.5, 0.8)\approx 0.952466$
(Figure 2).
$ R_{7,5}(0.5, 0.8)\approx 0.952466$
(Figure 2).

Figure 2 The pictorial representation of the radii in Remark 2.2.
In order to establish a harmonic analog of Theorem 1.3 for the class
![]() $ \mathcal {W}^{0}_{\mathcal {H}}(\alpha ,\beta ) $
, we obtain the following result.
$ \mathcal {W}^{0}_{\mathcal {H}}(\alpha ,\beta ) $
, we obtain the following result.
Theorem 2.3 Let
![]() $ f=h+\overline {g}\in \mathcal {W}^0_{\mathcal {H}}(\alpha , \beta ) $
for
$ f=h+\overline {g}\in \mathcal {W}^0_{\mathcal {H}}(\alpha , \beta ) $
for
![]() $ \alpha \geq 0 $
and
$ \alpha \geq 0 $
and
![]() $ 0\leq \beta <1 $
, be of the form (1.2). Then, for any integer
$ 0\leq \beta <1 $
, be of the form (1.2). Then, for any integer
![]() $ p\geq 1 $
,
$ p\geq 1 $
,
 $$ \begin{align*} |f(z)|^p+\sum_{n=2}^{\infty}\left(|a_n|+|b_n|\right)r^n+\left(\frac{1}{2}+\frac{r}{1-r}\right)\sum_{n=2}^{\infty}\left(|a_n|+|b_n|\right)^2r^{2n}\leq d(f(0),\partial f(\mathbb{D})) \end{align*} $$
$$ \begin{align*} |f(z)|^p+\sum_{n=2}^{\infty}\left(|a_n|+|b_n|\right)r^n+\left(\frac{1}{2}+\frac{r}{1-r}\right)\sum_{n=2}^{\infty}\left(|a_n|+|b_n|\right)^2r^{2n}\leq d(f(0),\partial f(\mathbb{D})) \end{align*} $$
for
![]() $ |z|=r\leq R^{*}_{p}(\alpha ,\beta ) $
, where
$ |z|=r\leq R^{*}_{p}(\alpha ,\beta ) $
, where
![]() $ R^{*}_{p}(\alpha ,\beta ) $
is the unique root of
$ R^{*}_{p}(\alpha ,\beta ) $
is the unique root of
 $$ \begin{align*} &\left(r+\sum_{n=2}^{\infty}\frac{2(1-\beta)r^n}{n\left(1+\alpha(n-1)\right)}\right)^p+\sum_{n=2}^{\infty}\frac{2(1-\beta)r^n}{n\left(1+\alpha(n-1)\right)}+\frac{1+r}{2(1-r)}\sum_{n=2}^{\infty}\frac{4(1-\beta)^2r^{2n}}{\left(n\left(1+\alpha(n-1)\right)\right)^2}\\&=1+\sum_{n=2}^{\infty}\frac{2(1-\beta)(-1)^{n-1}}{n\left(1+\alpha(n-1)\right)} \end{align*} $$
$$ \begin{align*} &\left(r+\sum_{n=2}^{\infty}\frac{2(1-\beta)r^n}{n\left(1+\alpha(n-1)\right)}\right)^p+\sum_{n=2}^{\infty}\frac{2(1-\beta)r^n}{n\left(1+\alpha(n-1)\right)}+\frac{1+r}{2(1-r)}\sum_{n=2}^{\infty}\frac{4(1-\beta)^2r^{2n}}{\left(n\left(1+\alpha(n-1)\right)\right)^2}\\&=1+\sum_{n=2}^{\infty}\frac{2(1-\beta)(-1)^{n-1}}{n\left(1+\alpha(n-1)\right)} \end{align*} $$
in
![]() $ (0, 1) $
. Here,
$ (0, 1) $
. Here,
![]() $ R^{*}_{p}(\alpha ,\beta ) $
is best possible.
$ R^{*}_{p}(\alpha ,\beta ) $
is best possible.
We have the following immediate corollary of Theorem 2.3 which is the harmonic analog of Theorem 1.3 for the class
![]() $ \mathcal {W}^0_{\mathcal {H}}(\alpha , \beta ) $
.
$ \mathcal {W}^0_{\mathcal {H}}(\alpha , \beta ) $
.
Corollary 2.1 Let
![]() $ f=h+\overline {g}\in \mathcal {W}^0_{\mathcal {H}}(\alpha , \beta ) $
for
$ f=h+\overline {g}\in \mathcal {W}^0_{\mathcal {H}}(\alpha , \beta ) $
for
![]() $ \alpha \geq 0 $
and
$ \alpha \geq 0 $
and
![]() $ 0\leq \beta <1 $
, be of the form (1.2). Then:
$ 0\leq \beta <1 $
, be of the form (1.2). Then:
-
(i)
 $ |f(z)|+\displaystyle \sum _{n=2}^{\infty }\left (|a_n|+|b_n|\right )|z|^n+\left (\frac {1}{2}+\frac {r}{1-r}\right )\sum _{n=2}^{\infty }\left (|a_n|+|b_n|\right )^2r^{2n}\leq d(f(0),\partial f(\mathbb {D})) $
for
$ |f(z)|+\displaystyle \sum _{n=2}^{\infty }\left (|a_n|+|b_n|\right )|z|^n+\left (\frac {1}{2}+\frac {r}{1-r}\right )\sum _{n=2}^{\infty }\left (|a_n|+|b_n|\right )^2r^{2n}\leq d(f(0),\partial f(\mathbb {D})) $
for
 $ |z|=r\leq R^{*}_1(\alpha ,\beta ) $
, where
$ |z|=r\leq R^{*}_1(\alpha ,\beta ) $
, where
 $ R^{*}_1(\alpha ,\beta ) $
is the unique root of
$ R^{*}_1(\alpha ,\beta ) $
is the unique root of $$ \begin{align*} r&+\sum_{n=2}^{\infty}\frac{2(1-\beta)r^n}{n\left(1+\alpha(n-1)\right)}+\sum_{n=2}^{\infty}\frac{2(1-\beta)r^n}{n\left(1+\alpha(n-1)\right)}+\frac{1+r}{2(1-r)}\sum_{n=2}^{\infty}\frac{4(1-\beta)^2r^{2n}}{\left(n\left(1+\alpha(n-1)\right)\right)^2}\\&=1+\sum_{n=2}^{\infty}\frac{2(1-\beta)(-1)^{n-1}}{n\left(1+\alpha(n-1)\right)} \end{align*} $$
$$ \begin{align*} r&+\sum_{n=2}^{\infty}\frac{2(1-\beta)r^n}{n\left(1+\alpha(n-1)\right)}+\sum_{n=2}^{\infty}\frac{2(1-\beta)r^n}{n\left(1+\alpha(n-1)\right)}+\frac{1+r}{2(1-r)}\sum_{n=2}^{\infty}\frac{4(1-\beta)^2r^{2n}}{\left(n\left(1+\alpha(n-1)\right)\right)^2}\\&=1+\sum_{n=2}^{\infty}\frac{2(1-\beta)(-1)^{n-1}}{n\left(1+\alpha(n-1)\right)} \end{align*} $$
in
 $ (0, 1) $
. Here,
$ (0, 1) $
. Here,
 $ R^{*}_1(\alpha ,\beta ) $
is best possible.
$ R^{*}_1(\alpha ,\beta ) $
is best possible. -
(ii)
 $ \displaystyle |f(z)|^2+\sum _{n=2}^{\infty }\left (|a_n|+|b_n|\right )r^n+\left (\frac {1}{2}+\frac {r}{1-r}\right )\sum _{n=2}^{\infty }\left (|a_n|+|b_n|\right )^2r^{2n}\leq d(f(0),\partial f(\mathbb {D})) $
for
$ \displaystyle |f(z)|^2+\sum _{n=2}^{\infty }\left (|a_n|+|b_n|\right )r^n+\left (\frac {1}{2}+\frac {r}{1-r}\right )\sum _{n=2}^{\infty }\left (|a_n|+|b_n|\right )^2r^{2n}\leq d(f(0),\partial f(\mathbb {D})) $
for
 $ |z|=r\leq R^{*}_{2}(\alpha ,\beta ) $
, where
$ |z|=r\leq R^{*}_{2}(\alpha ,\beta ) $
, where
 $ R^{*}_{2}(\alpha ,\beta ) $
is the unique root of
$ R^{*}_{2}(\alpha ,\beta ) $
is the unique root of $$ \begin{align*} &\left(r+\sum_{n=2}^{\infty}\frac{2(1-\beta)r^n}{n\left(1+\alpha(n-1)\right)}\right)^2+\sum_{n=2}^{\infty}\frac{2(1-\beta)r^n}{n\left(1+\alpha(n-1)\right)}+\frac{1+r}{2(1-r)}\sum_{n=2}^{\infty}\frac{4(1-\beta)^2r^{2n}}{\left(n\left(1+\alpha(n-1)\right)\right)^2}\\&\quad=1+\sum_{n=2}^{\infty}\frac{2(1-\beta)(-1)^{n-1}}{n\left(1+\alpha(n-1)\right)} \end{align*} $$
$$ \begin{align*} &\left(r+\sum_{n=2}^{\infty}\frac{2(1-\beta)r^n}{n\left(1+\alpha(n-1)\right)}\right)^2+\sum_{n=2}^{\infty}\frac{2(1-\beta)r^n}{n\left(1+\alpha(n-1)\right)}+\frac{1+r}{2(1-r)}\sum_{n=2}^{\infty}\frac{4(1-\beta)^2r^{2n}}{\left(n\left(1+\alpha(n-1)\right)\right)^2}\\&\quad=1+\sum_{n=2}^{\infty}\frac{2(1-\beta)(-1)^{n-1}}{n\left(1+\alpha(n-1)\right)} \end{align*} $$
in
 $ (0, 1) $
. Here,
$ (0, 1) $
. Here,
 $ R^{*}_{2}(\alpha ,\beta ) $
is best possible.
$ R^{*}_{2}(\alpha ,\beta ) $
is best possible.
3 Proof of the main results
Proof of Theorem 2.1
Let
![]() $ f\in \mathcal {W}^{0}_{\mathcal {H}}(\alpha ,\beta ) $
be given by (1.2). Then in view of Lemmas 1.2 and 1.3, it is easy to see that the Euclidean distance
$ f\in \mathcal {W}^{0}_{\mathcal {H}}(\alpha ,\beta ) $
be given by (1.2). Then in view of Lemmas 1.2 and 1.3, it is easy to see that the Euclidean distance
![]() $ d(f(0),\partial f(\mathbb {D})) $
between
$ d(f(0),\partial f(\mathbb {D})) $
between
![]() $ f(0) $
and the boundary of
$ f(0) $
and the boundary of
![]() $ f(\mathbb {D}) $
is
$ f(\mathbb {D}) $
is
 $$ \begin{align} d(f(0),\partial f(\mathbb{D}))=\liminf_{|z|\rightarrow 1}|f(z)-f(0)|\geq 1+\sum_{n=2}^{\infty}\frac{2(1-\beta)(-1)^{n-1}}{n\left(1+\alpha(n-1)\right)}. \end{align} $$
$$ \begin{align} d(f(0),\partial f(\mathbb{D}))=\liminf_{|z|\rightarrow 1}|f(z)-f(0)|\geq 1+\sum_{n=2}^{\infty}\frac{2(1-\beta)(-1)^{n-1}}{n\left(1+\alpha(n-1)\right)}. \end{align} $$
(i) Let
![]() $ F_1 : [0,1]\rightarrow \mathbb {R} $
be defined by
$ F_1 : [0,1]\rightarrow \mathbb {R} $
be defined by
 $$ \begin{align} F_1(r)&:= r+\sum_{n=2}^{\infty}\frac{2(1-\beta)r^n}{n\left(1+\alpha(n-1)\right)}+\sum_{n=N}^{\infty}\frac{2(1-\beta)r^n}{n\left(1+\alpha(n-1)\right)}\\&\nonumber\quad\quad-1-\sum_{n=2}^{\infty}\frac{2(1-\beta)(-1)^{n-1}}{n\left(1+\alpha(n-1)\right)}. \end{align} $$
$$ \begin{align} F_1(r)&:= r+\sum_{n=2}^{\infty}\frac{2(1-\beta)r^n}{n\left(1+\alpha(n-1)\right)}+\sum_{n=N}^{\infty}\frac{2(1-\beta)r^n}{n\left(1+\alpha(n-1)\right)}\\&\nonumber\quad\quad-1-\sum_{n=2}^{\infty}\frac{2(1-\beta)(-1)^{n-1}}{n\left(1+\alpha(n-1)\right)}. \end{align} $$
It is easy to see that
![]() $ F_1(r) $
is continuous on
$ F_1(r) $
is continuous on
![]() $ [0,1] $
and differentiable on
$ [0,1] $
and differentiable on
![]() $ (0,1) $
. Since
$ (0,1) $
. Since
 $$ \begin{align*} \bigg|\sum_{n=2}^{\infty}\frac{(-1)^{n-1}}{n\left(1+\alpha(n-1)\right)}\bigg|\leq \frac{1}{2(1-\beta)}\;\; \mbox{for}\;\; n\geq 2, \end{align*} $$
$$ \begin{align*} \bigg|\sum_{n=2}^{\infty}\frac{(-1)^{n-1}}{n\left(1+\alpha(n-1)\right)}\bigg|\leq \frac{1}{2(1-\beta)}\;\; \mbox{for}\;\; n\geq 2, \end{align*} $$
it follows from (3.2) that
 $$ \begin{align*} F_1(0)=-1-\sum_{n=2}^{\infty}\frac{2(1-\beta)(-1)^{n-1}}{n\left(1+\alpha(n-1)\right)}<0. \end{align*} $$
$$ \begin{align*} F_1(0)=-1-\sum_{n=2}^{\infty}\frac{2(1-\beta)(-1)^{n-1}}{n\left(1+\alpha(n-1)\right)}<0. \end{align*} $$
On the other hand, we see that
 $$ \begin{align*} \sum_{n=2}^{\infty}\frac{1}{\alpha n^2+(1-\alpha)n}\geq \sum_{n=2}^{\infty}\frac{(-1)^{n-1}}{\alpha n^2+(1-\alpha)n}. \end{align*} $$
$$ \begin{align*} \sum_{n=2}^{\infty}\frac{1}{\alpha n^2+(1-\alpha)n}\geq \sum_{n=2}^{\infty}\frac{(-1)^{n-1}}{\alpha n^2+(1-\alpha)n}. \end{align*} $$
Therefore, a simple computation shows that
 $$ \begin{align*} F_1(1)&=1+\sum_{n=2}^{\infty}\frac{2(1-\beta)}{n\left(1+\alpha(n-1)\right)}+\sum_{n=N}^{\infty}\frac{2(1-\beta)}{n\left(1+\alpha(n-1)\right)}\\&\nonumber\quad\quad-1-\sum_{n=2}^{\infty}\frac{2(1-\beta)(-1)^{n-1}}{n\left(1+\alpha(n-1)\right)}\\&\geq \sum_{n=N}^{\infty}\frac{2(1-\beta)}{n\left(1+\alpha(n-1)\right)}>0. \end{align*} $$
$$ \begin{align*} F_1(1)&=1+\sum_{n=2}^{\infty}\frac{2(1-\beta)}{n\left(1+\alpha(n-1)\right)}+\sum_{n=N}^{\infty}\frac{2(1-\beta)}{n\left(1+\alpha(n-1)\right)}\\&\nonumber\quad\quad-1-\sum_{n=2}^{\infty}\frac{2(1-\beta)(-1)^{n-1}}{n\left(1+\alpha(n-1)\right)}\\&\geq \sum_{n=N}^{\infty}\frac{2(1-\beta)}{n\left(1+\alpha(n-1)\right)}>0. \end{align*} $$
Clearly, we have
![]() $ F_1(0)F_1(1)<0 $
, and hence, by the intermediate value theorem,
$ F_1(0)F_1(1)<0 $
, and hence, by the intermediate value theorem,
![]() $ F_1(r) $
has at least one root in
$ F_1(r) $
has at least one root in
![]() $ (0,1) $
. To show that
$ (0,1) $
. To show that
![]() $ F_1(r) $
has the unique root in
$ F_1(r) $
has the unique root in
![]() $ (0,1) $
, it is sufficient to show that
$ (0,1) $
, it is sufficient to show that
![]() $ F_1 $
is strictly monotone in
$ F_1 $
is strictly monotone in
![]() $ (0,1) $
. Now a simple computation shows that
$ (0,1) $
. Now a simple computation shows that
 $$ \begin{align*} \frac{d}{dr}\left(F_1(r)\right)=1+\sum_{n=2}^{\infty}\frac{2(1-\beta)nr^{n-1}}{n\left(1+\alpha(n-1)\right)}+\sum_{n=N}^{\infty}\frac{2(1-\beta)nr^{n-1}}{n\left(1+\alpha(n-1)\right)}>0 \end{align*} $$
$$ \begin{align*} \frac{d}{dr}\left(F_1(r)\right)=1+\sum_{n=2}^{\infty}\frac{2(1-\beta)nr^{n-1}}{n\left(1+\alpha(n-1)\right)}+\sum_{n=N}^{\infty}\frac{2(1-\beta)nr^{n-1}}{n\left(1+\alpha(n-1)\right)}>0 \end{align*} $$
for all
![]() $ r\in (0,1) $
, and hence the function
$ r\in (0,1) $
, and hence the function
![]() $ F_1 $
is strictly increasing. Therefore,
$ F_1 $
is strictly increasing. Therefore,
![]() $ F_1(r) $
has the unique root in
$ F_1(r) $
has the unique root in
![]() $ (0,1) $
, say
$ (0,1) $
, say
![]() $ R_N(\alpha ,\beta ) $
. That is,
$ R_N(\alpha ,\beta ) $
. That is,
![]() $ F_1(R_N(\alpha ,\beta ))=0 $
and hence, from (3.2), we obtain
$ F_1(R_N(\alpha ,\beta ))=0 $
and hence, from (3.2), we obtain
 $$ \begin{align} &R_N(\alpha,\beta)+\sum_{n=2}^{\infty}\frac{2(1-\beta)(R_N(\alpha,\beta))^n}{n\left(1+\alpha(n-1)\right)}+\sum_{n=N}^{\infty}\frac{2(1-\beta)(R_N(\alpha,\beta))^n}{n\left(1+\alpha(n-1)\right)}\\&\nonumber\quad\quad=1+\sum_{n=2}^{\infty}\frac{2(1-\beta)(-1)^{n-1}}{n\left(1+\alpha(n-1)\right)}. \end{align} $$
$$ \begin{align} &R_N(\alpha,\beta)+\sum_{n=2}^{\infty}\frac{2(1-\beta)(R_N(\alpha,\beta))^n}{n\left(1+\alpha(n-1)\right)}+\sum_{n=N}^{\infty}\frac{2(1-\beta)(R_N(\alpha,\beta))^n}{n\left(1+\alpha(n-1)\right)}\\&\nonumber\quad\quad=1+\sum_{n=2}^{\infty}\frac{2(1-\beta)(-1)^{n-1}}{n\left(1+\alpha(n-1)\right)}. \end{align} $$
In order to show that
![]() $ R_N(\alpha ,\beta ) $
is the best possible, we consider the function
$ R_N(\alpha ,\beta ) $
is the best possible, we consider the function
![]() $ f=f_{\alpha ,\beta } $
given by (1.3). It is easy to see that
$ f=f_{\alpha ,\beta } $
given by (1.3). It is easy to see that
![]() $ f_{\alpha ,\beta }\in \mathcal {W}^{0}_{\mathcal {H}}(\alpha , \beta ) $
. For
$ f_{\alpha ,\beta }\in \mathcal {W}^{0}_{\mathcal {H}}(\alpha , \beta ) $
. For
![]() $ f=f_{\alpha , \beta } $
, a straightforward computation shows that
$ f=f_{\alpha , \beta } $
, a straightforward computation shows that
 $$ \begin{align} d(f_{\alpha,\beta}(0), \partial f_{\alpha,\beta}(\mathbb{D}))=1+\sum_{n=2}^{\infty}\frac{2(1-\beta)(-1)^{n-1}}{n\left(1+\alpha(n-1)\right)}. \end{align} $$
$$ \begin{align} d(f_{\alpha,\beta}(0), \partial f_{\alpha,\beta}(\mathbb{D}))=1+\sum_{n=2}^{\infty}\frac{2(1-\beta)(-1)^{n-1}}{n\left(1+\alpha(n-1)\right)}. \end{align} $$
Furthermore, it is easy to see that
 $$ \begin{align*} r+\sum_{n=2}^{\infty}\frac{2(1-\beta)r^n}{n\left(1+\alpha(n-1)\right)}+\sum_{n=N}^{\infty}\frac{2(1-\beta)r^n}{n\left(1+\alpha(n-1)\right)}\leq 1+\sum_{n=2}^{\infty}\frac{2(1-\beta)(-1)^{n-1}}{\alpha n^2+(1-\alpha)n} \end{align*} $$
$$ \begin{align*} r+\sum_{n=2}^{\infty}\frac{2(1-\beta)r^n}{n\left(1+\alpha(n-1)\right)}+\sum_{n=N}^{\infty}\frac{2(1-\beta)r^n}{n\left(1+\alpha(n-1)\right)}\leq 1+\sum_{n=2}^{\infty}\frac{2(1-\beta)(-1)^{n-1}}{\alpha n^2+(1-\alpha)n} \end{align*} $$
for
![]() $ r\leq R_N(\alpha ,\beta ) $
. A simple computation using (3.3) and (3.4) for the function
$ r\leq R_N(\alpha ,\beta ) $
. A simple computation using (3.3) and (3.4) for the function
![]() $ f=f_{\alpha , \beta } $
and
$ f=f_{\alpha , \beta } $
and
![]() $ r> R_N(\alpha ,\beta ) $
shows that
$ r> R_N(\alpha ,\beta ) $
shows that
 $$ \begin{align*} &|f_{\alpha,\beta}(z)|+\sum_{n=N}^{\infty}\left(|a_n|+|b_n|\right)|z|^n\\&=r+\sum_{n=2}^{\infty}\frac{2(1-\beta)r^n}{n\left(1+\alpha(n-1)\right)}+\sum_{n=N}^{\infty}\frac{2(1-\beta)r^n}{n\left(1+\alpha(n-1)\right)}\\&>R_N(\alpha,\beta)+\sum_{n=2}^{\infty}\frac{2(1-\beta)(R_N(\alpha,\beta))^n}{n\left(1+\alpha(n-1)\right)}+\sum_{n=N}^{\infty}\frac{2(1-\beta)(R_N(\alpha,\beta))^n}{n\left(1+\alpha(n-1)\right)}\\&=1+\sum_{n=2}^{\infty}\frac{2(1-\beta)(-1)^{n-1}}{n\left(1+\alpha(n-1)\right)}\\&=d(f_{\alpha,\beta}(0),\partial f_{\alpha,\beta}(\mathbb{D})). \end{align*} $$
$$ \begin{align*} &|f_{\alpha,\beta}(z)|+\sum_{n=N}^{\infty}\left(|a_n|+|b_n|\right)|z|^n\\&=r+\sum_{n=2}^{\infty}\frac{2(1-\beta)r^n}{n\left(1+\alpha(n-1)\right)}+\sum_{n=N}^{\infty}\frac{2(1-\beta)r^n}{n\left(1+\alpha(n-1)\right)}\\&>R_N(\alpha,\beta)+\sum_{n=2}^{\infty}\frac{2(1-\beta)(R_N(\alpha,\beta))^n}{n\left(1+\alpha(n-1)\right)}+\sum_{n=N}^{\infty}\frac{2(1-\beta)(R_N(\alpha,\beta))^n}{n\left(1+\alpha(n-1)\right)}\\&=1+\sum_{n=2}^{\infty}\frac{2(1-\beta)(-1)^{n-1}}{n\left(1+\alpha(n-1)\right)}\\&=d(f_{\alpha,\beta}(0),\partial f_{\alpha,\beta}(\mathbb{D})). \end{align*} $$
Therefore, the constant
![]() $R_N(\alpha ,\beta ) $
is best possible. This completes the proof of (i).
$R_N(\alpha ,\beta ) $
is best possible. This completes the proof of (i).
(ii). In view of Lemmas 1.2 and 1.3, for
![]() $ |z|=r $
, we easily obtain
$ |z|=r $
, we easily obtain
 $$ \begin{align} & |f(z)|^2+\sum_{n=N}^{\infty}\left(|a_n|+|b_n|\right)|z|^n\\&\nonumber\leq \left(|z|+\sum_{n=2}^{\infty}\frac{2(1-\beta)|z|^n}{n\left(1+\alpha(n-1)\right)}\right)^2+\sum_{n=N}^{\infty}\frac{2(1-\beta)|z|^n}{n\left(1+\alpha(n-1)\right)}\\&\nonumber=\left(r+\sum_{n=2}^{\infty}\frac{2(1-\beta)r^n}{n\left(1+\alpha(n-1)\right)}\right)^2+\sum_{n=N}^{\infty}\frac{2(1-\beta)r^n}{n\left(1+\alpha(n-1)\right)}. \end{align} $$
$$ \begin{align} & |f(z)|^2+\sum_{n=N}^{\infty}\left(|a_n|+|b_n|\right)|z|^n\\&\nonumber\leq \left(|z|+\sum_{n=2}^{\infty}\frac{2(1-\beta)|z|^n}{n\left(1+\alpha(n-1)\right)}\right)^2+\sum_{n=N}^{\infty}\frac{2(1-\beta)|z|^n}{n\left(1+\alpha(n-1)\right)}\\&\nonumber=\left(r+\sum_{n=2}^{\infty}\frac{2(1-\beta)r^n}{n\left(1+\alpha(n-1)\right)}\right)^2+\sum_{n=N}^{\infty}\frac{2(1-\beta)r^n}{n\left(1+\alpha(n-1)\right)}. \end{align} $$
It is easy to see that
 $$ \begin{align*} \left(r+\sum_{n=2}^{\infty}\frac{2(1-\beta)r^n}{n\left(1+\alpha(n-1)\right)}\right)^2+\sum_{n=N}^{\infty}\frac{2(1-\beta)r^n}{n\left(1+\alpha(n-1)\right)}\leq 1+\sum_{n=2}^{\infty}\frac{2(1-\beta)(-1)^{n-1}}{n\left(1+\alpha(n-1)\right)} \end{align*} $$
$$ \begin{align*} \left(r+\sum_{n=2}^{\infty}\frac{2(1-\beta)r^n}{n\left(1+\alpha(n-1)\right)}\right)^2+\sum_{n=N}^{\infty}\frac{2(1-\beta)r^n}{n\left(1+\alpha(n-1)\right)}\leq 1+\sum_{n=2}^{\infty}\frac{2(1-\beta)(-1)^{n-1}}{n\left(1+\alpha(n-1)\right)} \end{align*} $$
for
![]() $ r\leq R^{\prime }_N(f) $
, where
$ r\leq R^{\prime }_N(f) $
, where
![]() $ R^{\prime }_N(f) $
is a root of
$ R^{\prime }_N(f) $
is a root of
![]() $ F_2(r)=0 $
, where
$ F_2(r)=0 $
, where
![]() $ F_2 : [0,1]\rightarrow \mathbb {R} $
is defined by
$ F_2 : [0,1]\rightarrow \mathbb {R} $
is defined by
 $$ \begin{align*} F_2(r):&=\left(r+\sum_{n=2}^{\infty}\frac{2(1-\beta)r^n}{n\left(1+\alpha(n-1)\right)}\right)^2+\sum_{n=N}^{\infty}\frac{2(1-\beta)r^n}{n\left(1+\alpha(n-1)\right)}\\&\nonumber\quad\quad- 1-\sum_{n=2}^{\infty}\frac{2(1-\beta)(-1)^{n-1}}{n\left(1+\alpha(n-1)\right)}. \end{align*} $$
$$ \begin{align*} F_2(r):&=\left(r+\sum_{n=2}^{\infty}\frac{2(1-\beta)r^n}{n\left(1+\alpha(n-1)\right)}\right)^2+\sum_{n=N}^{\infty}\frac{2(1-\beta)r^n}{n\left(1+\alpha(n-1)\right)}\\&\nonumber\quad\quad- 1-\sum_{n=2}^{\infty}\frac{2(1-\beta)(-1)^{n-1}}{n\left(1+\alpha(n-1)\right)}. \end{align*} $$
By the similar argument being used in the proof of (i), it is easy to see that
![]() $ {F_2(0)<0 }$
and
$ {F_2(0)<0 }$
and
![]() $ F_2(1)>0 $
. Since
$ F_2(1)>0 $
. Since
![]() $ F_2 $
is continuous on
$ F_2 $
is continuous on
![]() $ [0,1] $
and differentiable on
$ [0,1] $
and differentiable on
![]() $ (0,1) $
, by the intermediate value theorem there exists a root, say
$ (0,1) $
, by the intermediate value theorem there exists a root, say
![]() $ R^{\prime }_N(\alpha ,\beta ) $
of
$ R^{\prime }_N(\alpha ,\beta ) $
of
![]() $ F_2 $
in
$ F_2 $
in
![]() $ (0,1) $
. In fact, we show that
$ (0,1) $
. In fact, we show that
![]() $ R^{\prime }_N(\alpha ,\beta ) $
is the unique root of
$ R^{\prime }_N(\alpha ,\beta ) $
is the unique root of
![]() $ F_2 $
in
$ F_2 $
in
![]() $ (0,1) $
. By a simple computation, we obtain
$ (0,1) $
. By a simple computation, we obtain
 $$ \begin{align*} \frac{d}{dr}\left(F_2(r)\right)&=2\left(r+\sum_{n=2}^{\infty}\frac{2(1-\beta)r^{n}}{n\left(1+\alpha(n-1)\right)}\right)\left(1+\sum_{n=2}^{\infty}\frac{2(1-\beta)nr^{n-1}}{n\left(1+\alpha(n-1)\right)}\right)\\&\quad\quad+\sum_{n=N}^{\infty}\frac{2(1-\beta)nr^{n-1}}{n\left(1+\alpha(n-1)\right)}>0 \end{align*} $$
$$ \begin{align*} \frac{d}{dr}\left(F_2(r)\right)&=2\left(r+\sum_{n=2}^{\infty}\frac{2(1-\beta)r^{n}}{n\left(1+\alpha(n-1)\right)}\right)\left(1+\sum_{n=2}^{\infty}\frac{2(1-\beta)nr^{n-1}}{n\left(1+\alpha(n-1)\right)}\right)\\&\quad\quad+\sum_{n=N}^{\infty}\frac{2(1-\beta)nr^{n-1}}{n\left(1+\alpha(n-1)\right)}>0 \end{align*} $$
for
![]() $ r\in (0,1) $
and this shows that
$ r\in (0,1) $
and this shows that
![]() $ F_2(r) $
is strictly increasing in
$ F_2(r) $
is strictly increasing in
![]() $ (0,1) $
. Therefore,
$ (0,1) $
. Therefore,
![]() $ R^{\prime }_N(\alpha ,\beta ) $
is the unique root of
$ R^{\prime }_N(\alpha ,\beta ) $
is the unique root of
![]() $ F_2 $
in
$ F_2 $
in
![]() $ (0,1) $
. Thus, we have
$ (0,1) $
. Thus, we have
 $$ \begin{align} &\left(R^{\prime}_N(\alpha,\beta)+\sum_{n=2}^{\infty}\frac{2(1-\beta)\left(R^{\prime}_N(\alpha,\beta)\right)^n}{n\left(1+\alpha(n-1)\right)}\right)^2+\sum_{n=N}^{\infty}\frac{2(1-\beta)\left(R^{\prime}_N(\alpha,\beta)\right)^n}{n\left(1+\alpha(n-1)\right)}\\&\nonumber= 1+\sum_{n=2}^{\infty}\frac{2(1-\beta)(-1)^{n-1}}{n\left(1+\alpha(n-1)\right)}. \end{align} $$
$$ \begin{align} &\left(R^{\prime}_N(\alpha,\beta)+\sum_{n=2}^{\infty}\frac{2(1-\beta)\left(R^{\prime}_N(\alpha,\beta)\right)^n}{n\left(1+\alpha(n-1)\right)}\right)^2+\sum_{n=N}^{\infty}\frac{2(1-\beta)\left(R^{\prime}_N(\alpha,\beta)\right)^n}{n\left(1+\alpha(n-1)\right)}\\&\nonumber= 1+\sum_{n=2}^{\infty}\frac{2(1-\beta)(-1)^{n-1}}{n\left(1+\alpha(n-1)\right)}. \end{align} $$
To show that
![]() $ R^{\prime }_N(\alpha ,\beta ) $
is the best possible, we consider the function
$ R^{\prime }_N(\alpha ,\beta ) $
is the best possible, we consider the function
![]() $ f=f_{\alpha ,\beta } $
defined by (1.3). Using (3.4)–(3.6), for
$ f=f_{\alpha ,\beta } $
defined by (1.3). Using (3.4)–(3.6), for
![]() $ f=f_{\alpha , \beta } $
and
$ f=f_{\alpha , \beta } $
and
![]() $ r> R^{\prime }_N(\alpha ,\beta )$
, it is easy to see that
$ r> R^{\prime }_N(\alpha ,\beta )$
, it is easy to see that
 $$ \begin{align*} &|f_{\alpha, \beta}(z)|^2+\sum_{n=N}^{\infty}\left(|a_n|+|b_n|\right)|z|^n\\&=\left(r+\sum_{n=2}^{\infty}\frac{2(1-\beta)r^n}{n\left(1+\alpha(n-1)\right)}\right)^2+\sum_{n=N}^{\infty}\frac{2(1-\beta)r^n}{n\left(1+\alpha(n-1)\right)}\\&>\left(R^{\prime}_N(\alpha,\beta)+\sum_{n=2}^{\infty}\frac{2(1-\beta)\left(R^{\prime}_N(\alpha,\beta)\right)^n}{n\left(1+\alpha(n-1)\right)}\right)^2+\sum_{n=N}^{\infty}\frac{2(1-\beta)\left(R^{\prime}_N(\alpha,\beta)\right)^n}{n\left(1+\alpha(n-1)\right)}\\&= 1+\sum_{n=2}^{\infty}\frac{2(1-\beta)(-1)^{n-1}}{n\left(1+\alpha(n-1)\right)}\\&=d(f_{\alpha,\beta}(0),\partial f_{\alpha,\beta}(\mathbb{D})), \end{align*} $$
$$ \begin{align*} &|f_{\alpha, \beta}(z)|^2+\sum_{n=N}^{\infty}\left(|a_n|+|b_n|\right)|z|^n\\&=\left(r+\sum_{n=2}^{\infty}\frac{2(1-\beta)r^n}{n\left(1+\alpha(n-1)\right)}\right)^2+\sum_{n=N}^{\infty}\frac{2(1-\beta)r^n}{n\left(1+\alpha(n-1)\right)}\\&>\left(R^{\prime}_N(\alpha,\beta)+\sum_{n=2}^{\infty}\frac{2(1-\beta)\left(R^{\prime}_N(\alpha,\beta)\right)^n}{n\left(1+\alpha(n-1)\right)}\right)^2+\sum_{n=N}^{\infty}\frac{2(1-\beta)\left(R^{\prime}_N(\alpha,\beta)\right)^n}{n\left(1+\alpha(n-1)\right)}\\&= 1+\sum_{n=2}^{\infty}\frac{2(1-\beta)(-1)^{n-1}}{n\left(1+\alpha(n-1)\right)}\\&=d(f_{\alpha,\beta}(0),\partial f_{\alpha,\beta}(\mathbb{D})), \end{align*} $$
which shows that
![]() $ R^{\prime }_N(\alpha ,\beta ) $
is best possible. This completes the proof of (ii).
$ R^{\prime }_N(\alpha ,\beta ) $
is best possible. This completes the proof of (ii).
Proof of Theorem 2.2
In view of Lemmas 1.2 and 1.3, for
![]() $ |z|=r $
, we obtain
$ |z|=r $
, we obtain
 $$ \begin{align} &|f(z^m)|+\sum_{n=N}^{\infty}\left(|a_n|+|b_n|\right)|z|^n\\&\leq \nonumber r^m+\sum_{n=2}^{\infty}\frac{2(1-\beta)r^{mn}}{n\left(1+\alpha(n-1)\right)}+\sum_{n=N}^{\infty}\frac{2(1-\beta)r^n}{n\left(1+\alpha(n-1)\right)}. \end{align} $$
$$ \begin{align} &|f(z^m)|+\sum_{n=N}^{\infty}\left(|a_n|+|b_n|\right)|z|^n\\&\leq \nonumber r^m+\sum_{n=2}^{\infty}\frac{2(1-\beta)r^{mn}}{n\left(1+\alpha(n-1)\right)}+\sum_{n=N}^{\infty}\frac{2(1-\beta)r^n}{n\left(1+\alpha(n-1)\right)}. \end{align} $$
It is easy to see that
 $$ \begin{align*} & r^m+\sum_{n=2}^{\infty}\frac{2(1-\beta)r^{mn}}{n\left(1+\alpha(n-1)\right)}+\sum_{n=N}^{\infty}\frac{2(1-\beta)r^n}{n\left(1+\alpha(n-1)\right)}\\&\leq 1+\sum_{n=2}^{\infty}\frac{2(1-\beta)(-1)^{n-1}}{n\left(1+\alpha(n-1)\right)} \end{align*} $$
$$ \begin{align*} & r^m+\sum_{n=2}^{\infty}\frac{2(1-\beta)r^{mn}}{n\left(1+\alpha(n-1)\right)}+\sum_{n=N}^{\infty}\frac{2(1-\beta)r^n}{n\left(1+\alpha(n-1)\right)}\\&\leq 1+\sum_{n=2}^{\infty}\frac{2(1-\beta)(-1)^{n-1}}{n\left(1+\alpha(n-1)\right)} \end{align*} $$
for
![]() $ r\leq R_{m,N}(\alpha ,\beta ) $
, where
$ r\leq R_{m,N}(\alpha ,\beta ) $
, where
![]() $ R_{m,N}(\alpha ,\beta ) $
is a root of
$ R_{m,N}(\alpha ,\beta ) $
is a root of
![]() $ F_3(r)=0 $
, where
$ F_3(r)=0 $
, where
![]() $ F_3 : [0,1]\rightarrow \mathbb {R} $
is defined by
$ F_3 : [0,1]\rightarrow \mathbb {R} $
is defined by
 $$ \begin{align} F_3(r)&:= r^m+\sum_{n=2}^{\infty}\frac{2(1-\beta)r^{mn}}{n\left(1+\alpha(n-1)\right)}+\sum_{n=N}^{\infty}\frac{2(1-\beta)r^n}{n\left(1+\alpha(n-1)\right)}\\&\nonumber\quad\quad-1-\sum_{n=2}^{\infty}\frac{2(1-\beta)(-1)^{n-1}}{n\left(1+\alpha(n-1)\right)}. \end{align} $$
$$ \begin{align} F_3(r)&:= r^m+\sum_{n=2}^{\infty}\frac{2(1-\beta)r^{mn}}{n\left(1+\alpha(n-1)\right)}+\sum_{n=N}^{\infty}\frac{2(1-\beta)r^n}{n\left(1+\alpha(n-1)\right)}\\&\nonumber\quad\quad-1-\sum_{n=2}^{\infty}\frac{2(1-\beta)(-1)^{n-1}}{n\left(1+\alpha(n-1)\right)}. \end{align} $$
Using the similar argument being used in the proof of Theorem 2.1, it can be shows that
![]() $ R_{m,N}(\alpha ,\beta ) $
is the unique root of
$ R_{m,N}(\alpha ,\beta ) $
is the unique root of
![]() $ F_3 $
in
$ F_3 $
in
![]() $ (0,1) $
. Hence, we have
$ (0,1) $
. Hence, we have
 $$ \begin{align} &\quad(R_{m,N}(\alpha,\beta))^m+\sum_{n=2}^{\infty}\frac{2(1-\beta)(R_{m,N}(\alpha,\beta))^{mn}}{n\left(1+\alpha(n-1)\right)}+\sum_{n=N}^{\infty}\frac{2(1-\beta)(R_{m,N}(\alpha,\beta))^n}{n\left(1+\alpha(n-1)\right)}\\&\nonumber\quad=1+\sum_{n=2}^{\infty}\frac{2(1-\beta)(-1)^{n-1}}{n\left(1+\alpha(n-1)\right)}. \end{align} $$
$$ \begin{align} &\quad(R_{m,N}(\alpha,\beta))^m+\sum_{n=2}^{\infty}\frac{2(1-\beta)(R_{m,N}(\alpha,\beta))^{mn}}{n\left(1+\alpha(n-1)\right)}+\sum_{n=N}^{\infty}\frac{2(1-\beta)(R_{m,N}(\alpha,\beta))^n}{n\left(1+\alpha(n-1)\right)}\\&\nonumber\quad=1+\sum_{n=2}^{\infty}\frac{2(1-\beta)(-1)^{n-1}}{n\left(1+\alpha(n-1)\right)}. \end{align} $$
Let
![]() $ f=f_{\alpha ,\beta } $
be defined by (1.3). In view of (3.4), (3.8), and (3.9), for
$ f=f_{\alpha ,\beta } $
be defined by (1.3). In view of (3.4), (3.8), and (3.9), for
![]() $ r>R_{m,N}(\alpha ,\beta )$
, a simple computation shows that
$ r>R_{m,N}(\alpha ,\beta )$
, a simple computation shows that
 $$ \begin{align*} &|f_{\alpha, \beta}(z^m)|+\sum_{n=N}^{\infty}\left(|a_n|+|b_n|\right)|z|^n\\&=r^m+\sum_{n=2}^{\infty}\frac{2(1-\beta)r^{mn}}{n\left(1+\alpha(n-1)\right)}+\sum_{n=N}^{\infty}\frac{2(1-\beta)r^n}{n\left(1+\alpha(n-1)\right)}\\&> (R_{m,N}(\alpha,\beta))^m+\sum_{n=2}^{\infty}\frac{2(1-\beta)(R_{m,N}(\alpha,\beta))^{mn}}{n\left(1+\alpha(n-1)\right)}+\sum_{n=N}^{\infty}\frac{2(1-\beta)(R_{m,N}(\alpha,\beta))^n}{n\left(1+\alpha(n-1)\right)}\\&=1+\sum_{n=2}^{\infty}\frac{2(1-\beta)(-1)^{n-1}}{n\left(1+\alpha(n-1)\right)}\\&=d(f_{\alpha,\beta}(0),\partial f_{\alpha,\beta}(\mathbb{D})), \end{align*} $$
$$ \begin{align*} &|f_{\alpha, \beta}(z^m)|+\sum_{n=N}^{\infty}\left(|a_n|+|b_n|\right)|z|^n\\&=r^m+\sum_{n=2}^{\infty}\frac{2(1-\beta)r^{mn}}{n\left(1+\alpha(n-1)\right)}+\sum_{n=N}^{\infty}\frac{2(1-\beta)r^n}{n\left(1+\alpha(n-1)\right)}\\&> (R_{m,N}(\alpha,\beta))^m+\sum_{n=2}^{\infty}\frac{2(1-\beta)(R_{m,N}(\alpha,\beta))^{mn}}{n\left(1+\alpha(n-1)\right)}+\sum_{n=N}^{\infty}\frac{2(1-\beta)(R_{m,N}(\alpha,\beta))^n}{n\left(1+\alpha(n-1)\right)}\\&=1+\sum_{n=2}^{\infty}\frac{2(1-\beta)(-1)^{n-1}}{n\left(1+\alpha(n-1)\right)}\\&=d(f_{\alpha,\beta}(0),\partial f_{\alpha,\beta}(\mathbb{D})), \end{align*} $$
and hence the radius
![]() $ R_{m,N}(\alpha ,\beta ) $
is best possible. This completes the proof.
$ R_{m,N}(\alpha ,\beta ) $
is best possible. This completes the proof.
Proof of Theorem 2.3
Let
![]() $ f\in \mathcal {W}^0_{\mathcal {H}}(\alpha , \beta ) $
and
$ f\in \mathcal {W}^0_{\mathcal {H}}(\alpha , \beta ) $
and
![]() $ p\geq 1 $
be an integer. Then, in view of the Lemmas 1.2 and 1.3, we see that
$ p\geq 1 $
be an integer. Then, in view of the Lemmas 1.2 and 1.3, we see that
 $$ \begin{align*} |f(z)|^p&+\displaystyle\sum_{n=2}^{\infty}\left(|a_n|+|b_n|\right)|z|^n+\left(\frac{1}{2}+\frac{r}{1-r}\right)\sum_{n=2}^{\infty}\left(|a_n|+|b_n|\right)^2r^{2n}\\&\leq \left(r+\sum_{n=2}^{\infty}\frac{2(1-\beta)r^n}{n\left(1+\alpha(n-1)\right)}\right)^p+\frac{1+r}{2(1-r)}\sum_{n=2}^{\infty}\frac{4(1-\beta)^2r^{2n}}{\left(n\left(1+\alpha(n-1)\right)\right)^2}\\&\quad +\sum_{n=2}^{\infty}\frac{2(1-\beta)r^n}{n\left(1+\alpha(n-1)\right)}\\&\leq 1+\sum_{n=2}^{\infty}\frac{2(1-\beta)(-1)^{n-1}}{n\left(1+\alpha(n-1)\right)} \end{align*} $$
$$ \begin{align*} |f(z)|^p&+\displaystyle\sum_{n=2}^{\infty}\left(|a_n|+|b_n|\right)|z|^n+\left(\frac{1}{2}+\frac{r}{1-r}\right)\sum_{n=2}^{\infty}\left(|a_n|+|b_n|\right)^2r^{2n}\\&\leq \left(r+\sum_{n=2}^{\infty}\frac{2(1-\beta)r^n}{n\left(1+\alpha(n-1)\right)}\right)^p+\frac{1+r}{2(1-r)}\sum_{n=2}^{\infty}\frac{4(1-\beta)^2r^{2n}}{\left(n\left(1+\alpha(n-1)\right)\right)^2}\\&\quad +\sum_{n=2}^{\infty}\frac{2(1-\beta)r^n}{n\left(1+\alpha(n-1)\right)}\\&\leq 1+\sum_{n=2}^{\infty}\frac{2(1-\beta)(-1)^{n-1}}{n\left(1+\alpha(n-1)\right)} \end{align*} $$
for
![]() $ |z|=r\leq R^{*}_p(\alpha , \beta ) $
, where
$ |z|=r\leq R^{*}_p(\alpha , \beta ) $
, where
![]() $ R^{*}_p(\alpha , \beta ) $
is a root in
$ R^{*}_p(\alpha , \beta ) $
is a root in
![]() $ (0, 1) $
of the equation
$ (0, 1) $
of the equation
![]() $ F_4(r)=0 $
, where
$ F_4(r)=0 $
, where
 $$ \begin{align*} F_4(r):=&\left(r+\sum_{n=2}^{\infty}\frac{2(1-\beta)r^n}{n\left(1+\alpha(n-1)\right)}\right)^p+\frac{1+r}{2(1-r)}\sum_{n=2}^{\infty}\frac{4(1-\beta)^2r^{2n}}{\left(n\left(1+\alpha(n-1)\right)\right)^2}\\&\quad\nonumber +\sum_{n=2}^{\infty}\frac{2(1-\beta)r^n}{n\left(1+\alpha(n-1)\right)}- 1-\sum_{n=2}^{\infty}\frac{2(1-\beta)(-1)^{n-1}}{n\left(1+\alpha(n-1)\right)}. \end{align*} $$
$$ \begin{align*} F_4(r):=&\left(r+\sum_{n=2}^{\infty}\frac{2(1-\beta)r^n}{n\left(1+\alpha(n-1)\right)}\right)^p+\frac{1+r}{2(1-r)}\sum_{n=2}^{\infty}\frac{4(1-\beta)^2r^{2n}}{\left(n\left(1+\alpha(n-1)\right)\right)^2}\\&\quad\nonumber +\sum_{n=2}^{\infty}\frac{2(1-\beta)r^n}{n\left(1+\alpha(n-1)\right)}- 1-\sum_{n=2}^{\infty}\frac{2(1-\beta)(-1)^{n-1}}{n\left(1+\alpha(n-1)\right)}. \end{align*} $$
By the similar argument being used in the proof of the previous theorems, it is easy to show that
![]() $ F_4(0)F_4(1)<0 $
and
$ F_4(0)F_4(1)<0 $
and
![]() $ \frac {d}{dr}(F_4(r))>0 $
in
$ \frac {d}{dr}(F_4(r))>0 $
in
![]() $ (0, 1) $
. Hence,
$ (0, 1) $
. Hence,
![]() $ F_4 $
being continuous and monotone increasing function,
$ F_4 $
being continuous and monotone increasing function,
![]() $ R^{*}_p(\alpha , \beta ) $
is the unique root of the equation
$ R^{*}_p(\alpha , \beta ) $
is the unique root of the equation
![]() $ F_4(r)=0 $
in
$ F_4(r)=0 $
in
![]() $ (0, 1) $
.
$ (0, 1) $
.
Thus, we have
 $$ \begin{align} &\left(R^{*}_p(\alpha, \beta)+\sum_{n=2}^{\infty}\frac{2(1-\beta)\left(R^{*}_p(\alpha, \beta)\right)^n}{n\left(1+\alpha(n-1)\right)}\right)^p+\frac{1+R^{*}_p(\alpha, \beta)}{2(1-rR^{*}_p(\alpha, \beta))}\sum_{n=2}^{\infty}\frac{4(1-\beta)^2\left(R^{*}_p(\alpha, \beta)\right)^{2n}}{\left(n\left(1+\alpha(n-1)\right)\right)^2}\\&\quad\nonumber +\sum_{n=2}^{\infty}\frac{2(1-\beta)\left(R^{*}_p(\alpha, \beta)\right)^n}{n\left(1+\alpha(n-1)\right)}= 1+\sum_{n=2}^{\infty}\frac{2(1-\beta)(-1)^{n-1}}{n\left(1+\alpha(n-1)\right)}.\nonumber \end{align} $$
$$ \begin{align} &\left(R^{*}_p(\alpha, \beta)+\sum_{n=2}^{\infty}\frac{2(1-\beta)\left(R^{*}_p(\alpha, \beta)\right)^n}{n\left(1+\alpha(n-1)\right)}\right)^p+\frac{1+R^{*}_p(\alpha, \beta)}{2(1-rR^{*}_p(\alpha, \beta))}\sum_{n=2}^{\infty}\frac{4(1-\beta)^2\left(R^{*}_p(\alpha, \beta)\right)^{2n}}{\left(n\left(1+\alpha(n-1)\right)\right)^2}\\&\quad\nonumber +\sum_{n=2}^{\infty}\frac{2(1-\beta)\left(R^{*}_p(\alpha, \beta)\right)^n}{n\left(1+\alpha(n-1)\right)}= 1+\sum_{n=2}^{\infty}\frac{2(1-\beta)(-1)^{n-1}}{n\left(1+\alpha(n-1)\right)}.\nonumber \end{align} $$
Therefore, in view of (3.1), we have
 $$ \begin{align*}|f(z)|^p+\sum_{n=2}^{\infty}\left(|a_n|+|b_n|\right)r^n+\left(\frac{1}{2}+\frac{r}{1-r}\right)\sum_{n=2}^{\infty}\left(|a_n|+|b_n|\right)^2r^{2n}\leq d(f(0),\partial f(\mathbb{D})) \end{align*} $$
$$ \begin{align*}|f(z)|^p+\sum_{n=2}^{\infty}\left(|a_n|+|b_n|\right)r^n+\left(\frac{1}{2}+\frac{r}{1-r}\right)\sum_{n=2}^{\infty}\left(|a_n|+|b_n|\right)^2r^{2n}\leq d(f(0),\partial f(\mathbb{D})) \end{align*} $$
for
![]() $ |z|=r\leq R^{*}_{p}(\alpha ,\beta ) $
.
$ |z|=r\leq R^{*}_{p}(\alpha ,\beta ) $
.
Considering the function
![]() $ f=f_{\alpha ,\beta } $
defined by (1.3). Using (3.4) and (3.10), for
$ f=f_{\alpha ,\beta } $
defined by (1.3). Using (3.4) and (3.10), for
![]() $ {f=f_{\alpha , \beta } }$
and
$ {f=f_{\alpha , \beta } }$
and
![]() $ r> R^{*}_p(\alpha ,\beta )$
, it can be shown that
$ r> R^{*}_p(\alpha ,\beta )$
, it can be shown that
 $$ \begin{align*} &|f_{\alpha, \beta}(z)|^p+\displaystyle\sum_{n=2}^{\infty}\left(|a_n|+|b_n|\right)|z|^n+\left(\frac{1}{2}+\frac{r}{1-r}\right)\sum_{n=2}^{\infty}\left(|a_n|+|b_n|\right)^2r^{2n}\\&>d(f_{\alpha,\beta}(0),\partial f_{\alpha,\beta}(\mathbb{D})) \end{align*} $$
$$ \begin{align*} &|f_{\alpha, \beta}(z)|^p+\displaystyle\sum_{n=2}^{\infty}\left(|a_n|+|b_n|\right)|z|^n+\left(\frac{1}{2}+\frac{r}{1-r}\right)\sum_{n=2}^{\infty}\left(|a_n|+|b_n|\right)^2r^{2n}\\&>d(f_{\alpha,\beta}(0),\partial f_{\alpha,\beta}(\mathbb{D})) \end{align*} $$
which shows that
![]() $ R^{*}_p(\alpha ,\beta ) $
is best possible. This completes the proof.
$ R^{*}_p(\alpha ,\beta ) $
is best possible. This completes the proof.
Acknowledgment
The authors would like to thank the referee for the helpful suggestions and comments to improve the paper. The second author is supported by SERB-CRG.
Conflict of interest
The authors declare that there is no conflict of interest regarding the publication of this paper.
Data availability statement
Data sharing not applicable to this article as no datasets were generated or analyzed during the current study.