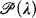Using square sequences, a stationary subset ST of אω1+1 is constructed from a tree T of height ω1, uniformly in T. Under suitable hypotheses, adding a closed unbounded subset to ST requires adding a cofinal branch to T or collapsing at least one of ω1, אω1, and אω1+1. An application is that in ZFC there is no parameter free definition of the family of subsets of אω1+1 that have a closed unbounded subset in some ω1, אω1, and אω1+1 preserving outer model.