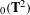In this paper, we show that each element in the convex hull of the rotation set of a compact invariant chain transitive set is realized by a Birkhoff solution, which is an improvement of the fundamental lemma of T. Zhou and W.-X. Qin [Pseudo solutions, rotation sets, and shadowing rotations for monotone recurrence relations. Math. Z. 297 (2021), 1673–1692] in the study of rotation sets for monotone recurrence relations. We then investigate the properties of rotation sets assuming the system has zero topological entropy. The rotation set for a Birkhoff recurrence class is a singleton and the forward and backward rotation numbers are identical for each solution in the same Birkhoff recurrence class. We also show the continuity of rotation numbers on the set of non-wandering points. If the rotation set is upper-stable, then we show that each boundary point is a rational number, and we also obtain a result of bounded deviation.

A classical article by Misiurewicz and Ziemian (J. Lond. Math. Soc.40(2) (1989), 490–506) classifies the elements in Homeo$_{0}(\mathbf{T}^{2})$ by their rotation set $\unicode[STIX]{x1D70C}$, according to wether $\unicode[STIX]{x1D70C}$ is a point, a segment or a set with nonempty interior. A recent classification of nonwandering elements in Homeo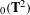$_{0}(\mathbf{T}^{2})$ by Koropecki and Tal was given in (Invent. Math.196 (2014), 339–381), according to the intrinsic underlying ambient space where the dynamics takes place: planar, annular and strictly toral maps. We study the link between these two classifications, showing that, even abroad the nonwandering setting, annular maps are characterized by rotation sets which are rational segments. Also, we obtain information on the sublinear diffusion of orbits in the—not very well understood—case that $\unicode[STIX]{x1D70C}$ has nonempty interior.