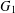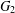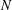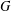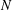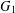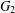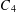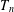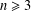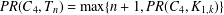1 results
QUADRILATERAL-TREE PLANAR RAMSEY NUMBERS
- Part of
-
- Journal:
- Bulletin of the Australian Mathematical Society / Volume 97 / Issue 2 / April 2018
- Published online by Cambridge University Press:
- 30 January 2018, pp. 194-199
- Print publication:
- April 2018
-
- Article
-
- You have access
- Export citation
-
For two given graphs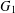
$G_{1}$ and 
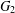$G_{2}$, the planar Ramsey number 
$PR(G_{1},G_{2})$ is the smallest integer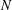 
$N$ such that every planar graph 
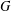$G$ on 
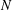$N$ vertices either contains 
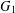$G_{1}$, or its complement contains 
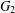$G_{2}$. Let 
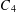$C_{4}$ be a quadrilateral, 
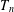$T_{n}$ a tree of order 
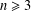$n\geq 3$ with maximum degree 
$k$, and 
$K_{1,k}$ a star of order 
$k+1$. We show that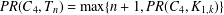 
$PR(C_{4},T_{n})=\max \{n+1,PR(C_{4},K_{1,k})\}$. Combining this with a result of Chen et al. [‘All quadrilateral-wheel planar Ramsey numbers’, Graphs Combin.33 (2017), 335–346] yields exact values of all the quadrilateral-tree planar Ramsey numbers.
