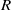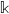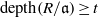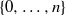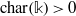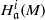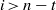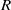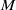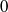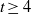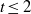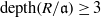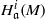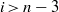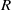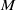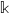1 results
Cohomological and projective dimensions
- Part of
-
- Journal:
- Compositio Mathematica / Volume 149 / Issue 7 / July 2013
- Published online by Cambridge University Press:
- 01 May 2013, pp. 1203-1210
- Print publication:
- July 2013
-
- Article
-
- You have access
- Export citation
-
Let
$\mathfrak{a}$ be a homogeneous ideal of a polynomial ring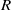 
$R$ in 
$n$ variables over a field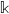 
$\mathbb{k}$. Assume that 
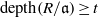$\mathrm{depth} (R/ \mathfrak{a})\geq t$, where 
$t$ is some number in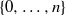 
$\{ 0, \ldots , n\} $. A result of Peskine and Szpiro says that if 
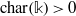$\mathrm{char} (\mathbb{k})\gt 0$, then the local cohomology modules 
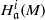${ H}_{\mathfrak{a}}^{i} (M)$ vanish for all 
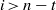$i\gt n- t$ and all 
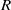$R$-modules 
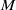$M$. In characteristic 
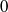$0$, there are counterexamples to this for all 
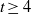$t\geq 4$. On the other hand, when 
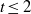$t\leq 2$, by exploiting classical results of Grothendieck, Lichtenbaum, Hartshorne and Ogus it is not difficult to extend the result to any characteristic. In this paper we settle the remaining case; specifically, we show that if 
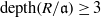$\mathrm{depth} (R/ \mathfrak{a})\geq 3$, then the local cohomology modules 
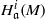${ H}_{\mathfrak{a}}^{i} (M)$ vanish for all 
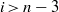$i\gt n- 3$ and all 
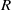$R$-modules 
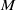$M$, whatever the characteristic of 
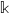$\mathbb{k}$ is.
