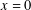1 results
OBLIQUE WAVE SCATTERING BY A RECTANGULAR SUBMARINE TRENCH
- Part of
-
- Journal:
- The ANZIAM Journal / Volume 56 / Issue 3 / January 2015
- Published online by Cambridge University Press:
- 16 March 2015, pp. 286-298
-
- Article
-
- You have access
- Export citation
-
The problem of oblique wave scattering by a rectangular submarine trench is investigated assuming a linearized theory of water waves. Due to the geometrical symmetry of the rectangular trench about the central line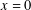
$x=0$, the boundary value problem is split into two separate problems involving the symmetric and antisymmetric potential functions. A multi-term Galerkin approximation involving ultra-spherical Gegenbauer polynomials is employed to solve the first-kind integral equations arising in the mathematical analysis of the problem. The reflection and transmission coefficients are computed numerically for various values of different parameters and different angles of incidence of the wave train. The coefficients are depicted graphically against the wave number for different situations. Some curves for these coefficients available in the literature and obtained by different methods are recovered.
