1 results
GLOBAL EXISTENCE AND BLOW-UP FOR A NON-NEWTON POLYTROPIC FILTRATION SYSTEM WITH NONLOCAL SOURCE
- Part of
-
- Journal:
- The ANZIAM Journal / Volume 50 / Issue 1 / July 2008
- Published online by Cambridge University Press:
- 01 July 2008, pp. 13-29
-
- Article
-
- You have access
- Export citation
-
This paper deals the global existence and blow-up properties of the following non-Newton polytropic filtration system with nonlocal source,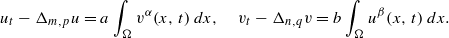
Under appropriate hypotheses, we prove that the solution either exists globally or blows up in finite time depending on the initial data and the relations between αβ and mn(p−1)(q−1). In the special case, α=n(q−1), β=m(p−1), we also give a criteria for the solution to exist globally or blow up in finite time, which depends on a,b and ζ(x),ϑ(x) as defined in our main results.
