We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
We provide a closed formula of Bowen type for the Hausdorff dimension of a very general shrinking target scheme generated by the nonautonomous dynamical system on the interval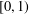 
$[0,1)$, viewed as 
$\mathbb{R}/\mathbb{Z}$, corresponding to a given method of Cantor series expansion. We also examine a wide class of examples utilising our theorem. In particular, we give a Diophantine approximation interpretation of our scheme.
