We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
We prove that the quantum cohomology ring of any minuscule or cominuscule homogeneous space, specialized at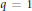 
$q\,=\,1$, is semisimple. This implies that complex conjugation defines an algebra automorphism of the quantum cohomology ring localized at the quantum parameter. We check that this involution coincides with the strange duality defined in our previous article. We deduce Vafa–Intriligator type formulas for the Gromov–Witten invariants.
