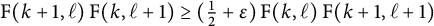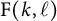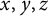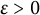1 results
On the cross-product conjecture for the number of linear extensions
- Part of
-
- Journal:
- Canadian Journal of Mathematics , First View
- Published online by Cambridge University Press:
- 19 January 2024, pp. 1-28
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
-
We prove a weak version of the cross-product conjecture: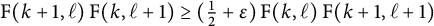
$\textrm {F}(k+1,\ell ) \hskip .06cm \textrm {F}(k,\ell +1) \ge (\frac 12+\varepsilon ) \hskip .06cm \textrm {F}(k,\ell ) \hskip .06cm \textrm {F}(k+1,\ell +1)$, where 
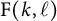$\textrm {F}(k,\ell )$ is the number of linear extensions for which the values at fixed elements 
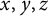$x,y,z$ are k and 
$\ell $ apart, respectively, and where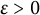 
$\varepsilon>0$ depends on the poset. We also prove the converse inequality and disprove the generalized cross-product conjecture. The proofs use geometric inequalities for mixed volumes and combinatorics of words.
