We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
${\textit{d}}$-process
The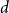 
$d$-process generates a graph at random by starting with an empty graph with 
$n$ vertices, then adding edges one at a time uniformly at random among all pairs of vertices which have degrees at most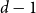 
$d-1$ and are not mutually joined. We show that, in the evolution of a random graph with 
$n$ vertices under the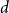 
$d$-process with 
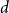$d$ fixed, with high probability, for each 
$j \in \{0,1,\dots,d-2\}$, the minimum degree jumps from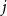 
$j$ to 
$j+1$ when the number of steps left is on the order of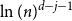 
$\ln (n)^{d-j-1}$. This answers a question of Ruciński and Wormald. More specifically, we show that, when the last vertex of degree 
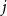$j$ disappears, the number of steps left divided by 
$\ln (n)^{d-j-1}$ converges in distribution to the exponential random variable of mean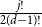 
$\frac{j!}{2(d-1)!}$; furthermore, these 
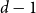$d-1$ distributions are independent.
