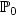We analyze two numerical schemes of Euler type in time and C 0finite-element type with $\mathbb{P}_1$ -approximation in space forsolving a phase-field model of a binary alloy with thermalproperties. This model is written as a highly non-linear parabolicsystem with three unknowns: phase-field, solute concentration andtemperature, where the diffusion for the temperature and soluteconcentration may degenerate. The first scheme is nonlinear, unconditionally stableand convergent. The other scheme is linear but conditionally stableand convergent. 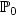A maximum principle is avoided in both schemes,using a truncation operator on the L 2 projection onto the $\mathbb{P}_0$ finite element for the discrete concentration. Inaddition, for the model when the heat conductivity and solutediffusion coefficients are constants, optimal error estimates forboth schemes are shown based on stability estimates.

Phase-field models, the simplest of which is Allen–Cahn's problem, are characterized by a small parameter ε that dictatesthe interface thickness. These models naturally call for mesh adaptation techniques, which rely on a posteriori error control. However, their error analysis usually deals with the underlying non-monotone nonlinearity via a Gronwall argument which leads to an exponential dependence on ε-2. Using an energy argument combined with a topological continuation argument and a spectral estimate, we establish an a posteriori error control result with only a low order polynomial dependence in ε-1. Our result is applicable to any conforming discretization technique that allows for a posteriori residual estimation. Residual estimators for an adaptive finite element scheme are derived to illustrate the theory.

In this paper we focus on melting and solidification processes described by phase-field models and obtain rigorous estimates for such processes. These estimates are derived in Section 2 and guarantee the convergence of solutions to non-constant equilibrium patterns. The most basic results conclude with the inequality (E2.31). The estimates in the remainder of Section 2 illustrate what obtains if the initial data is progressively more regular and may be omitted on first reading. We also present some interesting numerical simulations which demonstrate the equilibrium structures and the approach of the system to non-constant equilibrium patterns. The novel feature of these calculations is the linking of the small parameter in the system, δ, to the grid spacing, thereby producing solutions with approximate sharp interfaces. Similar ideas have been used by Caginalp and Sokolovsky [5]. A movie of these simulations may be found at http:www.math.cmu.edu/math/people/greenberg.html