We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
Let F(z) be a rational map with degree at least three. Suppose that there exists an annulus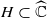 
such that (1) H separates two critical points of F, and (2) F:H→F(H) is a homeomorphism. Our goal in this paper is to show how to construct a rational map G by twisting F on H such that G has the same degree as F and, moreover, G has a Herman ring with any given Diophantine type rotation number.
