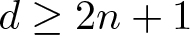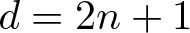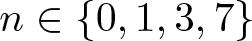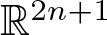We show that if an open set in $\mathbb{R}^d$ can be fibered by unit n-spheres, then $d \geq 2n+1$, and if $d = 2n+1$, then the spheres must be pairwise linked, and $n \in \left\{0, 1, 3, 7 \right\}$. For these values of n, we construct unit n-sphere fibrations in $\mathbb{R}^{2n+1}$.

Les mesures d'inégalité du revenu rassemblent deux types d'indicateurs décomposables : les indices décomposables en sous-populations et les indices décomposables en sources de revenu. Les premiers permettent de partager l'inégalité totale en une inégalité intragroupe et une inégalité intergroupe et les seconds d'attribuer à chaque facteur de revenu (revenu du travail, revenu du capital, taxes, etc.) une part de l'inégalité totale. Dans cet article, nous examinons d'une part la construction de ces techniques et d'autre part nous relatons les débats auxquels elles ont aboutis et plus particulièrement celui de la convergence vers un emploi simultané des deux types de décomposition.

A decomposition of a set X of words over a d-letter alphabet A = {a1,...,ad} is any sequence X1,...,Xd,Y1,...,Yd of subsets of A* such that the sets Xi, i = 1,...,d, are pairwise disjoint, their union is X, and for all i, 1 ≤ i ≤ d, Xi ~ aiYi, where ~ denotes the commutative equivalence relation. We introduce some suitable decompositions that we call good, admissible, and normal. A normal decomposition is admissible and an admissible decomposition is good. We prove that a set is commutatively prefix if and only if it has a normal decomposition. In particular, we consider decompositions of Bernoulli sets and codes. We prove that there exist Bernoulli sets which have no good decomposition. Moreover, we show that the classical conjecture of commutative equivalence of finite maximal codes to prefix ones is equivalent to the statement that any finite and maximal code has an admissible decomposition.

A stability theorem determines the extent to which the conclusions of a given theorem are affected if the assumptions of the theorem are not exactly but only approximately satisfied. The meaning of the word ‘approximately’ has to be defined exactly. The stability of decomposition theorems, of characterizations by independence and by regression properties are the primary object of the paper.