We consider a variation of the M/G/1 queue in which, when the system contains more than k customers, it switches from its initial general service distribution to a different general service distribution until the server is cleared, whereupon it switches back to the original service distribution. Using a technique due to Baccelli and Makowski we define a martingale with respect to an embedded process and from this arrive at a relationship between the process and a modified Markov renewal process. Using this an analysis of the stationary behaviour of the queue is possible.

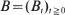 is standard Brownian motion and the supremum is taken over all stopping times
is standard Brownian motion and the supremum is taken over all stopping times 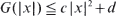 for some
for some 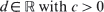 being given and fixed. The optimal stopping time is shown to be the hitting time by the reflecting Brownian motion
being given and fixed. The optimal stopping time is shown to be the hitting time by the reflecting Brownian motion 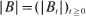 of the set of all (approximate) maximum points of the map
of the set of all (approximate) maximum points of the map 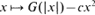 . The method of proof relies upon Wald's identity for Brownian motion and simple real analysis arguments. A simple proof of the Dubins–Jacka–Schwarz–Shepp–Shiryaev (square root of two) maximal inequality for randomly stopped Brownian motion is given as an application.
. The method of proof relies upon Wald's identity for Brownian motion and simple real analysis arguments. A simple proof of the Dubins–Jacka–Schwarz–Shepp–Shiryaev (square root of two) maximal inequality for randomly stopped Brownian motion is given as an application.