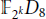Let $G$ be a finite group. We classify $G$-equivariant flow equivalence of non-trivial irreducible shifts of finite type in terms of
(i) elementary equivalence of matrices over $ZG$ and
(ii) the conjugacy class in $ZG$ of the group of G-weights of cycles based at a fixed vertex.
In the case $G = Z/2$, we have the classification for twistwise flow equivalence. We include some algebraic results and examples related to the determination of $E(ZG)$ equivalence, which involves $K_1(ZG)$.