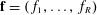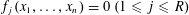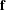Let 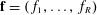$\mathbf{f}=(f_{1},\ldots ,f_{R})$ be a system of polynomials with integer coefficients in which the degrees need not all be the same. We provide sufficient conditions for which the system of equations 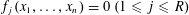$f_{j}(x_{1},\ldots ,x_{n})=0~(1\leqslant j\leqslant R)$ satisfies a general local to global type statement, and has a solution where each coordinate is prime. In fact we obtain the asymptotic formula for number of such solutions, counted with a logarithmic weight, under these conditions. We prove the statement via the Hardy–Littlewood circle method. This is a generalization of the work of Cook and Magyar [‘Diophantine equations in the primes’, Invent. Math.198 (2014), 701–737], where they obtained the result when the polynomials of 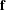$\mathbf{f}$ all have the same degree. Hitherto, results of this type for systems of polynomial equations involving different degrees have been restricted to the diagonal case.

For homogeneous decomposable forms F(X) in n variables with integer coefficients, we consider the number of integer solutions ${\bf x}\in\mathbb{Z}^n$ to the inequality $|F({\bf x})|\le m$ as $m\rightarrow\infty$. We give asymptotic estimates which improve on those given previously by the author in Ann. of Math. (2) 153 (2001), 767–804. Here our error terms display desirable behaviour as a function of the height whenever the degree of the form and the number of variables are relatively prime.