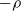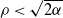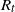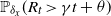A large deviation theorem for a supercritical super-Brownian motion with absorption

Abstract

We consider a one-dimensional superprocess with a supercritical local branching mechanism $\psi$, where particles move as a Brownian motion with drift $-\rho$ and are killed when they reach the origin. It is known that the process survives with positive probability if and only if $\rho<\sqrt{2\alpha}$, where $\alpha=-\psi'(0)$. When $\rho<\sqrt{2 \alpha}$, Kyprianou et al. [18] proved that $\lim_{t\to \infty}R_t/t =\sqrt{2\alpha}-\rho$ almost surely on the survival set, where $R_t$ is the rightmost position of the support at time t. Motivated by this work, we investigate its large deviation, in other words, the convergence rate of $\mathbb{P}_{\delta_x} (R_t >\gamma t+\theta)$ as $t \to \infty$, where $\gamma >\sqrt{2 \alpha} -\rho$, $\theta \ge 0$. As a by-product, a related Yaglom-type conditional limit theorem is obtained. Analogous results for branching Brownian motion can be found in Harris et al. [13].

1. Introduction and main results

Suppose that $X=\{X_t\,{:}\, t\ge 0\}$ is a one-dimensional super-Brownian motion (SBM) with motion corresponding to a Brownian motion with drift $-\rho $ , absorbed at 0, and branching mechanism $\psi$ taking the form

\begin{equation*} \psi(\lambda)=-\alpha \lambda+\beta \lambda^2 +\int_{(0,\infty)}({\textrm{e}}^{-\lambda x}-1+\lambda x)\Pi({\textrm{d}} x),\quad \lambda>0,\end{equation*}

where $\alpha=-\psi'(0)\in(0,\infty)$ , $\beta > 0$ and $\Pi$ is a $\sigma$ -finite measure on $(0,\infty)$ satisfying

\begin{equation*}\int_{(0,\infty)}(x\land x^2)\Pi({\textrm{d}} x)<\infty.\end{equation*}

This process is called an SBM with absorption.

Let $\mathcal{M}_F(0,\infty)$ be the space of finite measures on $(0,\infty)$ equipped with the topology of weak convergence, and note that X is an $\mathcal{M}_F(0,\infty)$ -valued Markov process under $\mathbb{P}_{\mu}$ for each $\mu \in \mathcal{M}_F(0,\infty)$ , where $\mathbb{P}_{\mu}$ is the law of X with initial configuration $\mu$ . The existence of such superprocesses is well known; see e.g. Dynkin [10] and Li [21]. Let $C_b^+(0,\infty) $ be the space of bounded, positive, continuous functions on $(0,\infty)$ and let $C_b^2(0,\infty)^+$ be the space of bounded, positive, twice continuously differentiable functions on $(0,\infty) $ . Let $C_0^2(0,\infty)^+$ denote the subset of $C_b^2(0,\infty)^+$ consisting of functions that vanish at the boundary of $(0,\infty)$ . We shall use standard inner product notation, for $f \in C_b^+(0,\infty) $ and $\mu \in \mathcal{M}_F(0,\infty)$ , $\langle f,\mu \rangle =\int_{\mathbb{R}}f(x)\mu({\textrm{d}} x)$ . Accordingly we shall write $\|\mu\|\,{:\!=}\, \langle 1,\mu\rangle $ . The following standard result from the theory of superprocesses describes the evolution of X as a Markov process. For all $f \in C_b^+(0,\infty) $ and $\mu \in \mathcal{M}_F(0,\infty)$ ,

(1.1) \begin{equation}\mathbb{E}_{\mu}\bigl({\textrm{e}}^{-\langle f,X_t \rangle}\bigr)={\textrm{e}}^{-\langle v_f(\cdot,t),\mu \rangle},\end{equation}

where $v_f(x,t)$ is the unique positive solution to the equation

(1.2) \begin{equation}v_f(x,t)=\mathbb{E}_{-\rho}^x[f(B(t));\tau_0>t]-\mathbb{E}_{-\rho}^x \biggl[\int_{0}^{t }\psi(v_f(B(s),t-s))\,\textrm{d} s;\tau_0>t\biggr],\quad x>0,\ t \ge 0.\end{equation}

Here, $\mathbb{E}_{-\rho}^x$ is the expectation with respect to $\mathbb{P}_{-\rho}^x$ , under which $\{B(t)\}_{t \ge 0}$ is a Brownian motion with drift $-\rho$ , starting from $x>0$ , and $\tau_0\,{:\!=}\, \inf\{t>0\,{:}\, B(t)=0\}$ . Note that the integral equation (1.2) is equivalent to the partial differential equation

(1.3) \begin{equation}\left\{\begin{aligned}& \dfrac{\partial}{\partial t} v_f(x,t)=\dfrac{1}{2}\dfrac{\partial^2}{\partial x^2} v_f(x,t)-\rho \dfrac{\partial}{\partial x} v_f(x,t)-\psi(v_f(x,t)),\\[3pt]& v_f(0+,t)=0,\quad v_f(x,0)=f(x),\quad t\ge 0,\ \ x>0,\end{aligned}\right.\end{equation}

where $f \in C_0^2(0,\infty)^+$ . We refer the reader to Dynkin [10], Li [21], and Kyprianou et al. [18] for further details.

In this article we always assume that

\begin{equation*} \int_{}^{\infty}\dfrac{1}{\sqrt{\int_{\lambda^*}^{r}\psi(u)\,{\textrm{d}} u}}\,{\textrm{d}} r<\infty,\end{equation*}

where $\lambda^*$ is the largest root of the equation $\psi(\lambda)=0$ . This condition implies that $\int_{}^{\infty}\psi(u)^{-1}\,{\textrm{d}} u<\infty$ (see [29, Proposition 4.2]), which in turn guarantees that X becomes extinct with positive probability in finite time. Furthermore, we shall also assume the integrability condition

(1.4) \begin{equation}\int_{0+} \dfrac{\varphi(\lambda)}{\lambda} \,{\textrm{d}} \lambda <\infty,\end{equation}

where $\varphi$ is defined by

(1.5) \begin{equation}\varphi(\lambda)=\dfrac{\psi(\lambda)}{\lambda} +\alpha, \quad \lambda>0.\end{equation}

Note that $\varphi(\lambda)$ is increasing in $\lambda$ and, for any $\lambda>0$ , $\varphi(\lambda)>0$ .

There exists a suite of literature which studies the asymptotic behavior of branching Brownian motion (BBM) with absorption. We define the process as follows. Starting with an initial particle sitting at $x>0$ , this particle moves according to a one-dimensional Brownian motion with drift $-\rho$ until an independent exponentially distributed time with rate q. As usual, $\mathbb{N}$ denotes the set $\{0,1,2, \ldots\}$ . When the particle dies, it gives birth to a random number $L\in \mathbb{N}\setminus\{1\}$ of independent branching Brownian motions started at the position where it dies. We refer the reader to Chauvin and Rouault [7] for a rigorous definition. Assume that $m=\mathbb{E}(L)\in(1,\infty)$ , which means that the process is supercritical. We kill the particles when they first hit the origin. Let $\textbf{P}_{x}$ be the probability measure of the above BBM with absorption. Let $\mathcal{N}_t^{-\rho} (a,b)$ denote the set of surviving particles and let $N_t^{-\rho} (a,b)$ be the number of surviving particles in the interval (a, b) at time t. Let the configuration of particles at time t be $\{Z_u(t)\,{:}\, u\in \mathcal{N}_t^{-\rho}(0,\infty)\}$ . The rightmost particle in this process is defined to be $R_t^{Z}\,{:\!=}\, \sup\{Z_u(t)\,{:}\, u \in \mathcal{N}_t^{-\rho}(0,\infty)\}$ . Kesten [16] proved that the process dies out almost surely when $\rho \ge \sqrt{2q(m-1)}$ , while there is a positive probability of survival when $\rho < \sqrt{2q(m-1)}$ . Thus $\rho=\sqrt{2q(m-1)}$ is the critical drift separating the supercritical case $\rho < \sqrt{2q(m-1)}$ and the subcritical case $\rho > \sqrt{2q(m-1)}$ . Moreover, in the critical case, upper and lower bounds on the survival probability which were obtained by Kesten [16] and improved by Berestycki et al. [2] have been further improved by Maillard and Schweinsberg [25]. In the supercritical case, Chauvin and Rouault [7] gave an asymptotic expression for the probability of existence of particles to the right of $ct+\theta$ at some large time in the setting of BBM without drift and absorption, where $c>\sqrt{2q(m-1)}$ . Furthermore, they obtained a limit distribution for the number of particles that drift above $ct+\theta$ at time t conditioned on the presence of such particles. Related results for BBM with absorption were proved in Harris et al. [13]. They derived the asymptotic behavior of the probability that the rightmost particle has traveled at a faster speed than usual through the one-sided F-KPP equation. Furthermore, they obtained an analogous Yaglom-type conditional limit theorem by using the method in [7]. The large-time asymptotic formula for the survival probability was given by Harris and Harris [12] in the subcritical case. In addition, we refer the reader to Liu [23] and Berestycki et al. [1] for the nearly critical case.

One important initial motivation for super-Brownian motion is the modeling of spatial populations. Moreover, remarkable connections have been obtained between super-Brownian motion and models from statistical mechanics (Derbez and Slade [8]) or infinite particle systems (Durrett and Perkins [9]). This suggests that, like ordinary Brownian motion, super-Brownian motion is a universal object which arises in a variety of different contexts. In addition, the SBM arises as the high-density limit of BBM. We refer the reader to Le Gall [19], who provides a construction of the SBM via an approximation by branching Brownian motions. However, unlike the case of BBM, there are very few results for the SBM with absorption. A conditional law of SBM with absorption was characterized by Li [20] in terms of the solution to the initial value problem of a parabolic differential equation. A large deviation result for the growth rate of the support was given in Engländer [11] for a d-dimensional supercritical SBM. However, he only gave a conjecture for the upper tail. Recently, conclusions regarding the growth in the rightmost point in the support were obtained by Kyprianou et al. [18]. The key tool there is the pathwise backbone decomposition in Berestycki et al. [3]. Furthermore, the survival probability for SBM with absorption was given in Li and Zhu [22], which is an analogue of the result in [12]. In the setting of standard SBM, Kyprianou et al. [17] offered a probabilistic treatment of the classical problem of existence, uniqueness, and asymptotics of monotone solutions to the traveling wave equation associated to the parabolic semigroup equation. Ren et al. [27] established limit theorems for the supremum of the support and gave some large deviation results. We refer the reader to Hou et al. [14] and Liu et al. [24] for further results of Yaglom limit theorem for superprocesses.

Let $R_t\,{:\!=}\, \inf\{y>0\,{:}\, X_t(y,\infty)=0\}$ be the rightmost position of X and denote the extinction time of X by $\zeta\,{:\!=}\, \inf \{t>0\,{:}\, \|X_t\|=0\}$ . Kyprianou et al. [18] proved that for any $-\infty<\rho<\sqrt{2\alpha}$ and $x>0$ ,

\begin{equation*}\lim_{t\to \infty} \dfrac{R_t}{t}=\sqrt{2\alpha}-\rho \quad \text{on $\{\zeta=\infty\}$}\end{equation*}

$\mathbb{P}_{\delta_x}$ -almost surely.

In this paper we will consider the asymptotic behavior of $\mathbb{P}_{\delta_x}(R_t > \gamma t+\theta)$ as $t\to\infty$ , where $\gamma >\sqrt{2\alpha}-\rho>0$ and $\theta \ge 0$ . Harris et al. [13] studied this question for BBM with absorption, in which particles undergo dyadic branching at rate q. For any $c>\sqrt{2q}-\rho$ and all $x>0$ , $\theta \ge 0$ , they obtained that

\begin{equation*} \lim_{t\to \infty}\textbf{P}_{x}(R_t^{Z}\ge ct+\theta)\dfrac{\sqrt{2\pi t}}{1-{\textrm{e}}^{-2cx}}\,{\textrm{e}}^{-(c+\rho)(x-\theta)+(\frac{1}{2}(c+\rho)^2-q)t}=K,\end{equation*}

where K is a positive constant independent of x and $\theta$ . Moreover, they showed that there exists a probability distribution $(\Pi_i)_{i \ge 1}$ defined on $\mathbb{N}$ such that

\begin{equation*}\lim_{t\to \infty} \textbf{P}_{x}(N_t^{-\rho} (ct,\infty) =i\mid N_t^{-\rho} (ct,\infty)>0) =\Pi_i,\end{equation*}

and the distribution has an expectation equal to $1/K(c+\rho)$ .

Our first result, which extends the conclusion of Harris et al. [13] to super-Brownian motion, gives a large-time asymptotic formula for the deviation probability $\mathbb{P}_{\delta_x}(R_t > \gamma t +\theta)$ which has a decay rate similar to $\textbf{P}_{x}(R_t^{Z}\ge ct+\theta)$ . Our second result yields a Yaglom-type conditional limit theorem for the SBM with absorption.

Theorem 1.1. For any $ \gamma>\sqrt{2\alpha}-\rho$ and all $x>0$ , $\theta \ge 0$ , we have

(1.6) \begin{equation}\lim_{t\to \infty} \mathbb{P}_{\delta_x}(R_t > \gamma t +\theta)\dfrac{\sqrt{2 \pi t}}{1-{\textrm{e}}^{-2\gamma x}} \,{\textrm{e}}^{(\frac{1}{2}(\gamma +\rho )^2-\alpha)t } \,{\textrm{e}}^{-(\gamma+\rho) (x-\theta)}=C,\end{equation}

where C is a positive constant independent of x and $\theta$ .

Theorem 1.2. For any $ \gamma>\sqrt{2\alpha}-\rho$ and all $x>0$ , there exists a probability distribution $\pi$ defined on $(0,\infty)$ such that

\begin{equation*}\lim_{t\to \infty} \mathbb{P}_{\delta_x} (X_t (\gamma t ,\infty) \in \cdot\mid R_t >\gamma t ) =\pi (\cdot),\end{equation*}

and the distribution $\pi$ has a finite expectation equal to ${{1}/{(C (\gamma+\rho))}}$ .

Suppose that $ \Theta_{\gamma t} $ is a shift operator for X, that is, a map of $\mathcal{M}_F (0,\infty)$ into itself satisfying $ \Theta_{\gamma t}X_t(\cdot)=X_t(\cdot+\gamma t)$ . For any $t>0$ , let $Y_t=\Theta_{\gamma t}X_t$ , then $Y_t \in \mathcal{M}_F(0,\infty)$ . Our third result gives a Yaglom limit theorem for the superprocess Y.

Theorem 1.3. For any $ \gamma>\sqrt{2\alpha}-\rho$ and $x>0$ , there exists a probability distribution $ \textbf{Q}$ on $ \mathcal{M}_F(0,\infty)$ such that

\begin{equation*}\mathbb{P}_{\delta_x} \big( Y_t \in \cdot| R_t >\gamma t \big) \xrightarrow [t \to \infty] {w} \textbf{Q} (\cdot),\end{equation*}

where $ \xrightarrow {w} $ stands for weak convergence. Moreover, for any $f \in C_b^+(0,\infty)$ ,

\begin{align*}\lim_{t \to \infty}\mathbb{P}_{\delta_x}\bigl({\textrm{e}}^{-\langle f, Y_t\rangle } \mid R_t > \gamma t \bigr) = 1-C^{-1} \int_{0}^{\infty} f(z) \,\mathbb{E} \bigl({\textrm{e}}^{ - \int_{0}^{\infty} \varphi (v_f (W_2(r)+z-\gamma r ,r)) \,{\textrm{d}} r}\bigr) \,{\textrm{e}}^{-(\rho+\gamma)z} \,{\textrm{d}} z ,\end{align*}

where C is as defined in (1.6), $v_f$ is the unique positive solution to equation (1.2), and $\{W_2(t)\}_{t\ge0}$ is a standard Brownian motion with associated expectation $\mathbb{E}$ .

The remainder of the paper is structured as follows. In the next section we first summarize some important results related to Brownian motion and the Brownian bridge. Then we introduce the maximum principle and obtain upper bounds for the solution of a singular boundary value problem. Section 3 will be devoted to the proof of Theorem 1.1. The proofs of Theorems 1.2 and 1.3 are given in Section 4.

2. Preliminaries

In this section we summarize results about Brownian motion and the Brownian bridge as well as the PDE with singular boundary conditions, all of which will play an important role in the proofs of the main results. We refer the reader to Borodin and Salminen [5] for further properties of Brownian motion and the Brownian bridge.

2.1. Brownian motion and the Brownian bridge

Recall that $\{\{B(t)\}_{t\ge0};\ \mathbb{P}_{-\rho}^x, x\in \mathbb{R} \}$ is a Brownian motion with drift $-\rho$ . For any $x \in \mathbb{R}$ , it is well known that $\{{\textrm{e}}^{-\rho (B(t)-x) -\rho^2 t/2} \,{:}\, t\ge 0\}$ is a positive $ \mathbb{P}_{0}^x$ -martingale with mean 1. We have the following change of measure (see [5, page 71]):

(2.1) \begin{equation}\dfrac{{\textrm{d}} \mathbb{P}_{-\rho}^x}{{\textrm{d}} \mathbb{P}_{0}^x}\bigg|_{\sigma(B(s)\,{:}\, 0\le s \le t)}={\textrm{e}}^{-\rho (B(t)-x) -\rho^2 t/2}, \quad \text{$\mathbb{P}_{0}^x$-a.s.}\end{equation}

According to Revuz and Yor [28, page 53], we have the following laws of the iterated logarithm of Brownian motion.

Lemma 2.1. (Laws of the iterated logarithm.)

\begin{align*}\limsup_{t \to \infty} \dfrac{B(t)}{\sqrt{2 t \ln \ln t}} & =1, \quad \text{$\mathbb{P}_0^x$-}a.s.,\\[3pt] \liminf_{t \to \infty} \dfrac{B(t)}{\sqrt{2 t \ln \ln t}} & =-1, \quad \text{$\mathbb{P}_0^x$-}a.s.\end{align*}

Below we are going to introduce results pertaining to the Brownian bridge. Under $\mathbb{P}$ , let $\{B_{x,z}^t(r)\}_{0 \le r\le t}$ be a Brownian bridge from x to z over time t, with associated expectation $\mathbb{E}$ . Roughly speaking, a Brownian motion starting from x and conditioned to be at point z at time t is a Brownian bridge from x to z. We can represent the Brownian bridge $\{B_{x,z}^t(r)\}_{0 \le r\le t}$ as

(2.2) \begin{equation}B_{x,z}^t(r)=W(r)-\dfrac{r}{t}W(t)+x+\dfrac{r}{t}(z-x),\end{equation}

where W is a standard Brownian motion starting at the origin. Moreover, we make the following simultaneous construction of all the Brownian bridges $B_{x,z}^{t}(\cdot)$ with indices $t>0$ , $x,z\in \mathbb{R}$ using two independent Brownian motions $\{W_1(t)\}_{t \ge 0}$ and $\{W_2(t)\}_{t\ge0}$ starting at the origin:

(2.3) \begin{equation}B_{x,z}^{t}(r)=\left \{\begin{aligned}W_1(r)-\dfrac{r}{t}W_1(t)+x+\dfrac{r}{t}(z-x), \quad & r \in [0,\tau_0^{t}),\\ \\[-8pt] \tilde{B}_{z,0}^{t-\tau_0^{t}}(t-r), \quad & r \in [\tau_0^{t},t],\end{aligned}\right.\end{equation}

where

\begin{align*} \tau_0^t=\tau_0^t(x,z)&\,{:\!=}\, \inf\{r\ge 0\,{:}\, B_{x,z}^t(r)=0\}\notag \\[3pt] &=\inf\biggl\{r \ge 0 \,{:}\, W_1(r)-\dfrac{r}{t}W_1(t)+x+\dfrac{r}{t}(z-x)=0\biggr\}.\end{align*}

In addition, for any $s>0$ ,

\begin{equation*}\tilde{B}_{z,0}^{s}(u)\,{:\!=}\, W_2(u)-\dfrac{u}{s}W_2(s)+z -\dfrac{u}{s} z, \quad u\in [0,s].\end{equation*}

Note that since $\tau_0^{t}$ is determined entirely by the path of $W_1$ , $\tau_0^{t}$ is independent of $W_2$ . Now we will be able to state the following lemma (see Harris et al. [13]), which considers how the Brownian bridge behaves for large t.

Lemma 2.2. For any $\gamma >0$ and $\theta \ge0$ , we have

\begin{equation*}\tau_0^{t}(x,z+\gamma t+\theta) \to T_0\,{:\!=}\, \inf\{r \ge 0 \,{:}\, W_1(r)+x+\gamma r=0\}\end{equation*}

$\mathbb{P}$ -almost surely as $t \to \infty$ . Furthermore, letting $t \to \infty$ , we have

\begin{equation*}B_{x,z+\gamma t+\theta}^{t}(r) \to W_1(r)+x+\gamma r, \quad \text{$\mathbb{P}$-}a.s.\end{equation*}

and uniformly on any interval $[0, s]$ where $s<T_0$ .

Note that although $T_0$ may be infinite, $T_0< \infty$ $\mathbb{P}$ -almost surely. The following lemma gives the time reversal property of the Brownian bridge, which will be used in the later proofs.

Lemma 2.3. (Time reversal property.) For any $t\ge0$ and x, $z \in \mathbb{R}$ , we have

\begin{equation*}\{\{B_{x,z}^t(r)\}_{0\le r \le t} ;\ \mathbb{P}\} \sim \{\{B_{z,x}^t(t-r)\}_{0\le r \le t};\ \mathbb{P}\},\end{equation*}

where ‘ $\sim$ ’ means identical in law.

Proof. This can be easily obtained by the construction of the Brownian bridge (2.2).

2.2. Some results on the PDE with singular boundary condition

For any $x \in \mathbb{R}$ , suppose that the superprocess $X^1=\{X_t^1 \,{:}\, t \ge0 \}$ under $\mathbb{P}_{\delta_x}$ has the same branching mechanism as $ (X, \mathbb{P}_{\delta_x})$ . However, the underlying motion associated with $X^1$ is that of a Brownian motion with drift $-\rho$ (i.e. without absorption). The random measure $X_t^1 $ is characterized by the Laplace functional

(2.4) \begin{equation}\mathbb{E}_{\delta_x}\bigl({\textrm{e}}^{-\langle f,X_t^1 \rangle}\bigr)={\textrm{e}}^{-u_f(x,t)},\end{equation}

where $f \in C_b^+(\mathbb{R})$ and $u_f(x,t)$ is the unique positive solution to the equation

(2.5) \begin{equation}\dfrac{\partial}{\partial t} u_f(x,t)=\dfrac{1}{2}\dfrac{\partial^2}{\partial x^2} u_f(x,t)-\rho \dfrac{\partial}{\partial x} u_f(x,t)-\psi(u_f(x,t)),\quad x \in \mathbb{R},\ t>0,\end{equation}

with initial condition $u_f(x,0)=f(x)$ . We refer the reader to Li [21] for further details. Based on the above description, it is easy to see that

(2.6) \begin{equation}\mathbb{E}_{\delta_x} \bigl({\textrm{e}}^{-\langle f,X_t^1 \rangle} \bigr)\le \mathbb{E}_{\delta_x} \bigl({\textrm{e}}^{-\langle f,X_t \rangle} \bigr),\end{equation}

where $f \in C_b^+(0,\infty)$ and $x>0$ . For any $n\in \mathbb{N}$ and $y \ge 0$ , define $g_n^y\,{:}\, \mathbb{R} \to [0,1]$ by

(2.7) \begin{equation}g_n^y(x)=\left \{\begin{aligned}0, \quad & x\le y \quad \text{or} \quad x\ge n+1,\\1, \quad & y+\dfrac{1}{n}\le x\le n.\end{aligned}\right.\end{equation}

Then $\{g_n^y\}_{n\ge 1}$ can be extended to a sequence of functions in $ C_0^2(\mathbb{R})^+$ which is pointwise increasing and satisfies $g_n^y \uparrow 1_{(y,\infty)}$ as $n \to\infty$ . Assume that $u_n^y(x,t,\theta)$ is the unique positive solution to the equation

(2.8) \begin{equation}\left\{\begin{aligned}&\dfrac{\partial}{\partial t}u_n^y(x,t,\theta)=\dfrac{1}{2}\dfrac{\partial^2}{\partial x^2}u_n^y(x,t,\theta)-\rho \dfrac{\partial}{\partial x}u_n^y(x,t,\theta)-\psi(u_n^y(x,t,\theta)),\\ \\[-8pt] &u_n^y(x,0,\theta)=\theta g_n^y(x), \quad t\ge 0, \ x \in \mathbb{R},\end{aligned}\right.\end{equation}

where $\rho \in \mathbb{R} $ and $g_n^y$ is defined in (2.7). It follows from (2.4) and (2.5) that

(2.9) \begin{equation}u_n^y(x,t,\theta)=-\!\log \mathbb{E}_{\delta_x} \bigl({\textrm{e}}^{-\theta \langle g_n^y,X_t^1 \rangle} \bigr).\end{equation}

For any $n\in \mathbb{N}$ and $y\ge 0$ , define

(2.10) \begin{equation}f_n^y (x)=g_n^y (x), \quad x>0.\end{equation}

Then $\{f_n^y\}_{n\ge 1}$ is a sequence of functions in $C_0^2(0,\infty)^+$ which is pointwise increasing and satisfies $f_n^y \uparrow 1_{(y,\infty)}$ as $n \to\infty$ . Suppose that $v_n^y(x,t,\theta)$ is the unique positive solution to the equation

(2.11) \begin{equation}\left\{\begin{aligned}&\dfrac{\partial}{\partial t}v_n^y(x,t,\theta)=\dfrac{1}{2}\dfrac{\partial^2}{\partial x^2}v_n^y(x,t,\theta)-\rho \dfrac{\partial}{\partial x}v_n^y(x,t,\theta)-\psi(v_n^y(x,t,\theta)),\\ \\[-8pt] &v_n^y(x,t,\theta)=0 , \quad v_n^y(x,0,\theta)=\theta f_n^y(x), \quad t\ge 0, \ x >0.\end{aligned}\right.\end{equation}

Using arguments similar to that leading to (2.9), we get

\begin{equation*} v_n^y(x,t,\theta)=-\log \mathbb{E}_{\delta_x} \bigl({\textrm{e}}^{-\theta \langle f_n^y,X_t \rangle} \bigr).\end{equation*}

The following lemma gives the maximum principle for solutions of partial differential equations, which is a slight modification of Lemma 2.2 in [27] and was proved in [22].

Lemma 2.4. (Maximum principle.) Let $v_1(x,t)$ and $v_2(x,t)$ be non-negative functions satisfying

\begin{align*}&\dfrac{\partial}{\partial t}v_2(x,t)-\dfrac{1}{2}\dfrac{\partial^2}{\partial x^2}v_2(x,t)- \rho \dfrac{\partial}{\partial x}v_2(x,t)+ \psi(v_2(x,t)) \\[3pt] &\quad \ge \dfrac{\partial}{\partial t}v_1(x,t)-\dfrac{1}{2}\dfrac{\partial^2}{\partial x^2}v_1(x,t)-\rho \dfrac{\partial}{\partial x}v_1(x,t) +\psi(v_1(x,t)),\end{align*}

where $t>0$ , $x\in(a,b)$ , $\rho \in \mathbb{R}$ . In addition, suppose that $v_1(x,t)$ and $v_2(x,t)$ satisfy the boundary conditions

\begin{equation*}v_1(x,0) \le v_2(x,0),\quad x\in(a,b),\end{equation*}

where $-\infty \le a<b\le \infty$ . Moreover, if $a>-\infty $ , we assume that $v_1(a,t) \le v_2(a,t)$ for all $t>0$ . If $b<\infty $ , we assume that $v_1(b,t) \le v_2(b,t)$ for all $t>0$ . Then we have

\begin{equation*}v_1(x,t) \le v_2(x,t),\quad t>0,\ x\in(a,b).\end{equation*}

Below we will give an upper bound for the solution to the partial differential equation (2.8) using Lemma 2.4.

Lemma 2.5. For any $\theta>0$ , $n\ge 1$ , suppose that $u_n^y(x,t,\theta)$ is the unique positive solution to equation (2.8). Then we have

\begin{equation*}u_n^y(x,t,\theta)\le \dfrac{\alpha}{\beta (1-{\textrm{e}}^{-\alpha t})}, \quad t>0, \ x\in \mathbb{R}\end{equation*}

and

\begin{equation*}u_n^y(x,t,\theta)\le \theta {\textrm{e}}^{\alpha t}, \quad t>0, \ x\in \mathbb{R}.\end{equation*}

Proof. Suppose that $u(x,t,\theta)$ is the solution to the equation

(2.12) \begin{equation}\left\{\begin{aligned}&\dfrac{\partial}{\partial t}u(x,t,\theta)=\dfrac{1}{2}\dfrac{\partial^2}{\partial x^2}u(x,t,\theta)-\rho \dfrac{\partial}{\partial x}u(x,t,\theta)+\alpha u(x,t,\theta)-\beta u(x,t,\theta)^2,\\ \\[-8pt] &u(x,0,\theta)=\theta, \quad t\ge 0,\ x\in \mathbb{R}.\end{aligned}\right.\end{equation}

It is easy to verify that

\begin{equation*}u(x,t,\theta)=\dfrac{\theta \alpha \textrm{e}^{\alpha t}}{\alpha+\theta \beta (\textrm{e}^{\alpha t}-1) }\end{equation*}

is a special solution to (2.12). Combining (2.8) and (2.12), we obtain that

\begin{align*}&\dfrac{\partial}{\partial t}u(x,t,\theta) -\dfrac{1}{2}\dfrac{\partial^2} {\partial x^2} u(x,t,\theta)+ \rho \dfrac{\partial}{\partial x}u(x,t,\theta) +\psi(u(x,t,\theta))\\[3pt] &\quad \ge \dfrac{\partial}{\partial t}u_n^y(x,t,\theta) -\dfrac{1}{2}\dfrac{\partial^2} {\partial x^2} u_n^y(x,t,\theta)+ \rho \dfrac{\partial}{\partial x}u_n^y(x,t,\theta)+\psi(u_n^y(x,t,\theta)),\end{align*}

and $u_n^y(x,0, \theta) \le u(x,0,\theta)$ , $x \in \mathbb{R}$ . Thus, for any $t\ge0$ and $n\ge 1$ , it follows from Lemma 2.2 that

\begin{equation*}u_n^y(x,t,\theta) \le u(x,t,\theta),\quad x \in \mathbb{R}.\end{equation*}

Therefore, for any $t>0$ and $x \in \mathbb{R}$ , we have

\begin{equation*}u_n^y(x,t,\theta) \le \dfrac{\theta \alpha {\textrm{e}}^{\alpha t}}{\alpha+\theta \beta ({\textrm{e}}^{\alpha t}-1) }\le \theta {\textrm{e}}^{\alpha t}.\end{equation*}

Moreover,

\begin{equation*}u_n^y(x,t,\theta) \le \dfrac{\theta \alpha {\textrm{e}}^{\alpha t}}{\alpha+\theta \beta ({\textrm{e}}^{\alpha t}-1) }\le \dfrac{\alpha}{\beta (1-{\textrm{e}}^{-\alpha t})},\quad t>0, \ x\in \mathbb{R}.\end{equation*}

This gives the desired result.

Remark 2.1. Based on the above analysis, it follows immediately from (2.6) and Lemma 2.5 that for any $x>0$ and $t>0$ ,

\begin{equation*}v_n^y(x,t,\theta) \le \dfrac{\alpha}{\beta (1-{\textrm{e}}^{-\alpha t})}\end{equation*}

and $v_n^y(x,t,\theta) \le \theta {\textrm{e}}^{\alpha t}$ .

Since $u_n^y(x,t,\theta)$ is monotone increasing in n, it is now possible to deduce by monotone convergence that $u^y(x,t,\theta)\,{:\!=}\, \lim_{n \to \infty}u_n^y(x,t,\theta)$ exists. Moreover, the dominated convergence theorem gives that

\begin{equation*}u^y(x,t,\theta)=-\log \mathbb{E}_{\delta_x} \bigl({\textrm{e}}^{-\theta \langle 1_{(y,\infty)},X_t^1 \rangle}\bigr).\end{equation*}

A similar statement tells us that $u^y(x,t)\,{:\!=}\, \lim_{\theta \to \infty}u^y(x,t,\theta)$ exists. Then we have

(2.13) \begin{equation}u^y(x,t)\,{:\!=}\, \lim_{\theta \to \infty}\lim_{n \to \infty} u_n^y(x,t,\theta), \quad t \ge 0,\ x>0.\end{equation}

Similarly, it is easy to verify that

(2.14) \begin{equation}v^y(x,t,\theta)\,{:\!=}\, \lim_{n\to \infty} v_n^y(x,t,\theta)=-\log \mathbb{E}_{\delta_x} \bigl({\textrm{e}}^{-\theta \langle 1_{(y,\infty)},X_t \rangle}\bigr)\end{equation}

and

(2.15) \begin{equation}v^y(x,t )\,{:\!=}\, \lim_{\theta \to \infty}\lim_{n\to \infty} v_n^y(x,t,\theta)\end{equation}

exist. In the following lemmas, we obtain upper bounds of $u^y(x,t)$ and $v^y(x,t)$ via Lemma 2.5 and Remark 2.1.

Lemma 2.6. For any $x \in \mathbb{R}$ , $y\ge 0$ , and $t > 0$ ,

\begin{equation*}u^y(x,t) \le \dfrac{\alpha}{\beta (1-{\textrm{e}}^{-\alpha t})}.\end{equation*}

Proof. This follows immediately from Lemma 2.5 and (2.13).

Lemma 2.7. For any $x >0$ , $y \ge 0$ , and $t > 0$ ,

\begin{equation*}v^y(x,t) \le \dfrac{\alpha}{\beta (1-{\textrm{e}}^{-\alpha t})}.\end{equation*}

Proof. This can be easily obtained by Remark 2.1 and (2.15).

3. Large deviation

In our proof of Theorem 1.1 we use ideas similar to those seen in Harris et al. [13], involving links with nonlinear partial differential equations and the Brownian bridge. Harris et al. [13] studied the BBM with absorption, in which particles undergo dyadic branching at rate q. Considering x, y, $t>0$ , It was shown in [13] that $u(t,x,y)\,{:\!=}\, \textbf{P}_x(R_t^Z>y)$ is the unique positive solution to the equation

\begin{equation*}\left\{\begin{aligned}&\dfrac{\partial}{\partial t}u(t,x,y)=\dfrac{1}{2}\dfrac{\partial^2}{\partial x^2}u(t,x,y)-\rho \dfrac{\partial}{\partial x}u(t,x,y)+qu(t,x,y)(1-u(t,x,y)),\\[3pt]&u(t,0+,y)=0 , \quad u(0,x,y)=1_{\{x>y\}}.\end{aligned}\right.\end{equation*}

When it comes to SBM with absorption, for any $x>0$ and $y\ge 0$ , we have

(3.1) \begin{align}\mathbb{P}_{\delta_x}(R_t > y)&= 1-\mathbb{P}_{\delta_x}(R_t \le y)\notag \\[3pt]&= 1-\mathbb{P}_{\delta_x}(X_t(y, \infty)=0)\notag \\[3pt]&= 1-\lim_{\theta \to \infty} \mathbb{E}_{\delta_x} \bigl({\textrm{e}}^{-\theta X_t(y,\infty)}\bigr)\notag \\[3pt]&= 1-\lim_{\theta \to \infty} \lim_{n \to \infty} \mathbb{E}_{\delta_x} \bigl({\textrm{e}}^{-\theta \langle f_n^y, X_t \rangle}\bigr)\notag \\[3pt]&= 1-\lim_{\theta \to \infty} \lim_{n \to \infty} \,{\textrm{e}}^{-v_n^y(x,t,\theta)},\end{align}

where $v_n^y(x,t,\theta)$ is the unique positive solution to (2.11). Thus additional work is needed to deal with the complications arising from the partial differential equation with singular boundary condition. Combining (2.15) and (3.1), we get

(3.2) \begin{equation}\mathbb{P}_{\delta_x}(R_t > y)=1-{\textrm{e}}^{-v^y(x,t)}.\end{equation}

Therefore, in order to investigate the large deviation probability $\mathbb{P}_{\delta_x}(R_t > \gamma t +\theta)$ , we only need to study the asymptotic order of $v^{\gamma t +\theta}(x,t)$ as $t\to \infty$ . Recall that, under $\mathbb{P}_{-\rho}^x $ , $\{B(t)\}_{t\ge 0}$ is a one-dimensional Brownian motion with drift $-\rho$ starting from $x>0$ and $\mathbb{E}_{-\rho}^x $ is the expectation with respect to $\mathbb{P}_{-\rho}^x $ . Define

(3.3) \begin{equation}\kappa(\lambda)=\lambda^{-1}\psi(\lambda)=-\alpha+\beta \lambda+\int_{(0,\infty)}\biggl(\dfrac{{\textrm{e}}^{-\lambda x}-1}{\lambda}+x\biggr)\Pi({\textrm{d}} x), \quad \lambda>0.\end{equation}

Below, we introduce a martingale for the SBM with absorption, which is a useful tool in the proof of Theorem 1.1.

Lemma 3.1. For any $t>0$ and $\theta \ge 0$ , the process

\begin{equation*}M_t^{y}(s,\theta)\,{:\!=}\, v_n^{y}(B(s\land \tau_0),t-(s\land \tau_0),\theta)\exp\biggl\{-\int_{0}^{s\land \tau_0} \kappa(v_n^{y}(B(r),t-r,\theta))\,{\textrm{d}} r\biggr\},\quad s \in [0,t],\end{equation*}

defines a uniformly integrable $\mathbb{P}_{-\rho}^x $ -martingale on $[0, t]$ , where $\tau_0=\inf \{s>0\,{:}\, B_s=0\}$ is the absorption time of $\{B(t)\}_{t\ge 0}$ and $\kappa$ is defined by (3.3).

Proof. According to Itô’s formula, $M_t^{y}(s,\theta)$ is a non-negative $\mathbb{P}_{-\rho}^x $ -local martingale on [0, t]. It follows from Remark 2.1 that

\begin{align*}M_t^{y}(s,\theta)&\le {\textrm{e}}^{\alpha t}v_n^{y}(B(s\land \tau_0),t-(s\land \tau_0),\theta)\exp\biggl\{-\int_{0}^{s\land \tau_0} \varphi (v_n^{y}(B(r), t-r,\theta)) \,{\textrm{d}} r\biggr\} \\ & \le \theta {\textrm{e}}^{2 \alpha t},\end{align*}

where $\varphi$ is defined in (1.5). Thus $M_t^{y}(s,\theta)$ is a uniformly integrable martingale on [0, t].

Although an upper bound of $v^y(x,t)$ has been obtained in Lemma 2.7, the bound is imprecise when t is sufficiently large. We will give another useful upper bound of $v^y(x,t)$ in the following lemma using the strong Markov property of Brownian motion and Chernoff’s inequality. Harris et al. [13] gave a similar upper bound for u(t, x, y), but due to the influence of the singular boundary condition, we cannot use their methods directly.

Lemma 3.2. For any $x>0$ and $y\ge 0$ , if $x\le y$ , then there exists a positive constant $C_0$ such that

\begin{equation*}v^y(x,t)\le C_0 \exp\biggl\{\biggl(\alpha -\frac{1}{2} \rho^2\biggr)t+\rho (x-y)-\frac{(y-x)^2}{2t}\biggr\}, \quad t>0.\end{equation*}

Proof. According to Lemma 3.1, we have $\mathbb{E}_{-\rho}^x[M_t^{y}(0,\theta)]=\mathbb{E}_{-\rho}^x[M_t^{y}(t,\theta)]$ , $t>0$ . Since $v_n^{y}(B(t\land \tau_0),t-(t\land \tau_0) =0$ for $ \tau_0 \le t$ , we can see that

\begin{equation*}v_n^y(x,t,\theta)=\mathbb{E}_{-\rho}^{x}\biggl[\theta 1_{\{\tau_0>t\}} f_n^y(B(t)) \exp\biggl\{-\int_{0}^{t} \kappa(v_n^y(B(r),t-r,\theta))\,{\textrm{d}} r\biggr\} \biggr],\end{equation*}

where $f_n^y$ is defined in (2.10). According to (2.14), taking limits as $n \to \infty$ , with the help of both monotone and dominated convergence, we have

(3.4) \begin{equation}v^y(x,t,\theta)=\mathbb{E}_{-\rho}^{x}\biggl[\theta 1_{\{\tau_0>t\}} 1_{(y,\infty)}(B(t)) \exp\biggl\{-\int_{0}^{t} \kappa(v^y(B(r),t-r,\theta))\,{\textrm{d}} r\biggr\} \biggr].\end{equation}

When $x \le y$ , it follows from (3.4) and the strong Markov property of Brownian motion that

\begin{align*}v^y(x,t,\theta)&=\mathbb{E}_{-\rho}^{x}\biggl[1_{\{\tau_y <t\}}\mathbb{E}_{-\rho}^y \biggl[ \theta 1_{ (y,\infty)}(B(t-\tau_y)) 1_{\{\tau_0>t-\tau_y\}}\\[3pt]&\quad \times\exp \biggl\{-\int_{0}^{t-\tau_y} \kappa(v^y(B(r),t-\tau_y-r,\theta))\,{\textrm{d}} r\biggr\}\biggr] \biggr]\\[3pt]&=\mathbb{E}_{-\rho}^{x}\bigl[1_{\{\tau_y <t\}}v^y(y,t-\tau_y,\theta) \bigr],\end{align*}

where $\tau_y\,{:\!=}\, \inf \{t>0\,{:}\, B(t)=y\}$ . Using arguments similar to that leading to (3.4), we get

(3.5) \begin{equation}v^y(x,t)=\mathbb{E}_{-\rho}^{x}\bigl[1_{\{\tau_y <t\}}v^y(y,t-\tau_y) \bigr].\end{equation}

We claim that for any $x>0$ , $M(x)\,{:\!=}\, \sup_{y \ge x, t>0} v^y(x,t)<\infty$ . If it is not true, then there exist $t_n \to 0$ and $y_n \ge x$ such that $v^{y_n} (x, t_n) \to \infty$ as $n \to \infty$ . It follows from (3.2) that

\begin{equation*}\lim_{n\to \infty } \mathbb{P}_{\delta_x}(R_{t_n}>y_n)=1-\lim_{n\to \infty }\,{\textrm{e}}^{-v^{y_n} (x, t_n)}=1.\end{equation*}

For any $x>0$ and $r\ge 0$ , let $B(x,r)=\{y\in \mathbb{R}\,{:}\, |x-y|\le r\}$ . On the other hand, according to Perkins [26, Section 3],

\begin{align*}\limsup_{n\to \infty} \mathbb{P}_{\delta_x}(R_{t_n}>y_n)&\le \limsup_{n\to \infty} \mathbb{P}_{\delta_x}(X_{t_n}[y_n,\infty)>0)\\[3pt] &\le \limsup_{n\to \infty} \mathbb{P}_{\delta_x}\biggl(X_{t_n}\biggl( B\biggl(x,\dfrac{y_n -x}{2}\biggr)^c\biggr)>0\biggr)\\[3pt] & =0,\end{align*}

which leads to a contradiction. Moreover, according to Borodin and Salminen [5, page 301],

\begin{equation*} \mathbb{E}_{-\rho}^{x} [{\textrm{e}}^{-s\tau_y }]= {\textrm{e}}^{\rho (x-y)-(y-x)\sqrt{2s+\rho^2}}.\end{equation*}

Thus Chernoff’s inequality reveals that

(3.6) \begin{equation}\mathbb{P}_{-\rho}^{x}( \tau_y <t)\le \inf_{s>0}\,{\textrm{e}}^{st} \mathbb{E}_{-\rho}^{x} [{\textrm{e}}^{-s\tau_y }]= {\textrm{e}}^{\rho (x-y)} \inf_{s>0} \,{\textrm{e}}^{st -(y-x)\sqrt{2s+\rho^2}}\le \exp\biggl\{-\frac{(x-y)^2}{2t}+\rho(x-y)\biggr\}.\end{equation}

Combining (3.5) and (3.6), we get

\begin{equation*}v^y(x,t)\le M(y) \mathbb{P}_{-\rho}^{x}( \tau_y <t)\le M(y) \exp\biggl\{-\frac{(x-y)^2}{2t}+\rho(x-y)\biggr\}.\end{equation*}

Since M(y) is decreasing (and thus bounded) and $\rho<\sqrt{2\alpha}$ , there exists some constant $C_0>0$ such that for any $x\le y$ ,

\begin{equation*} v^y(x,t)\le C_0\exp\biggl\{-\frac{(x-y)^2}{2t}+\rho(x-y)+\biggl(\alpha-\frac{1}{2}\rho^2\biggr) t\biggr\}.\end{equation*}

This gives the desired result.

Recall that, under $\mathbb{P}$ , $\{B_{x,z}^t(r)\}_{0\le r\le t}$ is a Brownian bridge from x to z over time t, with associated expectation $\mathbb{E}$ ; $\tau_0^t(x,z)$ is the notation for the hitting time of the origin by $\{B_{x,z}^t(r)\}_{0\le r\le t}$ . Moreover, we refer the reader to the preliminaries for the constructions of the Brownian bridge. In the following proposition, we will rewrite our expression for $v^y(x,t)$ in terms of the Brownian bridge.

Proposition 3.1. For any $t>1$ , $x>0$ , and $y\ge 0$ ,

\begin{align*} & v^y(x,t) \\ & \quad =\dfrac{{\textrm{e}}^{\rho x -(\rho^2/2-\alpha)(t-1)}}{\sqrt{2\pi(t-1)}} \int_{(0,\infty)}v^y(z,1)\mathbb{E}\bigl[1_{\{\tau_0^{t-1}(x,z)>t-1 \}}\,{\textrm{e}}^{-J_{x,z}^y(0,t-1) } \bigr] \exp\biggl\{-\frac{(x-z)^2}{2(t-1)} -\rho z\biggr\} \,{\textrm{d}} z,\end{align*}

where for all $0 \le a \le b$ and $z \in \mathbb{R}$ ,

(3.7) \begin{equation}J_{x,z}^y(a,b)=\int_{a}^{b}\varphi (v^y(B_{x,z}^{t-1}(r),t-r)) \,{\textrm{d}} r\end{equation}

is a functional of the Brownian bridge.

Proof. It follows from Lemma 3.1 that $\mathbb{E}_{-\rho}^x[M_t^{y}(0,\theta)]=\mathbb{E}_{-\rho}^x[M_t^{y}(t-1,\theta)]$ . Thus, for all $t>1$ , we have

(3.8) \begin{align}v_n^y(x,t,\theta)&=\mathbb{E}_{-\rho}^x \biggl[ v_n^{y}(B((t-1)\land \tau_0),t-((t-1)\land \tau_0),\theta)\notag \\ & \quad\times \exp\biggl\{-\int_{0}^{(t-1)\land \tau_0} \kappa(v_n^{y}(B(r),t-r,\theta))\,{\textrm{d}} r\biggr\}\biggr]\notag \\ & =\mathbb{E}_{-\rho}^x \biggl[ 1_{\{\tau_0>t-1\}} v_n^y( B(t-1), 1 ,\theta)\exp\biggl\{-\int_{0}^{t-1}\kappa(v_n^y(B(r),t-r,\theta)) \,{\textrm{d}} r\biggr\}\biggr],\end{align}

where the last equality follows from that $v_n^{y}(B((t-1)\land \tau_0),t-((t-1)\land \tau_0) =0$ for $ \tau_0 \le t-1$ . By Remark 2.1, $v_n^y( B(t-1), 1 ,\theta) \le{{\alpha}/{(\beta (1-\textrm{e}^{-\alpha}))}}$ . Therefore an application of the dominated convergence theorem shows that, for any $t> 1$ ,

(3.9) \begin{equation}v^y(x,t)=\mathbb{E}_{-\rho}^x \biggl[1_{\{\tau_0>t-1\}} v^y(B(t-1),1)\exp{\biggl\{-\int_{0}^{t-1}\kappa(v^y(B(r),t-r))\,{\textrm{d}} r\biggr\}} \biggr] .\end{equation}

For all $0 \le a \le b$ , define the functional of Brownian motion $\{B(t)\}_{t\ge 0}$ by

\begin{equation*}I^y(a,b)=\int_{a}^{b}\varphi (v^y(B(r),t-r))\,{\textrm{d}} r.\end{equation*}

Since a Brownian motion starting from x and conditioned to be at point z at time t is a Brownian bridge from x to z, equation (3.9) when combined with the change of measure (2.1) allows us to conclude that

This gives the desired result.

Considering $y=\gamma t+\theta$ , the proposition above implies that

\begin{align*}v^{\gamma t+\theta}(x,t)&=\dfrac{{\textrm{e}}^{\rho x -(\rho^2/2-\alpha)(t-1)}}{\sqrt{2\pi(t-1)}} \int_{(-\gamma t-\theta,\infty)}v^{\gamma t+\theta}(z+\gamma t+\theta,1)\\[2pt]&\quad \times \mathbb{E}\Bigl[ 1_{\{\tau_0^{t-1}(x,z+\gamma t+\theta)>t-1 \}}\,{\textrm{e}}^{-J_{x,z+\gamma t+\theta}^{\gamma t+\theta }(0,t-1) } \Bigr] \\[2pt]&\quad \times\exp\biggl\{-\frac{(x-\gamma t-\theta)^2}{2(t-1)} -\rho (z+\gamma t+\theta)\biggr\} \,{\textrm{d}} z.\end{align*}

Thus, in order to investigate the asymptotic behavior of $v^{\gamma t +\theta}(x,t)$ , we need to study the asymptotic property of the expectation in the above equality. Although we are essentially following the strategy of Harris et al. [13], extra effort is required to deal with the complications arising from the absorption. In particular, a carefully chosen construction of the family of Brownian bridges from two independent Brownian motions allows us to give intuitive proofs of the convergence in Proposition 3.2 below. As a first step, the following lemma shows that $J_{x,z+\gamma t+\theta}^{\gamma t+\theta }(0,\tau_0^{t-1}(x,z+\gamma t+\theta)) $ converges to zero almost surely.

Lemma 3.3. For any $z \in \mathbb{R}$ , on the event $\{T_0 <\infty \}$ , we have

\begin{equation*} \int_{0}^{\tau_0^{t-1}(x,z+\gamma t+\theta)}\varphi \bigl(v^{\gamma t +\theta}\bigl(B_{x,z+\gamma t +\theta}^{t-1}(r),t-r\bigr)\bigr) \,{\textrm{d}} r \to 0\end{equation*}

$\mathbb{P}$ -almost surely as $t \to \infty$ .

Proof. Let $s<T_0<\infty$ . From Lemma 2.2, we deduce that there exists some $t_0>1$ such that $s<\tau_0^{t-1}(x,z+\gamma t+\theta)$ for all $t \ge t_0$ . By the construction in (2.3) and Lemma 2.2,

\begin{equation*}B_{x,z+\gamma t +\theta}^{t-1}(r)\le \gamma t + \theta\end{equation*}

$\mathbb{P}$ -almost surely as $t \to \infty$ . Therefore, under the integrability condition (1.4), combining these facts with Lemma 3.2, we see that

\begin{align*}&\lim_{t\to \infty}\,{\textrm{e}}^{(\frac{1}{2}(\gamma+\rho)^2-\alpha)t}\int_{0}^{s}\varphi \bigl(v^{\gamma t +\theta}\bigl(B_{x,z+\gamma t +\theta}^{t-1}(r),t-r\bigr)\bigr) \,{\textrm{d}} r\\[3pt]&\quad \le \lim_{t\to \infty}\,{\textrm{e}}^{(\frac{1}{2}(\gamma+\rho)^2-\alpha)t}\int_{0}^{s} \varphi \biggl( C_0 {\textrm{e}}^{(\alpha -{{\rho^2 }/{2}}) (t-r)} \,{\textrm{e}}^{\rho (B_{x,z+\gamma t +\theta}^{t-1}(r)-\gamma t -\theta)} \\[3pt] &\quad\quad \times \exp\biggl\{-\frac{(B_{x,z+\gamma t +\theta}^{t-1}(r)-\gamma t -\theta)^2}{2(t-r)}\biggr\}\biggr) \,{\textrm{d}} r\\[3pt] &\quad=\lim_{t \to \infty} \,{\textrm{e}}^{(\frac{1}{2}(\gamma+\rho)^2-\alpha)t}\int_{0}^{s} \varphi \bigl(C_0{\textrm{e}}^{(\alpha-\frac{1}{2}(\gamma+\rho)^2)(t-r)}\,{\textrm{e}}^{(W_1(r)+x-\theta)(\gamma + \rho)}\bigr) \,{\textrm{d}} r <\infty{,}\end{align*}

since $\gamma>\sqrt{2 \alpha}-\rho$ and the above holds for all $s<T_0<\infty$ . This gives the desired result.

For any $z \in \mathbb{R}$ , define the functional of Brownian motion $\{W_2(t)\}_{t \ge 0}$ by

(3.10) \begin{equation}I(z)\,{:\!=}\, \int_{0}^{\infty}\varphi(u^{0}(W_2(r)+z-\gamma r,r +1))\,{\textrm{d}} r,\end{equation}

where $u^0(x,t)$ is defined in (2.13). It is clear that I(z) does not depend on $\theta$ and x. Moreover, since I(z) is independent of $\{W_1(t)\}_{t \ge 0}$ , one can see that it is also independent of $\tau_0^t$ and $T_0$ . The convergence of $ J_{x,z+\gamma t+\theta}^{\gamma t+\theta }(0,t-1) $ is shown in the following lemma.

Lemma 3.4. For any $z \in \mathbb{R}$ , on the event $\{T_0 <\infty \}$ , we have

(3.11) \begin{equation}\int_{0}^{t-1}\varphi \bigl(v^{\gamma t+\theta}\bigl(B_{x,z+\gamma t +\theta }^{t-1}(r),t-r\bigr)\bigr) \,{\textrm{d}} r \to I(z)\end{equation}

$\mathbb{P}$ -almost surely as $t \to \infty$ , where I(z) is defined in (3.10). In addition, $I(z) \in (0,\infty)$ $\mathbb{P}$ -almost surely.

Proof. Since the effect of the killing vanishes as the particle’s start position tends to infinity, it follows from (2.3) that

\begin{align*}\int_{\tau_0^{t-1}}^{t-1}\varphi \bigl(v^{\gamma t+\theta }\bigl(B_{x,z+\gamma t +\theta}^{t-1}(r),t-r\bigr)\bigr) \,{\textrm{d}} r& = \int_{\tau_0^{t-1}}^{t-1}\varphi \bigl(v^{\gamma t +\theta }\bigl(\tilde{B}_{z+\gamma t +\theta,0}^{t-1-\tau_0^{t-1}}(t-1-r),t-r\bigr)\bigr) \,{\textrm{d}} r \\[3pt]&= \int_{0}^{t-1-\tau_0^{t-1}}\varphi \bigl(v^{\gamma t +\theta }\bigl(\tilde{B}_{z+\gamma t +\theta,0}^{t-1-\tau_0^{t-1}}(r),r + 1\bigr)\bigr) \,{\textrm{d}} r \\[3pt]& \to \int_{0}^{\infty}\varphi \bigl(u^{0}(W_2(r)+z-\gamma r),r +1\bigr) \,{\textrm{d}} r\end{align*}

$\mathbb{P}$ -almost surely as $t \to \infty$ . This, combined with Lemma 3.3, gives (3.11). Thus it remains to show that I(z) is strictly positive and finite. Since $I(z)>0$ is obvious, it is sufficient to prove that $I(z)<\infty$ $\mathbb{P}$ -almost surely. According to Lemma 2.1, we have

(3.12) \begin{equation}\lim_{r \to \infty} \dfrac{W(r)}{r}=0\end{equation}

$\mathbb{P}$ -almost surely. Using this fact, one can easily verify that for sufficiently large r,

\begin{equation*}B_{z+\gamma t +\theta,x }^{t-1}(r)\le \gamma t+\theta\end{equation*}

$\mathbb{P}$ -almost surely as $t \to \infty$ . Thus it follows from (2.2) and Lemma 3.2 that for sufficiently large r,

(3.13) \begin{align}&v^{\gamma t+\theta}\bigl(B_{z+\gamma t +\theta,x }^{t-1}(r),r +1\bigr)\notag \\[3pt]&\quad \le C_0 {\textrm{e}}^{(\alpha -\frac{1}{2} \rho^2)(r +1) } \,{\textrm{e}}^{\rho (B_{z+\gamma t +\theta,x }^{t-1}(r)-\gamma t-\theta)} \exp\biggl\{-\frac{(\gamma t+\theta-B_{z+\gamma t +\theta,x }^{t-1}(r))^2}{2(r+1)}\biggr\} \notag \\[3pt]& \quad \to C_0 {\textrm{e}}^{(\alpha -\frac{1}{2} \rho^2)(r +1) } \,{\textrm{e}}^{\rho (W(r) +z -\gamma r)} \exp\biggl\{-\frac{(W(r) +z -\gamma r)^2}{2(r+1)}\biggr\}\end{align}

almost surely as $t \to \infty$ . According to (3.12), for any sufficiently small $\epsilon >0$ satisfying $\delta\,{:\!=}\, \frac{1}{2}(\gamma +\rho)^2 -\alpha -\epsilon (\gamma +\rho) >0$ , there exists a random time T such that for any $r>T$ , ${{|W(r)|}/{r}} < \epsilon$ almost surely and (3.13) holds. Thus

\begin{align*}\lim_{t\to \infty} v^{\gamma t +\theta }\bigl(B_{z+\gamma t + \theta ,x}^{t-1}(r),r+1\bigr)&\le C_0 {\textrm{e}}^{\alpha-\frac{1}{2}\rho^2+\gamma^2 } \,{\textrm{e}}^{-\frac{1}{2}(\gamma +\rho)^2 r +\alpha r}\,{\textrm{e}}^{(\gamma +\rho) |W(r)+z|}\\[3pt]& \le C_0 {\textrm{e}}^{\alpha-\frac{1}{2}\rho^2+\gamma^2 } \,{\textrm{e}}^{(\gamma+\rho) |z|} \,{\textrm{e}}^{-\delta r}.\end{align*}

When $r<T$ , note that

\begin{equation*}v^{\gamma t +\theta }\bigl(B_{z+\gamma t + \theta ,x}^{t-1}(r),r+1\bigr)\le \dfrac{\alpha}{\beta (1-{\textrm{e}}^{-\alpha(r +1)})}\le \dfrac{\alpha}{\beta (1-{\textrm{e}}^{-\alpha})}.\end{equation*}

It follows from the integrability condition (1.4) that

\begin{align*}& \lim_{t\to \infty}\int_{0}^{t-1}\varphi \bigl(v^{\gamma t +\theta }\bigl(B_{z+\gamma t + \theta ,x}^{t-1}(r),r+1\bigr)\bigr)\,{\textrm{d}} r \\ & \quad\le \int_{0}^{T} \varphi \biggl(\dfrac{\alpha}{\beta (1-{\textrm{e}}^{-\alpha})} \biggr)\,{\textrm{d}} r+\int_{T}^{\infty} \varphi \bigl( C_0 {\textrm{e}}^{\alpha-\frac{1}{2}\rho^2+\gamma^2 } \,{\textrm{e}}^{(\gamma+\rho) |z|} \,{\textrm{e}}^{-\delta r} \bigr)\,{\textrm{d}} r< \infty\end{align*}

almost surely. According to the time reversal property of the Brownian bridge,

\begin{equation*}\int_{0}^{t-1} v^y\bigl(B_{x,z}^{t-1}(r),t-r\bigr) \,{\textrm{d}} r=\int_{1}^{t} v^y\bigl(B_{z,x}^{t-1}(r-1),r\bigr)\,{\textrm{d}} r=\int_{0}^{t-1} v^y\bigl(B_{z,x}^{t-1}(r),r+1\bigr) \,{\textrm{d}} r\end{equation*}

in the sense of distribution. This gives the desired result.

Immediately from Lemmas 3.3 and 3.4, we have the following proposition.

Proposition 3.2. For any $\gamma >\sqrt{2\alpha}-\rho$ and $x >0$ , $z \in \mathbb{R}$ , we have

\begin{equation*}\mathbb{E}\biggl[1_{\{\tau_0^{t-1}(z+\gamma t+\theta,x)>t-1 \}}\exp\biggl\{-\int_{0}^{t-1}\varphi\bigl(v^{ \gamma t+\theta}\bigl(B_{z+\gamma t+\theta,x}^{t-1}(r),r+1\bigr)\bigr) \,{\textrm{d}} r\biggr\} \biggr] \to (1-{\textrm{e}}^{-2\gamma x})g(z)\end{equation*}

as $t \to \infty$ , where $g(z)\,{:\!=}\, \mathbb{E}({\textrm{e}}^{-I(z)})\in (0,1]$ is a strictly positive function independent of x and $\theta$ .

Proof. It follows from Lemmas 2.2 and 3.4 that

\begin{equation*}\mathbb{E}\biggl[1_{\{\tau_0^{t-1}(z+\gamma t+\theta,x)<t-1 \}}\exp\biggl\{-\int_{0}^{t-1}\varphi\bigl(v^{ \gamma t+\theta}\bigl(B_{z+\gamma t+\theta,x}^{t-1}(r),r+1\bigr)\bigr) \,{\textrm{d}} r\biggr\} \biggr]\to \mathbb{E}\bigl(1_{\{ T_0<\infty\}}\,{\textrm{e}}^{-I(z)}\bigr)\end{equation*}

as $t \to \infty$ . For $t > 1$ , it follows from [15, Problem 8.6] that

\begin{equation*}\mathbb{P}\bigl(\tau_0^{t-1} (x,z)>t-1\bigr)=1-{\textrm{e}}^{-{{2xz}/{(t-1)}}}.\end{equation*}

By the definition of $T_0$ , we have $\mathbb{P}(T_0<\infty)={\textrm{e}}^{-2\gamma x}$ . Note that $T_0$ is independent of I(z). Hence

\begin{align*}& \lim_{t\to \infty}\mathbb{E} \biggl[1_{\{\tau_0^{t-1}(z+\gamma t+\theta,x)>t-1 \}}\exp\biggl\{-\int_{0}^{t-1}\varphi\bigl(v^{ \gamma t+\theta}\bigl(B_{z+\gamma t+\theta,x}^{t-1}(r),r+1\bigr)\bigr) \,{\textrm{d}} r\biggr\} \biggr]\\[3pt]&\quad =\lim_{t\to \infty}\mathbb{E}\biggl[\exp\biggl\{-\int_{0}^{t-1}\varphi\bigl(v^{ \gamma t+\theta}\bigl(B_{z+\gamma t+\theta,x}^{t-1}(r),r+1\bigr)\bigr) \,{\textrm{d}} r\biggr\} \biggr]\\[3pt]&\quad\quad -\lim_{t\to \infty}\mathbb{E}\biggl[1_{\{\tau_0^{t-1}(z+\gamma t+\theta,x)<t-1 \}}\exp\biggl\{-\int_{0}^{t-1}\varphi\bigl(v^{ \gamma t+\theta}\bigl(B_{z+\gamma t+\theta,x}^{t-1}(r),r+1\bigr)\bigr) \,{\textrm{d}} r\biggr\} \biggr]\\[3pt]&\quad =\mathbb{E}({\textrm{e}}^{-I(z)})(1-{\textrm{e}}^{-2\gamma x}).\end{align*}

This, combined with Lemma 3.4, gives the desired result.

Unlike the case of BBM with absorption, we use $v^{{\gamma t+\theta}}(z,1)$ instead of $v^{{\gamma t+\theta}}(z,0)$ to give the integral expression of $v^{\gamma t+\theta}(x,t)$ (due to the singular boundary condition). Moreover, since the effect of the absorption vanishes as the start position tends to infinity, for any $z\in \mathbb{R}$ , $v^{{\gamma t+\theta}}(z+ \gamma t+\theta,1) \to u^{0}(z,1)$ as $t \to \infty$ . Based on the above analysis, below we will give the detailed proof of Theorem 1.1.

Proof. For $t>1$ , it follows from Proposition 3.1 and Lemma 2.3 that

(3.14) \begin{align}v^{\gamma t+\theta}(x,t)&=\dfrac{{\textrm{e}}^{\rho x -(\rho^2/2-\alpha)(t-1)}}{\sqrt{2\pi(t-1)}} \int_{(0,\infty)}v^{{\gamma t+\theta}}(z,1)\notag \\ & \quad \times\mathbb{E}\bigl[1_{\{\tau_0^{t-1}(z,x)>t-1 \}}\,{\textrm{e}}^{-\int_{0}^{t-1}\varphi(v^{ \gamma t+\theta}(B_{z,x}^{t-1}(r),r+1))\,{\textrm{d}} r} \bigr] \exp\biggl\{-\frac{(x-z)^2}{2(t-1)} -\rho z\biggr\} \,{\textrm{d}} z.\end{align}

Define the functional of the Brownian bridge by

\begin{align*}&K(x,t,\gamma t+\theta)\\ & \quad\,{:\!=}\, \int_{(0,\infty)}v^{{\gamma t+\theta}}(z,1)\mathbb{E}\bigl[1_{\{\tau_0^{t-1}(z,x)>t-1 \}}\,{\textrm{e}}^{-\int_{0}^{t-1}\varphi(v^{ \gamma t+\theta}(B_{z,x}^{t-1}(r),r+1))\,{\textrm{d}} r} \bigr] \exp\biggl\{-\frac{(x-z)^2}{2(t-1)} -\rho z\biggr\} \,{\textrm{d}} z.\end{align*}

Then we have

\begin{align*}K(x,t,\gamma t+\theta)&=\int_{(-\gamma t-\theta,\infty)}v^{{\gamma t+\theta}}(z+\gamma t+\theta,1)\,\mathbb{E}\bigl[1_{\{\tau_0^{t-1}(z+\gamma t+\theta,x)>t-1 \}}\\ &\quad\times {\textrm{e}}^{-J_{z+\gamma t+\theta,x}^{\gamma t+\theta}(0,t-1)} \bigr] \exp\biggl\{-\frac{(x-z-\gamma t-\theta)^2}{2(t-1)} -\rho (z+\gamma t+\theta)\biggr\} \,{\textrm{d}} z\\&=\int_{(0,\infty)}v^{{\gamma t+\theta}}(z+\gamma t+\theta,1)\,\mathbb{E}\bigl[1_{\{\tau_0^{t-1}(z+\gamma t+\theta,x)>t-1 \}}\\ &\quad\times {\textrm{e}}^{-J_{z+\gamma t+\theta,x}^{\gamma t+\theta}(0,t-1)} \bigr] \exp\biggl\{-\frac{(x-z-\gamma t-\theta)^2}{2(t-1)} -\rho (z+\gamma t+\theta)\biggr\} \,{\textrm{d}} z\\&\quad+\int_{(-\gamma t-\theta,0)}v^{{\gamma t+\theta}}(z+\gamma t+\theta,1)\,\mathbb{E}\bigl[1_{\{\tau_0^{t-1}(z+\gamma t+\theta,x)>t-1 \}}\\ &\quad\times {\textrm{e}}^{-J_{z+\gamma t+\theta,x}^{\gamma t+\theta}(0,t-1)} \bigr] \exp\biggl\{-\frac{(x-z-\gamma t-\theta)^2}{2(t-1)} -\rho (z+\gamma t+\theta)\biggr\} \,{\textrm{d}} z\\ & \,{=\!:}\, K_1(x,t,\gamma t+\theta) +K_2(x,t,\gamma t+\theta),\end{align*}

where $J_{z+\gamma t +\theta,x}^{\gamma t +\theta}(0,t-1 )$ is defined in (3.7). Next, we will investigate the asymptotic behaviors of $K_1(x,t,\gamma t+\theta)$ and $K_2(x,t,\gamma t+\theta)$ as $t \to \infty$ . According to Lemma 2.7,

\[v^{{\gamma t+\theta}}(z+\gamma t+\theta,1) \le \frac{\alpha}{\beta(1-\textrm{e}^{-\alpha})},\]

and then the dominated convergence theorem gives

\begin{align*}&\lim_{t\to \infty}K_1 (x,t,\gamma t+\theta)\exp\biggl\{\rho(\gamma t +\theta) +\frac{\gamma^2 t^2}{2(t-1)}\biggr\} \,{\textrm{e}}^{(\theta-x)\gamma}\\ & \quad=\int_{(0,\infty)} \lim_{t\to \infty} v^{{\gamma t+\theta}}(z+\gamma t+\theta,1)\,\mathbb{E}\biggl[1_{\{\tau_0^{t-1}(z+\gamma t+\theta,x)>t-1 \}}\\ & \quad\quad\times\exp\biggl\{-\int_{0}^{t-1}\varphi\bigl(v^{ \gamma t+\theta}\bigl(B_{z+\gamma t+\theta,x}^{t-1}(r),r+1\bigr)\bigr)\,{\textrm{d}} r\biggr\} \biggr] \,{\textrm{e}}^{ -(\rho+\gamma) z} \,{\textrm{d}} z.\end{align*}

Since the effect of the absorption vanishes as the start position tends to infinity, it follows from Proposition 3.2 that

\begin{align*}&\lim_{t\to \infty}K_1 (x,t,\gamma t+\theta)\,{\textrm{e}}^{\rho(\gamma t +\theta) +{{\gamma^2 t}/{2}}} \,{\textrm{e}}^{(\theta-x)\gamma}\\[3pt]&\quad =\int_{(0,\infty)} u^{0}(z,1)\lim_{t\to \infty}\mathbb{E}\biggl[1_{\{\tau_0^{t-1}(z+\gamma t+\theta,x)>t-1 \}}\\[3pt]&\quad\quad\times\exp\biggl\{-\int_{0}^{t-1}\varphi(v^{ \gamma t+\theta}(B_{z+\gamma t+\theta,x}^{t-1}(r),r+1))\,{\textrm{d}} r\biggr\} \biggr]\,{\textrm{e}}^{ -(\rho+\gamma) z} \,{\textrm{d}} z\\[3pt]&\quad=(1-{\textrm{e}}^{-2\gamma x}) \int_{(0,\infty)} u^{0}(z,1) g(z)\,{\textrm{e}}^{ -(\rho+\gamma) z} \,{\textrm{d}} z.\end{align*}

Therefore

(3.15) \begin{equation} \lim_{t\to \infty} \dfrac{K_1(x,t,\gamma t+\theta)}{ 1-{\textrm{e}}^{-2\gamma x}} \,{\textrm{e}}^{\rho(\gamma t +\theta) +{{\gamma^2 t}/{2}}} \,{\textrm{e}}^{(\theta-x)\gamma}=\int_{(0,\infty)} u^{0}(z,1) g(z)\,{\textrm{e}}^{ -(\rho+\gamma) z} \,{\textrm{d}} z\,{=\!:}\, C_1.\end{equation}

Because g(z) does not depend on x and $\theta$ , neither does $C_1$ . As for $K_2(x,t,\gamma t+\theta)$ , if $t\ge 2$ , according to Lemma 3.2, we have

\begin{align*}&K_2 (x,t,\gamma t+\theta)\,{\textrm{e}}^{\rho(\gamma t +\theta) +{{\gamma^2 t}/{2}}}\\[3pt]&\quad \le \int_{(-\gamma t-\theta,0)}C_0{\textrm{e}}^{\alpha-{{\rho^2}/{2}} } \exp\biggl\{-\frac{z^2}{2} -\frac{(x-z-\theta)^2}{2(t-1)} +2 \gamma (x-z-\theta) \biggr\} \,{\textrm{d}} z\\[3pt]&\quad \le \int_{(-\infty,0)} C_0 {\textrm{e}}^{\alpha-{{\rho^2}/{2}} } \,{\textrm{e}}^{-{{z^2}/{2}}+ 2 \gamma (x-z-\theta)} \,{\textrm{d}} z<\infty.\end{align*}

By the dominated convergence theorem,

\begin{align*}&\lim_{t\to \infty}K_2(x,t,\gamma t+\theta) \,{\textrm{e}}^{\rho(\gamma t +\theta) +{{\gamma^2 t}/{2}}} = {\textrm{e}}^{\gamma (x-\theta)} (1-{\textrm{e}}^{-2\gamma x})\int_{(-\infty,0)} u^{0}(z,1)g(z) \,{\textrm{e}}^{ -(\rho +\gamma) z} \,{\textrm{d}} z<\infty.\end{align*}

Therefore

\begin{equation*} \lim_{t\to \infty}\dfrac{K_2(x,t,\gamma t+\theta) }{1-{\textrm{e}}^{-2\gamma x}}\,{\textrm{e}}^{\rho(\gamma t +\theta) +{{\gamma^2 t}/{2}}} \,{\textrm{e}}^{(\theta -x) \gamma}= \int_{(-\infty,0)} u^{0}(z,1)g(z) \,{\textrm{e}}^{ -(\rho +\gamma) z} \,{\textrm{d}} z\,{=\!:}\, C_2\end{equation*}

and $C_2$ is independent of x and $\theta$ . This, combined with (3.14) and (3.15), gives

\begin{align*}& \lim_{t\to \infty}v^{\gamma t +\theta}(x,t) \dfrac{\sqrt{2\pi(t-1)}}{{\textrm{e}}^{\rho x -(\rho^2/2-\alpha)(t-1)}}\dfrac{{\textrm{e}}^{\rho(\gamma t +\theta) +{{\gamma^2 t}/{2}}} \,{\textrm{e}}^{(\theta-x)\gamma}}{1-{\textrm{e}}^{-2\gamma x } } \\[2pt]&\quad= \lim_{t\to \infty} ( K_1(x,t,\gamma t+\theta) +K_2 (x,t,\gamma t+\theta)) \dfrac{{\textrm{e}}^{\rho(\gamma t +\theta) +{{\gamma^2 t}/{2}}} \,{\textrm{e}}^{(\theta-x)\gamma}}{1-{\textrm{e}}^{-2\gamma x } }\\[2pt]&\quad=\int_{\mathbb{R}} u^{0}(z,1) g(z)\,{\textrm{e}}^{ -(\rho+\gamma) z} \,{\textrm{d}} z=C_1+C_2.\end{align*}

It follows that

(3.16) \begin{equation}\lim_{t\to \infty}v^{\gamma t +\theta} (x,t) \dfrac{\sqrt{2 \pi t}} {1-{\textrm{e}}^{-2\gamma x }} \,{\textrm{e}}^{\frac{1}{2} (\gamma + \rho)^2 t -\alpha t} \,{\textrm{e}}^{(\gamma +\rho) (\theta -x)}=C,\end{equation}

where C is a positive constant independent of x and $\theta$ . Note that $x-{{x^2}/{2}}<1-{\textrm{e}}^{-x}<x$ , $x>0$ . Thus

\begin{equation*}1-\dfrac{v^y(x,t)}{2}<\dfrac{1-{\textrm{e}}^{-v^y(x,t)}}{v^y(x,t)}<1,\quad t>0,\ x>0.\end{equation*}

Together with (3.16), for any $\gamma>\sqrt{2\alpha}-\rho$ this implies

\begin{equation*}\lim_{t\to \infty}\dfrac{1-{\textrm{e}}^{-v^{\gamma t +\theta}(x,t)}}{v^{\gamma t +\theta}(x,t)}=1.\end{equation*}

This, combined with (3.2) and (3.16), yields that

\begin{align*}& \lim_{t\to \infty} \mathbb{P}_{\delta_x}(R_t > \gamma t +\theta) \dfrac{\sqrt{2\pi t}}{1-{\textrm{e}}^{-2\gamma x }} \,{\textrm{e}}^{\frac{1}{2} (\gamma +\rho)^2 t -\alpha t} \,{\textrm{e}}^{(\gamma +\rho) (\theta -x)} \\[2pt]&\quad = \lim_{t\to \infty}\bigl(1-{\textrm{e}}^{-v^{\gamma t + \theta} (x,t)}\bigr) \dfrac{\sqrt{2\pi t}}{1-{\textrm{e}}^{-2\gamma x }} \,{\textrm{e}}^{\frac{1}{2} (\gamma +\rho)^2 t -\alpha t} \,{\textrm{e}}^{(\gamma +\rho) (\theta -x)}\\[2pt]&\quad = \lim_{t\to \infty} v^{\gamma t + \theta} (x,t) \dfrac{\sqrt{2\pi t}}{1-{\textrm{e}}^{-2\gamma x }} \,{\textrm{e}}^{\frac{1}{2} (\gamma +\rho)^2 t -\alpha t} \,{\textrm{e}}^{(\gamma +\rho) (\theta -x)}=C.\end{align*}

This gives the desired result.

4. Yaglom limit theorem

In this section we will give the proofs of Theorems 1.2 and 1.3. First we prove Theorem 1.2 using ideas similar to those in [7].

Proof. For any $\theta \ge 0$ , according to (1.1) and (2.14), we have

(4.1) \begin{align}\mathbb{P}_{\delta_x} \bigl({\textrm{e}}^{-\theta \langle 1_{( \gamma t ,\infty)},X_t\rangle }\mid R_t > \gamma t \bigr)&=\dfrac{\mathbb{P}_{\delta_x}\bigl({\textrm{e}}^{-\theta \langle 1_{( \gamma t ,\infty)},X_t\rangle }, R_t > \gamma t \bigr)}{\mathbb{P}_{\delta_x}( R_t > \gamma t )}\notag \\[3pt]&=\dfrac{\mathbb{P}_{\delta_x}\bigl({\textrm{e}}^{-\theta \langle 1_{( \gamma t ,\infty)},X_t\rangle }\bigr)-\mathbb{P}_{\delta_x}( R_t \le \gamma t )}{\mathbb{P}_{\delta_x}( R_t > \gamma t )}\notag \\[3pt]&=1-\dfrac{1-\mathbb{P}_{\delta_x}\bigl({\textrm{e}}^{-\theta \langle 1_{(\gamma t ,\infty)},X_t\rangle }\bigr)}{\mathbb{P}_{\delta_x}( R_t > \gamma t )}\notag \\[3pt]&=1-\dfrac{1-{\textrm{e}}^{-v^{\gamma t}(x,t,\theta) }}{1-{\textrm{e}}^{-v^{\gamma t }(x,t)}},\end{align}

where $v^{\gamma t}(x,t,\theta)\,{:\!=}\, \lim_{n \to \infty}v_n^{\gamma t}(x,t,\theta)$ and $v_n^{\gamma t}(x,t,\theta)$ is the unique positive solution to equation (2.11) with initial condition $v_n^{\gamma t}(x,0,\theta)=\theta f_n^{\gamma t} (x)$ . By (3.16), we have

(4.2) \begin{equation}\lim_{t\to \infty}v^{\gamma t } (x,t) \dfrac{\sqrt{2 \pi t}} {1-{\textrm{e}}^{-2\gamma x }} \,{\textrm{e}}^{\frac{1}{2} (\gamma + \rho)^2 t -\alpha t} \,{\textrm{e}}^{-x(\gamma +\rho) }=C.\end{equation}

According to (3.8), for all $t>1$ ,

\begin{equation*}v_n^{\gamma t}(x,t,\theta)=\mathbb{E}_{-\rho}^x \biggl[ 1_{\{\tau_0>t-1\}} v_n^{\gamma t}( B(t-1), 1 ,\theta)\exp\biggl\{-\int_{0}^{t-1}\kappa\bigl(v_n^{\gamma t}(B(r),t-r,\theta)\bigr) \,{\textrm{d}} r\biggr\}\biggr].\end{equation*}

An application of the dominated convergence theorem gives

(4.3) \begin{equation}v^{\gamma t}(x,t,\theta)=\mathbb{E}_{-\rho}^x \biggl[ 1_{\{\tau_0>t-1\}} v^{\gamma t}( B(t-1), 1 ,\theta)\exp\biggl\{-\int_{0}^{t-1}\kappa(v^{\gamma t}(B(r),t-r,\theta)) \,{\textrm{d}} r\biggr\}\biggr].\end{equation}

Recall that, for any $y\ge 0$ , $M_t^{y}(s,\theta)$ is a uniformly integrable $\mathbb{P}_{-\rho}^x $ -martingale on [0, t]. Thus $\mathbb{E}_{-\rho}^x[M_t^{\gamma t}(0,\theta)]=\mathbb{E}_{-\rho}^x[M_t^{\gamma t}(t,\theta)]$ . Then we have

\begin{equation*}v_n^{\gamma t}(x,t,\theta)=\mathbb{E}_{-\rho}^x \biggl[1_{\{\tau_0>t \}}\theta f_n^{\gamma t}(B(t))\exp\biggl\{-\int_{0}^{t}\kappa\bigl(v_n^{\gamma t }(B(r),t-r, \theta)\bigr)\,{\textrm{d}} r)\biggr\}\biggr],\quad t>0.\end{equation*}

Now taking $n \to \infty$ , with the help of both monotone and dominated convergence we have

(4.4) \begin{equation}v^{\gamma t}(x,t,\theta)=\mathbb{E}_{-\rho}^x \biggl[1_{\{\tau_0>t \}}\theta 1_{(\gamma t ,\infty) }(B(t))\exp{\biggl\{-\int_{0}^{t}\kappa(v^{\gamma t }(B(r),t-r, \theta))\,{\textrm{d}} r\biggr\}}\biggr],\quad t>0.\end{equation}

Define the functional of the Brownian bridge $\{B_{x,z}^t(r)\}_{0\le r\le t}$ by

\begin{equation*}J_{x,z}^{y}(a,b,\theta)=\int_{a}^{b}\varphi \bigl(v^{y}\bigl(B_{x,z}^{t-1}(r),t-r,\theta\bigr)\bigr) \,{\textrm{d}} r,\quad 0\le a\le b.\end{equation*}

By (4.3), it follows from an analysis similar to the proof of Theorem 1.1 that

(4.5) \begin{equation}\lim_{t\to \infty}v^{\gamma t}(x,t,\theta)\dfrac{\sqrt{2 \pi t}}{1-{\textrm{e}}^{-2\gamma x}} \,{\textrm{e}}^{(\frac{1}{2}(\gamma +\rho )^2-\alpha)t } \,{\textrm{e}}^{-(\gamma+\rho) x} =\int_{\mathbb{R}}u^0(z,1,\theta)g^{\theta}(z)\,{\textrm{e}}^{-(\rho +\gamma)z}\,{\textrm{d}} z,\end{equation}

where

\[g^{\theta}(z)\,{:\!=}\, \lim_{t\to \infty}\mathbb{E}\bigl[ {\textrm{e}}^{-J_{z+\gamma t,x}^{\gamma t}(0,t-1,\theta) }\bigr]\]

exists and $g^{\theta}(z) \in (0,1]$ for all $z \in \mathbb{R}$ . Furthermore, according to (4.4),

(4.6) \begin{equation}\lim_{t\to \infty}v^{\gamma t}(x,t,\theta)\dfrac{\sqrt{2 \pi t}}{1-{\textrm{e}}^{-2\gamma x}} \,{\textrm{e}}^{(\frac{1}{2}(\gamma +\rho )^2-\alpha)t } \,{\textrm{e}}^{-(\gamma+\rho) x} =\int_{(0,\infty)}\theta h^{\theta}(z)\,{\textrm{e}}^{-(\rho +\gamma)z}\,{\textrm{d}} z,\end{equation}

where

\[h^{\theta}(z)=\lim_{t\to \infty}\mathbb{E}\bigl[ {\textrm{e}}^{-J_{x,z+\gamma t}^{\gamma t}(0,t,\theta) }\bigr]\]

exists. Note that $\lim_{\theta \to 0} h^{\theta}(z)=1$ . Define $F\,{:}\, (0,\infty)\to [0,1]$ by

\begin{equation*}F(\theta)\,{:\!=}\, \lim_{t\to \infty}\mathbb{P}_{\delta_x}\bigl({\textrm{e}}^{-\theta \langle 1_{( \gamma t ,\infty)}, X_t\rangle } \mid R_t > \gamma t \bigr).\end{equation*}

Using ideas similar to the proof of Theorem 3 of [7], we have to prove that F is the Laplace transform of some distribution on $(0,\infty)$ . This is equivalent to proving that

\begin{equation*}\lim_{\theta \to \infty}F(\theta)=0 \quad \text{and} \quad \lim_{\theta \to 0}F(\theta)=1.\end{equation*}

Equation (4.1) combined with (4.2) and (4.5) gives

\begin{equation*}F(\theta)=1-\lim_{t\to \infty}\dfrac{v^{\gamma t}(x,t,\theta) }{v^{\gamma t }(x,t)}.\end{equation*}

Therefore it is sufficient to prove that

(4.7) \begin{equation}\lim_{\theta \to \infty}\lim_{t\to \infty}\dfrac{v^{\gamma t}(x,t,\theta) }{v^{\gamma t }(x,t)}=1\end{equation}

and

(4.8) \begin{equation}\lim_{\theta \to 0}\lim_{t\to \infty}\dfrac{v^{\gamma t}(x,t,\theta) }{v^{\gamma t }(x,t)}=0.\end{equation}

Since $v^{\gamma t}(x,t) =\lim_{\theta \to \infty}v^{\gamma t}(x,t,\theta) $ , (4.7) is clear. Moreover, it follows from (4.4) that $\lim_{\theta \to 0}v^{\gamma t}(x,t,\theta)=0$ . This, combined with (4.6), yields (4.8). It remains to show that the limit law has a finite expectation. It is sufficient to prove that

\[\lim_{\theta \to 0}\lim_{t\to \infty}\frac{v^{\gamma t}(x,t,\theta) }{\theta v^{\gamma t }(x,t)}\]

exists and is bounded. By (4.2) and (4.6),

\begin{equation*}\lim_{t\to \infty}\dfrac{v^{\gamma t}(x,t,\theta) }{\theta v^{\gamma t }(x,t)} =\dfrac{1}{C}\int_{0}^{\infty} h^{\theta}(z) \,{\textrm{e}}^{-(\rho +\gamma) z}\,{\textrm{d}} z.\end{equation*}

It follows that

\begin{equation*}\lim_{\theta \to 0}\lim_{t\to \infty}\dfrac{v^{\gamma t}(x,t,\theta) }{\theta v^{\gamma t }(x,t)}=\dfrac{1}{C}\int_{0}^{\infty}\,{\textrm{e}}^{-(\rho +\gamma) z}\,{\textrm{d}} z=\dfrac{1}{C (\rho +\gamma)}.\end{equation*}

This gives the desired result.

For any $f \in C_b^+(0,\infty)$ and $x>0$ , define

\begin{equation*} \tilde{f}(x)= \begin{cases} 0, & 0< x \le \gamma t,\\ \\[-8pt]f(x-\gamma t),& x>\gamma t,\end{cases}\end{equation*}

and $l(x) ={{a}/{(1+ x^2)}}$ , where $a= \pi^{-1}$ and $\int_{0}^{\infty} l(x) \,{\textrm{d}} x=1$ . It follows that $l \in C_0^2(0,\infty)^+$ . For each $n \ge 1$ , define $l_n(x) = n l ( nx )$ and $ \tilde{f}_n(x) =(l_n *\tilde{f} )(x)$ , where $l_n *\tilde{f} $ stands for the convolution of $l_n$ and $\tilde{f}$ . It is easy to verify that $ \tilde{f}_n \in C_0^2(0,\infty)^+$ and $\lim_{n \to \infty} \tilde{f}_n(x)=\tilde{f}(x)$ , $x>0$ . The random measure $Y_t$ is characterized by the Laplace functional

\begin{align*}\mathbb{P}_{\delta_x}\bigl( {\textrm{e}}^{-\langle f,Y_t \rangle} \bigr)&=\mathbb{P}_{\delta_{x}}\bigl({\textrm{e}}^{-\int_{\gamma t}^{\infty} f(z-\gamma t)X_t({\textrm{d}} z)}\bigr)\\[3pt]&=\mathbb{P}_{\delta_{x}}\bigl( {\textrm{e}}^{-\langle \tilde{f},X_t \rangle} \bigr)\\[3pt]&=\lim_{n \to \infty}\mathbb{P}_{\delta_{x}}\bigl( {\textrm{e}}^{-\langle \tilde{f}_n,X_t \rangle} \bigr)\\[3pt]&=\lim_{n\to \infty} \,{\textrm{e}}^{- v_{\tilde{f}_n} (x,t)}.\end{align*}

Therefore

\begin{equation*}v_{\tilde{f}}(x,t)\,{:\!=}\, \lim_{n \to \infty}v_{\tilde{f}_n}(x,t)\end{equation*}

exists, where $v_{\tilde{f}_n}(x,t)$ is the unique positive solution to the equation

\begin{equation*}\left \{\begin{aligned}& \dfrac{\partial}{\partial t} v_{\tilde{f}_n} (x,t)=\dfrac{1}{2}\dfrac{\partial^2}{\partial x^2} v_{\tilde{f}_n} (x,t)-\rho \dfrac{\partial}{\partial x} v_{\tilde{f}_n} (x,t)-\psi(v_{\tilde{f}_n} (x,t)),\\ \\[-8pt] & v_{\tilde{f}_n} (0+,t)=0,\quad v_{\tilde{f}_n}(x,0)=\tilde{f}_n(x),\quad t\ge 0,\ x>0.\end{aligned}\right.\end{equation*}

Next, we will give the proof of Theorem 1.3 using an argument similar to that used in the proof of Theorem 1.1.

Proof. For any $t\ge 0$ , define

\begin{equation*}N_t^{\tilde{f}_n}(s)\,{:\!=}\, v_{\tilde{f}_n}(B(s\land \tau_0),t-(s\land \tau_0))\exp\biggl\{-\int_{0}^{s\land \tau_0} \kappa(v_{\tilde{f}_n}(B(r),t-r))\,{\textrm{d}} r\biggr\},\quad s \in [0,t].\end{equation*}

A similar analysis to $M_t^y(s,\theta)$ tells us that $ N_t^{\tilde{f}_n}(s)$ is a uniformly integrable $\mathbb{P}_{-\rho}^x$ -martingale on [0, t]. Therefore

\begin{equation*}v_{\tilde{f}_n}(x,t)=\mathbb{E}_{-\rho}^x \biggl[1_{\{ \tau_0>t\}}\tilde{f}_n(B(t))\exp\biggl\{-\int_{0}^{t}\kappa(v_{\tilde{f}_n}(B(r),t-r)) \,{\textrm{d}} r\biggr\}\biggr],\quad x>0,\ t \ge 0.\end{equation*}

Since for all $n\ge 1$ , $\tilde{f}_n \le M_f$ , where $M_f \,{:\!=}\, \sup_{x>0} f(x)$ , an application of the dominated convergence theorem shows that

\begin{equation*}v_{\tilde{f}}(x,t)=\mathbb{E}_{-\rho}^x \biggl[1_{\{ \tau_0>t\}}\tilde{f}(B(t))\exp\biggl\{-\int_{0}^{t}\kappa(v_{\tilde{f}}(B(r),t-r)) \,{\textrm{d}} r\biggr\} \biggr],\quad x>0,\ t \ge 0.\end{equation*}

It follows from (2.1) that

(4.9) \begin{align}v_{\tilde{f}}(x,t)&={\textrm{e}}^{\alpha t} \mathbb{E}_{-\rho}^x \biggl[1_{\{\tau_0>t\}}\tilde{f}(B(t))\exp\biggl\{-\int_{0}^{t}\varphi(v_{\tilde{f}}(B(r),t-r)) \,{\textrm{d}} r\biggr\}\biggr] \notag \\ & ={\textrm{e}}^{\alpha t} \int_{\gamma t}^{\infty} f(z-\gamma t)\,\mathbb{E}\biggl[ 1_{\{\tau_0^{t }(x,z)>t\}} \notag \\ & \quad\times\exp\biggl\{-\int_{0}^{t}\varphi\bigl(v_{\tilde{f}}\bigl(B_{x,z}^t(r),t-r\bigr)\bigr) \,{\textrm{d}} r\biggr\} \biggr] \mathbb{P}_{-\rho}^x[B(t)\in {\textrm{d}} z] \notag \\&={\textrm{e}}^{\alpha t} \int_{0}^{\infty} f(z)\,\mathbb{E}\biggl[1_{\{\tau_0^{t }(x,z+\gamma t)>t \}} \notag \\ & \quad\times\exp\biggr\{-\int_{0}^{t}\varphi\bigl(v_{\tilde{f}}\bigl(B_{x,z+\gamma t}^t(r),t-r\bigr)\bigr) \,{\textrm{d}} r\biggr\}\biggr] \dfrac{1}{\sqrt{2 \pi t}} \exp\biggl\{-\frac{(x-z-\gamma t-\rho t)^2}{ 2t}\biggr\} \,{\textrm{d}} z \notag \\&=\dfrac{{\textrm{e}}^{\alpha t-\frac{1}{2}(\rho +\gamma)^2 t} }{\sqrt{2 \pi t}} \,{\textrm{e}}^{(\rho +\gamma)x}\int_{0}^{\infty} f(z)\,\mathbb{E}\biggl[1_{\{\tau_0^{t }(x,z+\gamma t)>t \}} \notag \\ & \quad\times \exp\biggr\{-\int_{0}^{t}\varphi\bigl(v_{\tilde{f}}\bigl(B_{x,z+\gamma t}^t(r),t-r\bigr)\bigr) \,{\textrm{d}} r\biggr\} \biggr] \exp\biggl\{-\frac{(x-z)^2}{ 2t} -(\rho+\gamma)z\biggr\} \,{\textrm{d}} z.\end{align}

Hence

(4.10) \begin{align}&v_{\tilde{f}}(x,t) \sqrt{2 \pi t} \,{\textrm{e}}^{\frac{1}{2}(\rho +\gamma)^2 t - \alpha t} \,{\textrm{e}}^{-(\rho +\gamma)x} \notag \\[3pt]&\quad=\int_{0}^{\infty} f(z)\,\mathbb{E}\biggl[ 1_{\{\tau_0^{t }(x,z+\gamma t)>t \}} \notag \\[3pt]&\quad\quad\times\exp\biggl\{-\int_{0}^{t}\varphi\bigl(v_{\tilde{f}}\bigl(B_{x,z+\gamma t}^t(r),t-r\bigr)\bigr) \,{\textrm{d}} r\biggr\}\biggr] \exp\biggl\{-\frac{(x-z)^2}{ 2t} -(\rho+\gamma)z\biggr\} \,{\textrm{d}} z.\end{align}

Now as $t \to \infty$ ,

\[\exp\biggl\{-\frac{(x-z)^2}{ 2t}\biggr\} \to 1,\]

so it is sufficient to show that, as $t \to \infty$ ,

(4.11) \begin{equation}\mathbb{E}\biggl[1_{\{\tau_0^{t }(x,z+\gamma t)>t \}}\exp\biggl\{-\int_{0}^{t}\varphi\bigl(v_{\tilde{f}}\bigl(B_{x,z+\gamma t}^t(r),t-r\bigr)\bigr) \,{\textrm{d}} r\biggr\} \biggr]\to h_f(z) (1- {\textrm{e}}^{-2\gamma x})\end{equation}

for some function $h_f\,{:}\, (0,\infty) \to (0,1]$ , since then by dominated convergence the expression given on the right-hand side of (4.10) tends to

\[ (1- {\textrm{e}}^{-2\gamma x}) \int_{0}^{\infty} f(z) h_f(z) \,{\textrm{e}}^{-(\rho+\gamma)z} \,{\textrm{d}} z \]

as $t \to \infty$ . Note that the rigorous proof of (4.11) is given in Proposition 4.1 below. Assume that (4.11) holds, then we have

(4.12) \begin{equation}\lim_{t \to \infty} \dfrac{v_{\tilde{f}}(x,t) }{ 1- {\textrm{e}}^{-2\gamma x} } \sqrt{2 \pi t} \,{\textrm{e}}^{\frac{1}{2}(\rho +\gamma)^2 t - \alpha t} \,{\textrm{e}}^{-(\rho +\gamma)x}=\int_{0}^{\infty} f(z)h_f(z)\,{\textrm{e}}^{-(\rho+\gamma)z} \,{\textrm{d}} z.\end{equation}

For any $ f \in C_b^+( 0,\infty)$ , we have

\begin{align*}\mathbb{P}_{\delta_x}\bigl({\textrm{e}}^{-\langle f, Y_t\rangle } \mid R_t > \gamma t \bigr)&=\dfrac{ \mathbb{P}_{\delta_x}\bigl( {\textrm{e}}^{- \langle f, Y_t \rangle } \bigr)-\mathbb{P}_{\delta_x}( R_t \le \gamma t )}{\mathbb{P}_{\delta_x}( R_t > \gamma t )}\\[3pt]&=1-\dfrac{1-\mathbb{P}_{\delta_x}\bigl( {\textrm{e}}^{- \langle f, Y_t \rangle }\bigr)}{\mathbb{P}_{\delta_x}( R_t > \gamma t )}\\[3pt]&=1-\dfrac{1-{\textrm{e}}^{-v_{\tilde{f}}(x,t ) }}{1-{\textrm{e}}^{-v^{\gamma t }(x,t)}}.\end{align*}

This, combined with (4.2) and (4.12), yields that

\begin{equation*}\lim_{t \to \infty}\mathbb{P}_{\delta_x}\bigl({\textrm{e}}^{- \langle f, Y_t \rangle }\mid R_t > \gamma t \bigr)=1- \lim_{t \to \infty} \dfrac{ v_{\tilde{f}}(x,t ) }{v^{\gamma t }(x,t)}= 1- C^{-1} \int_{0}^{\infty} f(z) h_f(z) \,{\textrm{e}}^{-(\rho+\gamma)z} \,{\textrm{d}} z.\end{equation*}

This gives the desired result.

The following proposition completes the proof of Theorem 1.3. In the remainder of this section we will give a rigorous proof. For any $ f \in C_b^+( 0,\infty)$ , define the functional of Brownian motion $\{W_2(t)\}_{t\ge 0}$ by

(4.13) \begin{equation}J_f(z)\,{:\!=}\, \int_{0}^{\infty}\varphi(v_f(W_2(r) +z -\gamma r, r)) \,{\textrm{d}} r,\end{equation}

where $v_f$ is the unique positive solution to equation (1.2). Note that $J_f(z)$ is independent of $W_1$ (hence also of $\tau_0^t$ and $T_0$ ).

Proposition 4.1. For any $z>0$ , we have

\begin{equation*}\lim_{t \to \infty} \mathbb{E}\biggl[ 1_{\{\tau_0^{t }(x,z+\gamma t)>t \}}\exp\biggl\{-\int_{0}^{t} \varphi(v_{\tilde{f}}(B_{x,z+\gamma t}^t(r),t-r)) \,{\textrm{d}} r\biggr\} \biggr]= h_f(z) (1- {\textrm{e}}^{-2 \gamma x}),\end{equation*}

where $h_f(z)\,{:\!=}\, \mathbb{E} ( {\textrm{e}}^{-J_f(z)} ) \in (0,1]$ .

To prove the proposition, we need the following lemma, whose proof is similar to that of Lemmas 3.3 and 3.4.

Lemma 4.1. For any $z>0$ , on the event $ \{T_0<\infty\} $ , we have

(4.14) \begin{equation}\lim_{t \to \infty} \int_{0}^{t} \varphi(v_{\tilde{f}}(B_{x,z+\gamma t}^t(r),t-r)) \,{\textrm{d}} r=J_f(z),\quad \text{$\mathbb{P}$-}a.s.,\end{equation}

where $J_f$ is defined in (4.13).

Proof. Immediately from (4.9), we see that

(4.15) \begin{align}v_{\tilde{f}}(x,t)& \le \dfrac{{\textrm{e}}^{\alpha t-\frac{1}{2}(\rho +\gamma)^2 t} }{\sqrt{2 \pi t}} \,{\textrm{e}}^{(\rho +\gamma)x}\int_{0}^{\infty} M_f \exp\biggl\{-\frac{(x-z)^2}{ 2t}\biggr\} \,{\textrm{d}} z \notag \\[3pt] & \le M_f \,{\textrm{e}}^{\alpha t-\frac{1}{2}(\rho +\gamma)^2 t} \,{\textrm{e}}^{(\rho +\gamma)x} , \quad t>0,\ x>0.\end{align}

Let $s<T_0<\infty$ . It follows from Lemma 2.2 that there exists some $t_0$ such that $s<\tau_0^{t }(x,z+\gamma t)$ for all $t \ge t_0$ . Based on the integrability condition (1.4) and (4.15), we have

\begin{align*}& \lim_{t\to \infty} \,{\textrm{e}}^{ (\frac{1}{2}(\rho +\gamma)^2 -\alpha) t} \int_{0}^{s} \varphi\bigl(v_{\tilde{f}}\bigl( B_{x,z+\gamma t}^t(r),t-r\bigr)\bigr) \,{\textrm{d}} r\\[3pt]& \quad\le \lim_{t\to \infty} \,{\textrm{e}}^{ (\frac{1}{2}(\rho +\gamma)^2 -\alpha) t} \int_{0}^{s} \varphi \bigl( M_f {\textrm{e}}^{ (\alpha -\frac{1}{2}(\rho +\gamma)^2) (t-r) } \,{\textrm{e}}^{(\rho +\gamma) B_{x,z+\gamma t}^t(r)} \bigr) \,{\textrm{d}} r\\[3pt]& \quad = \lim_{t\to \infty} \,{\textrm{e}}^{ (\frac{1}{2}(\rho +\gamma)^2 -\alpha) t} \int_{0}^{s} \varphi \bigl( M_f {\textrm{e}}^{ (\alpha -\frac{1}{2}(\rho +\gamma)^2) (t-r) } \,{\textrm{e}}^{(\rho +\gamma) ( W(r) +x +\gamma r) }\bigr) \,{\textrm{d}} r <\infty.\end{align*}

Since $\gamma >\sqrt{2 \alpha}-\rho$ and the above holds for all $s<T_0<\infty$ , it gives that

(4.16) \begin{equation}\int_{0}^{ \tau_0^{t }(x,z+\gamma t) } \varphi\bigl(v_{\tilde{f}}\bigl( B_{x,z+\gamma t}^t(r),t-r\bigr)\bigr) \,{\textrm{d}} r \to 0\end{equation}

$\mathbb{P}$ -almost surely as $t \to \infty$ . For any $f \in C_b^+( 0,\infty)$ , there exists a sequence of functions $\{f_n\}_{n\ge 1}$ in $C_0^2(0,\infty)^+$ which is pointwise increasing and satisfies $f_n \uparrow f$ as $n \to \infty$ . Note that $v_{f_n}$ is the unique positive solution to equation (1.3) with boundary condition $ v_{f_n} (x,0)=f_n (x)$ . Since $ N_t^{f_n}(s)$ is a uniformly integrable martingale on [0, t], then for any $x,\, t>0$ and $n\ge 1$ , we have

\begin{equation*}v_{f_n}(x,t)= {\textrm{e}}^{\alpha t} \mathbb{E}_{-\rho}^x \biggl[ 1_{\{\tau_0>t\}}f_n(B(t))\exp\biggl\{-\int_{0}^{t}\varphi(v_{f_n}(B(r),t-r)) \,{\textrm{d}} r\biggr\}\biggr].\end{equation*}

Thus $v_{f_n}(x,t)\le M_f{\textrm{e}}^{\alpha t}$ . An application of the dominated convergence theorem gives

(4.17) \begin{equation}v_{f}(x,t)= {\textrm{e}}^{\alpha t} \mathbb{E}_{-\rho}^x \biggl[ 1_{\{ \tau_0>t\}}f(B(t))\exp\biggl\{-\int_{0}^{t}\varphi(v_{f}(B(r),t-r)) \,{\textrm{d}} r\biggr\}\biggr], \quad x,\ t>0.\end{equation}

It follows from (2.1) that

\begin{align*}v_f(x,t)& = \dfrac{{\textrm{e}}^{\alpha t} }{\sqrt{2 \pi t}} \int_{0}^{\infty} f(z) \,\mathbb{E} \biggl[1_{\{\tau_0^t(x,z )>t \}} \exp\biggl\{-\int_{0}^{t}\varphi\bigl(v_f\bigl(B_{x,z}^{t}(r),t-r\bigr)\bigr) \,{\textrm{d}} r\biggr\}\Biggr] \\[3pt]&\quad\times \exp\biggl\{-\frac{(x-z-\rho t)^2}{2 t}\biggr\} \,{\textrm{d}} z.\end{align*}

This, combined with (4.9), gives

(4.18) \begin{equation}v_{\tilde{f}}(x+\gamma t ,t) = v_f(x,t),\quad x \ge 0 ,\ t>0.\end{equation}

As for the integral on $( \tau_0^{t }(x,z+\gamma t) , t )$ , by (4.18) and the construction of the Brownian bridge in (2.3), we have

\begin{align*}& \lim_{t \to \infty} \int_{\tau_0^{t }(x,z+\gamma t)}^{t} \varphi\bigl( v_{\tilde{f}}\bigl( B_{x,z+\gamma t}^t(r),t-r\bigr)\bigr) \,{\textrm{d}} r \\[3pt]&\quad = \lim_{t \to \infty} \int_{\tau_0^{t }(x,z+\gamma t)}^{t}\varphi \Bigl(v_{\tilde{f}} \Bigl(\tilde{B}_{z+\gamma t , 0}^{t-\tau_0^{t }(x,z+\gamma t)}(t-r),t-r\Bigr)\Bigr) \,{\textrm{d}} r \\[3pt]&\quad = \lim_{t \to \infty} \int_{0 }^{t-\tau_0^{t }(x,z+\gamma t) }\varphi \Bigl(v_{\tilde{f}}\Bigl(\tilde{B}_{z+\gamma t , 0}^{t-\tau_0^{t }(x,z+\gamma t)}( r), r\Bigr)\Bigr) \,{\textrm{d}} r \\[3pt]&\quad = \int_{0 }^{\infty} \varphi ( v_f (W_2(r) +z -\gamma r, r)) \,{\textrm{d}} r\end{align*}

$\mathbb{P}$ -almost surely. This, combined with (4.16), gives (4.14). Since $J_f(z)>0$ is obvious, it remains to show that $J_f(z)<\infty$ . By (4.17) and Chernoff’s inequality, for any $x>0$ and $t>0$ ,

\begin{align*}v_f(x,t)\le M_f {\textrm{e}}^{\alpha t} \mathbb{P}_{-\rho}^x [\tau_0>t ]\le M_f \exp\biggl\{\alpha t -\frac{1}{2} \rho^2 t +\rho x -\frac{x^2}{2 t }\biggr\}.\end{align*}

Hence, for any $r>0$ ,

\begin{align*}v_f ( W_2(r) +z -\gamma r, r)& \le M_f \exp\biggl\{\alpha r-\frac{1}{2} \rho^2 r +\rho (W_2(r) +z -\gamma r) -\frac{ (W_2(r) +z -\gamma r)^2}{2 r }\biggr\} \\[3pt]& \le M_f {\textrm{e}}^{ (\alpha -\frac{1}{2}(\rho +\gamma)^2 ) r } \,{\textrm{e}}^{(\rho + \gamma ) (W_2(r) + z )}.\end{align*}

According to Lemma 2.1, for any sufficiently small $\epsilon >0$ satisfying

\[\delta\,{:\!=}\, \dfrac{1}{2}(\gamma +\rho)^2 -\alpha -\epsilon (\gamma +\rho) >0,\]

there exists a random time T such that for any $r>T$ , $\mathbb{P}$ -almost surely ${{W_2(r)}/{r}} < \epsilon$ . Thus

\begin{equation*}v_f ( W_2(r) +z -\gamma r, r)\le M_f {\textrm{e}}^{(\rho + \gamma ) z} \,{\textrm{e}}^{ -\delta r}\end{equation*}

$\mathbb{P}$ -almost surely. When $r<T$ , note that

\begin{equation*}v_f ( W_2(r) +z -\gamma r, r)\le M_f {\textrm{e}}^{ \alpha r}.\end{equation*}

It follows from the integrability condition (1.4) that

\begin{equation*}\int_{0 }^{\infty} \varphi ( v_f (W_2(r) +z -\gamma r, r)) \,{\textrm{d}} r\le \int_{0}^{T} \varphi ( M_f {\textrm{e}}^{ \alpha r} )\,{\textrm{d}} r+\int_{T}^{\infty} \varphi \bigl( M_f {\textrm{e}}^{(\rho + \gamma ) z} \,{\textrm{e}}^{ -\delta r} \bigr)\,{\textrm{d}} r< \infty\end{equation*}

$\mathbb{P}$ -almost surely. This gives the desired result.

Now, using arguments similar to that used in the proof of Proposition 3.2, we give the proof of Proposition 4.1.

Proof. It follows immediately from Lemmas 2.2 and 4.1 that

\begin{equation*}\lim_{t \to \infty} \mathbb{E}\biggl[ 1_{\{ \tau_0^{t }(x,z+\gamma t)<t \}}\exp\biggl\{-\int_{0}^{t} \varphi\bigl(v_{\tilde{f}}\bigl(B_{x,z+\gamma t}^t(r),t-r\bigr)\bigr) \,{\textrm{d}} r\biggr\} \biggr]=\mathbb{E} \bigl( 1_{ \{T_0<\infty\}}\,{\textrm{e}}^{-J_f(z)} \bigr).\end{equation*}

Note that $T _0$ is independent of $J_f(z)$ . Therefore

\begin{align*}&\lim_{t \to \infty} \mathbb{E}\biggl[ 1_{\{\tau_0^{t }(x,z+\gamma t)>t\}}\exp\biggl\{-\int_{0}^{t} \varphi\bigl(v_{\tilde{f}}\bigl(B_{x,z+\gamma t}^t(r),t-r\bigr)\bigr) \,{\textrm{d}} r\biggr\} \biggr] \\[3pt]&\quad =\lim_{t \to \infty} \mathbb{E}\biggl[\exp\biggl\{-\int_{0}^{t}\varphi\bigl(v_{\tilde{f}}\bigl(B_{x,z+\gamma t}^t(r),t-r\bigr)\bigr) \,{\textrm{d}} r\biggr\} \biggr] \\[3pt]&\quad\quad - \lim_{t \to \infty} \mathbb{E}\biggl[ 1_{\{\tau_0^{t }(x,z+\gamma t)<t\}}\exp\biggl\{-\int_{0}^{t} \varphi\bigl(v_{\tilde{f}}\bigl(B_{x,z+\gamma t}^t(r),t-r\bigr)\bigr) \,{\textrm{d}} r\biggr\} \biggr] \\[3pt]&\quad = h_f(z) \bigl(1- {\textrm{e}}^{-2 \gamma x}\bigr),\end{align*}

where for any $z>0$ ,

\[h_f(z)= \mathbb{E} \bigl( {\textrm{e}}^{-J_f(z)}\bigr) \in (0,1]. \]

This gives the desired result.