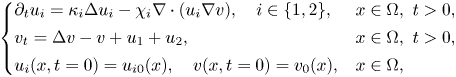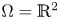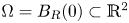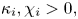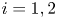On the global existence of solutions to chemotaxis system for two populations in dimension two

Abstract

We consider the global existence for the following fully parabolic chemotaxis system with two populations

\[\left\{ \begin{array}{@{}ll} \partial_tu_i=\kappa_i\Delta u_i-\chi_i\nabla\cdot(u_i\nabla v),\quad i\in\{1,2\}, & x\in\Omega,\ t>0, \\ v_t=\Delta v-v+u_1+u_2, & x\in\Omega,\ t>0,\\ u_i(x,t=0)=u_{i0}(x),\quad v(x,t=0)=v_0(x), & x\in\Omega, \end{array} \right. \]

where $\Omega =\mathbb {R}^2$ or $\Omega =B_R(0)\subset \mathbb {R}^2$ supplemented with homogeneous Neumann boundary conditions, $\kappa _i,\chi _i>0,$ $i=1,2$. The global existence remains open for the fully parabolic case as far as the author knows, while the existence of global solution was known for the parabolic-elliptic reduction with the second equation replaced by $0=\Delta v-v+u_1+u_2$ or $0=\Delta v+u_1+u_2$. In this paper, we prove that there exists a global solution if the initial masses satisfy the certain sub-criticality condition. The proof is based on a version of the Moser–Trudinger type inequality for system in two dimensions.

1. Introduction

Chemotaxis is a common phenomenon in mathematical biology. Since Keller and Segel [14] suggested a mathematical chemotaxis model for chemotactic aggregation of the cellular slime mold Dictyostelium discoideum in the early 1970s, a large number of theoretical (mathematical) models, including the chemotactic movement of multi populations along with multiple stimuli in the environment, have been proposed by many researchers (see [12]). In this paper, a chemotaxis system for two populations interaction via the same chemical signal will be considered as follows:

(1.1)\begin{equation} \left\{\begin{array}{@{}ll} \partial_tu_1=\kappa_1\Delta u_1-\chi_1\nabla\cdot(u_1\nabla v), & x\in\Omega,\ t>0,\\ \partial_tu_2=\kappa_2\Delta u_2-\chi_2\nabla\cdot(u_2\nabla v), & x\in\Omega,\ t>0,\\ v_t=\Delta v-v+u_1+u_2, & x\in\Omega,\ t>0,\\ u_i(x,t=0)=u_{i0}(x),\quad v(x,t=0)=v_0(x),\ i=1,2, & x\in\Omega, \end{array} \right. \end{equation}

where $u_i$ denotes the population density for the $i$-th population, and $v$ represents the chemical signal concentration. $\kappa _i>0$ is the diffusion coefficient for the $i$-th population and the chemotactic coefficient $\chi _i>0$ measures the strength of the chemical signal with respect to $u_i$. Here the domain $\Omega$ is

(1.2)\begin{equation} \text{either the whole space}\ \mathbb{R}^2\ \text{or a disk}\ \Omega=B_R(0)\subset \mathbb{R}^2\ \text{with some}\ R>0. \end{equation}

When $\Omega$ is the above bounded domain, the system (1.1) is supplemented with homogenous Neumann boundary condition

(1.3)\begin{equation} \partial u_i/\partial\nu=\partial v/\partial\nu=0,\quad i=1,2. \end{equation}

For a two-dimensional domain, one of the most interesting and important question for the chemotaxis system in both biological and mathematical contexts is to determine critical mass phenomenon, namely, the behaviour of the solutions is only dependent on the initial mass of the system. This mass threshold phenomenon was exactly confirmed in the well-known Keller–Segel chemotaxis model for one population:

(1.4)\begin{equation} \left\{ \begin{array}{@{}ll} \partial_tu_1=\kappa_1\Delta u_1-\chi_1\nabla\cdot(u_1\nabla v), & x\in\Omega,\ t>0,\\ v_t=\Delta v-v+u_1, & x\in\Omega,\ t>0,\\ u_1(x,t=0)=u_{10}(x),\quad v(x,t=0)=v_0(x), & x\in\Omega. \end{array} \right. \end{equation}

Let $m_1(u_{10};D)=\|u_{10}\|_1=\int _{D}u_{10}(x)\,{\rm d}x$ for $D\subset \mathbb {R}^2$. Consider (1.4) with boundary condition (1.3) in a bounded domain $\Omega \subset \mathbb {R}^2$. An application of the Moser–Trudinger inequality to (1.4) ensures that the solution exists globally in time provided $m_1(u_{10};\Omega )<4\pi \kappa _1/\chi _1$ for arbitrary smooth domain or $m_1(u_{10};\Omega )<8\pi \kappa _1/\chi _1$ for radial domain [21]. Conversely, if $m_1(u_{10};\Omega )>8\pi \kappa _1/\chi _1$, then there exists a blow-up solution in finite time [10]. Similar to [10], there also exists a blow-up solution for (1.4) for $\Omega =\mathbb {R}^2$ when $m_1(u_{10};\mathbb {R}^2)>8\pi \kappa _1/\chi _1$. However, it was shown in [3] that the solution with $m_1(u_{10};\mathbb {R}^2)<8\pi \kappa _1/\chi _1$ exists globally over time under the following conditions $u_{10}\log (1 + |x|^2)\in L^1(\mathbb {R}^2)$ and $u_{10}\log u_{10}\in L^1(\mathbb {R}^2)$. While these additional initial data conditions have been completely removed in [15] by terms of the Moser–Trudinger inequality. Moreover, the critical case $m_1(u_{10};\mathbb {R}^2)=8\pi \kappa _1/\chi _1$ was also studied in [15], the solutions exist globally or the blow-up set of solutions equals $\mathbb {R}^2$. Because chemicals diffuse much faster than population then it is feasible to study a simple parabolic-elliptic version of (1.4), i.e., the second parabolic equation is replaced with an elliptic form

\[ 0=\Delta v-v+u_1, \quad\text{or}\quad 0=\Delta v+u_1,\quad \text{if}\ \Omega=\mathbb{R}^2, \]

or

\[ 0=\Delta v-v+u_1, \quad\text{or}\quad 0=\Delta v-\mu+u_1,\quad \text{if}\ \Omega\subset\mathbb{R}^2\ \text{is a bounded domain}, \]

where $\mu :=\|u_{10}\|_1/|\Omega |$. We refer the readers to the papers [2, 11, 13, 17–19] for a similar and satisfactory analytical description about the critical mass for these situations in two dimensions. The above results show that $8\pi \kappa _1/\chi _1$ is the critical mass for (1.4), and determines that the solutions exist globally or blow up if $\Omega$ satisfies (1.2).

For multi-population chemotaxis system, a natural question arises: do there exist critical numbers such that whenever the initial masses for populations are smaller than them then the solution will exist globally, whereas the masses are larger then the solution will blow up? Espejo et al. [5, 7–9] consider a simplified parabolic-elliptic version of two-population system likes

(1.5)\begin{equation} \left\{ \begin{array}{@{}ll} \partial_tu_1=\kappa_1\Delta u_1-\chi_1\nabla\cdot(u_1\nabla v), & \\ \partial_tu_2=\kappa_2\Delta u_2-\chi_2\nabla\cdot(u_2\nabla v), & \\ 0=\Delta v+u_1+u_2, & \text{if}\ x\in\Omega=\mathbb{R}^2,\quad \text{or}\\ 0=\Delta v-\mu'+u_1+u_2, & \text{if}\ x\in\Omega\subset\mathbb{R}^2\ \text{is a disk}, \end{array}\right. \end{equation}

where $\mu ':=(\|u_{10}\|_1+\|u_{20}\|_1)/|\Omega |$. The proof of blow-up solutions is based on a suitable adaptation of the moments technique [5, 7]. To see the known results for the global existence, based on the expression for $v$ in terms of $u_1$ and $u_2$ through the fundamental solution or the Green function associated to the Laplace operator, the main tool used in the paper by Espejo et al. is the logarithmic Hardy–Littlewood–Sobolev (HLS) inequality for system (see [4, 22, 23]): the function

(1.6)\begin{equation} \Phi(\boldsymbol{\rho})=\sum_{i\in \mathcal{I}}\int_{\mathbb{R}^2}\rho_i\log\rho_i\,{\rm d}x+\frac{1}{4\pi}\sum_{i,j\in \mathcal{I}}a_{i,j}\iint_{\mathbb{R}^2\times\mathbb{R}^2}\rho_i(x)\log|x-y|\rho_j(y)\,{\rm d}x\,{\rm d}y \end{equation}

over the class

\begin{align*} \Gamma_{\boldsymbol{M}}(\mathbb{R}^2)& =\left\{\boldsymbol{\rho}=(\rho_1,\dots,\rho_n):\rho_i \geq 0,\int_{\mathbb{R}^2}\rho_i|\log\rho_i|\,{\rm d}x<\infty,\right.\\ & \left.\qquad \int_{\mathbb{R}^2}\rho_i\,{\rm d}x=M_i, \int_{\mathbb{R}^2}\rho_i\log\left(1+|x|^2\right){\rm d}x<\infty,\ \forall\ i\in \mathcal{I}\right\} \end{align*}

is bounded from below if and only if $\Lambda _{\mathcal {I}}(\boldsymbol {M})= 0$ and

(1.7)\begin{equation} \left\{\begin{array}{@{}ll} \Lambda_{\mathcal{J}}(\boldsymbol{M})\geq 0, & \forall\,\emptyset\neq\mathcal{J}\subset\mathcal{I}, \\ \text{if}\ \Lambda_{\mathcal{J}}(\boldsymbol{M})=0\ \text{for some} \mathcal{J},\ \text{then}\ a_{i,i}+\Lambda_{\mathcal{J}\setminus\{i\}}(\boldsymbol{M})>0, & \forall i\in \mathcal{J}, \end{array} \right. \end{equation}

where $\mathcal {I}:=\{1,2,\dots,n\}$, $\boldsymbol {M}:=\{M_1,\dots,M_n\}\in (\mathbb {R}_+)^n$, $A:=(a_{i,j})_{n\times n}$ is a $n\times n$ symmetric matrix with nonnegative elements, i.e., $a_{i,j}\geq 0$, $i,j\in \mathcal {I},$ and the quadratic polynomial is given by

\[ \Lambda_{\mathcal{J}}(\boldsymbol{M}):=8\pi\sum\limits_{i\in\mathcal{J}}M_i-\sum\limits_{i,j\in\mathcal{J}}a_{i,j}M_iM_j,\quad\forall\mathcal{J} \subset\mathcal{I},\ \mathcal{J}\neq\emptyset. \]

While replacing the $-({1}/{2\pi })\log |x-y|$ in (1.6) by the Green function $G_{\Omega }(x,y)$ for the Laplace operator, then another version of the HLS inequality for system is given when $\Omega$ is a bounded domain (see [23, theorem 5]). Here we summarize the main results for (1.5) obtained by Espejo et al. through above methods for convenience (see [5, 8]): the system admits a globally bounded solution if

(1.8)\begin{equation} m_1<\frac{8\pi\kappa_1}{\chi_1},\quad m_2<\frac{8\pi\kappa_2}{\chi_2},\quad (m_1+m_2)^2<8\pi\left(\frac{\kappa_1m_1}{\chi_1}+\frac{\kappa_2m_2}{\chi_2}\right), \end{equation}

on the other hand, the solution blows up if $m_1,m_2$ satisfy any of the inequalities

\[ m_1>\frac{8\pi\kappa_1}{\chi_1},\quad m_2>\frac{8\pi\kappa_2}{\chi_2},\quad (m_1+m_2)^2>8\pi\left(\frac{\kappa_1m_1}{\chi_1}+\frac{\kappa_2m_2}{\chi_2}\right), \]

where $m_i=m_i(u_{i0};\mathbb {R}^2)=\|u_{i0}\|_1$, $i=1,2$. Similar results for Dirichlet boundary problem (1.5) was obtained in [25] by Wolansky. Hence in the plane, the critical curve of initial masses for (1.5) had been achieved.

However, there is still no available result for the parabolic-parabolic chemotaxis system (1.1) as far as the authors know. In this work, we will show that any solution of the system (1.1) exists globally in time under the sub-criticality condition (1.8). The main tool for the analysis is a version of the Moser–Trudinger inequality for system in a bounded domain $\Omega \subset \mathbb {R}^2$ [4, 22], that is, for $\forall \ \rho _i\in H^1_0(\Omega ),\ i\in \mathcal {I}$,

(1.9)\begin{equation} \Psi(\boldsymbol{\rho})=\frac{1}{2}\sum_{i,j\in\mathcal{I}}\int_{\Omega}a_{i,j}\nabla \rho_i\cdot\nabla \rho_j\,{\rm d}x -\sum_{i\in\mathcal{I}}M_i\log\left(\int_{\Omega}\exp\left(\sum\limits_{j\in\mathcal{I}}a_{i,j}\rho_j\right) {\rm d}x\right) \end{equation}

is bounded from below if and only if (1.7) holds, where the matrix $A=(a_{i,j})_{n\times n}$ is a positive definite matrix with nonnegative elements, see [23, theorem 5$(i)$].

We list two basic facts about the solution of (1.1). In the case that $\Omega$ is a bounded domain, the boundary condition (1.3) should be added. The first one is the formal conservation of the total mass:

\[ m_1=\|u_1(t)\|_{L^1(\Omega)}=\|u_{10}\|_{L^1(\Omega)},\quad m_2=\|u_2(t)\|_{L^1(\Omega)}=\|u_{20}\|_{L^1(\Omega)}\quad \text{for all}\ t>0, \]

due to the integration (1.1) $_1$ and (1.1) $_2$ over the domain, respectively. For $v$, integrating over the domain yields that

(1.10)\begin{align} \|v(t)\|_{L^1(\Omega)}=e^{{-}t}\|v_0\|_{L^1(\Omega)}+(1-e^{{-}t})\left(\|u_{10}\|_{L^1(\Omega)}+\|u_{20}\|_{L^1(\Omega)}\right)\quad \text{for all}\ t>0. \end{align}

Secondly, the system (1.1) always admits a unique nonnegative (local) solution under some mild assumptions on the nonnegative initial data if $\Omega =\mathbb {R}^2$ or $\Omega \subset \mathbb {R}^2$ is a bounded domain with smooth boundary. This fact can be proved by using some similar arguments as in one-population chemotaxis model [6, 21]. However we omit the proof for simplicity since our main interest is to find optimal conditions on the initial data, which guarantee the local solution to be global one. Through this paper, we assume that the initial data satisfies

(1.11)\begin{equation} u_{i0}\in L^1(\Omega)\cap L^{\infty}(\Omega),\ v_0\in L^1(\Omega)\cap H^1(\Omega),\quad i=1,2,\quad\text{if}\ \Omega=\mathbb{R}^2, \end{equation}

or

(1.12)\begin{equation} u_{i0}\in C^0(\bar{\Omega}),\ v_0\in C^1(\bar{\Omega}),\quad i=1,2,\quad\text{if}\ \Omega\subset\mathbb{R}^2\ \text{is a bounded domain}. \end{equation}

Let $T_{\max }>0$ be a maximal existence time of $(u_1,u_2,v)$ to (1.1). The first result states that

Theorem 1.1 Let $\Omega =B_R(0)\subset \mathbb {R}^2$ with $R>0$. Assume that nonnegative functions $u_{i0}(x),$ $i=1,2,$ and $v_0(x)$ satisfy (1.8) and (1.12). Then there exists a unique triple $(u_1,u_2,v)$ of non-negative bounded function which solves (1.1) with boundary condition (1.3) globally, i.e., $T_{\max }=\infty$.

Now, we would like to extend the global result of bounded domain to the whole space. More precisely,

Theorem 1.2 Let $\Omega =\mathbb {R}^2$. Assume that nonnegative functions $u_{i0}(x),$ $i=1,2,$ and $v_0(x)$ satisfy (1.8) and (1.11). Then $T_{\max }=\infty$.

The paper is organized as follows. In § 2, compared with (1.9), we give another version of the Moser–Trudinger inequality for system if $\rho _i\in H^1(\Omega )$, $i\in \mathcal {I}$. The third section is dedicated to the global existence in bounded domain. Section 4 is contributed to show the solution exists globally in the whole space.

2. Preliminaries

In this section, let us recall the following well-known Moser's inequality given by [16] as

\[ \frac{1}{2}\int_{\Omega}|\nabla \rho|^2\,{\rm d}x-8\pi\log\left(\int_{\Omega}\exp{\rho}\,{\rm d}x\right) \geq{-}C,\quad\forall \rho\in H^1_0(\Omega), \]

where $\Omega \subset \mathbb {R}^2$ is a domain with finite Lebesgue measure. In [22, theorem 3] or [23, theorem 5$(i)$], there exists an analogous inequality for system defined on a bounded domain of $\mathbb {R}^2$.

Lemma 2.1 Let $\mathcal {I}=\{1,\ldots,n\}$, and let $\boldsymbol{M}=(M_1,\dots,M_n)\in (\mathbb {R}_+)^n$. Assume that $A=(a_{i,j})_{n\times n}$ is a positive definite matrix with nonnegative elements. Then for any $\boldsymbol {\rho }=(\rho _1,\dots,\rho _n)\in (H^1_0(\Omega ))^n$,

\[ \frac{1}{2}\sum_{i,j\in\mathcal{I}}\int_{\Omega}a_{i,j}\nabla \rho_i\cdot\nabla \rho_j\,{\rm d}x-\sum_{i\in\mathcal{I}}M_i\log\left(\int_{\Omega}\exp \left(\sum\limits_{j\in\mathcal{I}}a_{i,j}\rho_j\right){\rm d}x\right) \]

is bounded from below if and only if

\[ \left\{\begin{array}{@{}ll} \Lambda_{\mathcal{J}}(\boldsymbol{M})\geq 0, & \forall\,\emptyset\neq\mathcal{J}\subset\mathcal{I},\\ \text{if}\ \Lambda_{\mathcal{J}}(\boldsymbol{M})=0\ \text{for some}\ \mathcal{J}, \text{then}\ a_{i,i}+\Lambda_{\mathcal{J}\setminus\{i\}}(\boldsymbol{M})>0, & \forall{i\in \mathcal{J}}. \end{array} \right. \]

Inspired by [21, theorem 2.1], for radially symmetric functions we extend the Moser–Trudinger inequality for system to the Sobolev space $H^1(\Omega )$ with trace boundary.

Lemma 2.2 Let $\Omega =B_R(0)\subset \mathbb {R}^2$ with $R>0$, and let $A=(a_{i,j})_{n\times n}$ be a positive definite matrix with nonnegative elements. Then for nonnegative $\boldsymbol{w}=(w_1,\dots,w_n)\in (H^1(\Omega ))^n$ and $\eta >0$, then there exists a constant $C(\eta )$ such that

\begin{align*} & \frac{1}{2}\sum_{i,j\in\mathcal{I}}\int_{\Omega}a_{i,j}\nabla w_i\cdot\nabla w_j\,{\rm d}x+\eta\sum\limits_{i,j\in\mathcal{I}}a_{i,j}M_i\int_{\Omega}|\nabla w_j|^2\,{\rm d}x\\ & \quad +\frac{2}{|\Omega|}\sum\limits_{i,j\in\mathcal{I}}a_{i,j}M_i \int_{\Omega}w_j\,{\rm d}x-\sum_{i\in\mathcal{I}}M_i\log\left(\int_{\Omega}\exp \left(\sum\limits_{j\in\mathcal{I}}a_{i,j}w_j\right){\rm d}x\right) \geq C(\eta) \end{align*}

if and only if

\[ \left\{\begin{array}{@{}ll} \Lambda_{\mathcal{J}}(\boldsymbol{M})\geq 0, & \forall\,\emptyset\neq\mathcal{J}\subset\mathcal{I},\\ \text{if}\ \Lambda_{\mathcal{J}}(\boldsymbol{M})=0\ \text{for some} \mathcal{J},\ \text{then}\ a_{i,i}+\Lambda_{\mathcal{J}\setminus\{i\}}(\boldsymbol{M})>0, & \forall{i\in \mathcal{J}}. \end{array} \right. \]

Proof. We only consider nonnegative $\boldsymbol {w}\in (C^1(\bar {\Omega }))^n$ because $C^1(\bar {\Omega })$ is dense in $H^1(\Omega )$. Define

\[ z_i(x):=w_i(x)-w_i(R),\quad i\in\mathcal{I}. \]

Thanks to $\boldsymbol{z}=(z_1,\dots,z_n)\in (H^1_0(\Omega ))^n$, lemma 2.1 implies that

(2.1)\begin{equation} \frac{1}{2}\sum_{i,j\in\mathcal{I}}\int_{\Omega}a_{i,j}\nabla z_i\cdot\nabla z_j\,{\rm d}x-\sum_{i\in\mathcal{I}}M_i\log\left(\int_{\Omega}\exp \left(\sum\limits_{j\in\mathcal{I}}a_{i,j}z_j\right){\rm d}x\right)\geq{-}C \end{equation}

holds if and only if

(2.2)\begin{equation} \left\{ \begin{array}{@{}ll} \Lambda_{\mathcal{J}}(\boldsymbol{M})\geq 0, & \forall\,\emptyset\neq\mathcal{J}\subset\mathcal{I},\\ \text{if}\ \Lambda_{\mathcal{J}}(\boldsymbol{M})=0\ \text{for some}\ \mathcal{J},\ \text{then}\ a_{i,i}+\Lambda_{\mathcal{J}\setminus\{i\}}(\boldsymbol{M})>0, & \forall{i\in \mathcal{J}}. \end{array} \right. \end{equation}

It is clear that

\begin{align*} & \log\left[\int_{\Omega}\exp\left(\sum\limits_{j\in\mathcal{I}}a_{i,j}z_j\right){\rm d}x\right]\\ & \quad = \log\left[\int_{\Omega}\exp\left(\sum\limits_{j\in\mathcal{I}}a_{i,j}(w_j(x)-w_j(R))\right){\rm d}x\right]\\ & \quad=\log\left[\exp\left(-\sum\limits_{j\in\mathcal{I}}a_{i,j}w_j(R)\right) \int_{\Omega}\exp\left(\sum\limits_{j\in\mathcal{I}}a_{i,j}w_j(x)\right){\rm d}x\right]\\ & \quad =\log\left[\exp\left(-\sum\limits_{j\in\mathcal{I}}a_{i,j}w_j(R)\right)\right] +\log\left[\int_{\Omega}\exp\left(\sum\limits_{j\in\mathcal{I}}a_{i,j}w_j(x)\right){\rm d}x\right]\\ & \quad=\log\left[\int_{\Omega}\exp \left(\sum\limits_{j\in\mathcal{I}}a_{i,j}w_j(x)\right){\rm d}x\right]- \sum\limits_{j\in\mathcal{I}}a_{i,j}w_j(R). \end{align*}

Then

(2.3)\begin{align} & \sum_{i\in\mathcal{I}}M_i\log\left[\int_{\Omega}\exp\left(\sum\limits_{j\in\mathcal{I}}a_{i,j}w_j(x)\right) {\rm d}x\right]\nonumber\\ & \quad =\sum_{i\in\mathcal{I}}M_i\log\left[\int_{\Omega}\exp \left(\sum\limits_{j\in\mathcal{I}}a_{i,j}z_j\right){\rm d}x\right] +\sum\limits_{i,j\in\mathcal{I}}a_{i,j}M_iw_j(R). \end{align}

Now we proceed to estimate the boundary value $w_j(R)$. Fixed $r_0\in (R/2,R)$ such that

\begin{align*} w_j(r_0)& \leq\frac{2}{r_0R}\int^{R}_{R/2}w_j(\rho)\rho \,{\rm d}\rho\\& \leq\frac{1}{\pi r_0 R}\int_{\Omega}w_j(x)\,{\rm d}x= \frac{R}{r_0|\Omega|}\int_{\Omega}w_j(x)\,{\rm d}x\leq\frac{2\|w_j\|_{L^1(\Omega)}}{|\Omega|}, \end{align*}

then from

\[ w_j(R)=w_j(r_0)+\int^R_{r_0}w'_j(\rho)\,{\rm d}\rho, \]

applying Hölder's inequality and Young's inequality with $\eta >0$, then it yields that

\begin{align*} w_j(R)& \leq w_j(r_0)+\left(\int^R_{r_0}\rho^{{-}1}\,{\rm d}\rho\right)^{{1}/{2}} \left(\int^R_{r_0}|w'_j(\rho)|^2\rho \,{\rm d}\rho\right)^{{1}/{2}}\\ & \leq w_j(r_0)+\left(\int^R_{R/2}\rho^{{-}1}\,{\rm d}\rho\right)^{{1}/{2}}\left(\int^R_{R/2}|w'_j(\rho)|^2\rho \,{\rm d}\rho\right)^{{1}/{2}}\\ & \leq w_j(r_0)+\left(\frac{\log 2}{2\pi}\right)^{{1}/{2}}\|\nabla w_j\|_{L^2(\Omega)}\\ & \leq\eta\|\nabla w_j\|^2_{L^2(\Omega)}+\frac{2\|w_j\|_{L^1(\Omega)}}{|\Omega|}+\frac{\log 2}{8\pi\eta}. \end{align*}

By (2.3), we have

\begin{align*} & \sum_{i\in\mathcal{I}}M_i\log\left(\int_{\Omega}\exp\left(\sum\limits_{j\in\mathcal{I}}a_{i,j}w_j(x)\right) {\rm d}x\right)\\ & \quad \leq\sum_{i\in\mathcal{I}}M_i\log\left(\int_{\Omega}\exp \left(\sum\limits_{j\in\mathcal{I}}a_{i,j}z_j\right){\rm d}x\right)+ \eta\sum\limits_{i,j\in\mathcal{I}}a_{i,j}M_i\|\nabla w_j\|^2_{L^2(\Omega)}\\ & \qquad +\sum\limits_{i,j\in\mathcal{I}}a_{i,j}M_i\left[\frac{2\|w_j\|_{L^1(\Omega)}}{|\Omega|}+\frac{\log 2}{8\pi\eta}\right]. \end{align*}

Observing that

\[ \sum_{i,j\in\mathcal{I}}\int_{\Omega}a_{i,j}\nabla w_i\cdot\nabla w_j\,{\rm d}x=\sum_{i,j\in\mathcal{I}}\int_{\Omega}a_{i,j}\nabla z_i\cdot\nabla z_j\,{\rm d}x, \]

it implies that

\begin{align*} & \frac{1}{2}\sum_{i,j\in\mathcal{I}}\int_{\Omega}a_{i,j}\nabla w_i\cdot\nabla w_j\,{\rm d}x-\sum_{i\in\mathcal{I}}M_i\log\left(\int_{\Omega}\exp \left(\sum\limits_{j\in\mathcal{I}}a_{i,j}w_j\right){\rm d}x\right)\\ & \quad \geq\frac{1}{2}\sum_{i,j\in\mathcal{I}}\int_{\Omega}a_{i,j}\nabla z_i\cdot\nabla z_j\,{\rm d}x-\sum_{i\in\mathcal{I}}M_i\log\left(\int_{\Omega}\exp \left(\sum\limits_{j\in\mathcal{I}}a_{i,j}z_j\right){\rm d}x\right)\\ & \qquad -\sum\limits_{i,j\in\mathcal{I}}a_{i,j}M_i\left[\eta\|\nabla w_j\|^2_{L^2(\Omega)}+\frac{2\|w_j\|_{L^1(\Omega)}}{|\Omega|}+\frac{\log 2}{8\pi\eta}\right]. \end{align*}

After a simple arrangement, we finally have

\begin{align*} & \frac{1}{2}\sum_{i,j\in\mathcal{I}}\int_{\Omega}a_{i,j}\nabla w_i\cdot\nabla w_j\,{\rm d}x+\eta\sum\limits_{i,j\in\mathcal{I}}a_{i,j}M_i\int_{\Omega}|\nabla w_j|^2\,{\rm d}x\\ & \qquad +\frac{2}{|\Omega|}\sum\limits_{i,j\in\mathcal{I}}a_{i,j}M_i\int_{\Omega}w_j\,{\rm d}x -\sum_{i\in\mathcal{I}}M_i\log\left(\int_{\Omega}\exp \left(\sum\limits_{j\in\mathcal{I}}a_{i,j}w_j\right){\rm d}x\right)\\ & \quad\geq\frac{1}{2}\sum_{i,j\in\mathcal{I}}\int_{\Omega}a_{i,j}\nabla z_i\cdot\nabla z_j\,{\rm d}x-\sum_{i\in\mathcal{I}}M_i\log\left(\int_{\Omega}\exp \left(\sum\limits_{j\in\mathcal{I}}a_{i,j}z_j\right){\rm d}x\right)\\ & \qquad-\frac{\log 2}{8\pi\eta}\sum\limits_{i,j\in\mathcal{I}}a_{i,j}M_i. \end{align*}

Therefore, this lemma has been proved by (2.1)–(2.2).

As a consequence of lemma 2.2, we have

Lemma 2.3 Let $\mathcal {I}=\{1,2\}$, $\Omega =B_R(0)\subset \mathbb {R}^2$ with $R>0$, and let $A=(a_{i,j})_{2\times 2}$ be a positive definite matrix with nonnegative elements. Then for nonnegative $\boldsymbol{w}=(w,w)\in (H^1(\Omega ))^2$ and $\eta >0$, then there exists a constant $C(\eta )$ such that

\begin{align*} & \sum_{i,j\in\mathcal{I}}a_{i,j}\left(\frac{1}{2}+ M_i\eta\right)\int_{\Omega}|\nabla w|^2\,{\rm d}x+\frac{2}{|\Omega|}\sum\limits_{i,j\in\mathcal{I}}a_{i,j}M_i\int_{\Omega}w\,{\rm d}x\\ & \quad -\sum_{i\in\mathcal{I}}M_i\log\left(\int_{\Omega}\exp\left(\sum\limits_{j\in\mathcal{I}}a_{i,j}w\right) {\rm d}x\right)\geq C(\eta) \end{align*}

if and only if

\[\left\{\begin{array}{@{}ll} \Lambda_{\mathcal{J}}(\boldsymbol{M})\geq 0, & \forall\,\emptyset\neq\mathcal{J}\subset\mathcal{I},\\ \text{if}\ \Lambda_{\mathcal{J}}(\boldsymbol{M})=0\ \text{for some}\ \mathcal{J}, \text{then}\ a_{i,i}+\Lambda_{\mathcal{J}\setminus\{i\}}(\boldsymbol{M})>0, & \forall{i\in \mathcal{J}}. \end{array} \right. \]

3. The bounded domain

The global existence of solution to (1.1) in a bounded domain $\Omega =B_R(0)\subset \mathbb {R}^2$ will be considered in this section. The proof of theorem 1.1 will be divided into several lemmas.

3.1 Free energy functional

The free energy functional

\begin{align*} \mathcal{F}[u_1,u_2,v]& :=\frac{\kappa_1}{\chi_1}\int_{\Omega}u_1\log u_1\,{\rm d}x+\frac{\kappa_2}{\chi_2}\int_{\Omega}u_2\log u_2\,{\rm d}x-\int_{\Omega}(u_1+u_2)v\,{\rm d}x\\ & \quad+\frac{1}{2}\int_{\Omega}|\nabla v|^2\,{\rm d}x+\frac{1}{2}\int_{\Omega}v^2\,{\rm d}x \end{align*}

plays an important role in the analysis of the global existence.

Lemma 3.1 Consider the local smooth solution $(u_1,u_2,v)$ to (1.1), subject to initial data $(u_{10},u_{20},v_0)$. Then

\begin{align*} \frac{{\rm d}}{{\rm d}t}\mathcal{F}[u_1,u_2,v]+\int_{\Omega}v^2_t\,{\rm d}x& ={-}\frac{1}{\chi_1}\int_{\Omega}u_1\left|\kappa_1\nabla \log u_1-\chi_1\nabla v\right|^2\,{\rm d}x\\ & \quad -\frac{1}{\chi_2}\int_{\Omega}u_2\left|\kappa_2\nabla \log u_2-\chi_2\nabla v\right|^2\,{\rm d}x. \end{align*}

Proof. Multiplying (1.1) $_i$ by $\kappa _i\log u_i-\chi _iv,i\in \{1,2\},$ respectively, we see that

(3.1)\begin{equation} \int_{\Omega}(u_1)_t\left(\kappa_1\log u_1-\chi_1 v\right){\rm d}x={-}\int_{\Omega}u_1\left|\kappa_1\nabla \log u_1-\chi_1\nabla v\right|^2\,{\rm d}x \end{equation}

and

(3.2)\begin{equation} \int_{\Omega}(u_2)_t\left(\kappa_2\log u_2-\chi_2 v\right){\rm d}x={-}\int_{\Omega}u_2\left|\kappa_2\nabla \log u_2-\chi_2\nabla v\right|^2\,{\rm d}x. \end{equation}

Testing (3.1) by $1/\chi _1$ and (3.2) by $1/\chi _2$, respectively, it is easy to obtain that

\begin{align*} & \frac{{\rm d}}{{\rm d}t}\left(\frac{\kappa_1}{\chi_1}\int_{\Omega}u_1\log u_1\,{\rm d}x+\frac{\kappa_2}{\chi_2}\int_{\Omega}u_2\log u_2\,{\rm d}x-\int_{\Omega}(u_1+u_2)v\,{\rm d}x\right)\\ & \qquad+\int_{\Omega}(u_1+u_2)v_t\,{\rm d}x\\ & \quad={-}\frac{1}{\chi_1}\int_{\Omega}u_1\left|\kappa_1\nabla \log u_1-\chi_1\nabla v\right|^2\,{\rm d}x-\frac{1}{\chi_2}\int_{\Omega}u_2\left|\kappa_2\nabla \log u_2-\chi_2\nabla v\right|^2\,{\rm d}x, \end{align*}

where we have used the fact that $({{\rm d}}/{{\rm d}t})\int _{\Omega }u_i\,{\rm d}x=0$. Notice that

\begin{align*} \int_{\Omega}(u_1+u_2)v_t\,{\rm d}x& =\int_{\Omega}(v_t-\Delta v+v)v_t\,{\rm d}x\\ & =\int_{\Omega}v^2_t\,{\rm d}x+\frac{1}{2}\frac{{\rm d}}{{\rm d}t}\left(\int_{\Omega}|\nabla v|^2\,{\rm d}x+\int_{\Omega}v^2\,{\rm d}x\right). \end{align*}

Hence

\begin{align*} & \frac{{\rm d}}{{\rm d}t}\left(\frac{\kappa_1}{\chi_1}\int_{\Omega}u_1\log u_1\,{\rm d}x+\frac{\kappa_2}{\chi_2}\int_{\Omega}u_2\log u_2\,{\rm d}x-\int_{\Omega}(u_1+u_2)v\,{\rm d}x\right.\\ & \qquad \left.+\frac{1}{2}\int_{\Omega}|\nabla v|^2\,{\rm d}x+\frac{1}{2}\int_{\Omega}v^2\,{\rm d}x\right)+\int_{\Omega}v^2_t\,{\rm d}x\\ & \quad ={-}\frac{1}{\chi_1}\int_{\Omega}u_1\left|\kappa_1\nabla \log u_1-\chi_1\nabla v\right|^2\,{\rm d}x-\frac{1}{\chi_2}\int_{\Omega}u_2\left|\kappa_2\nabla \log u_2-\chi_2\nabla v\right|^2\,{\rm d}x, \end{align*}

which implies that we have finished the proof of this lemma.

A simple fact from lemma 3.1 yields an upper bound for $\mathcal {F}$.

Lemma 3.2 Assume that $(u_1,u_2,v)$ is a local smooth solution to (1.1) in $\Omega \times (0,T_{\max })$ with initial data $(u_{10},u_{20},v_0)$ satisfying (1.12). Then

\[ \mathcal{F}[u_1,u_2,v]\leq \mathcal{F}[u_{10},u_{20},v_0]. \]

3.2 An upper bound for the entropy

In two-dimensional case, the natural way to prove the global existence of solutions to chemotaxis system is to give a bound for the entropy $\|u_i\log u_i\|_{L^1(\Omega )}$, $i=1,2$. From lemma 3.2, this can be actually achieved if the term

(3.3)\begin{equation} \int_{\Omega}(u_1+u_2)v\,{\rm d}x. \end{equation}

can be controlled by the entropy. To see this, we derive a general form as follows.

Lemma 3.3 Let $\alpha _i,\kappa _i,\chi _i>0$, $i=1,2$. For any nonnegative functions $\phi _i\in L^1(\Omega )\cap L\log L(\Omega ),\psi \in L^{\infty }(\Omega )$ satisfying $m_i=\int _{\Omega }\phi _{i}\,{\rm d}x>0$, $i=1,2$, it holds that

(3.4)\begin{align} & \alpha_1\int_{\Omega}\phi_1\psi \,{\rm d}x+\alpha_2\int_{\Omega}\phi_2\psi \,{\rm d}x\nonumber\\ & \quad \leq\frac{\kappa_1}{\chi_1}\int_{\Omega}\phi_1\log \phi_1\,{\rm d}x+\frac{\kappa_2}{\chi_2}\int_{\Omega}\phi_2\log \phi_2\,{\rm d}x +\frac{\kappa_1m_1}{\chi_1}\log\left[\int_{\Omega}\exp{\left(\frac{\chi_1\alpha_1}{\kappa_1}\psi\right)} {\rm d}x\right]\nonumber\\ & \qquad +\frac{\kappa_2m_2}{\chi_2}\log\left[\int_{\Omega}\exp{\left(\frac{\chi_2\alpha_2}{\kappa_2}\psi\right)} {\rm d}x\right]+\frac{\kappa_1}{e\chi_1}+\frac{\kappa_2}{e\chi_2}. \end{align}

Proof. It follows from the Jensen's inequality that

(3.5)\begin{align} & \alpha_1\int_{\Omega}\phi_1\psi \,{\rm d}x-\frac{\kappa_1}{\chi_1}\int_{\Omega}\phi_1\log \phi_1 \,{\rm d}x\nonumber\\& \quad=\frac{\kappa_1}{\chi_1}\left[\int_{\Omega}\phi_1\left(\frac{\chi_1\alpha_1}{ \kappa_1}\psi-\log \phi_1\right){\rm d}x\right]\nonumber\\ & \quad= \frac{\kappa_1m_1}{\chi_1}\left[\int_{\Omega}\frac{\phi_1}{m_1}\log\frac{\exp{\left( \frac{\chi_1\alpha_1}{\kappa_1}\psi\right)}}{\phi_1}{\rm d}x\right]\nonumber\\ & \quad\leq\frac{\kappa_1m_1}{\chi_1}\log\left[\int_{\Omega}\frac{\exp{\left(\frac{\chi_1\alpha}{\kappa_1} \psi\right)}}{\phi_1}\cdot\frac{\phi_1}{m_1}{\rm d}x\right]\nonumber\\ & \quad=\frac{\kappa_1m_1}{\chi_1}\log\left[\int_{\Omega}\exp{\left(\frac{\chi_1\alpha_1}{\kappa_1}\psi\right)} {\rm d}x\right]-\frac{\kappa_1m_1}{\chi_1}\log m_1\nonumber\\ & \quad\leq\frac{\kappa_1m_1}{\chi_1}\log\left[\int_{\Omega}\exp{\left(\frac{\chi_1\alpha_1}{\kappa_1} \psi\right)}{\rm d}x\right]+\frac{\kappa_1}{e\chi_1}, \end{align}

where we have used the fact that $m_1=\int _{\Omega }\phi _1\,{\rm d}x$, and $x\log x>-1/e$ for all $x>0$. Similarly, given any $\alpha _2>0$ we also have

\[ \alpha_2\int_{\Omega}\phi_2\psi \,{\rm d}x-\frac{\kappa_2}{\chi_2}\int_{\Omega}\phi_2\log \phi_2\,{\rm d}x\leq\frac{\kappa_2m_2}{\chi_2}\log\left[\int_{\Omega}\exp{\left(\frac{\chi_2 \alpha_2}{\kappa_2}\psi\right)}{\rm d}x\right]+\frac{\kappa_2}{e\chi_2}. \]

Putting the above inequalities together, it yields (3.4).

Lemma 3.4 Let $(u_1,u_2,v)$ be the local smooth solution to (1.1), subject to initial data $(u_{10},u_{20},v_0)$ satisfying (1.12). Assume that $\kappa _i>0$, $\chi _i>0$ and $m_i=\int _{\Omega }u_{i0}\,{\rm d}x,i=1,2,$ fulfill

(3.6)\begin{align} m_1<8\pi\kappa_1/\chi_1,\quad m_2<8\pi\kappa_2/\chi_2,\quad (m_1+m_2)^2<8\pi\left(\kappa_1m_1/\chi_1+\kappa_2m_2/\chi_2\right). \end{align}

Then there exists $\alpha _1>1$ and $\alpha _2>1$ such that

\begin{align*} & \alpha_1\int_{\Omega}u_1v\,{\rm d}x+\alpha_2\int_{\Omega}u_2v\,{\rm d}x\\ & \quad \leq\frac{\kappa_1}{\chi_1}\int_{\Omega}u_1\log u_1\,{\rm d}x+\frac{\kappa_2}{\chi_2}\int_{\Omega}u_2\log u_2\,{\rm d}x+\frac{1}{2}\int_{\Omega}|\nabla v|^2\,{\rm d}x+C \end{align*}

for some $C>0$.

Proof. In view of (3.6), we can choose small $\epsilon >0$ such that

(3.7)\begin{equation} \begin{gathered} 8\pi> m_1\left[\frac{\chi_1}{\kappa_1}+\epsilon\left( \frac{\chi_1}{\kappa_1}+ \frac{\chi_2}{\kappa_2}\right)\right](1+2\epsilon),\\ 8\pi> m_2\left[\frac{\chi_2}{\kappa_2}+\epsilon\left( \frac{\chi_1}{\kappa_1}+ \frac{\chi_2}{\kappa_2}\right)\right](1+2\epsilon), \end{gathered} \end{equation}

and

(3.8)\begin{align} 8\pi\left(\frac{\kappa_1m_1}{\chi_1}+\frac{\kappa_2m_2}{\chi_2}\right)> \left[(m_1+m_2)^2+\epsilon\left(\frac{\kappa_1}{\chi_1}m^2_1+ \frac{\kappa_2}{\chi_2}m^2_2\right)\left(\frac{\chi_1}{\kappa_1}+\frac{\chi_2}{ \kappa_2}\right)\right](1+2\epsilon). \end{align}

Choose $\alpha _1>0$ and $\alpha _2>0$ in lemma 3.3 as

\[ \alpha_1=\alpha_2=:1+\epsilon. \]

Denote

\[ M_1=\frac{\kappa_1m_1}{\chi_1}\left(\frac{\chi_1}{\kappa_1}+\frac{\chi_2}{ \kappa_2}\right)(1+2\epsilon),\quad M_2=\frac{\kappa_2m_2}{\chi_2}\left( \frac{\chi_1}{\kappa_1}+\frac{\chi_2}{\kappa_2}\right)(1+2\epsilon), \]

(3.9)\begin{align} a_{11}=\frac{\chi_1}{\kappa_1}\left[\frac{\frac{\chi_1}{\kappa_1}}{\frac{\chi_1}{\kappa_1}+ \frac{\chi_2}{\kappa_2}}+\epsilon\right],\quad a_{12}=a_{21}=\frac{\frac{\chi_1\chi_2}{\kappa_1\kappa_2}}{\frac{\chi_1}{\kappa_1}+ \frac{\chi_2}{\kappa_2}},\quad a_{22}=\frac{\chi_2}{\kappa_2}\left[\frac{\frac{\chi_2}{\kappa_2}}{{\frac{\chi_1}{\kappa_1}+ \frac{\chi_2}{\kappa_2}}}+\epsilon\right], \end{align}

then it is clear that $a_{11}+a_{12}=\chi _1\alpha _1/\kappa _1$, $a_{21}+a_{22}=\chi _2\alpha _2/\kappa _2$ and

\[ A=\begin{bmatrix} a_{11} & a_{12}\\ a_{21} & a_{22} \end{bmatrix} \]

is a positive definite matrix. Fixed a positive constant $\eta >0$ small enough such that

(3.10)\begin{equation} \eta\leq \frac{\epsilon}{2(\epsilon+1)}\frac{\frac{\chi_1}{\kappa_1}+\frac{\chi_2}{\kappa_2}}{\frac{\chi_1M_1}{\kappa_1}+\frac{\chi_2M_2}{\kappa_2}}. \end{equation}

Since we have

\begin{align*} \Lambda_{\{1\}}(\boldsymbol{M})& =8\pi M_1-a_{11}M^2_1 =M_1\left(8\pi-a_{11}M_1\right)\\ & =\frac{\kappa_1m_1}{\chi_1}\left( \frac{\chi_1}{\kappa_1}+ \frac{\chi_2}{\kappa_2}\right)(1+2\epsilon)\left[8\pi-a_{11}\frac{\kappa_1m_1}{\chi_1}\left( \frac{\chi_1}{\kappa_1}+ \frac{\chi_2}{\kappa_2}\right)(1+2\epsilon)\right] \\ & =\frac{\kappa_1m_1}{\chi_1}\left( \frac{\chi_1}{\kappa_1}+ \frac{\chi_2}{\kappa_2}\right)(1+2\epsilon)\\ & \quad\times\left[8\pi-m_1\left( \frac{\chi_1}{\kappa_1}+ \frac{\chi_2}{\kappa_2}\right) \left[\frac{\frac{\chi_1}{\kappa_1}}{\frac{\chi_1}{\kappa_1}+ \frac{\chi_2}{\kappa_2}}+\epsilon\right](1+2\epsilon)\right]\\ & > 0, \end{align*}

\begin{align*} \Lambda_{\{2\}}(\boldsymbol{M}) & =8\pi M_2-a_{22}M^2_2\\ & =\frac{\kappa_2m_2}{\chi_2}\left( \frac{\chi_1}{\kappa_1}+ \frac{\chi_2}{\kappa_2}\right)(1+2\epsilon)\\ & \quad\times\left[8\pi-m_2\left( \frac{\chi_1}{\kappa_1}+ \frac{\chi_2}{\kappa_2}\right)\left[\frac{\frac{\chi_2}{\kappa_2}}{\frac{\chi_1}{\kappa_1}+ \frac{\chi_2}{\kappa_2}}+\epsilon\right](1+2\epsilon)\right]\\ & > 0, \end{align*}

\begin{align*} \Lambda_{\{1,2\}}(\boldsymbol{M})& =8\pi(M_1+M_2)-a_{11}M^2_1-(a_{12}+a_{21})M_1M_2-a_{22}M^2_2\\ & =8\pi\left(\frac{\kappa_1m_1}{\chi_1}+\frac{\kappa_2m_2}{\chi_2}\right)\left(\frac{\chi_1}{\kappa_1}+ \frac{\chi_2}{\kappa_2}\right)(1+2\epsilon)\\ & \quad -a_{11}\left[\frac{\kappa_1m_1}{\chi_1}\left( \frac{\chi_1}{\kappa_1}+ \frac{\chi_2}{\kappa_2}\right)(1+2\epsilon)\right]^2\\ & \quad -(a_{12}+a_{21})\left[\frac{\kappa_1m_1}{\chi_1}\left( \frac{\chi_1}{\kappa_1}+\frac{\chi_2}{\kappa_2}\right)(1+2\epsilon)\right]\\ & \quad \times \left[\frac{\kappa_2m_2}{\chi_2}\left( \frac{\chi_1}{\kappa_1}+ \frac{\chi_2}{\kappa_2}\right)(1+2\epsilon)\right]\\ & \quad -a_{22}\left[\frac{\kappa_2m_2}{\chi_2}\left(\frac{\chi_1}{\kappa_1}+\frac{\chi_2}{\kappa_2}\right)(1+2\epsilon)\right]^2 \\ & =8\pi\left(\frac{\kappa_1m_1}{\chi_1}+\frac{\kappa_2m_2}{\chi_2}\right)\left(\frac{\chi_1}{\kappa_1}+ \frac{\chi_2}{\kappa_2}\right)(1+2\epsilon) \\ & \quad -\left[1+\frac{\kappa_1}{\chi_1}\left(\frac{\chi_1}{\kappa_1}+\frac{\chi_2}{\kappa_2}\right)\epsilon\right]\left(\frac{\chi_1}{\kappa_1}+ \frac{\chi_2}{\kappa_2}\right)(1+2\epsilon)^2m^2_1\\ & \quad -2m_1m_2\left(\frac{\chi_1}{\kappa_1}+ \frac{\chi_2}{\kappa_2}\right)(1+2\epsilon)^2 \\ & \quad -\left[1+\frac{\kappa_2}{\chi_2}\left(\frac{\chi_1}{\kappa_1}+ \frac{\chi_2}{\kappa_2}\right)\epsilon\right]\left(\frac{\chi_1}{\kappa_1}+ \frac{\chi_2}{\kappa_2}\right)(1+2\epsilon)^2m^2_2\\ & =\left(\frac{\chi_1}{\kappa_1}+\frac{\chi_2}{\kappa_2}\right)(1+2\epsilon) \left\{8\pi\left(\frac{\kappa_1m_1}{\chi_1}+\frac{\kappa_2m_2}{\chi_2}\right)\right.\\ & \quad \left.-\left[(m_1+m_2)^2+\epsilon\left(\frac{\kappa_1}{\chi_1}m^2_1+ \frac{\kappa_2}{\chi_2}m^2_2\right)\left(\frac{\chi_1}{\kappa_1}+\frac{\chi_2}{\kappa_2} \right)\right](1+2\epsilon)\right\}\\ & > 0 \end{align*}

by (3.7) and (3.8), applying lemma 2.3, then there exists a positive constant $C>0$ such that

\begin{align*} & M_1\log\left[\int_{\Omega}\exp\left[(a_{11}+a_{12})v\right]{\rm d}x\right]+ M_2\log\left[\int_{\Omega}\exp\left[(a_{21}+a_{22})v\right]{\rm d}x\right]\\ & \quad=\frac{\kappa_1m_1}{\chi_1}\left( \frac{\chi_1}{\kappa_1}+ \frac{\chi_2}{\kappa_2}\right)(1+2\epsilon)\log\left[\int_{\Omega}\exp\left(\frac{\chi_1\alpha_1}{\kappa_1} v\right){\rm d}x\right]\\ & \qquad +\frac{\kappa_2m_2}{\chi_2}\left(\frac{\chi_1}{\kappa_1}+ \frac{\chi_2}{\kappa_2}\right)(1+2\epsilon)\log\left[\int_{\Omega}\exp \left(\frac{\chi_2\alpha_2}{\kappa_2}v\right){\rm d}x\right]\\ & \quad \leq\left(\sum\limits^2_{i=1}\sum\limits^2_{j=1}a_{ij}\left(\frac{1}{2}+M_i\eta\right)\right)\int_{\Omega}| \nabla v|^2\,{\rm d}x+\frac{2}{|\Omega|}\left(\sum\limits^2_{i=1}\sum\limits^2_{j=1} a_{ij}M_i\right)\int_{\Omega}v\,{\rm d}x+C\\ & \quad =(1+\epsilon)\left[\sum\limits^2_{i=1}\left(\frac{1}{2}+M_i\eta\right) \frac{\chi_i}{\kappa_i}\right]\int_{\Omega}|\nabla v|^2\,{\rm d}x \\ & \qquad +\frac{2}{|\Omega|}\left( \frac{\chi_1M_1}{\kappa_1}+\frac{\chi_2M_2}{\kappa_2}\right)(1+\epsilon)\int_{\Omega}v\,{\rm d}x+C, \end{align*}

which together with (3.10) implies that

\begin{align*} & \frac{\kappa_1m_1}{\chi_1}\log\left[\int_{\Omega}\exp\left(\frac{\chi_1\alpha_1}{\kappa_1} v\right){\rm d}x\right]+\frac{\kappa_2m_2}{\chi_2}\log\left[\int_{\Omega}\exp \left(\frac{\chi_2\alpha_2}{\kappa_2}v\right){\rm d}x\right]\\ & \quad \leq\frac{1}{2}\int_{\Omega}|\nabla v|^2\,{\rm d}x+\frac{2(1+\epsilon)}{|\Omega|(1+2\epsilon)}\frac{\frac{\chi_1M_1}{\kappa_1}+ \frac{\chi_2M_2}{\kappa_2}}{\frac{\chi_1}{\kappa_1}+\frac{\chi_2}{\kappa_2}}\int_{\Omega}v\,{\rm d}x+C\\ & \quad \leq\frac{1}{2}\int_{\Omega}|\nabla v|^2\,{\rm d}x+\frac{2}{|\Omega|}\max\{M_1,M_2\}\int_{\Omega}v\,{\rm d}x+C. \end{align*}

Then lemma 3.3 tells that

\begin{align*} & \alpha_1\int_{\Omega}u_1v\,{\rm d}x+\alpha_2\int_{\Omega}u_2v\,{\rm d}x\\ & \quad \leq\frac{\kappa_1}{\chi_1}\int_{\Omega}u_1\log u_1\,{\rm d}x+\frac{\kappa_2}{\chi_2}\int_{\Omega}u_2\log u_2\,{\rm d}x +\frac{1}{2}\int_{\Omega}|\nabla v|^2\,{\rm d}x\\ & \qquad+\frac{2}{|\Omega|}\max\{M_1,M_2\}\int_{\Omega}v\,{\rm d}x+C, \end{align*}

which proves the lemma by (1.10).

Lemma 3.5 Under the same assumptions in lemma 3.4, then there exists $C>0$ such that

\[ \|u_1\ln u_1\|_{L^1(\Omega)}+\|u_2\ln u_2\|_{L^1(\Omega)}\leq C. \]

Proof. Lemma 3.1 asserts that

\[ \mathcal{F}[u_1,u_2,v]\leq\mathcal{F}[u_{10},u_{20},v_0] \]

in the sense that

(3.11)\begin{align} & \frac{\kappa_1}{\chi_1}\int_{\Omega}u_1\log u_1\,{\rm d}x+\frac{\kappa_2}{\chi_2}\int_{\Omega}u_2\log u_2\,{\rm d}x+\frac{1}{2}\int_{\Omega}|\nabla v|^2\,{\rm d}x+\frac{1}{2}\int_{\Omega}v^2\,{\rm d}x\nonumber\\ & \quad \leq\int_{\Omega}(u_1+u_2)v\,{\rm d}x+\mathcal{F}[u_{10},u_{20},v_0]. \end{align}

According to the choices of $\alpha _1>1$ and $\alpha _2>1$ in lemma 3.4, we may find $C>0$ such that

\begin{align*} \alpha_1\int_{\Omega}u_1v\,{\rm d}x+\alpha_2\int_{\Omega}u_2v\,{\rm d}x & \leq\frac{\kappa_1}{\chi_1}\int_{\Omega}u_1\log u_1\,{\rm d}x+\frac{\kappa_2}{\chi_2}\int_{\Omega}u_2\log u_2\,{\rm d}x\\& \quad +\frac{1}{2}\int_{\Omega}|\nabla v|^2\,{\rm d}x+C, \end{align*}

which yields that

\[ (\alpha_1-1)\int_{\Omega}u_1v\,{\rm d}x+ (\alpha_2-1)\int_{\Omega}u_1v\,{\rm d}x\leq C \]

by (1.10) and (3.11). From (3.11), this in turn shows that there exists $C>0$ such that

\[ \int_{\Omega}u_1\log u_1\,{\rm d}x+\int_{\Omega}u_2\log u_2\,{\rm d}x\leq C. \]

Proof of theorem 1.1 Assume that $(u_1,u_2,v)$ is a local classical solution of (1.1) over $(0,T_{\max })$ with the following blow-up criterion: either $T_{\max }=\infty$, or if $T_{\max }<\infty$, it should satisfy:

\[ \|u_1({\cdot},t)\|_{L^{\infty}(\Omega)}+\|u_2({\cdot},t)\|_{L^{\infty}(\Omega)}\rightarrow \infty,\quad\text{as}\ t\rightarrow T_{\max}. \]

A version of the Gagliardo–Nirenberg inequality in two-dimensional bounded domain shows that for each $\epsilon >0$, there exists a positive constant $C_{\epsilon }>0$ such that (see [21, lemma 3.5], [24, lemma A.5])

(3.12)\begin{equation} \|\phi\|^3_{L^3(\Omega)}\leq \epsilon\|\nabla \phi\|^{2}_{L^2(\Omega)}\|\phi\log|\phi|\|_{L^1(\Omega)}+C_{\epsilon}\|\phi\|^3_{L^1(\Omega)}+C_{\epsilon},\quad \forall\ \phi\in H^{1}(\Omega). \end{equation}

By means of (3.12) and lemma 3.5, we follow a similar argument in [21, lemma 3.6] to find $C>0$ such that

\[ \|u_1({\cdot},t)\|_{L^2(\Omega)}+\|u_2({\cdot},t)\|_{L^2(\Omega)}\leq C,\quad\forall\ t\in(0,T_{\max}). \]

By the well-known Moser–Alikakos iteration procedure [1], the solutions of (1.1) must be uniformly bounded for all $t\in (0,T_{\max })$, that is, $T_{\max }=\infty$.

4. The whole space

The proof of global existence for the whole space $\mathbb {R}^2$ also relies on the Moser–Trudinger inequality for system given in lemma 2.1. Similar to the bounded domain case, it is possible to control (3.3) by the entropy. For this purpose, we have

Lemma 4.1 Consider a local solution $(u_1,u_2,v)$ to (1.1) in $\mathbb {R}^2\times (0,T)$ with $T>0$, subject to initial data $(u_{10},u_{20},v_0)$ satisfying (1.11). Suppose that $m_i=\int _{\Omega }u_{i0}\,{\rm d}x$, $i=1,2$, fulfills

(4.1)\begin{equation} m_1<8\pi\kappa_1/\chi_1,\quad m_2<8\pi\kappa_2/\chi_2,\quad (m_1+m_2)^2<8\pi\left(\kappa_1m_1/\chi_1+\kappa_2m_2/\chi_2\right). \end{equation}

Then there exists $\epsilon >0$ such that

\begin{align*} \int_{\mathbb{R}^2}(u_1+u_2)v\,{\rm d}x& \leq\frac{\kappa_1}{\chi_1(1+\epsilon)}\int_{\mathbb{R}^2}(u_1+1)\log (u_1+1)\,{\rm d}x\\ & \quad +\frac{\kappa_2}{\chi_2(1+\epsilon)}\int_{\mathbb{R}^2}(u_2+1)\log (u_2+1)\,{\rm d}x\\ & \quad +\frac{1}{2(1+\epsilon)}\int_{\mathbb{R}^2}|\nabla v|^2\,{\rm d}x+C,\quad t>0, \end{align*}

for some $C>0$.

Proof. Inspired by lemma from [15, lemma 2.1] for a single-species chemotaxis system, we use the similar argument to deal with multi-species scenario on the base of the Moser–Trudinger inequality for system. For any initial data $(u_{10},u_{20},v_0)$ satisfying (1.11), we

\[ \widetilde{m}=\|u_{10}\|_{L^1(\mathbb{R}^2)}+\|u_{20}\|_{L^1(\mathbb{R}^2)}+\|v_0\|_{L^1(\mathbb{R}^2)}. \]

Choose $\epsilon >0$ small enough and $s>0$ large enough, then the assumption (4.1) ensures that

(4.2)\begin{equation} \begin{gathered} 8\pi> (m_1+\widetilde{m}/s)\left[\frac{\chi_1}{\kappa_1}+\epsilon\left( \frac{\chi_1}{\kappa_1}+\frac{\chi_2}{\kappa_2}\right)\right](1+2\epsilon),\\ 8\pi> (m_2+\widetilde{m}/s)\left[\frac{\chi_2}{\kappa_2}+\epsilon\left( \frac{\chi_1}{\kappa_1}+ \frac{\chi_2}{\kappa_2}\right)\right](1+2\epsilon), \end{gathered} \end{equation}

and

(4.3)\begin{align} & 8\pi\left[\frac{\kappa_1}{\chi_1}(m_1+\widetilde{m}/s)+\frac{\kappa_2}{\chi_2}(m_2+\widetilde{m}/s)\right]\nonumber\\ & \quad >\left\{\vphantom{\left[\frac{\kappa_1}{\chi_1}(m_1+ \widetilde{m}/s)^2+\frac{\kappa_2}{\chi_2}(m_2+\widetilde{m}/s)^2\right]}(m_1+m_2+2\widetilde{m}/s)^2\right.\nonumber\\ & \left.\quad +\epsilon\left[\frac{\kappa_1}{\chi_1}(m_1+ \widetilde{m}/s)^2+\frac{\kappa_2}{\chi_2}(m_2+\widetilde{m}/s)^2\right] \left(\frac{\chi_1}{\kappa_1}+\frac{\chi_2}{\kappa_2}\right)\right\}(1+2\epsilon). \end{align}

Let

\[ \widetilde{v}(x,t)=\max\{v(x,t)-s,0\},\quad \forall (x,t)\in\mathbb{R}^2\times(0,T), \]

and

\[ \Omega(t)=\left\{x\in\mathbb{R}^2:v(x,t)>s\right\},\quad \forall t\in(0,T). \]

Note that the Lebesgue measure of $\Omega (t)$ denoted by $|\Omega (t)|$ is finite, because (1.10) and

\[ s\cdot|\Omega(t)|\leq\|v(t)\|_1\leq \|u_{10}\|_{L^1(\mathbb{R}^2)}+\|u_{20}\|_{L^1(\mathbb{R}^2)}+\|v_0\|_{L^1(\mathbb{R}^2)}=\widetilde{m},\quad \forall\ t\in(0,T), \]

imply that

(4.4)\begin{equation} |\Omega(t)|\leq\widetilde{m}/s,\quad \forall\ t\in(0,T). \end{equation}

Moreover, we see that

\begin{align*} \int_{\mathbb{R}^2}(u_1+u_2)v\,{\rm d}x& =\int_{\Omega(t)}(u_1+u_2)( \widetilde{v}+s)\,{\rm d}x+\int_{\mathbb{R}^2\setminus\Omega(t)}(u_1+u_2)v\,{\rm d}x\\ & \leq\int_{\Omega(t)}(u_1+u_2) \widetilde{v}\,{\rm d}x+s\left(\|u_{10}\|_1+\|u_{20}\|_1\right). \end{align*}

A similar computation as (3.5) and utilizing (4.4), we obtain that

(4.5)\begin{align} & \alpha_i\int_{\Omega(t)}(u_i+1)\widetilde{v}\,{\rm d}x\nonumber\\ & \quad \leq\frac{\kappa_i}{\chi_i}\int_{\Omega(t)}(u_i+1)\log (u_i+1)\,{\rm d}x\nonumber\\ & \qquad +\frac{\kappa_i(m_i(t)+|\Omega(t)|)}{\chi_i}\log\left[\int_{\Omega(t)} \exp\left(\frac{\chi_i\alpha_i}{\kappa_i}\widetilde{v}\right){\rm d}x\right]\nonumber\\ & \qquad -\frac{\kappa_i(m_i(t)+|\Omega(t)|)}{\chi_i}\log(m_i(t)+|\Omega(t)|)\nonumber\\ & \quad \leq\frac{\kappa_i}{\chi_i}\int_{\Omega(t)}(u_i+1)\log (u_i+1)\,{\rm d}x \nonumber\\ & \qquad +\frac{\kappa_i(m_i+\widetilde{m}/s)}{\chi_i}\log\left[\int_{\Omega(t)} \exp\left(\frac{\chi_i\alpha_i}{\kappa_i}\widetilde{v}\right){\rm d}x\right]+C, \end{align}

where $\alpha _i>1$, $m_i(t)=\int _{\Omega (t)}u_i\,{\rm d}x\leq m_i$, $i=1,2$, and

\[ C=\frac{\kappa_i(m_i+\widetilde{m}/s)}{\chi_i}\log(m_i+\widetilde{m}/s). \]

Without loss of generality, we assume that $|\Omega (t)|>1$, otherwise we may take $\widetilde {\Omega }(t)$ such that $|\widetilde {\Omega }(t)|>1$ and $\Omega (t)\subset \widetilde {\Omega }(t)$. Define $M_1,M_2>0$ as

\begin{align*} M_1& =\frac{\kappa_1}{\chi_1}(m_1+\widetilde{m}/s)\left(\frac{\chi_1}{\kappa_1}+\frac{\chi_2}{ \kappa_2}\right)(1+2\epsilon),\\ M_2& =\frac{\kappa_2}{\chi_2}(m_2+\widetilde{m}/s)\left( \frac{\chi_1}{\kappa_1}+\frac{\chi_2}{\kappa_2}\right)(1+2\epsilon). \end{align*}

Let $A=(a_{i,j})_{2\times 2}$ be a positive definite matrix with elements from (3.9) and $\alpha _1=\alpha _2=1+\epsilon$. According to (4.2)–(4.3), we have

\[ \Lambda_{\mathcal{J}}(\textbf{M})>0,\quad \forall\mathcal{J}\subset\mathcal{I}=\{1,2\},\ \mathcal{J}\neq\emptyset, \]

then applying lemma 2.1 to see that

\begin{align*} & M_1\log\left[\int_{\Omega(t)}\exp\left[(a_{11}+a_{12})\widetilde{v}\right]{\rm d}x\right]+M_2 \log\left[\int_{\Omega(t)}\exp\left[(a_{21}+a_{22})\widetilde{v}\right]{\rm d}x\right]\\ & \quad = \frac{\kappa_1}{\chi_1}(m_1+\widetilde{m}/s)\left( \frac{\chi_1}{\kappa_1}+ \frac{\chi_2}{\kappa_2}\right)(1+2\epsilon)\log\left[\int_{\Omega(t)}\exp\left(\frac{\chi_1}{\kappa_1} (1+\epsilon)\widetilde{v}\right){\rm d}x\right]\\ & \qquad +\frac{\kappa_2}{\chi_2}(m_2+\widetilde{m}/s)\left(\frac{\chi_1}{\kappa_1}+ \frac{\chi_2}{\kappa_2}\right)(1+2\epsilon)\log\left[\int_{\Omega(t)}\exp \left(\frac{\chi_2}{\kappa_2}(1+\epsilon)\widetilde{v}\right){\rm d}x\right]\\ & \quad \leq\frac{1}{2}\left(\sum\limits^2_{i=1}\sum\limits^2_{j=1}a_{ij}\right)\int_{\Omega(t)}|\nabla \widetilde{v}|^2\,{\rm d}x =\frac{1}{2}\left(\frac{\chi_1}{\kappa_1}+\frac{\chi_2}{\kappa_2}\right)(1+\epsilon)\int_{\Omega(t)}|\nabla \widetilde{v}|^2\,{\rm d}x. \end{align*}

Then we have the following inequality

\begin{align*} & \frac{\kappa_1}{\chi_1}(m_1+\widetilde{m}/s)\log\left[\int_{\Omega(t)} \exp\left(\frac{\chi_1\alpha_1}{\kappa_1} \widetilde{v}\right){\rm d}x\right]\\ & \qquad+\frac{\kappa_2}{\chi_2}(m_2+\widetilde{m}/s) \log\left[\int_{\Omega(t)}\exp\left(\frac{\chi_2\alpha_2}{\kappa_2}\widetilde{v}\right){\rm d}x\right]\\ & \quad \leq\frac{1+\epsilon}{2(1+2\epsilon)}\int_{\Omega(t)}|\nabla \widetilde{v}|^2\,{\rm d}x\leq\frac{1}{2}\int_{\Omega(t)}|\nabla \widetilde{v}|^2\,{\rm d}x. \end{align*}

Inserting the above into (4.5) yields that

\begin{align*} & (1+\epsilon)\int_{\Omega(t)}(u_1+1)\widetilde{v}\,{\rm d}x+(1+\epsilon) \int_{\Omega(t)}(u_2+1)\widetilde{v}\,{\rm d}x\\ & \quad \leq\frac{\kappa_1}{\chi_1}\int_{\Omega(t)}(u_1+1)\log (u_1+1)\,{\rm d}x\\ & \qquad +\frac{\kappa_2}{\chi_2}\int_{\Omega(t)}(u_2+1)\log (u_2+1)\,{\rm d}x +\frac{1}{2}\int_{\Omega(t)}|\nabla \widetilde{v}|^2\,{\rm d}x+C \end{align*}

for all $t\in (0,T)$, so we have

\begin{align*} \int_{\mathbb{R}^2}(u_1+u_2)v\,{\rm d}x & \leq\int_{\Omega(t)}(u_1+u_2) \widetilde{v}\,{\rm d}x+ s\left(\|u_{10}\|_1+\|u_{20}\|_1\right)\\ & \leq\int_{\Omega(t)}(u_1+1)\widetilde{v}\,{\rm d}x+\int_{\Omega(t)}(u_2+1)\widetilde{v}\,{\rm d}x+ s\left(\|u_{10}\|_1+\|u_{20}\|_1\right) \\ & \leq\frac{\kappa_1}{\chi_1(1+\epsilon)}\int_{\Omega(t)}(u_1+1)\log (u_1+1)\,{\rm d}x\\ & \quad +\frac{\kappa_2}{\chi_2(1+\epsilon)}\int_{\Omega(t)}(u_2+1)\log (u_2+1)\,{\rm d}x\\ & \quad + \frac{1}{2(1+\epsilon)}\int_{\Omega(t)}|\nabla \widetilde{v}|^2\,{\rm d}x+C\\ & \leq\frac{\kappa_1}{\chi_1(1+\epsilon)}\int_{\mathbb{R}^2}(u_1+1)\log (u_1+1) \,{\rm d}x \\ & \quad +\frac{\kappa_2}{\chi_2(1+\epsilon)}\int_{\mathbb{R}^2}(u_2+1)\log (u_2+1)\,{\rm d}x\\ & \quad +\frac{1}{2(1+\epsilon)}\int_{\mathbb{R}^2}|\nabla v|^2\,{\rm d}x+C,\quad \forall\ t\in(0,T). \end{align*}

This lemma is complete.

The following proposition could be regarded as an analogue of the result for one-single Keller–Segel chemotaxis model (see [20, proposition 4.1]).

Lemma 4.2 Consider a local solution $(u_1,u_2,v)$ to (1.1), subject to initial data $(u_{10},u_{20},v_0)$ satisfying (1.11). Then

(4.6)\begin{align} & \frac{{\rm d}}{{\rm d}t}\mathcal{G}[u_1,u_2,v]+\int_{\mathbb{R}^2}v^2_t\,{\rm d}x\nonumber\\ & \quad ={-}\chi_1\int_{\mathbb{R}^2}u_1\left|\nabla\left(\frac{\kappa_1}{\chi_1}\log(u_1+1)-v\right)\right|^2 -\chi_2\int_{\mathbb{R}^2}u_2\left|\nabla\left(\frac{\kappa_2}{\chi_2}\log(u_2+1)-v\right)\right|^2\nonumber\\ & \qquad -\chi_1\int_{\mathbb{R}^2}\left|\nabla\left(\frac{\kappa_1}{\chi_1}\log(u_1+1)-\frac{1}{2}v\right)\right|^2 -\chi_2\int_{\mathbb{R}^2}\left|\nabla\left(\frac{\kappa_2}{\chi_2}\log(u_2+1)-\frac{1}{2}v\right)\right|^2\nonumber\\ & \qquad +\frac{\chi_1+\chi_2}{4}\int_{\mathbb{R}^2}|\nabla v|^2\,{\rm d}x, \end{align}

where

\begin{align*} \mathcal{G}[u_1,u_2,v]& =\frac{\kappa_1}{\chi_1}\int_{\mathbb{R}^2}(u_1+1)\log (u_1+1)\,{\rm d}x+\frac{\kappa_2}{\chi_2}\int_{\mathbb{R}^2}(u_2+1)\log (u_2+1)\,{\rm d}x\\ & \quad-\int_{\mathbb{R}^2}(u_1+u_2)v\,{\rm d}x+\frac{1}{2}\int_{\mathbb{R}^2}|\nabla v|^2\,{\rm d}x+\frac{1}{2}\int_{\mathbb{R}^2}v^2\,{\rm d}x. \end{align*}

Proof. We adopt the similar arguments as lemma 3.1 to prove this lemma. Multiplying (1.1) $_i$ by $\kappa _i/\chi _i\log (u_i+1),$ $i=1,2,$ and integrating over $\mathbb {R}^2$, it induces that

\begin{align*} \frac{\kappa_i}{\chi_i}\int_{\mathbb{R}^2}(u_i+1)_t\log (u_i+1)& ={-}\frac{\kappa^2_i}{\chi_i}\int_{\mathbb{R}^2}\frac{|\nabla u_i|^2}{u_i+1}\,{\rm d}x\\& \quad +\kappa_i\int_{\mathbb{R}^2}\frac{u_i}{u_i+1}\nabla u_i\cdot\nabla v\,{\rm d}x,\quad i=1,2. \end{align*}

Moreover, we have

\begin{align*} & \frac{{\rm d}}{{\rm d}t}\left[\frac{\kappa_1}{\chi_1}\int_{\mathbb{R}^2}(u_1+1)\log (u_1+1)\,{\rm d}x+\frac{\kappa_2}{\chi_2}\int_{\mathbb{R}^2}(u_2+1)\log (u_2+1)\,{\rm d}x\right.\\ & \qquad \left.-\int_{\mathbb{R}^2}(u_1+u_2)v\,{\rm d}x\right]+ \int_{\mathbb{R}^2}(u_1+u_2)v_t\,{\rm d}x+\int_{\mathbb{R}^2}(u_1+u_2)_tv\,{\rm d}x\\ & \quad ={-}\frac{\kappa^2_1}{\chi_1}\int_{\mathbb{R}^2}\frac{|\nabla u_1|^2}{u_1+1}\,{\rm d}x-\frac{\kappa^2_2}{\chi_2}\int_{\mathbb{R}^2}\frac{|\nabla u_2|^2}{u_2+1}\,{\rm d}x\\ & \qquad +\kappa_1\int_{\mathbb{R}^2}\frac{u_1}{u_1+1}\nabla u_1\cdot\nabla v\,{\rm d}x+\kappa_2\int_{\mathbb{R}^2}\frac{u_2}{u_2+1}\nabla u_2\cdot\nabla v\,{\rm d}x, \end{align*}

where we use the fact that $({{\rm d}}/{{\rm d}t})\int _{\mathbb {R}^2}(u_i+1)\,{\rm d}x=0$, $i=1,2$. Since

\begin{align*} \int_{\mathbb{R}^2}(u_1+u_2)v_t\,{\rm d}x& =\int_{\mathbb{R}^2}(v_t-\Delta v+v)v_t\,{\rm d}x\\ & =\int_{\mathbb{R}^2}v^2_t\,{\rm d}x+\frac{1}{2}\frac{{\rm d}}{{\rm d}t}\left(\int_{\mathbb{R}^2}|\nabla v|^2\,{\rm d}x+\int_{\mathbb{R}^2}v^2\,{\rm d}x\right) \end{align*}

and

\begin{align*} \int_{\mathbb{R}^2}(u_1+u_2)_tv\,{\rm d}x& =\chi_1\int_{\mathbb{R}^2}u_1|\nabla v|^2\,{\rm d}x+ \chi_2\int_{\mathbb{R}^2}u_2|\nabla v|^2\,{\rm d}x\\ & \quad -\kappa_1\int_{\mathbb{R}^2}\nabla u_1\cdot\nabla v\,dx-\kappa_2\int_{\mathbb{R}^2}\nabla u_2\cdot \nabla v\,{\rm d}x \end{align*}

hold out, then it is obvious that

\begin{align*} & \frac{{\rm d}}{{\rm d}t}\left(\frac{\kappa_1}{\chi_1}\int_{\mathbb{R}^2}(u_1+1)\log (u_1+1)\,{\rm d}x+\frac{\kappa_2}{\chi_2}\int_{\mathbb{R}^2}(u_2+1)\log (u_2+1)\,{\rm d}x\right)\\ & \qquad -\frac{{\rm d}}{{\rm d}t}\left(\int_{\mathbb{R}^2}(u_1+u_2)v\,{\rm d}x+\frac{1}{2}\int_{\mathbb{R}^2}|\nabla v|^2\,{\rm d}x+\frac{1}{2}\int_{\mathbb{R}^2}v^2\,{\rm d}x\right)+\int_{\mathbb{R}^2}v^2_t\,{\rm d}x\\ & \quad ={-}\frac{\kappa^2_1}{\chi_1}\int_{\mathbb{R}^2}\frac{|\nabla u_1|^2}{u_1+1}\,{\rm d}x-\frac{\kappa^2_2}{\chi_2}\int_{\mathbb{R}^2}\frac{|\nabla u_2|^2}{u_2+1}\,{\rm d}x -\chi_1\int_{\mathbb{R}^2}u_1|\nabla v|^2\,{\rm d}x\\ & \qquad-\chi_2\int_{\mathbb{R}^2}u_2|\nabla v|^2\,{\rm d}x\\ & \qquad +\kappa_1\int_{\mathbb{R}^2}\frac{2u_1+1}{u_1+1}\nabla u_1\cdot\nabla v\,{\rm d}x+\kappa_2\int_{\mathbb{R}^2}\frac{2u_2+1}{u_2+1}\nabla u_2\cdot\nabla v\,{\rm d}x\\ & \quad ={-}\chi_1\int_{\mathbb{R}^2}u_1\left|\nabla\left(\frac{\kappa_1}{\chi_1}\log(u_ 1+1)-v\right)\right|^2-\chi_2\int_{\mathbb{R}^2}u_2\left|\nabla\left(\frac{\kappa_2}{\chi_ 2}\log(u_2+1)-v\right)\right|^2\\ & \qquad-\chi_1\int_{\mathbb{R}^2}\left|\nabla\left(\frac{\kappa_1}{\chi_1}\log(u_1+1)-\frac{ 1}{2}v\right)\right|^2-\chi_2\int_{\mathbb{R}^2}\left|\nabla\left(\frac{\kappa_2}{\chi_2}\log( u_2+1)-\frac{1}{2}v\right)\right|^2\\ & \qquad +\frac{\chi_1+\chi_2}{4}\int_{\mathbb{R}^2}|\nabla v|^2\,{\rm d}x. \end{align*}

Therefore, we have finished the proof of this lemma.

Lemma 4.3 Consider a local solution $(u_1,u_2,v)$ to (1.1) in $\mathbb {R}^2\times (0,T)$, subject to initial data $(u_{10},u_{20},v_0)$ satisfying (1.11). Under the same assumptions in lemma 4.1, then there exists a positive constant $C>0$ such that

(4.7)\begin{equation} \int_{\mathbb{R}^2}(u_1+1)\log(u_1+1)\,{\rm d}x+\int_{\mathbb{R}^2}(u_2+1)\log(u_2+1)\,{\rm d}x\leq C,\quad\forall t\in(0,T), \end{equation}

and

(4.8)\begin{equation} \int^t_0\int_{\mathbb{R}^2}v^2_t(s)\,{\rm d}x\,{\rm d}s\leq C,\quad\forall t\in(0,T). \end{equation}

Proof. Invoking the definition of $\mathcal {G}$ and lemma 4.1, we firstly obtain

(4.9)\begin{align} \mathcal{G}[u_1,u_2,v]& =\frac{\kappa_1}{\chi_1}\int_{\mathbb{R}^2}(u_1+1) \log (u_1+1)\,{\rm d}x+\frac{\kappa_2}{\chi_2}\int_{\mathbb{R}^2}(u_2+1)\log (u_2+1)\,{\rm d}x\nonumber\\ & \quad -\int_{\mathbb{R}^2}(u_1+u_2)v\,{\rm d}x+\frac{1}{2}\int_{\mathbb{R}^2}|\nabla v|^2\,{\rm d}x+\frac{1}{2}\int_{\mathbb{R}^2}v^2\,{\rm d}x\nonumber\\ & \geq\frac{\kappa_1\epsilon}{\chi_1(1+\epsilon)}\int_{\mathbb{R}^2}(u_1+1)\log (u_1+1)\,{\rm d}x\nonumber\\ & \quad +\frac{\kappa_2\epsilon}{\chi_2(1+\epsilon)}\int_{\mathbb{R}^2}(u_2+1)\log (u_2+1)\,{\rm d}x\nonumber\\ & \quad +\frac{\epsilon}{2(1+\epsilon)}\int_{\mathbb{R}^2}|\nabla v|^2\,{\rm d}x+\frac{1}{2}\int_{\mathbb{R}^2}v^2\,{\rm d}x+C,\quad\forall\ t\in(0,T). \end{align}

Moreover, lemma 4.1 ensures that there exist $\epsilon >0$ and $C>0$ such that

\begin{align*} \int_{\mathbb{R}^2}(u_1+u_2)v\,{\rm d}x & \leq\frac{\kappa_1}{\chi_1}\int_{\mathbb{R}^2}(u_1+1)\log (u_1+1)\,{\rm d}x+\frac{\kappa_2}{\chi_2}\int_{\mathbb{R}^2}(u_2+1)\log (u_2+1)\,{\rm d}x\\ & \quad +\frac{1}{2(1+\epsilon)}\int_{\mathbb{R}^2}|\nabla v|^2\,{\rm d}x+C,\quad\forall\ t\in(0,T). \end{align*}

Reversely, it implies that

\begin{align*} \frac{1}{2}\int_{\mathbb{R}^2}|\nabla v|^2\,{\rm d}x& = \mathcal{G}[u_1,u_2,v]-\frac{\kappa_1}{\chi_1}\int_{\mathbb{R}^2}(u_1+1)\log (u_1+1)\,{\rm d}x\\ & \quad -\frac{\kappa_2}{\chi_2}\int_{\mathbb{R}^2}(u_2+1)\log (u_2+1)\,{\rm d}x -\frac{1}{2}\int_{\mathbb{R}^2}v^2\,{\rm d}x+\int_{\mathbb{R}^2}(u_1+u_2)v\,{\rm d}x\\ & \leq\mathcal{G}[u_1,u_2,v]+\frac{1}{2(1+\epsilon)}\int_{\mathbb{R}^2}|\nabla v|^2\,{\rm d}x+C. \end{align*}

Hence, one can see that

\[ \int_{\mathbb{R}^2}|\nabla v|^2\,{\rm d}x\leq \frac{2(1+\epsilon)}{\epsilon}\mathcal{G}[u_1,u_2,v]+\frac{2(1+\epsilon)C}{\epsilon}. \]

Combining it with (4.6) yields that

\begin{align*} \frac{{\rm d}}{{\rm d}t}\mathcal{G}[u_1,u_2,v]+\int_{\mathbb{R}^2}v^2_t\,{\rm d}x& \leq \frac{(1+\epsilon)(\chi_1+\chi_2)}{2\epsilon}\mathcal{G}[u_1,u_2,v]\\& \quad +\frac{( 1+\epsilon)(\chi_1+\chi_2)C}{2\epsilon},\quad \forall\ t\in(0,T). \end{align*}

Using Gronwall's inequality to above inequality, it means that

\[ \mathcal{G}[u_1,u_2,v](t)+\int^t_0\int_{\mathbb{R}^2}v^2_t\,{\rm d}x\,{\rm d}s\leq \widetilde{C},\quad \forall\ t\in(0,T). \]

Then we obtain (4.7)–(4.8) by terms of (4.9).

Proof of theorem 1.2 Prove by contradiction. Under the assumptions in theorem 1.2, suppose that there exists a solution $(u_1,u_2,v)$ of (1.1) which blows up at finite time $T<\infty$. Lemma 4.3 tells us that there exists $C>0$ such that

\[ \int_{\mathbb{R}^2}(u_1+1)\log(u_1+1)\,{\rm d}x+\int_{\mathbb{R}^2}(u_2+1)\log(u_2+1)\,{\rm d}x+ \int^t_0\int_{\mathbb{R}^2}v^2_t(s)\,{\rm d}x\,{\rm d}s\leq C. \]

Based on the following inequality in two-dimensional domain

\[ \|\phi\|^3_{L^3(\mathbb{R}^2)}\leq \epsilon\|\nabla \phi\|^{2}_{L^2(\mathbb{R}^2)}\|(\phi+1)\log(\phi+1)|\|_{L^1(\mathbb{R}^2)}+C_{ \epsilon}\|\phi\|^3_{L^1(\mathbb{R}^2)},\quad\forall\ \phi\in H^{1}(\mathbb{R}^2), \]

we have

\[ \|u_1({\cdot},t)\|_{L^2(\mathbb{R}^2)}+\|u_2({\cdot},t)\|_{L^2(\mathbb{R}^2)}\leq C,\quad\forall\ t\in(0,T) \]

by a similar argument in [20, proposition 5.1]. However, through the standard theory of the parabolic regularity, it is straightforward to show that the solution $(u_1,u_2,v)$ remains in $L^{\infty }(\mathbb {R}^2)$ for all $t\in (0,T]$. It is a contradiction with the blow-up criteria, which implies the solution $(u_1,u_2,v)$ exists globally in time.