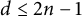1 Introduction
When does a projective variety X admit a flat degeneration to a toric variety? Among other applications, such degenerations are used in the mirror-theoretic approach to the classification of Fano varieties [Reference Coates, Corti, Galkin, Golyshev and KasprzykCCG+13], the construction of integrable systems [Reference Harada and KavehHK15], and in bounding Seshadri constants [Reference ItoIto14]. The many applications of toric degenerations notwithstanding, there is as of yet no general method for determining if a given variety admits a toric degeneration.
In this note, we will consider the special case of toric degenerations of some
![]() $X\subset \mathbb {P}^n$
obtained as the flat limit of X under a
$X\subset \mathbb {P}^n$
obtained as the flat limit of X under a
![]() $\mathbb {G}_m$
-action on
$\mathbb {G}_m$
-action on
![]() $\mathbb {P}^n$
. In the case that the
$\mathbb {P}^n$
. In the case that the
![]() $\mathbb {G}_m$
-action arises as a one-parameter subgroup of the standard torus on
$\mathbb {G}_m$
-action arises as a one-parameter subgroup of the standard torus on
![]() $\mathbb {P}^n$
, the situation may be well understood by studying the Gröbner fan and tropicalization of X [Reference Maclagan and SturmfelsMS15]. However, if we consider arbitrary
$\mathbb {P}^n$
, the situation may be well understood by studying the Gröbner fan and tropicalization of X [Reference Maclagan and SturmfelsMS15]. However, if we consider arbitrary
![]() $\mathbb {G}_m$
-actions on
$\mathbb {G}_m$
-actions on
![]() $\mathbb {P}^n$
, the situation becomes more complicated. As a test case, we investigate the existence of such toric degenerations when X is a hypersurface.
$\mathbb {P}^n$
, the situation becomes more complicated. As a test case, we investigate the existence of such toric degenerations when X is a hypersurface.
In order to state our result, we introduce some notation. Throughout the paper,
![]() $\mathbb {K}$
will be an algebraically closed field of characteristic zero. Let
$\mathbb {K}$
will be an algebraically closed field of characteristic zero. Let
![]() $\omega \in \mathbb {R}^{n+1}$
. Consider any polynomial
$\omega \in \mathbb {R}^{n+1}$
. Consider any polynomial
![]() $f\in \mathbb {K}[x_0,\ldots ,x_n]$
, where we write
$f\in \mathbb {K}[x_0,\ldots ,x_n]$
, where we write
using multi-index notation. The initial term of f with respect to the weight vector
![]() $\omega $
is
$\omega $
is
where
![]() $\lambda $
is the maximum of
$\lambda $
is the maximum of
![]() $\langle u,\omega \rangle $
as u ranges over all
$\langle u,\omega \rangle $
as u ranges over all
![]() $u\in \mathbb {Z}_{\geq 0}$
with
$u\in \mathbb {Z}_{\geq 0}$
with
![]() $c_u\neq 0$
. For an ideal
$c_u\neq 0$
. For an ideal
![]() $J\subset \mathbb {K}[x_0,\ldots ,x_n]$
, its initial ideal with respect to the weight vector
$J\subset \mathbb {K}[x_0,\ldots ,x_n]$
, its initial ideal with respect to the weight vector
![]() $\omega $
is
$\omega $
is
The weight of a monomial
![]() $x^u$
with respect to
$x^u$
with respect to
![]() $\omega $
is the scalar product
$\omega $
is the scalar product
![]() $\langle u,\omega \rangle \in \mathbb {R}$
.
$\langle u,\omega \rangle \in \mathbb {R}$
.
Definition 1.1 Let
![]() $X\subset \mathbb {P}^n$
be a projective variety over
$X\subset \mathbb {P}^n$
be a projective variety over
![]() $\mathbb {K}$
. We say that X admits a toric Gröbner degeneration up to change of coordinates if there exist a
$\mathbb {K}$
. We say that X admits a toric Gröbner degeneration up to change of coordinates if there exist a
![]() $\operatorname {\mathrm {PGL}}(n+1)$
translate
$\operatorname {\mathrm {PGL}}(n+1)$
translate
![]() $X'$
of X and a weight vector
$X'$
of X and a weight vector
![]() $\omega \in \mathbb {R}^{n+1}$
such that the initial ideal
$\omega \in \mathbb {R}^{n+1}$
such that the initial ideal
of the ideal
![]() $I(X')\subseteq \mathbb {K}[x_0,\ldots ,x_n]$
of
$I(X')\subseteq \mathbb {K}[x_0,\ldots ,x_n]$
of
![]() $X'$
is a prime binomial ideal.
$X'$
is a prime binomial ideal.
We can now state our result.
Theorem 1.2 Let
![]() $d,n\in \mathbb {N}$
. There is a non-empty Zariski open subset U of the linear system of degree d hypersurfaces in
$d,n\in \mathbb {N}$
. There is a non-empty Zariski open subset U of the linear system of degree d hypersurfaces in
![]() $\mathbb {P}^n$
with the property that every hypersurface in U admits a toric Gröbner degeneration up to change of coordinates if and only if
$\mathbb {P}^n$
with the property that every hypersurface in U admits a toric Gröbner degeneration up to change of coordinates if and only if
![]() $d\leq 2n-1$
.
$d\leq 2n-1$
.
Before proving this theorem in the following section, we discuss connections to the existing literature.
A common source of toric degenerations of a projective variety
![]() $X\subset \mathbb {P}^n$
arises by considering the Rees algebra associated with a full-rank homogeneous valuation
$X\subset \mathbb {P}^n$
arises by considering the Rees algebra associated with a full-rank homogeneous valuation
![]() $\mathfrak {v}$
on the homogeneous coordinate ring of X [Reference AndersonAnd13]. As long as the homogeneous coordinate ring of X contains a finite set
$\mathfrak {v}$
on the homogeneous coordinate ring of X [Reference AndersonAnd13]. As long as the homogeneous coordinate ring of X contains a finite set
![]() $\mathcal {S}$
whose valuations generate the value semigroup of
$\mathcal {S}$
whose valuations generate the value semigroup of
![]() $\mathfrak {v}$
, one obtains a toric degeneration. Such a set
$\mathfrak {v}$
, one obtains a toric degeneration. Such a set
![]() $\mathcal {S}$
is called a finite Khovanskii basis for the coordinate ring of X. This construction is in fact quite general: essentially any
$\mathcal {S}$
is called a finite Khovanskii basis for the coordinate ring of X. This construction is in fact quite general: essentially any
![]() $\mathbb {G}_m$
-equivariant degeneration of X over
$\mathbb {G}_m$
-equivariant degeneration of X over
![]() $\mathbb {A}^1$
arises by this construction (see [Reference Kaveh, Manon and MurataKMM23, Theorem 1.11] for a precise statement). There has been some work on algorithmically constructing valuations with finite Khovanskii bases (see, e.g., [Reference Bossinger, Lamboglia, Mincheva, Mohammadi, Smith and SturmfelsBLMM17] for applications to degenerations of certain flag varieties), but as of yet, there is no general effective criterion for deciding when such a valuation exists.
$\mathbb {A}^1$
arises by this construction (see [Reference Kaveh, Manon and MurataKMM23, Theorem 1.11] for a precise statement). There has been some work on algorithmically constructing valuations with finite Khovanskii bases (see, e.g., [Reference Bossinger, Lamboglia, Mincheva, Mohammadi, Smith and SturmfelsBLMM17] for applications to degenerations of certain flag varieties), but as of yet, there is no general effective criterion for deciding when such a valuation exists.
Drawing on [Reference Kaveh and ManonKM19] which connects Khovanskii bases and tropical geometry, we may rephrase our results in the language of Khovanskii bases. It is straightforward to show that X admits a toric Gröbner degeneration up to change of coordinates if and only if there is some full-rank homogeneous valuation
![]() $\mathfrak {v}$
for which the homogeneous coordinate ring has a finite Khovanskii basis consisting of degree one elements. Thus, our theorem shows the existence of finite Khovanskii bases for general hypersurfaces of degree at most
$\mathfrak {v}$
for which the homogeneous coordinate ring has a finite Khovanskii basis consisting of degree one elements. Thus, our theorem shows the existence of finite Khovanskii bases for general hypersurfaces of degree at most
![]() $2n-1$
, and shows that any finite Khovanskii basis for a general hypersurface of larger degree necessarily contains elements of degrees larger than one. In fact, we suspect that a general hypersurface of sufficiently large degree does not admit any finite Khovanskii basis at all.
$2n-1$
, and shows that any finite Khovanskii basis for a general hypersurface of larger degree necessarily contains elements of degrees larger than one. In fact, we suspect that a general hypersurface of sufficiently large degree does not admit any finite Khovanskii basis at all.
We note in passing that a general hypersurface of arbitrary degree will admit a toric degeneration in a weaker sense. Indeed, the universal hypersurface over the linear system of degree d hypersurfaces is a flat family, and for any degree d, there is a toric hypersurface of degree d. However, such a degeneration is not
![]() $\mathbb {G}_m$
-equivariant.
$\mathbb {G}_m$
-equivariant.
An interesting comparison of our result can be made with [Reference Kaveh, Manon and MurataKMM21], which states that after a generic change of coordinates, any arithmetically Cohen Macaulay variety
![]() $X\subset \mathbb {P}^n$
has a Gröbner degeneration to a (potentially non-normal) variety equipped with an effective action of a codimension-one torus. Such varieties, called complexity-one T-varieties, are in a sense one step away from being toric. The hypersurfaces we consider in our main result (Theorem 1.2) are of course arithmetically Cohen Macaulay, so they admit Gröbner degenerations to complexity-one T-varieties. Our result characterizes when we can go one step further and Gröbner degenerate to something toric. When
$X\subset \mathbb {P}^n$
has a Gröbner degeneration to a (potentially non-normal) variety equipped with an effective action of a codimension-one torus. Such varieties, called complexity-one T-varieties, are in a sense one step away from being toric. The hypersurfaces we consider in our main result (Theorem 1.2) are of course arithmetically Cohen Macaulay, so they admit Gröbner degenerations to complexity-one T-varieties. Our result characterizes when we can go one step further and Gröbner degenerate to something toric. When
![]() $d\leq 2n-1$
and we are in the range for which this is possible for a generic hypersurface, the change of coordinates required is a special one as opposed to the generic change of coordinates of [Reference Kaveh, Manon and MurataKMM21].
$d\leq 2n-1$
and we are in the range for which this is possible for a generic hypersurface, the change of coordinates required is a special one as opposed to the generic change of coordinates of [Reference Kaveh, Manon and MurataKMM21].
2 Proof of the theorem
2.1 Setup
Throughout, we will assume that
![]() $d,n>1$
since the theorem is clearly true if
$d,n>1$
since the theorem is clearly true if
![]() $d=1$
or
$d=1$
or
![]() $n=1$
. We will view the coefficients
$n=1$
. We will view the coefficients
![]() $c_u$
of f in (1.1) as coordinates on affine space
$c_u$
of f in (1.1) as coordinates on affine space
![]() $\mathbb {A}^{d+n \choose n}$
. To indicate the dependence of f on the choice of coefficients c, we will often write
$\mathbb {A}^{d+n \choose n}$
. To indicate the dependence of f on the choice of coefficients c, we will often write
![]() $f=f_c$
. Let K be the subset of all
$f=f_c$
. Let K be the subset of all
![]() $u\in \mathbb {Z}_{\geq 0}^{n+1}$
such that
$u\in \mathbb {Z}_{\geq 0}^{n+1}$
such that
![]() $u_0+u_1=d$
,
$u_0+u_1=d$
,
![]() $u_i=0$
for
$u_i=0$
for
![]() $i>1$
, and
$i>1$
, and
![]() $u_1<d$
. We then set
$u_1<d$
. We then set
The family of polynomials parameterized by W consists of all degree d forms such that the only monomial involving only
![]() $x_0$
and
$x_0$
and
![]() $x_1$
is
$x_1$
is
![]() $x_1^d$
.
$x_1^d$
.
We will be considering the map
 $$ \begin{align*} \phi:\operatorname{\mathrm{GL}}(n+1)\times W&\to \mathbb{K}[x_0,\ldots,x_n]_d\\ (A,c)&\mapsto A.f_c, \end{align*} $$
$$ \begin{align*} \phi:\operatorname{\mathrm{GL}}(n+1)\times W&\to \mathbb{K}[x_0,\ldots,x_n]_d\\ (A,c)&\mapsto A.f_c, \end{align*} $$
where
![]() $A.f_c$
denotes the action of
$A.f_c$
denotes the action of
![]() $A\in \operatorname {\mathrm {GL}}(n+1)$
on a polynomial
$A\in \operatorname {\mathrm {GL}}(n+1)$
on a polynomial
![]() $f_c=\sum c_ux^u$
via linear change of coordinates. We will be especially interested in the differential of
$f_c=\sum c_ux^u$
via linear change of coordinates. We will be especially interested in the differential of
![]() $\phi $
at
$\phi $
at
![]() $(e,c)$
, where
$(e,c)$
, where
![]() $e\in \operatorname {\mathrm {GL}}(n+1)$
is the identity. A straightforward computation shows that the image of the differential at
$e\in \operatorname {\mathrm {GL}}(n+1)$
is the identity. A straightforward computation shows that the image of the differential at
![]() $(e,c)$
is generated by
$(e,c)$
is generated by
The following lemma is the key to our proof.
Lemma 2.1 The differential
![]() $\phi $
is surjective at
$\phi $
is surjective at
![]() $(e,c)$
for general
$(e,c)$
for general
![]() $c\in W$
if and only if
$c\in W$
if and only if
![]() $d\leq 2n-1$
.
$d\leq 2n-1$
.
Proof Consider the image of the differential of
![]() $\phi $
at
$\phi $
at
![]() $(e,c)$
. From (2.1), we obtain the span of all monomials of
$(e,c)$
. From (2.1), we obtain the span of all monomials of
![]() $\mathbb {K}[x_0,\ldots ,x_n]_d$
with the exceptions of the d monomials
$\mathbb {K}[x_0,\ldots ,x_n]_d$
with the exceptions of the d monomials
![]() $x_0^d,x_0^{d-1}x_1,\ldots ,x_0x_1^{d-1}$
. From (2.2) with
$x_0^d,x_0^{d-1}x_1,\ldots ,x_0x_1^{d-1}$
. From (2.2) with
![]() $i=1$
and
$i=1$
and
![]() $j=0$
, modulo (2.1), we additionally obtain the monomial
$j=0$
, modulo (2.1), we additionally obtain the monomial
![]() $x_0x_1^{d-1}$
. We do not obtain anything new from (2.2) when
$x_0x_1^{d-1}$
. We do not obtain anything new from (2.2) when
![]() $i=1$
and
$i=1$
and
![]() $j=1$
, when
$j=1$
, when
![]() $i=0$
, or when
$i=0$
, or when
![]() $j>1$
.
$j>1$
.
It remains to consider the contributions to the image from (2.2) with
![]() $i>1$
and
$i>1$
and
![]() $j=0,1$
. For
$j=0,1$
. For
![]() $2\leq i \leq n$
and
$2\leq i \leq n$
and
![]() $1\leq m\leq d-1$
, let
$1\leq m\leq d-1$
, let
![]() $u(i,m)\in \mathbb {Z}^{n+1}$
be the exponent vector with
$u(i,m)\in \mathbb {Z}^{n+1}$
be the exponent vector with
![]() $u_i=1$
,
$u_i=1$
,
![]() $u_0=m$
,
$u_0=m$
,
![]() $u_1=d-m-1$
. Modulo the span of (2.1) and
$u_1=d-m-1$
. Modulo the span of (2.1) and
![]() $x_0x_1^{d-1}$
, from (2.2), we obtain
$x_0x_1^{d-1}$
, from (2.2), we obtain
 $$ \begin{align*} \frac{\partial f_c}{\partial x_i}\cdot x_0\equiv &c_{u(i,d-1)}x_0^d+c_{u(i,d-2)}x_0^{d-1}x_1+\cdots+c_{u(i,1)}x_0^2x_1^{d-2},\\ \frac{\partial f_c}{\partial x_i}\cdot x_1\equiv &\phantom{c_{u(i,d-1)}x_0^d+}c_{u(i,d-1)}x_0^{d-1}x_1+\cdots+c_{u(i,2)}x_0^2x_1^{d-2}. \end{align*} $$
$$ \begin{align*} \frac{\partial f_c}{\partial x_i}\cdot x_0\equiv &c_{u(i,d-1)}x_0^d+c_{u(i,d-2)}x_0^{d-1}x_1+\cdots+c_{u(i,1)}x_0^2x_1^{d-2},\\ \frac{\partial f_c}{\partial x_i}\cdot x_1\equiv &\phantom{c_{u(i,d-1)}x_0^d+}c_{u(i,d-1)}x_0^{d-1}x_1+\cdots+c_{u(i,2)}x_0^2x_1^{d-2}. \end{align*} $$
Varying i from
![]() $2$
to n, we obtain
$2$
to n, we obtain
![]() $2n-2$
polynomials of degree d. The
$2n-2$
polynomials of degree d. The
![]() $(2n-2)\times (d-1)$
matrix of their coefficients has the form
$(2n-2)\times (d-1)$
matrix of their coefficients has the form
 $$\begin{align*}\left(\begin{array}{c c c c c} c_{u(2,d-1)} &c_{u(2,d-2)} &\ldots &c_{u(2,1)}\\ 0 &c_{u(2,d-1)} &\ldots &c_{u(2,2)}\\ c_{u(3,d-1)} &c_{u(3,d-2)} &\ldots &c_{u(3,1)}\\ 0 &c_{u(3,d-1)} &\ldots & c_{u(3,2)}\\ \vdots&\vdots&&\vdots\\ c_{u(n,d-1)}&c_{u(n,d-2)}&\ldots &c_{u(n,1)}\\ 0&c_{u(n,d-1)}&\ldots &c_{u(n,2)} \end{array} \right). \end{align*}$$
$$\begin{align*}\left(\begin{array}{c c c c c} c_{u(2,d-1)} &c_{u(2,d-2)} &\ldots &c_{u(2,1)}\\ 0 &c_{u(2,d-1)} &\ldots &c_{u(2,2)}\\ c_{u(3,d-1)} &c_{u(3,d-2)} &\ldots &c_{u(3,1)}\\ 0 &c_{u(3,d-1)} &\ldots & c_{u(3,2)}\\ \vdots&\vdots&&\vdots\\ c_{u(n,d-1)}&c_{u(n,d-2)}&\ldots &c_{u(n,1)}\\ 0&c_{u(n,d-1)}&\ldots &c_{u(n,2)} \end{array} \right). \end{align*}$$
Since
![]() $c\in W$
is general, this matrix has full rank, that is, its rank is
$c\in W$
is general, this matrix has full rank, that is, its rank is
![]() $\min \{d-1, 2n-2\}$
. Hence, the image of the differential of
$\min \{d-1, 2n-2\}$
. Hence, the image of the differential of
![]() $\phi $
has codimension
$\phi $
has codimension
so the differential is surjective if and only if
![]() $d\leq 2n-1$
.
$d\leq 2n-1$
.
We now move on to prove the theorem.
2.2 Existence
We will first show that if
![]() $d\leq 2n-1$
, a general degree d hypersurface admits a toric Gröbner degeneration up to change of coordinates. As noted above, the family of polynomials parameterized by W consists of all degree d forms such that the only monomial involving only
$d\leq 2n-1$
, a general degree d hypersurface admits a toric Gröbner degeneration up to change of coordinates. As noted above, the family of polynomials parameterized by W consists of all degree d forms such that the only monomial involving only
![]() $x_0$
and
$x_0$
and
![]() $x_1$
is
$x_1$
is
![]() $x_1^d$
. Consider any
$x_1^d$
. Consider any
![]() $\omega \in \mathbb {R}^{n+1}$
such that
$\omega \in \mathbb {R}^{n+1}$
such that
For general
![]() $c\in W$
, the initial term of
$c\in W$
, the initial term of
![]() $f_{c}$
is
$f_{c}$
is
for some
![]() $a,b\neq 0$
; this is a prime binomial. Thus, we will be done with our first claim if we can show that the image of
$a,b\neq 0$
; this is a prime binomial. Thus, we will be done with our first claim if we can show that the image of
![]() $\phi $
contains a non-empty open subset of
$\phi $
contains a non-empty open subset of
![]() $\mathbb {K}[x_0,\ldots ,x_n]_d$
.
$\mathbb {K}[x_0,\ldots ,x_n]_d$
.
To this end, we consider the image of the differential at
![]() $(e,c)$
for general
$(e,c)$
for general
![]() $c\in W$
. By Lemma 2.1, we conclude that
$c\in W$
. By Lemma 2.1, we conclude that
![]() $\phi $
has surjective differential at
$\phi $
has surjective differential at
![]() $(e,c)$
for general
$(e,c)$
for general
![]() $c\in W$
; it follows that
$c\in W$
; it follows that
![]() $\phi $
has surjective differential at a general point of
$\phi $
has surjective differential at a general point of
![]() $\operatorname {\mathrm {GL}}(n+1)\times W$
. Thus, the dimension of the image of
$\operatorname {\mathrm {GL}}(n+1)\times W$
. Thus, the dimension of the image of
![]() $\phi $
is the dimension of
$\phi $
is the dimension of
![]() $\mathbb {K}[x_0,\ldots ,x_n]_d$
, and the image of
$\mathbb {K}[x_0,\ldots ,x_n]_d$
, and the image of
![]() $\phi $
contains a non-empty open subset of
$\phi $
contains a non-empty open subset of
![]() $\mathbb {K}[x_0,\ldots ,x_n]_d$
.
$\mathbb {K}[x_0,\ldots ,x_n]_d$
.
2.3 Nonexistence
Assume now that
![]() $d>2n-1$
. We first give an overview of the proof strategy. There are only finitely many prime binomials g of degree d. Likewise, there are only finitely many linear orderings
$d>2n-1$
. We first give an overview of the proof strategy. There are only finitely many prime binomials g of degree d. Likewise, there are only finitely many linear orderings
![]() $\prec $
of the variable indices
$\prec $
of the variable indices
![]() $0,\ldots ,n$
. We say that a weight vector
$0,\ldots ,n$
. We say that a weight vector
![]() $\omega $
is compatible with
$\omega $
is compatible with
![]() $\prec $
and g if whenever
$\prec $
and g if whenever
![]() $i\prec j$
in the linear ordering, then
$i\prec j$
in the linear ordering, then
![]() $\omega _i\geq \omega _j$
, and the two monomials of g have the same weight with respect to
$\omega _i\geq \omega _j$
, and the two monomials of g have the same weight with respect to
![]() $\omega $
.
$\omega $
.
For fixed g and linear ordering on the variables, we may consider the set S of all polynomials f in
![]() $\mathbb {K}[x_0,\ldots ,x_n]_d$
for which there exists a compatible weight vector
$\mathbb {K}[x_0,\ldots ,x_n]_d$
for which there exists a compatible weight vector
![]() $\omega \in \mathbb {R}^{n+1}$
such that initial term of f with respect to
$\omega \in \mathbb {R}^{n+1}$
such that initial term of f with respect to
![]() $\omega $
is g. We will show that up to permutation of the coordinates, this set S can be identified as a subfamily of W. By Lemma 2.1, the map
$\omega $
is g. We will show that up to permutation of the coordinates, this set S can be identified as a subfamily of W. By Lemma 2.1, the map
![]() $\phi $
has nowhere surjective differential. Thus, by generic smoothness, the dimension of the image of
$\phi $
has nowhere surjective differential. Thus, by generic smoothness, the dimension of the image of
![]() $\phi $
must be strictly less than the dimension of
$\phi $
must be strictly less than the dimension of
![]() $\mathbb {K}[x_0,\ldots ,x_n]_d$
. It follows that there cannot be a Zariski-open subset of
$\mathbb {K}[x_0,\ldots ,x_n]_d$
. It follows that there cannot be a Zariski-open subset of
![]() $\mathbb {K}[x_0,\ldots ,x_n]_d$
such that every hypersurface in this subset admits a toric Gröbner degeneration up to change of coordinates.
$\mathbb {K}[x_0,\ldots ,x_n]_d$
such that every hypersurface in this subset admits a toric Gröbner degeneration up to change of coordinates.
To complete the proof, we will fix a prime binomial
![]() $g=g'+g''$
of degree d and a linear ordering of the variables. Here,
$g=g'+g''$
of degree d and a linear ordering of the variables. Here,
![]() $g'$
and
$g'$
and
![]() $g''$
are the two terms of g. After permuting the variables and appropriately adapting g, we may assume without loss of generality that the indices are ordered as
$g''$
are the two terms of g. After permuting the variables and appropriately adapting g, we may assume without loss of generality that the indices are ordered as
![]() $0\prec 1 \prec 2 \prec \cdots \prec n$
. The irreducibility of g implies that g involves at least three distinct variables, and no variable appears in both
$0\prec 1 \prec 2 \prec \cdots \prec n$
. The irreducibility of g implies that g involves at least three distinct variables, and no variable appears in both
![]() $g'$
and
$g'$
and
![]() $g''$
. Let p be the smallest index such that
$g''$
. Let p be the smallest index such that
![]() $x_p$
appears in g; we denote the corresponding term by
$x_p$
appears in g; we denote the corresponding term by
![]() $g'$
. Let q be the smallest index such that
$g'$
. Let q be the smallest index such that
![]() $x_q$
appears in the term
$x_q$
appears in the term
![]() $g''$
.
$g''$
.
If
![]() $g'$
only involves variables
$g'$
only involves variables
![]() $x_i$
with indices
$x_i$
with indices
![]() $i<q$
, then any compatible term order
$i<q$
, then any compatible term order
![]() $\omega $
must satisfy
$\omega $
must satisfy
![]() $\omega _p=\omega _q=\omega _j$
for all
$\omega _p=\omega _q=\omega _j$
for all
![]() $p\leq j \leq q$
. Indeed, if not, the term
$p\leq j \leq q$
. Indeed, if not, the term
![]() $g''$
would necessarily have smaller weight. Without loss of generality, we may thus permute indices without changing the set of compatible weight vectors to also assume that
$g''$
would necessarily have smaller weight. Without loss of generality, we may thus permute indices without changing the set of compatible weight vectors to also assume that
![]() $g'$
involves some
$g'$
involves some
![]() $x_i$
with
$x_i$
with
![]() $i>q$
. For this, we are using that the irreducibility of g guarantees that at least one of
$i>q$
. For this, we are using that the irreducibility of g guarantees that at least one of
![]() $g'$
and
$g'$
and
![]() $g''$
is not a dth power.
$g''$
is not a dth power.
Consider the set S of polynomials
![]() $f_c$
such that there is a compatible weight
$f_c$
such that there is a compatible weight
![]() $\omega $
for which
$\omega $
for which
![]() $f_c$
has g as its initial term. We claim that S is a subset of the family parameterized by
$f_c$
has g as its initial term. We claim that S is a subset of the family parameterized by
![]() $W.$
Indeed, since
$W.$
Indeed, since
![]() $q>0$
,
$q>0$
,
![]() $g''$
has weight at most equal to the weight of
$g''$
has weight at most equal to the weight of
![]() $x_1^d$
. The monomials
$x_1^d$
. The monomials
![]() $x_0^d,x_0^{d-1}x_1,\ldots ,x_0x_1^{d-1}$
all have weight at least as big as the weight of
$x_0^d,x_0^{d-1}x_1,\ldots ,x_0x_1^{d-1}$
all have weight at least as big as the weight of
![]() $x_1^d$
, and are not scalar multiples of
$x_1^d$
, and are not scalar multiples of
![]() $g'$
or
$g'$
or
![]() $g''$
. Hence, none of these monomials can appear in any element of S, and the claim follows.
$g''$
. Hence, none of these monomials can appear in any element of S, and the claim follows.
The proof of the theorem now follows from the argument given above.