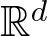Decomposability of multiparameter car flows

Abstract

Let P be a closed convex cone in $\mathbb{R}^d$ which is assumed to be spanning $\mathbb{R}^d$ and contains no line. In this article, we consider a family of CAR flows over P and study the decomposability of the associated product systems. We establish a necessary and sufficient condition for CAR flow to be decomposable. As a consequence, we show that there are uncountable many CAR flows which are cocycle conjugate to the corresponding CCR flows.

1. Introduction

Powers introduced the theory of one-parameter E ₀-semigroups in [8]. A one-parameter E ₀-semigroup is a weak $*$-continuous semigroup $\alpha=\{\alpha_t\}_{t\geq 0}$ of unital normal $*$-endomorphisms on a von Neumann algebra M. After the introduction of E ₀-semigroups over $\mathbb{R}_+$, the theory quickly grew with a lot of interesting structures and many surprises. The classification of E ₀-semigroups is far from being complete and remains mysterious even for the case of E ₀-semigroups over $\mathbb{R}_+$ on B(H). Arveson introduced the notion of product systems to study the theory of E ₀-semigroups on the $*$-algebra B(H) of all bounded operators on a separable Hilbert space H. He showed that the classification of E ₀-semigroups on a type I factor, up to cocycle conjugacy, is equivalent to the classification of product systems up to isomorphism. E ₀-semigroups on type I factors are roughly divided into three types, namely types I, II and III. In the beginning, the work progressed by studying CCR and CAR flows and trying to classify them up to cocycle conjugacy. Later, it was shown that CCR flows and CAR flows are cocycle conjugate [9], so while Powers worked on CAR flows and Arveson worked on CCR flows, this was a matter of convenience or perhaps even taste. Arveson proved that they were classified by the Powers–Arveson index, and not long afterward, Arveson proved that all decomposable E ₀-semigroups are cocycle conjugate to CAR or CCR flows with the same Powers–Arveson index. In the one-parameter case, there exists only countable many CCR flows (see [4]). However, in the multiparameter context, there are uncountable many CCR flows over a closed convex cone P [2, 3, 12]. The multiparameter CCR flows are decomposable (see [12]). It was shown in [10] that for certain P-spaces, CCR flows are not cocycle conjugate to the corresponding CAR flows. Let P be a closed convex cone in $\mathbb{R}^d$, and let V be an isometric representation of P. Denote by α the CAR flow associated to the isometric representation V and by E the product system associated to α. A natural question that arises is the following. Under what condition on the isometric representation V, the corresponding product system E is decomposable $?$. In this paper, we answer this for the isometric representations given by P-spaces. More precisely, we show that the CAR flow associated to a P-space is decomposable if and only if the P-space is a half-space.

The organization of the paper is as follows. In § 2, we recall a few definitions that are required to study non-commutative stochastic calculus. We define the notion of additive decomposable section, and using that we define a non-commutative Itô integral of an adapted process with respect to a centred additive decomposable section (a generalization of the non-commutative Itô integral with respect to a centred addit considered in [6]). By building the necessary tools, we obtain a centred coherent section from a centred additive decomposable section by solving the quantum stochastic integral equation, and on the other hand, we obtain a centred additive decomposable section as a logarithm of a given centred coherent section by an appropriate limit; see Proposition 3 and Proposition 4. We establish a bijective correspondence between the set of all additive decomposable sections and the set of all coherent sections for the product system over $\mathbb{R}_+$, where the product system is assumed to have a coherent section. This section may be of independent interest for some specialists in quantum stochastic differential equations. In § 3, for a fixed P-space and a ray in a closed convex cone P, by using the bijective correspondence obtained in § 1, we compute the decomposable vectors of the CAR flow along the ray. In § 4, the homeomorphism map given in Proposition 7 involving the boundary of P-space A and the interior of A, together with the description of the boundary of P-space A given in Lemma 7, provide us a useful tool to study the geometry of the space A when the product system for the CAR flow associated to A is decomposable (see Theorem 3). We show that there are uncountable many CCR flows that are cocycle conjugate to CAR flows over a closed convex cone.

2. Non-commutative stochastic calculus

The notion of addits was introduced independently in [6] and [5]. The sole purpose of this section is to record for future reference that the bijection between addits and units established in [6] works equally well to provide a bijection between the set of all centred coherent sections and the set of all centred additive decomposable sections, and we do not claim much originality. As an immediate application, we obtain another proof for the fact that e-logarithm of a coherent section $e=(e_t)_{t\in \mathbb{R}_+}$ is positive definite. We leave it to the reader for the proof of many results, and we only provide the proof for the necessary places. We also adapt most of the notation from [6].

Let $E=\{(t,\xi): t\geq 0, \; \xi\in E_t\}$ be a product system over $\mathbb{R}_+$, and in short, we write $E=\bigcup_{t\geq 0}E_t$. A family $\{x_s:0\leq s\lt t\}$ of vectors is said to be left coherent if each $x_s\in E_s$ and for $0\leq r\lt s\lt t$, there exists a vector $x(r,s)\in E_{s-r}$ such that $x_s=x_rx(r,s).$ A left coherent section of E is a left coherent family $\{x_s:0\leq s\lt t\}$ with $t=\infty$ (similarly we also have the notion of right coherent section). Now onwards we simply call the left coherent family a coherent family. Then the family $\{x(r,s):0\leq r\lt s\lt t\}$ satisfies $x(q,r)x(r,s)=x(q,s),\text{for every } 0\leq q\lt r\lt s.$ We assume that all the product system in our discussion will have a coherent section. Fix a coherent section $\Omega=(\Omega_t)_{t\geq 0}$ along with the product system E such that $\|\Omega_t\|=1$ for $t\geq 0$. For a fixed $r\in \mathbb{R}_+$, let $\widetilde{\Omega}_{t}=\Omega(r,r+t)$ for $t\geq 0.$ Note that $\widetilde{\Omega}=(\widetilde{\Omega}_t)_{t\in\mathbb{R}_+}$ defines a coherent section. A coherent section $(x_t)_{t\in \mathbb{R}_+}$ is called a centred coherent section with respect to Ω (or simply a centred coherent section) if $\langle x_t|\Omega_t\rangle =1$, for each $t\geq 0$.

Definition 1. Let $\Omega=\{\Omega_s:0\leq s\lt t\}$ be a coherent family. A family $\{b_s: 0\leq s\lt t\}$ of vectors is said to be a left additive decomposable family with respect to Ω if for any $0\leq r\lt s\lt t,$ there exists a vector $b(r,s)\in E_{s-r}$ such that

\begin{equation*}b_r\otimes \Omega(r,s)+ \Omega_r\otimes b(r,s)=b_s.\end{equation*}

We simply say $\{b_t: 0\leq s\lt t\}$ is an additive decomposable family if Ω is clear from the context. If in addition the family $\{b_s: 0\leq s\lt t\}$ satisfies $\langle b_s| \Omega_s\rangle =0$ for each $0\leq s\lt t.$ We say that the family $\{b_s:0\leq s\lt t\}$ is a centred additive decomposable family.

Every additive decomposable family $\{b_s:0\leq s\lt t\}$ has the following decomposition: For $s\geq 0$, $b_s=c_s+\langle b_s|\Omega_s\rangle \Omega_s$, where $\{c_s:0\leq s\lt t\}$ is a unique centred additive decomposable family. We observe that the family $\{b(r,s):0\leq r\lt s\lt t\}$ satisfies $b(q,s)=b(q,r)\otimes \Omega(r,s)+\Omega(q,r)\otimes b(r,s)$ for every $0\leq q\lt r\lt s.$ If an additive decomposable family $\{b_s:0\leq s\lt t\}$ is centred, we also have $\langle b(r,s)| \Omega(r,s)\rangle=0 $ for every $0\leq r\lt s\lt t.$ An additive decomposable section is an additive decomposable family $\{b_s:0\leq s\lt t\}$ with $t=\infty$.

Lemma 1. Let $\Omega=\{\Omega_s:0\leq s\lt t\}$ be a left coherent family with $\|\Omega_s\|=1$ for each s, and let $b=\{b_s:0\leq s\lt t \}$ and $c=\{c_s:0\leq s\lt t \}$ be centred left additive decomposable families with respect to Ω. Then $\displaystyle\lim_{h\to 0^+}\langle b_h|c_h\rangle=0.$

Proof. For $0\lt r\lt s$, define a map $L_{r}:E_{s-r}\to E_{s}$ by $L_r(\xi)=\Omega_r\xi$ for each $\xi \in E_{s-r}$. Then L _r is an isometry. Denote the range projection $L_rL_r^*$ of L _r by P _r, that is, we can view $P_r=\langle .|\Omega_r\rangle\Omega_r \otimes 1_{E_{s-r}}:E_r\otimes E_{s-r}\to E_r\otimes E_{s-r}$. Then P _r strongly converges to 1 as r decreases to zero (see [4, Theorem 6.1.1] for the proof of this fact). Let $0\lt h\lt s\lt t$. We observe that $\|b_s\|^2=\|b_h\|^2+\|b(h,s)\|^2$, and we have

\begin{align*} \|b_s\|^2 &=\displaystyle \lim_{h\to 0^+}\| P_h(b_s)\|^2\\ &=\displaystyle \lim_{h\to 0^+}\|P_h(b_h\otimes \Omega(h,s)+\Omega_h \otimes b(h,s) )\|\\ &=\displaystyle \lim_{h\to 0^+}\|b(h,s) )\|\\ &=\displaystyle \lim_{h\to 0^+}\left(\|b_s\|^2-\|b_h\|^2\right). \end{align*}

From the above, we conclude that $\displaystyle\lim_{h\to 0^+}\| b_h\|^2=0$, and hence $\displaystyle\lim_{h\to 0^+}\langle b_h|c_h\rangle=0$.

Proposition 1. Let $\{b_s:0\lt s\lt t\}$ and $\{c_s:0\lt s\lt t\}$ be centred additive decomposable families. Then the map $(0,t)\ni s\mapsto \langle b_s | c_s\rangle \in \mathbb{C}$ is continuous.

Proof. Let $s_0\in (0,t)$ be given. We show that the map $ (0,t)\ni s\mapsto \langle b_s |c_s\rangle \in \mathbb{C}$ is both left and right continuous at s ₀. For any $0\lt h\lt t-s_0$, we observe that

\begin{align*} \langle b_{s_0+h}|c_{s_0+h} \rangle&=\langle b_{s_0}|c_{s_0} \rangle+\langle b(s_0,s_0+h)|c(s_0,s_0+h) \rangle\\ &=\langle b_{s_0}|c_{s_0} \rangle+\langle \widetilde{b}_h|\widetilde{c}_h \rangle, \end{align*}

where $\widetilde{b}_{h}=b(s_0,s_0+h)$ and $\widetilde{c}_{h}=c(s_0,s_0+h)$. Then the sets $\{\widetilde{b}_{r}:0\lt r\lt t-s_0\}$ and $\{\widetilde{c}_{r}:0\lt r\lt t-s_0\}$ form centred left additive decomposable families. Indeed, for $0\lt q\lt r\lt t-s_0$, we have

\begin{equation*}\widetilde{b}_r=\widetilde{b}_{q}\otimes \widetilde{\Omega}(q,r)+\widetilde{\Omega}_{q}\otimes \widetilde{b}(q,r),\end{equation*}

where $\widetilde{\Omega}(q,r)=\Omega(s_0+q,s_0+r)$, $\widetilde{\Omega}_q=\Omega(s_0,s_0+q)$ and $\widetilde{b}(q,r)=b(s_0+q,s_0+r)$. Similarly, $ \{\widetilde{c}_{r}:0\lt r\lt t-s_0\}$ forms a centred left additive decomposable family. By Lemma 1, $\displaystyle\lim_{h\to 0^+}\langle \widetilde{b}_h |\widetilde{c}_h\rangle =0$. Hence, the equation $\langle b_{s_0+h}|c_{s_0+h} \rangle =\langle b_{s_0}|c_{s_0} \rangle+\langle \widetilde{b}_h|\widetilde{c}_h\rangle$ implies that $\langle b_{s_0+h}|c_{s_0+h} \rangle \to\langle b_{s_0}|c_{s_0} \rangle$ as h goes to zero. This means that the map $(0,t)\ni s\mapsto \langle b_s|c_s\rangle \in \mathbb{C}$ is right continuous.

For $0\lt h\lt s_0$, let $b^\prime:=\{b^\prime_s=b(s_0-s,s_0): 0\lt s\lt s_0\}$ and $\Omega^\prime:=\{\Omega^\prime_s=\Omega(s_0-s,s_0): 0\lt s\lt s_0\}$. Note that $\Omega^\prime$ is a right coherent family. We leave it to the reader to verify that b ^ʹ is a centred right additive decomposable family with respect to $\Omega^\prime$ for the product system E. In the opposite product system E ^op, $\Omega^\prime$ is a left coherent family and b ^ʹ is a centred left additive decomposable family with respect to $\Omega^\prime$. Similar to the above argument, we see that $\langle b_{s_0-h}|c_{s_0-h} \rangle\to \langle b_{s_0}|c_{s_0} \rangle$ as h goes to zero. Hence, the map $(0,t)\ni s\mapsto \langle b_s|c_s\rangle \in \mathbb{C}$ is left continuous. This proves the proposition.

Definition 2.

1. (1) By an adapted process of E, we mean a measurable map $\mathbb{R}_{+}\ni t\mapsto x_t\in E$ satisfying $x_t\in E_t$ for each $t\geq 0.$ We say that an adapted process $(x_t)_{t\in\mathbb{R}_+}$ is simple if there exists a partition $0\leq t_0\lt t_1\lt\cdots \lt t_n\lt\cdots $ of $\mathbb{R}_{+}$ such that

   (2.1)\begin{equation} x_t=\sum_{i=0}^{\infty} x_i \otimes \Omega(t_i,t) \;\chi_{[t_i,t_{i+1})}(t) \ \ {\rm for\ every }\ t\geq 0\quad {\rm and }\quad x_i\in E_{t_i}\ \ {\rm for }\ i\geq 0. \end{equation}

2. (2) Let $(x_t)_{t\in\mathbb{R}_+}$ be a simple adapted process of the form (2.1) and let $(b_t)_{t\in\mathbb{R}_+}$ be a centred additive decomposable section of E. Then the integral of a simple adapted process $(x_t)_{t\in\mathbb{R}_+}$ with respect to the centred additive decomposable section $(b_t)_{t\in\mathbb{R}_+}$ over the interval [a,b] is denoted by $\int_{a}^{b} x_t\, {\rm d}b_{t}$ and is defined as follows:

   (2.2)\begin{equation} \int_{a}^{b} x_t\,{\rm d}b_{t}=\sum_{i=m}^{n-1} x_i\otimes b(t_i, t_{i+1})\otimes \Omega(t_{i+1},b). \end{equation}

   Here we have refined the partition such that $a=t_m$ and $b=t_n$ for some $m,n\in \mathbb{N}$.

3. (3) Let $(x_t)_{t\in\mathbb{R}_+}$ be an adapted process, and let $(b_t)_{t\in\mathbb{R}_+}$ be a centred additive decomposable section of E. We say that an adapted process $(x_t)_{t\in\mathbb{R}_+}$ is Itô integrable with respect to $(b_t)_{t\in\mathbb{R}_+}$ if for every $a,b\in \mathbb{R}_+$ with a < b, there exists a sequence of simple adapted processes $\{x^{(n)}\}_{n=1}^{\infty}$ with $x^{(n)}=(x^{(n)}_t)_{t\in\mathbb{R}_+}$ such that $\{x^{(n)}\}_{n=1}^{\infty}$ converges to $x=(x_t)_{t\in\mathbb{R}_+}$ in L ²-norm on $[a, b]$ and the sequence $\int_{a}^{b} x^{(n)}_t\, {\rm d}b_{t}$ is Cauchy. In that case, we define $\int_{a}^{b} x_t\, {\rm d}b_{t}$ as follows:

   (2.3)\begin{equation} \int_{a}^{b} x_t\, {\rm d}b_{t}:=\lim_{n\to \infty}\int_{a}^{b} x^{(n)}_t\, {\rm d}b_{t}. \end{equation}

   We will see that the above integral is well-defined.

The definition for the Itô integrable adapted process is slightly different from the one considered in [6, see discussion after Proposition 5.4].

Lemma 2. Let $(x_t)_{t\in\mathbb{R}_+}$ and $(y_t)_{t\in\mathbb{R}_+}$ be Itô integrable adapted processes with respect to the centred additive decomposable section $(b_t)_{t\in\mathbb{R}_+}$. Then we have the following properties.

1. (1) For $0\leq s\leq t$, we have $\left\langle\displaystyle \int_{s}^{t} x_r\, {\rm d}b_r\Bigg| \Omega_t\right\rangle=0$.

2. (2) For $0\leq s_0\leq s$ and $t\geq 0$, $\displaystyle\int_{s_0}^{s+t}x_r\,{\rm d}b_r=\int_{s_0}^{s}x_r\,{\rm d}b_r \otimes \Omega(s,s+t)+ \int_{s}^{s+t}x_r\,{\rm d}b_r$.

3. (3) For $s,t\geq 0$, $\displaystyle\int_{s}^{s+t} x_s\otimes y_{r-s}\, {\rm d}b_r=x_s\otimes \int_{0}^{t} y_r\, {\rm d}\tilde{b}_r$.

   Here $\tilde{b}=(\tilde{b_r})_{r\geq 0}:=(b(s,s+r))_{r\geq 0}$ is a centred additive decomposable section with respect to the coherent section $\widetilde{\Omega}=(\Omega(s,s+r))_{r\geq 0}$.

4. (4) For $0\leq s_0\leq s$ and $0\leq t_0\leq t$, we have $\left\langle \displaystyle\int_{s+t_0}^{s+t} x_r\, {\rm d}b_r\Bigg| \int_{s_0}^{s} x_r\,{\rm d}b_r \otimes \Omega(s,s+t)\right\rangle=0.$

5. (5) For $s,t\geq 0$, $ \displaystyle\int_{s}^{s+t} \Omega_r\, {\rm d}b_r=b_{s+t}-b_s\otimes \Omega(s,s+t).$

Proof. By definition, it is enough to prove the above results for a simple adapted process and we leave it to the reader’s verification.

Let $(b_t)_{t\in \mathbb{R}_+}$ be a centred additive decomposable section. Define a map $F:\mathbb{R}\to \mathbb{R}$ by

\begin{equation*}F(s):=\begin{cases} \|b_s\|^2 & \mbox{if } s\gt0,\cr 0 & \mbox{if } s\leq 0. \end{cases} \end{equation*}

Since F is a non-decreasing right continuous function on $\mathbb{R}$, there exists a unique Borel measure µ on $\mathbb{R}$ such that $\mu((-\infty,s])=F(s)$ for $s\in \mathbb{R}$. For simple adapted processes $(x_t)_{t\in \mathbb{R}_+}$ and $(y_t)_{t\in \mathbb{R}_+}$, we have

(2.4)\begin{equation} \left\langle \int_{s}^{t} x_r\, {\rm d}b_r\Bigg| \int_{s}^{t} y_r\, {\rm d}b_r\right\rangle=\int_{s}^{t} \langle x_r|y_r \rangle\, {\rm d}\mu(r). \end{equation}

We can see using the above equality that Equation (2.3) is well-defined. With this result, we have the following lemma, which can also be thought of as a version of the Itô identity.

Lemma 3. Let $(x_t)_{t\in \mathbb{R}_+}$ and $(y_t)_{t\in \mathbb{R}_+}$ be Itô integrable adapted processes, and let $(b_t)_{t\in \mathbb{R}_+}$ be a centred additive decomposable section. Then we have

(2.5)\begin{equation} \left\langle \int_{s}^{t} x_r\, {\rm d}b_r\Bigg| \int_{s}^{t} y_r\,{\rm d}b_r\right\rangle=\int_{s}^{t} \langle x_r|y_r \rangle\, {\rm d}\mu(r). \end{equation}

The following proposition provides a condition for a continuous adapted process to be Itô integrable, and the proof follows from Lemma 3.

Proposition 2. Let $(b_t)_{t\in \mathbb{R}_+}$ be a centred additive decomposable section, and let $x=(x_t)_{t\in \mathbb{R}_+}$ be a continuous adapted process such that

(2.6)\begin{equation} \langle x_{r+s}|x_r\otimes \Omega(r,r+s)\rangle =\|x_r\|^2,\quad {\rm for\ every }\ \ r,s\geq 0. \end{equation}

Then $ x=(x_t)_{t\in \mathbb{R}_+}$ is Itô integrable with respect to $(b_t)_{t\in \mathbb{R}_+}$. In fact, for any given interval $[a,b]$, the sequence $x^{(n)}$ of simple adapted processes is given by $x^{(n)}_t=\sum_{i=0}^{n-1} x_{r^{(n)}_i} \otimes \Omega(r^{(n)}_i,t)\chi_{[r^{(n)}_i,r^{(n)}_{i+1})}(t)$ for each $t\geq 0$ and $r^{(n)}_i=a+(b-a)\frac{i}{n-1}$ with $0\leq i\leq n-1$ and converges to $ x=(x_t)_{t\geq 0}$ in L ²-norm.

Lemma 4. Let $(b_t)_{t\in \mathbb{R}_+}$ be a centred additive decomposable section and µ be its associated measure given after Lemma 2. Then we have

\begin{equation*}\displaystyle \int_{0}^t \|b_r\|^{2n}\, {\rm d}\mu(r)=\frac{\|b_t\|^{2(n+1)}}{n+1} \quad{\rm for\ every }\ \ n\geq 0.\end{equation*}

Proof. Recall that [11, Theorem 6.5.10] if f and α are continuous monotone non-decreasing functions on $[a,b]$, then $f\in R(\alpha)$ and $ \alpha\in R(f)$ (here $R(\alpha)$ denotes the space of Riemann–Stieltjes functions with respect to α). Moreover, we have

(2.7)\begin{equation} \int_{a}^{b}f(s)\,{\rm d}\alpha(s)+\int_a^b\alpha(s)\,{\rm d}f(s)=\alpha(b)f(b)-\alpha(a)f(a). \end{equation}

Take $\alpha(s)=f(s)=\|b_s\|^2$. Then for $t\gt 0,$ we have $\int_0^t\|b_s\|^2\,{\rm d}\mu(s)={\|b_t\|^4}/{2}.$ Let us take $\alpha(s)=\|b_s\|^{2(k+1)}$ for $k\geq 1$ and $f(s)=\|b_s\|^2$. Assume that $ \int_0^t\|b_s\|^{2k}\, {\rm d}\mu(s)={\|b_t\|^{2(k+1)}}/{(k+1)}$ is true for k. This means that $d\alpha(s)=(k+1)\|b_s\|^{2k}\,{\rm d}\mu(s)$. Then we have

\begin{align*} \|b_t\|^{2(k+2)}&= \int_0^t \|b_s\|^2\, {\rm d}\alpha(s) + \int_0^t \|b_s\|^{2(k+1)} \,{\rm d}\mu(s)\ {\rm (by\ integration\ by\ parts) }\\ &= \int_0^t \|b_s\|^{2} (k+1)\|b_s\|^{2k}\,{\rm d}\mu(s)+\int_0^t \|b_s\|^{2(k+1)}\, {\rm d}\mu(s)\\ &=\int_0^t (k+2)\|b_s\|^{2(k+1)}\,{\rm d}\mu(s). \end{align*}

Hence, we have shown the lemma by induction.

Denote the space of all centred additive decomposable sections by $\mathcal{A}$ and the set of all centred coherent sections by $\mathcal{C}$. The following proposition is very similar to [6, Proposition 5.9], and we provide the proof for completeness.

Proposition 3. Let $(b_t)_{t\in \mathbb{R}_+}$ be a centred additive decomposable section. Then there exists a unique solution to the quantum stochastic integral equation

(2.8)\begin{equation} u_t=\Omega_t+\int_0^tu_s \,{\rm d}b_s \quad {\rm for\ each }\ t\geq 0, \end{equation}

and the solution is a centred coherent section. Moreover, the map $\mathcal{A}\ni(b_t)_{t\in \mathbb{R}_+}\mapsto (u_t)_{t\in \mathbb{R}_+} \in \mathcal{C}$ is injective.

Proof. Let $x_t^{(0)}=\Omega_t$, $x_t^{(1)}=b_t $ and $x_t^{(n)}=\displaystyle\int_{0}^t x_r^{(n-1)}\,{\rm d}b_r$ for $n\geq 1$. Define $u_t=\displaystyle\sum_{n=0}^{\infty} x_t^{(n)}$ for $t\geq 0$. Then we can see the following.

1. (1) $\langle x_t^{(n)}|x_t^{(m)}\rangle =\delta_{m,n}\frac{\|b_t\|^{2n}}{n!}$ and $\|u_t\|^2={\rm e}^{\|b_t\|^2}$.

2. (2) $(u_t)_{t\in \mathbb{R}_+}$ is Itô integrable and satisfies the quantum stochastic integral equation (2.8).

To show the uniqueness part, let u and v be adapted processes satisfying Equation (2.8). This implies that $\|u_t\|^2=\|v_t\|^2={\rm e}^{\|b_t\|^2}$ and the maps $\mathbb{R}_+\ni t\mapsto \|u_t\|, \|v_t\|\in \mathbb{R}_+$ are continuous. Let $M_t=\displaystyle\sup_{r\in [0,t]}\|u_{r}-v_{r}\|$. Then for $t\geq 0$, $\|u_t-v_t\|^2 =\displaystyle\int_0^t \|u_r-v_r\|^2\,{\rm d}\mu(r)$, and by iteration, we have

\begin{align*} \|u_t-v_t\|^2&= \int_{0}^{t}\;\int_{0}^{r_1}\cdots\int_{0}^{r_{n-1}} \|u_{r_n}-v_{r_n}\|^2 \,{\rm d}\mu(r_n)\, {\rm d}\mu(r_{n-1})\cdots {\rm d}\mu(r_1)\\ &\leq \int_{0}^{t}\;\int_{0}^{r_1}\cdots\int_{0}^{r_{n-1}} M_t^2 \,{\rm d}\mu(r_n)\,{\rm d}\mu(r_{n-1})\cdots {\rm d}\mu(r_1)\\ &= M_t^2 \frac{\|b_t \|^{2n}}{n!}\quad \text{ (by Lemma 4)}\\ &\to 0 \quad \text{as }\ n\to \infty. \end{align*}

Hence, we have proved the uniqueness part. Let $u=(u_t)_{t\in \mathbb{R}_+}$ be the solution of Equation (2.8).

Fix s > 0. Define $w=(w_t)_{t\in \mathbb{R}_+}$ as

\begin{equation*}w_t:=\begin{cases} u_t & \mbox{if } t\in (0,s),\cr u_s\otimes \widetilde{u}_{t-s} & \mbox{if } t\geq s, \end{cases} \end{equation*}

where $\widetilde{u}_{t}=u(s,s+t)$. Then we see that $w=(w_t)_{t\in \mathbb{R}_+}$ satisfies Equation (2.8). By the uniqueness of the solution, $u_{s+t}=w_{s+t}=u_s\otimes \widetilde{u}_t=u_s\otimes u(s,s+t).$ It is also centred. Hence, $u=(u_t)_{t\in \mathbb{R}_+}$ is a centred coherent section. One can check that the map $\mathcal{A}\ni(b_t)_{t\in \mathbb{R}_+}\mapsto (u_t)_{t\in \mathbb{R}_+} \in \mathcal{C}$ is injective.

We will use the notation $\text{Exp}(b)$ to denote the solution of Equation (2.8). Let $(x_t)_{t\in \mathbb{R}_+}$ be any adapted process. Let T > 0 be given. From now onwards, we fix the following notation.

\begin{align*} x_t^\prime&=x_t\otimes \Omega(t,T)\quad \text{for any }\ \ 0\leq t\leq T, \quad \text{and}\\ x_{s,s+t}^\prime&=\Omega_{s}\otimes x_t\otimes \Omega(s+t,T)\quad \text{for }\ \ s,t\geq 0\quad \text{with }s+t\leq T. \end{align*}

We also provide the sketch of the following proposition.

Proposition 4. Let $u=(u_t)_{t\in \mathbb{R}_+}$ be a centred coherent section, and for $t\geq 0$, $n \in \mathbb{N}$, define $ y_t^{(n)}:=\displaystyle\sum_{i=1}^{2^n} y_t^{i,n}$, where for $1\leq i\leq 2^n$,

\begin{align*} y^{i,n}_t=\Omega_{\frac{t}{2^n}} \otimes &\Omega\bigg(\frac{t}{2^n},\frac{2t}{2^n}\bigg)\otimes\cdots \otimes \Omega\bigg(\frac{(i-2)t}{2^n},\frac{(i-1)t}{2^n} \bigg)\otimes \\ &\bigg( u\bigg(\frac{(i-1)t}{2^n},\frac{it}{2^n} \bigg)-\Omega\bigg(\frac{(i-1)t}{2^n},\frac{it}{2^n} \bigg) \bigg) \otimes \cdots \otimes \Omega\bigg(t-\frac{t}{2^n},t\bigg).\\ \end{align*}

Then $\displaystyle \lim_{n\to \infty} y_t^{(n)}$ exists and denote its limit by $\text{log}(u)_t.$ Show that $b=(b_t)_{t\in \mathbb{R}_+}:=(\text{log}(u)_t)_{t\in \mathbb{R}_+}$ is a centred additive decomposable section.

Proof. For s > 0, $\|u_s-\Omega_s\|^2={\rm e}^{\varphi(s)}-1$, where $\varphi(s)=\|b_s\|^2.$ Note that $\langle y^{(n)}_t| y^{(m)}_t\rangle =\sum_{k=1}^{2^m}\left( {\rm e}^{\varphi(\frac{kt}{2^m})-\varphi(\frac{(k-1)t}{2^m})}-1\right) \text{ for }n\leq m$ and $\|y_t^{(n)}\|^2\to \varphi(t)$ as $n\to \infty$. Now for $n\leq m$, we have

\begin{align*} \|y_t^{(n)}-y_t^{(m)}\|^2&=\|y_t^{(n)}\|^2+\|y_t^{(m)}\|^2-2\ \text{Re }\langle y_t^{(n)}|y_t^{(m)}\rangle\\ &\to \varphi(t)+\varphi(t)-2\varphi(t)=0 \ \text{as }\ n,m\to \infty.\\[-18pt] \end{align*}

Hence, $y_t^{(n)}$ is a Cauchy sequence in E _t and it is convergent.

For $s,t\geq 0$, let $y^{(n)}_{s,s+t}=\sum_{i=1}^{2^n} y^{i,n}_{s,s+t}$, where

\begin{align*} &y^{i,n}_{s,s+t}=\Omega\left(s,s+\frac{t}{2^n}\right) \otimes \Omega\left(s+\frac{t}{2^n},s+\frac{2t}{2^n}\right)\otimes\cdots \otimes \Omega\left(s+\frac{(i-2)t}{2^n}, s+\frac{(i-1)t}{2^n} \right)\otimes \\ &\bigg( u\left(s+\frac{(i-1)t}{2^n},s+\frac{it}{2^n} \right)-\Omega\left(s+\frac{(i-1)t}{2^n},s+\frac{it}{2^n}\right ) \bigg) \otimes \cdots \otimes \Omega\left(s+t-\frac{t}{2^n},s+t\right).\\[-12pt] \end{align*}

Let $\widetilde{\Omega}_t=\Omega(s,s+t)$ and $\widetilde{u}_t=u(s,s+t)$ for each $t\geq 0$. Observe that $y^{(n)}_{s,s+t}=\widetilde{y}_{t}^{(n)}$, where $\widetilde{y}_{t}^{(n)}$ is defined using the left coherent section $(\widetilde{u}_t)_{t\in \mathbb{R}_+}$ with respect to the fixed coherent section $(\widetilde{\Omega}_t)_{t\in \mathbb{R}_+}$ which is similar to the above construction. This implies that $\lim_{n\to \infty} y^{(n)}_{s,s+t}$ exists, and we denote its limit by $b(s,s+t)$. First we claim that for $m\in \mathbb{N}$ and $t\geq 0$, $b^\prime_{mt}=b^\prime_{t}+b^\prime_{t,2t}+\cdots + b^\prime_{(m-1)t,mt}.$ For it is enough to show that $\|y^{(n)^\prime}_{mt}-\sum_{k=0}^{m-1}y^{(n)^\prime}_{kt,(k+1)t}\|^2\to 0$ as $n\to \infty.$

Now consider the following expression.

(2.9)\begin{equation} \left\|{y^{(n)^\prime}_{mt}-\sum_{k=0}^{m-1} y^{(n)^\prime}_{kt,(k+1)t}}\right\|^2 =\left\|{y^{(n)}_{mt}}\right\|^2+\left\|{\sum_{k=0}^{m-1} y^{(n)^\prime}_{kt,(k+1)t}}\right\|^2- \sum_{k=0}^{m-1} 2\text{Re}\bigg\langle y^{(n)^\prime}_{mt}\bigg| y^{(n)^\prime}_{kt,(k+1)t}\bigg\rangle. \end{equation}

Note that $\left\|{y^{(n)}_{mt}}\right\|^2=\displaystyle\sum_{k=1}^{2^n}\left( {\rm e}^{\varphi\left(\frac{kmt}{2^n}\right)-\varphi\left(\frac{(k-1)mt}{2^n}\right)}-1\right)$.

Now we have

\begin{align*} &\left\|{\sum_{k=0}^{m-1} y^{(n)^\prime}_{kt,(k+1)t}}\right\|^2\\ &= \sum_{k,l=0}^{m-1}\bigg\langle \Omega_{kt}\otimes y^{(n)}_{kt,(k+1)t}\otimes \Omega\left(\left(k+1\right)t,T\right)\bigg| \Omega_{lt}\otimes y^{(n)}_{lt,(l+1)t}\otimes \Omega\left(\left(l+1\right)t,T\right)\bigg\rangle \\ &=\sum_{k=0}^{m-1}\left\|{y^{(n)}_{kt,(k+1)t} }\right\|^2 \\ &=\sum_{k=0}^{m-1}\sum_{i=1}^{2^n} \left({\rm e}^{\varphi\left(kt+\frac{it}{2^n}\right)-\varphi\left(kt+\frac{(i-1)t}{2^n}\right)}-1\right). \end{align*}

Moreover, finally,

\begin{align*} \bigg\langle y^{(n)^\prime}_{mt}\bigg| y^{(n)^\prime}_{kt,(k+1)t} \bigg\rangle &=\bigg\langle y^{(n)}_{mt}\bigg|\Omega_{kt}\otimes y^{(n)}_{kt,(k+1)t} \otimes \Omega\left(\left(k+1\right)t,mt\right) \bigg\rangle\\ &=\sum_{i,j=1}^{2^n} \bigg\langle y^{j,n}_{mt}\bigg|\Omega_{kt}\otimes y^{i,n}_{kt,(k+1)t} \otimes \Omega((k+1)t,mt) \bigg\rangle\\ &=\sum_{i=1}^{2^n} \left\|{u\left(kt+\frac{(i-1)t}{2^n}, kt+\frac{it}{2^n} \right)- \Omega\left(kt+\frac{(i-1)t}{2^n}, kt+\frac{it}{2^n} \right)}\right\|^2\\ &=\sum_{i=1}^{2^n} \left({\rm e}^{\varphi\left(kt+\frac{it}{2^n}\right)-\varphi\left(kt+\frac{(i-1)t}{2^n}\right)}-1\right). \end{align*}

Using above expressions, Equation (2.9) becomes

\begin{align*} \left\|{y^{(n)^\prime}_{mt}-\sum_{k=0}^{m-1} y^{(n)^\prime}_{kt,(k+1)t}}\right\|^2&= \displaystyle\sum_{j=1}^{2^n}\left( {\rm e}^{\varphi\left(\frac{jmt}{2^n}\right)-\varphi\left(\frac{(j-1)mt}{2^n}\right)}-1\right)\\ &\;\;\quad\quad-\sum_{k=0}^{m-1}\sum_{i=1}^{2^n} \left({\rm e}^{\varphi\left(kt+\frac{it}{2^n}\right)-\varphi\left(kt+\frac{(i-1)t}{2^n}\right)}-1\right)\\ &\to \varphi(mt)-\sum_{k=0}^{m-1}\left(\varphi\left(\left(k+1\right)t\right)-\varphi\left(kt\right) \right)=0,\ \text{as }\ n\to \infty. \end{align*}

Hence, for $m\in \mathbb{N}$, $b^\prime_{mt}=b^\prime_{t}+b^\prime_{t,2t}+\cdots + b^\prime_{(m-1)t,mt}$. From which we deduce that $b^\prime_{(m+n)t}=b^\prime_{nt}+b^\prime_{nt,(m+n)t}$ for $0\leq (m+n)t\leq T$. For any $q\in \mathbb{Q}_+$ with $s+qs\in [0,T]$, we observe that $b^\prime_{s+qs}=b^\prime_{s}+b^\prime_{s,s+qs}$ and $b^\prime_{s,s+t}$ is a limit of $\sum_{i=1}^{2^n} \left(u^\prime_{s+\frac{(i-1)t}{2^n}, s+\frac{it}{2^n}} -\Omega_T \right).$ We see that for any $s,t\geq 0$, $b^\prime_{s+t}=b^\prime_{s}+b^\prime_{s,s+t}$. This implies that $b_{s+t}=b_{s}\otimes\Omega(s,s+t) +\Omega_s\otimes b(s,s+t)$. By definition, it is clear that $(b_t)_{t\in \mathbb{R}_+}$ is centred. Hence, $(b_t)_{t\in \mathbb{R}_+}$ is a centred additive decomposable section.

Lemma 5. Let $(u_t)_{t\in \mathbb{R}_+}$ be a centred coherent section, and let $(x_t)_{t\in \mathbb{R}_+}$ be an adapted process satisfying $\langle x_{r+s}|x_r\otimes \Omega(r,r+s)\rangle =\|x_r\|^2\ \text{for every }r,s\geq 0.$ Then $(x_t)_{t\in \mathbb{R}_+}$ is an Itô integrable adapted process with respect to $(\text{log}_{\Omega}(u)_t)_{t\in\mathbb{R}_+} $. Moreover, we have

\begin{equation*}\bigg\langle u_t\bigg|\int_{0}^{t} x_r \,{\rm d}\text{log}_{\Omega}(u)_r\bigg\rangle =\int_0^t \big\langle u_r\big| x_r\big\rangle\,{\rm d}\mu(r). \end{equation*}

The proof follows from Lemma 2.

Let $b=(b_t)_{t\in \mathbb{R}_+}$ and $c=(c_t)_{t\in \mathbb{R}_+}$ be centred additive decomposable sections. Define a measure on ν on $\mathbb{R}_+$ by $\nu([s,t))=\langle b(s,t)|c(s,t)\rangle$ for every $0\leq s\lt t.$ Let $x=(x_t)_{t\in \mathbb{R}_+}$ and $y=(y_t)_{t\in \mathbb{R}_+}$ be two Itô integrable adapted processes. Then we have,

(2.10)\begin{equation} \left\langle \int_{s}^{t} x_r \,{\rm d}b_r\Bigg| \int_{s}^{t} y_r \,{\rm d}c_r\right\rangle=\int_{s}^{t} \langle x_r|y_r \rangle \,{\rm d}\nu(r). \end{equation}

We require this result in the following theorem and leave it to the reader for verification.

Theorem 1

1. (1) The map $\text{Exp}_{\Omega}: \mathcal{A}\ni b=(b_t)_{t\in \mathbb{R}_+}\mapsto \text{Exp}(b)=(\text{Exp}(b)_t)_{t\in \mathbb{R}_+}\in \mathcal{C}$ is a bijection.

2. (2) Let $b=(b_t)_{t\in \mathbb{R}_+}$ and $c=(c_t)_{t\in \mathbb{R}_+}$ be two centred additive decomposable sections. Then we have

   \begin{equation*} \left\langle \text{Exp}(b)_t\big| \text{Exp}(c)_t\right\rangle ={\rm e}^{\langle b_t|c_t \rangle}\quad \text{for every }\ t\geq 0.\end{equation*}

We remark here that the above Theorem 1 remains true when we replace the additive decomposible section $(b_t)_{t\in \mathbb{R}_+}$ and the corresponding coherent section $(\text{Exp}(b)_t)_{t\in \mathbb{R}_+}$ by the additive decomposable family $(b_t)_{0\lt t\leq T}$ and the coherent family $(\text{Exp}(b)_t)_{0\lt t\leq T}$ for any T > 0.

Let us recall the definition of e-Logarithm L ^e. For t > 0 and $x,y\in D(t)$, we say that $x\sim y$ if there exists a non-zero complex number λ such that $x=\lambda y$. Then ∼ defines an equivalence relation on D(t). Denote by $\dot{x}$ the equivalence class of x and by $\Delta(t)$ the equivalence classes of D(t). Let $\Delta^{(2)}=\{(t;\dot{x},\dot{y}): x,y\in D(t)\}$ for some $\{t\gt 0\}$. We say that a function $f:\Delta^{(2)}\to \mathbb{C}$ is continuous if for any given coherent sections $(x_t)_{t\in\mathbb{R}_+}$ and $(y_t)_{t\in\mathbb{R}_+}$, the map $(0,\infty)\ni t\mapsto f(t;\dot{x_t},\dot{y_t})\in\mathbb{C}$ is continuous. We say that $f:\Delta^{(2)}\to \mathbb{C}$ is vanishing at zero if the limit $\displaystyle \lim_{t\to 0^+} f(t;\dot{x_t},\dot{y_t})=0$. Let $e=(e_t)_{t\in\mathbb{R}_+}$ be a left coherent decomposible section such that $\|e_t\|=1$. By Theorem [4, Theorem 6.4.2], there exists a unique continuous function $L^e:\Delta^{(2)}\to \mathbb{C}$ vanishing at zero such that

\begin{equation*}{\rm e}^{L^e(t;\dot{x},\dot{y})}=\frac{\langle x|y \rangle}{\langle x|e_t \rangle \langle e_t|y \rangle}.\end{equation*}

The function L ^e is called the e-Logarithm. As a consequence of the above theorem, we have the following corollory.

Corollary 1. Let $e=\{e_t\}_{t\in\mathbb{R}_+}$ be a centred coherent section. Then the e-Logarithm is positive definite. More precisely, for every t > 0, the map $D(t)\times D(t)\ni (x,y)\mapsto L^e(t;\dot{x},\dot{y})\in\mathbb{C}$ is positive definite.

Proof. For $x,y\in D(t)$, let $\{x_s:0\lt s\leq t\}$ and $\{y_s:0\lt s\leq t\}$ be the left coherent decomposible families such the $x_t=x$ and $y_t=y$. Then by remark following Theorem 1, there exist left additive decomposible families $\{b_s:0\lt s\leq t\}$ and $\{c_s:0\lt s\leq t\}$ such that

\begin{equation*}\langle x_s| y_s\rangle ={\rm e}^{\langle b_s|c_s \rangle}\quad \text{for every }\ 0\lt s\leq t.\end{equation*}

Set $b=b_t$ and $c=c_t$. Recall that L ^e is homogeneous, that is, for t > 0, we have $L(t;\lambda x,\mu y)=L(t; x, y)$, where $x,y\in D(t)$ and $\lambda,\mu\neq 0$.

For t > 0, let $x,y\in D(t)$. Since L ^e is homogeneous, we can assume that $\langle x|e_t\rangle=1$ and $\langle y|e_t\rangle=1$. With the foregoing notation, we have ${\rm e}^{L^e(t;\dot{x},\dot{y})}=\langle x|y\rangle =\langle x_t|y_t\rangle ={\rm e}^{\langle b_t|c_t\rangle}={\rm e}^{\langle b|c\rangle}.$ Hence, for $x,y\in D(t)$, there exists unique $b,c\in E(t)$ such that $L^e(t;\dot{x},\dot{y})=\langle b|c\rangle$. This implies that for t > 0, the map $D(t)\times D(t)\ni (x,y)\mapsto L^e(t;\dot{x},\dot{y})\in\mathbb{C}$ is positive definite.

3. Decomposable vectors of one-parameter CAR flows

In this section, we describe left coherent sections for one-parmeter CAR flows. We achieve this by using the bijective correspondence between the set of all additive decomposable sections and the set of all left coherent sections obtained in the previous section. Let H be a Hilbert space and let $H^{\otimes n}$ be the n-fold tensor product of H for $n\in \mathbb{N}$. For $\sigma\in S_n$, define a unitary U _σ on $H^{\otimes n}$ by

\begin{equation*}U_{\sigma}(\xi_1\otimes\xi_2\otimes \cdots \otimes\xi_n)=\xi_{\sigma(1)}\otimes\xi_{\sigma(2)}\otimes \cdots \otimes\xi_{\sigma(n)}\quad \text{for every }\ \xi_1,\xi_2,\ldots,\xi_n\in H.\end{equation*}

Let $H^{\unicode{x24D0}^n}$ be the subspace of $H^{\otimes n}$ given by

\begin{equation*} H^{\unicode{x24D0}^n}=\left\{u\in H^{\otimes n}:U_{\sigma}(u)=\varepsilon(\sigma)u,\; \text{for all }\sigma\in S_n \right\}.\end{equation*}

Here $\varepsilon(\sigma)$ is 1 if σ is even and −1 if σ is odd. We define $\xi_1\wedge\xi_2\wedge \cdots\wedge\xi_n\in H^{\unicode{x24D0}^n}$ as

\begin{equation*}\xi_1\wedge\xi_2\wedge \cdots\wedge\xi_n=\frac{1}{\sqrt{n!}}\sum_{\sigma\in S_n}\text{sgn}(\sigma)\xi_{\sigma(1)}\otimes\xi_{\sigma(2)}\otimes \cdots\otimes\xi_{\sigma(n)}\end{equation*}

and the inner product on $H^{\unicode{x24D0}^n}$ as

\begin{equation*}\left\langle \xi_1\wedge\xi_2\wedge \cdots\wedge\xi_n\big|\eta_1\wedge\eta_2\wedge \cdots\wedge\eta_n \right\rangle=\text{det}\left(\left\langle\xi_i|\eta_j\right\rangle\right).\end{equation*}

Let $\Gamma_a(H)$ be the antisymmetric Fock space given by

\begin{equation*} \Gamma_a(H)=\displaystyle\bigoplus_{n=0}^{\infty} H^{\unicode{x24D0}^n}=\mathbb{C}\Omega\oplus \bigoplus_{n=1}^{\infty} H^{\unicode{x24D0}^n}. \end{equation*}

Here, Ω is the vacuum vector. Let H ₁ and H ₂ be Hilbert spaces. Then the map $\Gamma_a(H_1)\otimes \Gamma_a(H_2) \to \Gamma_a(H_1\oplus H_2)$ is given by

\begin{equation*}\left(\xi_1\wedge \xi_2\wedge\cdots \wedge \xi_n\right)\otimes \left(\eta_1\wedge \eta_2\wedge\cdots \wedge \eta_m\right)\mapsto \xi_1\wedge \xi_2\wedge\cdots \wedge \xi_n\wedge\eta_1\wedge \eta_2\wedge\cdots \wedge \eta_m \end{equation*}

for $\xi_i\in H_1$ and $\eta_j\in H_2$ with $1\leq i\leq n$ and $1\leq j\leq m$, $m,n\in \mathbb{N},$ which extends to a unitary operator. We freely use this identification in the rest of the paper. For $\xi\in H$, define a bounded operator $a^*(\xi)$ on $\Gamma_a(H)$ by

(3.1)\begin{equation} a^*(\xi)\eta:=\begin{cases} \xi & \mbox{if } \eta=\Omega\cr \xi\wedge \eta & \mbox{if } \eta\perp \Omega \end{cases} \end{equation}

and denote the adjoint of $a^*(\xi)$ by $a(\xi)$. The operators $a^*(\xi)$ and $a(\xi)$ are called the creation and the annihilation operator associated to a vector ξ. For an isometric representation V of P on H, there exists a unique E ₀-semigroup $\beta=\{\beta_x\}_{x\in P}$ on $B(\Gamma_a(H))$ satisfying

\begin{equation*}\beta_x(a(\xi))=a(V_x\xi)\end{equation*}

for every $\xi\in H$, called the CAR flow associated to an isometric representation V of P (see [10]). Let $x\in P$ be given. Define a unitary $U_x: \Gamma_a(\text{Ker}(V_x^*))\otimes \Gamma_a(H) \to \Gamma_a(H)$ by

\begin{equation*}\left(\xi_1\wedge \xi_2\wedge\cdots \wedge \xi_n\right)\otimes \left(\eta_1\wedge \eta_2\wedge\cdots \wedge \eta_m\right)\mapsto V_x\eta_1 \wedge V_x\eta_2\wedge\cdots \wedge V_x\eta_m\wedge\xi_1\wedge \xi_2\wedge\cdots \wedge \xi_n \end{equation*}

for $\xi_i\in \text{Ker}(V_x^*)$ with $1\leq i\leq n$ and $\eta_j\in H$ with $1\leq j\leq m$, $m,n\in \mathbb{N}$. With little abuse of notation, we write for $\xi\in \Gamma_a(\text{Ker}(V_x^*))$ and $\eta \in \Gamma_a(H)$, $U_x(\xi\otimes \eta)= \Gamma_a(V_x)\eta\wedge \xi.$ For $x\in P$ and $\xi\in \Gamma_a( \text{Ker}(V_x^*))$, define a bounded operator $T^x_{\xi}:\Gamma_a(H)\to \Gamma_a(H)$ by $T^x_{\xi}\eta=\Gamma_a(V_x)\eta\wedge \xi$. The product system is given by $E(x)=\{T^x_{\xi}: \xi\in \Gamma_a( \text{Ker}(V_x^*))\}.$ For notational convenience, in many places, we simply write ξ for $T^x_{\xi}$ in our calculation.

Denote by $\text{int}(P)$ the interior of P. By a P-space, we mean a non-empty closed subset A of $\mathbb{R}^d$ such that $A+P\subseteq A$. Let A be a P-space and $b\in \text{int}(P)$ be given. Define a function $\psi_b^A: \mathbb{R}^d\to \mathbb{R}$ by $\psi_b^A(x)=\text{sup}\{t\in \mathbb{R}: x-tb\in A \}$ for $x\in \mathbb{R}^d$. We simply write ψ _b when A is clear from the context. For $k\in\mathbb{N},$ denote the set $\{(r_1,r_2,\ldots,r_k)\in \mathbb{R}^k: r_i=r_j\ \text{for some\ }i\neq j\ \text{with } 1\leq i,j\leq d\}$ by N which is a null-set of $\mathbb{R}^k$ and define $\varepsilon^{(k)}:\mathbb{R}^k\to \{-1,0,1\}$ by

(3.2)\begin{equation} \varepsilon^{(k)}(r):=\begin{cases} 0 & \mbox{if } r=(r_1,r_2,\ldots,r_k)\in N,\cr \text{sgn}(\sigma) & \mbox{if } r\notin N\ \text{and }\ \sigma\in S_k\ \text{such that } r_{\sigma(1)} \gt r_{\sigma(2)}\gt\cdots \gt r_{\sigma(k)}. \end{cases} \end{equation}

Define a map $ \varepsilon_b^{(k)}:A^k\to \{-1,0,1\}$ by

\begin{equation*} \varepsilon_b^{(k)}(x_1,x_2,\ldots,x_k)=\varepsilon^{(k)}(\psi_b(x_1),\psi_b(x_2),\ldots,\psi_b(x_k)) \end{equation*}

for $(x_1,x_2,\ldots,x_k)\in A^k$, and for $\xi\in L^2(A,K)$ define ${\rm e}^{\varepsilon_b}(\xi)\in \Gamma_a(L^2(A,K))$ by

\begin{equation*}{\rm e}^{\varepsilon_b}(\xi)=\displaystyle\sum_{k=0}^{\infty} \frac{\varepsilon_b^{(k)}\xi^{\otimes k}}{\sqrt{k!}}.\end{equation*}

Let K be a Hilbert space of dimensional k with $k\in \mathbb{N}$. Denote the space of all K-valued square integrable functions on A by $L^2(A,K)$. For $x\in P$, define an operator $V_x^{(A,K)}$ on $L^2(A,K)$ by

\begin{equation*}\left(V_x^{(A,K)}\xi\right)(y)=\begin{cases} \xi(y-x) & \mbox{if } y-x\in A,\cr 0 & \mbox{if } y-x\notin A. \end{cases}\end{equation*}

Then $V^{(A,K)}$ defines an isometric representation of P, called the isometric representation of P associated to A of multiplicity k.

Lemma 6. Let $b,c\in \text{int}(P)$ be given. For any $t\geq 0$ and $\eta\in L^2(A,K)$, we have

1. (1) $\Gamma_a\left(V^{(A,K)}_{tb}\right)\left(\varepsilon_c^{(k)} \eta^{\otimes k}\right)= \varepsilon_c^{(k)} \left(V^{(A,K)}_{tb}\eta\right)^{\otimes k}.$

2. (2) $\Gamma_a\left(V^{(A,K)}_{tb}\right){\rm e}^{\varepsilon_c}(\eta)={\rm e}^{\varepsilon_c}\left(V^{(A,K)}_{tb}\eta\right).$

Proof. Let $x_1,x_2,\ldots,x_k\in A$, then we have

\begin{align*} \left(\Gamma_a\left(V^{(A,K)}_{tb}\right) \right.&\left.\varepsilon_c^{(k)} \eta^{\otimes k}\right)\left(x_1,x_2,\ldots,x_k\right) \\ &\!\!\!\!\!= \left(\varepsilon_c^{(k)} \eta^{\otimes k}\right)\left(x_1-tb,x_2-tb,\ldots,x_k-tb\right)1_{A^k}\left(x_1-tb,x_2-tb,\ldots,x_k-tb\right)\\ &\!\!\!\!\!= \varepsilon^{(k)}\left(\psi^A_c( x_1-tb), \psi^A_c(x_2-tb),\ldots,\psi^A_c( x_k-tb)\right) \\ &\quad(\eta^{\otimes k})\left(x_1-tb,x_2-tb,\ldots,x_k-tb\right)1_{A^k}\left(x_1-tb,x_2-tb,\ldots,x_k-tb\right)\\ &\!\!\!\!\!= \varepsilon^{(k)}\left(\psi^{A+tb}_c( x_1), \psi^{A+tb}_c(x_2),\ldots,\psi^{A+tb}_c( x_k)\right) \left(V^{(A,K)}_{tb}\eta\right)^{\otimes k}\left(x_1,x_2,\ldots,x_k\right)\\ &\!\!\!\!\!= \varepsilon^{(k)}\left(\psi^{A}_c( x_1), \psi^{A}_c(x_2),\ldots,\psi^{A}_c( x_k)\right) \left(V^{(A,K)}_{tb}\eta\right)^{\otimes k}\left(x_1,x_2,\ldots,x_k\right)\\ &\!\!\!\!\!=\left(\varepsilon_c^{(k)} \left(V^{(A,K)}_{tb}\eta\right)^{\otimes k}\right)\left(x_1,x_2,\ldots,x_k\right). \end{align*}

The above equality holds for almost every $(x_1,x_2,\ldots,x_k)\in A^k$. This implies part (1). Clearly, part (2) follows from part (1).

Fix $a\in\text{int}(P)$. Denote the CAR flow associated to the isometric representation $\{V^{(A,K)}_{ta}\}_{t\geq 0}$ by $\{\beta_t\}_{t\geq 0}$. We leave it to the reader to verify that $T^{ta}_{{\rm e}^{\varepsilon_a}(E^{\perp}_{ta}\xi)}$ is a decomposable vector of β for any t > 0 and $\xi\in L^2(A,K)$. In fact, we have the following proposition.

Proposition 5. The set of all decomposable vectors of $\{\beta_t\}_{t\geq 0}$ is given by $\{\lambda T_{{\rm e}^{\varepsilon_a}(E^{\perp}_{ta}\xi)}: \lambda \in \mathbb{C}\setminus\{0\},\; t\lt 0\ \text{and } \xi\in L^2(A,K)\}$.

Proof. Let $b=(b_t)_{t\geq 0}$ be an additive decomposable section for β. Then for $t\geq 0$, $b_t=E^{\perp}_{ta}\xi$ for some $\xi\in L^2(A,K)$. By the proof of Proposition 3, the corresponding left coherent section $u=(u_t)_{t\geq 0}$ is given by

\begin{equation*}u_t=\displaystyle \sum_{k=0}^{\infty} x_t^{(k)},\quad \text{where } x_t^{(0)}=\Omega_t,\;x_t^{(1)}=b_t\;\text{and } x_t^{(k)}=\int_{0}^{t} x_r^{(k-1)}\, {\rm d}b_r \ \text{for }t\geq 0. \end{equation*}

First let us compute $x_t^{(2)}$ for $t\geq 0$. For each $n\in \mathbb{N}$, let $r_{i,n}=\frac{ita}{n}$ with $0\leq i\leq n$.

\begin{align*} x_t^{(2)}&=\int_{0}^{t} E_{ra}^{\perp}\xi\, {\rm d}E_{ra}^{\perp}\xi \\ &= \lim_{n\to \infty} \sum_{i=0}^{n-1} T^{r_{i,n}}_{E^{\perp}_{r_{i,n}}\xi}\;\circ \; T^{r_{i+1,n}-r_{i,n}}_{V^*_{r_{i,n}}E^{\perp}_{r_{i+1,n}}\xi}\;\circ\; T_{\Omega_{ta-r_{i+1,n}}}^{ta-r_{i+1,n}} \;(\text{by Proposition~}2) \\ &=\displaystyle \lim_{n\to \infty} T^{ta}_{\sum_{i=0}^{n-1} E_{r_{i,n}}E^{\perp}_{r_{i+1,n}}\xi\wedge E^{\perp}_{r_{i,n}}\xi }\\ &=\displaystyle \lim_{n\to \infty} \sum_{i=0}^{n-1} E_{r_{i,n}}E^{\perp}_{r_{i+1,n}}\xi\wedge E^{\perp}_{r_{i,n}}\xi . \end{align*}

In the view of Proposition 2, it is enough to check the pointwise convergence of $\sum_{i=0}^{n-1} E_{r_{i,n}}E^{\perp}_{r_{i+1,n}}\xi\wedge E^{\perp}_{r_{i,n}}\xi$ to $\frac{\varepsilon^{(2)}_a \; (E_{ta}^{\perp}\xi)^{\otimes 2}}{\sqrt{2!}}$ almost everywhere. For $x\in A$, there exists a unique $\widetilde{x}\in \partial A$ such that $x=\widetilde{x}+\psi_a(x)a$. For almost every $(x,y)\in A\times A$ and for large n, we have

\begin{align*} &\left( \sum_{i=0}^{n-1} E_{r_{i,n}}E^{\perp}_{r_{i+1,n}}\xi\wedge E^{\perp}_{r_{i,n}}\xi\right)(x,y) = \frac{1}{\sqrt{2!}}\sum_{i=0}^{n-1}\left(\chi_{\left(A+\frac{ita}{n}\right)\cap \left(A\setminus A+\frac{(i+1)ta}{n}\right)}(x)\right.\\ &\quad\quad\quad\quad\chi_{\left(A\setminus A+\frac{ita}{n}\right)}(y) \left.- \chi_{\left(A\setminus A+\frac{ita}{n}\right)}(x) \chi_{\left(A+\frac{ita}{n}\right)\cap \left(A\setminus A+\frac{(i+1)ta}{n}\right)}(y)\right) \xi(x)\otimes \xi(y) \end{align*}

When $\psi_a(x)\lt\psi_a(y)$, $x\in A\setminus A+\frac{ita}{n}$ and $y\in (A+\frac{ita}{n})\cap (A\setminus A+\frac{(i+1)ta}{n})$ for some $0\leq i\leq n-1$. Hence, $\left( \sum_{i=0}^{n-1} E_{r_{i,n}a}E^{\perp}_{r_{i+1,n}a}\xi\wedge E^{\perp}_{r_{i,n}a}\xi\right)(x,y)=\frac{-(E_{ta}^{\perp}\xi)(x)\otimes (E_{ta}^{\perp}\xi)(y)}{\sqrt{2!}}$. Similarly if $\psi_a(x)\gt\psi_a(y)$, $\left( \sum_{i=0}^{n-1} E_{r_{i,n}a}E^{\perp}_{r_{i+1,n}a}\xi\wedge E^{\perp}_{r_{i,n}a}\xi\right)(x,y)=\frac{(E_{ta}^{\perp}\xi)(x)\otimes (E_{ta}^{\perp}\xi)(y)}{\sqrt{2!}}$. We conclude that $x_t^{(2)}=\int_{0}^{t} E_{ra}^{\perp}\xi \,{\rm d}E_{ra}^{\perp}\xi=\frac{\varepsilon^{(2)}_a \; (E_{ta}^{\perp}\xi)^{\otimes 2}}{\sqrt{2!}}$.

Before proving $x_t^{(k)}=\frac{\varepsilon^{(k)}_a \; (E_{ta}^{\perp}\xi)^{\otimes k}}{\sqrt{k!}}$ for any $k\in\mathbb{N}$, let us fix few notation. For $\xi_1,\xi_2,\ldots,\xi_n,\eta\in H$, set $\eta^{(k)}\odot (\xi_1\otimes \xi_2\otimes\cdots\otimes \xi_{n})=\xi_1\otimes \xi_2\otimes\cdots \otimes\xi_{k-1}\otimes \eta\otimes \xi_{k}\otimes\cdots\otimes \xi_{n}$ for $1\leq k\leq n$. With this notation, we can see that

\begin{equation*}\xi_1\wedge (\xi_2\wedge \xi_3\wedge\cdots\wedge\xi_n)=\displaystyle\frac{1}{\sqrt{k}}\sum_{j=1}^n (-1)^{j-1} \xi_1^{(j)}\odot (\xi_2\wedge \xi_3\wedge\cdots\wedge\xi_n).\end{equation*}

Assume that $x_t^{(k-1)}=\frac{\varepsilon^{(k-1)}_a \; (E_{ta}^{\perp}\xi)^{\otimes (k-1)}}{\sqrt{(k-1)!}}$ for some $k\in \mathbb{N}$. Now consider the following expression. For almost every $(x_1,x_2,\ldots,x_k)\in A^k$ and for large n, we have

\begin{align*} \left( \sum_{i=0}^{n-1}E_{r_{i,n}} \right.& \left.E^{\perp}_{r_{i+1,n}}\xi\wedge \frac{\varepsilon^{(k-1)}_a \; (E_{r_{i,n}a}^{\perp}\xi)^{\otimes (k-1)}}{\sqrt{(k-1)!}} \right)(x_1,x_2,\ldots,x_k)\\ &\!\!\!\!\!\!\!\!\!=\sum_{i=0}^{n-1}\frac{1}{\sqrt{k}}\sum_{j=1}^k (-1)^{j-1} \left( (E_{r_{i,n}}E^{\perp}_{r_{i+1,n}}\xi)^{(j)} \right.\\ &\left.\quad\quad\quad\quad\quad\quad\odot \frac{\varepsilon^{(k-1)}_a \; (E_{r_{i,n}a}^{\perp}\xi)^{\otimes (k-1)}}{\sqrt{(k-1)!}} \right)(x_1,x_2,\ldots,x_k)\\ &\!\!\!\!\!\!\!\!\!=\sum_{i=0}^{n-1}\frac{1}{\sqrt{k!}}\sum_{j=1}^k (-1)^{j-1} \chi_{\left(A+\frac{ita}{n}\right)\cap \left(A\setminus A+\frac{(i+1)ta}{n}\right)}(x_j) \varepsilon^{(k-1)}_a(x_1,x_2,\ldots,\widehat{x_j},\ldots,x_k) \\ &\quad\quad\prod_{l=1,l\neq j}^{k} \chi_{\left(A+\frac{ita}{n}\right)}(x_l) (E_{ta}^{\perp}\xi)^{\otimes k} (x_1,x_2,\ldots,x_k). \end{align*}

There exist unique i and j such that $x_j\in(A+\frac{ita}{n})\cap (A\setminus A+\frac{(i+1)ta}{n})$ and $x_1,x_2,\ldots,\widehat{x_j},\ldots,x_k\in A+\frac{ita}{n}$.

Hence, $\left( \sum_{i=0}^{n-1}E_{r_{i,n}}E^{\perp}_{r_{i+1,n}}\xi\wedge \frac{\varepsilon^{(k-1)}_a \; (E_{r_{i,n}a}^{\perp}\xi)^{\otimes (k-1)}}{\sqrt{(k-1)!}} \right)=\frac{\varepsilon^{(k)}_a \; (E_{ta}^{\perp}\xi)^{\otimes k}}{\sqrt{k!}}$ almost everywhere. By definition, we have

\begin{equation*} x_t^{(k)}=\int_{0}^{t} \frac{\varepsilon^{(k-1)}_a \; (E_{ra}^{\perp}\xi)^{\otimes (k-1)}}{\sqrt{(k-1)!}} \,{\rm d}E_{ra}^{\perp}\xi =\displaystyle \lim_{n\to \infty} \sum_{i=0}^{n-1} E_{r_{i,n}}E^{\perp}_{r_{i+1,n}}\xi\wedge \frac{\varepsilon^{(k-1)}_a \; (E_{r_{i,n}a}^{\perp}\xi)^{\otimes (k-1)}}{\sqrt{(k-1)!}}. \end{equation*}

Hence, we conclude that for each $k\in \mathbb{N}$, $x_t^{(k)}=\frac{1}{\sqrt{k!}}\varepsilon_a^{(k)}(E_{ta}^{\perp}\xi)^{\otimes k}$ and $u_t={\rm e}^{\varepsilon_a}(E_{ta}^{\perp}\xi)$. This completes the proof.

4. Characterization for decomposablility of CAR flows

Let us recall the definition of a decomposable product system from [12]. Let $\alpha=\{\alpha_x\}_{x\in P}$ be an E ₀-semigroup over P on B(H). For $x\in P$, let $E(x)=\{T\in B(H): \alpha_x(A)T=TA\ \text{for all }A\in B(H)\}.$ Then $E=\{E(x):x\in P\}$ has the structure of the product system. We say that a product system over P is spatial if it admits a unit. We also use the notation α ^V and β ^V to denote the CCR flow and the CAR flow associated to the isometric representation V.

For $x\in P$, a non-zero element $u\in E(x)$ is said to be a decomposable vector if for $y\in P$ with $y\leq x$, there exist $v\in E(y)$ and $w\in E(x-y)$ such that u = vw. Denote the set of all decomposable vectors in E(x) by D(x). We say that the product system $E=\{E(x):x\in P\}$ is decomposable if the following conditions are satisfied.

1. (1) For every $x,y\in P$, $D(x)D(y)\subseteq D(x+y)$.

2. (2) For every $x\in P$, D(x) is total in E(x).

We call an E ₀-semigroup is decomposable if its associated product system is decomposable. It is known that the CCR flow α ^V associated to an isometric representation V of P is decomposable (see [12, Proposition]). The converse of this statement is also true and is given in the following theorem.

Theorem 1. [12, Theorem 4.6]

Let E be a decomposable product system over P which admits a unit. Then there exists an isometric representation V of P such that E is isomorphic to $\mathcal{E}_{\alpha^V}$ as product systems. Here $\mathcal{E}_{\alpha^V}$ denotes the product system associated to the CCR flow α ^V.

In fact, a much stronger result was proved in [12]. More precisely, we have the following theorem.

Theorem 2. The set of all isometric representations of P, up to unitary equivalence, are in bijective correspondence with the set of all spatial decomposable product systems over P, up to cocycle conjugacy.

Let E be a spatial product system over P. Fix a unit $(\Omega_x)_{x\in P}$ of E. An element $u\in E(x)$ is said to be an additive decomposable vector if for any $y\in P$ with $x-y\in P$, there exist $v\in E(y)$ and $w\in E(x-y)$ such that $u=v\otimes \Omega_{x-y}+\Omega_y \otimes w$. Denote the set of all additive decomposable vectors in E(x) by A(x). A spatial product system E is said to be embeddable if the following conditions hold.

\begin{equation*}A(x)\otimes \Omega_y\subseteq A(x+y)\quad \text{and}\quad \Omega_x\otimes A(y)\subseteq A(x+y)\quad \text{for every}\ x,y\in P.\end{equation*}

In [12], Sundar has provided the construction of an isometric representation of P from a decomposable product system that admits a unit. But in [10], Srinivasan has provided the construction of an isometric representation of P from a spatial embeddable product system over P. It was shown that the isometric representation constructed out of α ^V and β ^V is unitary equivalent to V itself (see [10, Proposition 4.1]). He also showed that any isomorphism between two spatial embeddable product systems over P, by fixing the canonical unit, induces a conjugate unitary between the corresponding isometric representations of P (see [10, Definition 3.3]) and the discussion following that.

Let A be a P-space and let K be a Hilbert space of dimension k with $k\in \mathbb{N}$. Let us denote the CAR flow associated to the isometric representation $V^{(A,K)}$ by β. The goal of this section is to exhibit the necessary and sufficient condition for the CAR flow β to be decomposable. With the foregoing notation, we have the following proposition.

Proposition 6. Let E be the product system associated to β. Assume that $E=\{E(x): x\in P\}$ is decomposable. Then for any given $b,c\in \text{int}(P)$, we have

\begin{equation*}\varepsilon^{(2)}_b\left(E_{sb}^{\perp}E_{tc}^{\perp}\xi\right)^{\otimes 2}=\varepsilon^{(2)}_c\left(E_{sb}^{\perp}E_{tc}^{\perp}\xi\right)^{\otimes 2} \quad a.e \ \text{for all}\ \xi\in L^2(A,K).\end{equation*}

Proof. Without loss of generality, we assume that b and c are linearly independent. Choose a linearly independent collection $v_1,v_2,\ldots,v_d\in\text{int}(P)$ with $v_1=b$ and $v_2=c$. Let $Q=\{r_1v_1+r_2v_2+\cdots+r_dv_d:\text{for all } r_i\geq 0 \ \text{with }1\leq i\leq d\}$. Since E is decomposable over P, it is also decomposable over Q. For the product system $\{E(x)\}_{x\in Q}$ over Q, let D(x) be the set of all decomposable vectors in E(x). By Proposition 5, we have

\begin{align*} D(sb)&=\{\lambda T_{{\rm e}^{\varepsilon_b}(E^{\perp}_{sb}\xi)}: \lambda \in \mathbb{C}\setminus \{0\}\; \text{and } \xi\in L^2(A,K)\}, \text{and}\\ D(tc)&=\{\mu T_{{\rm e}^{\varepsilon_c}(E^{\perp}_{tc}\eta)}: \mu \in \mathbb{C}\setminus \{0\}\; \text{and } \eta\in L^2(A,K)\}. \end{align*}

As E is decomposable over Q, $D(sb)D(tc)=D(sb+tc)=D(tc)D(sb)$, that is, for any $\xi, \eta \in L^2(A,K)$, there exist $\xi^\prime, \eta^\prime \in L^2(A,K)$ such that

\begin{equation*}T^{sb}_{{\rm e}^{\varepsilon_b}(E^{\perp}_{sb}\xi)}T^{tc}_{{\rm e}^{\varepsilon_c}(E^{\perp}_{tc}\eta)}=T^{tc}_{{\rm e}^{\varepsilon_c}(E^{\perp}_{tc}\eta^\prime)}T^{sb}_{{\rm e}^{\varepsilon_b}(E^{\perp}_{sb}\xi^\prime)}.\end{equation*}

By applying ζ on both sides, we have

\begin{align*} \Gamma_a(V_{sb+tc})\zeta\wedge\Gamma_a(V_{sb}){\rm e}^{\varepsilon_c}(E^{\perp}_{tc}\eta)\wedge &{\rm e}^{\varepsilon_b}(E^{\perp}_{sb}\xi)=\\ &\Gamma_a(V_{sb+tc})\zeta\wedge \Gamma_a(V_{tc}){\rm e}^{\varepsilon_b}(E^{\perp}_{sb}\xi^\prime)\wedge {\rm e}^{\varepsilon_c}(E^{\perp}_{tc}\eta^\prime). \end{align*}

The above equation together with Lemma 6 gives

\begin{equation*}{\rm e}^{\varepsilon_c}(V_{sb}E^{\perp}_{tc}\eta)\wedge {\rm e}^{\varepsilon_b}(E^{\perp}_{sb}\xi)= {\rm e}^{\varepsilon_b}(V_{tc}E^{\perp}_{sb}\xi^\prime)\wedge {\rm e}^{\varepsilon_c}(E^{\perp}_{tc}\eta^\prime).\end{equation*}

Applying $\Gamma_a(E^{\perp}_{sb}E^{\perp}_{tc})$ on both sides, we have $ {\rm e}^{\varepsilon_b}(E^{\perp}_{sb}E^{\perp}_{tc}\xi)= {\rm e}^{\varepsilon_c}(E^{\perp}_{sb}E^{\perp}_{tc}\eta^\prime).$ Equating one-particle space and two-particle space on both sides, we have $E^{\perp}_{sb}E^{\perp}_{tc}\xi=E^{\perp}_{sb}E^{\perp}_{tc}\eta^\prime$, and hence,

\begin{equation*}\varepsilon^{(2)}_b(E_{sb}^{\perp}E_{tc}^{\perp}\xi)^{\otimes 2}=\varepsilon^{(2)}_c(E_{sb}^{\perp}E_{tc}^{\perp}\xi)^{\otimes 2} \quad a.e\ \text{for all } \xi\in L^2(A,K).\\[-24.5pt] \end{equation*}

Proposition 7. [2, Proposition 2.3(3)]

Let A be a P-space and let $a\in \text{int}(P)$ be given. Then the map $\partial A\times (0,\infty)\ni (x,t)\mapsto x+ta\in \text{Int}(A)$ is a homeomorphism.

Let $v_1,v_2,\ldots,v_d\in \text{int}(P)$ be a linearly independent set in $\mathbb{R}^d$. Define a function $\varphi: \mathbb{R}^{d-1}\to \mathbb{R}$ by $\varphi(r_1,r_2,\ldots,r_{d-1}):=r_d-\psi_{v_d}(r_1v_1+r_2v_2+\cdots+r_dv_d)$. The map is well-defined. This follows from the observation that for $s\in \mathbb{R}$, $\psi_{v_d}(r_1v_1+r_2v_2+\cdots +(r_{d}+s)v_d)=s+\psi_{v_d}(r_1v_1+r_2v_2+\cdots +r_{d}v_d)$. With the foregoing notation, we have the following lemma.

Lemma 7. Let $a\in \text{int}(P)$ be given. Then we have the following.

1. (1) The map $\partial A\times \mathbb{R}\ni (x,t)\mapsto x+ta \in \mathbb{R}^d$ defines a homeomorphism.

2. (2) The P-space A can be described as $A=\{x\in \mathbb{R}^d: \psi_a(x)\geq 0\}$.

3. (3) The boundary of A is given by $\partial A=\{x\in \mathbb{R}^d: \psi_{v_d}(x)=0\}$. Moreover, the map $B:\mathbb{R}^{d-1}\to \partial A$ is given by

   \begin{equation*}B(r_1,r_2,\ldots,r_{d-1}):=r_1v_1+r_2v_2+\cdots+r_{d-1}v_{d-1}+\varphi(r_1,r_2,\ldots,r_{d-1})v_d\end{equation*}
   for $(r_1,r_2,\ldots,r_{d-1})\in \mathbb{R}^{d-1}$ defines a homeomorphism.

Proof. The proof of part (1) follows similar to the proof of [2, Proposition 2.3(3)]. Part (2) is clear from the definition. Note that $\partial A=\{x\in \mathbb{R}^d: \psi_{v_d}(x)= 0\}$. Define $\varphi:\mathbb{R}^{d-1}\to \mathbb{R}$ by

\begin{equation*}\varphi(r_1,r_2,\ldots,r_{d-1})=r_d-\psi_{v_d}(r_1v_1+r_2v_2+\cdots+r_{d-1}v_{d-1}+ r_dv_d)\end{equation*}

for every $(r_1,r_2,\ldots,r_{d-1},r_d)\in\mathbb{R}^d$. Now the part (3) is clear from the fact that $\partial A=\{x\in \mathbb{R}^d: \psi_{v_d}(x)= 0\}$, and the remaining we leave it to the reader for verification.

Theorem 3. Let E be the product system over P corresponding to β. Suppose E is decomposable, then there exists an element $\lambda\in P^*$ such that $A=\{x\in \mathbb{R}^d: \langle x|\lambda\rangle \geq 0\}$.

Proof. Let $v_1,v_2,\ldots,v_d\in \text{int}(P)$ be a linearly independent set in $\mathbb{R}^d$. Fix i with $1\leq i\leq d-1$ and $t_0\gt 0$. By Proposition 7 and Lemma 7(3), there exist functions $f_0:\mathbb{R}^{d-1}\to \mathbb{R}^{d-1}$ and $g_0:\mathbb{R}^{d-1}\to (0,\infty)$ such that for every $r=(r_1,r_2,\ldots,r_{d-1})\in \mathbb{R}^{d-1}$, we have

\begin{equation*}B(r)+t_0v_i=B(f_0(r))+g_0(r)v_d,\end{equation*}

\begin{align*} \text{that is, } r_1v_1+&r_2v_2+\cdots+r_{d-1}v_{d-1}+\varphi(r)v_d+t_0v_i=\\ &i_1\circ f_0(r)v_1+i_2\circ f_0(r)v_2+\cdots+i_{d-1}\circ f_0(r)v_{d-1}+\varphi(f_0(r))v_d+g_0(r)v_d. \end{align*}

Here $i_l:\mathbb{R}^{d-1}\to \mathbb{R}$ denotes the projection onto the lth coordinate for each $1\leq l\leq d-1$. By equating the coefficients of v’s on both sides, we see that $f_0(r)=r+t_0e_i$ and $g_0(r)=\varphi(r)-\varphi(r+t_0e_i)$. Here $e_i=(0,0,\ldots,1,\ldots,0)\in \mathbb{R}^{d-1}$, where 1 in the ith place and elsewhere 0. Hence, f ₀ and g ₀ are continuous. Similarly for δ > 0, there exist continuous functions $f_{\delta}:\mathbb{R}^{d-1}\to \mathbb{R}^{d-1}$ and $g_{\delta}:\mathbb{R}^{d-1}\to (0,\infty)$ such that

\begin{equation*}B(r)+(t_0+\delta)v_i=B(f_{\delta}(r))+g_{\delta}(r)v_d \quad \text{for all }r\in \mathbb{R}^{d-1}. \end{equation*}

We claim that $g_0(.)$ is constant. Suppose not, choose $r^\prime, r^{\prime\prime}\in \mathbb{R}^{d-1}$ such that $g_0(r^\prime)\gt g_0(r^{\prime\prime})$. Note that the map $[0,\infty)\times \mathbb{R}^{d-1} \ni (\delta, r)\mapsto g_{\delta}(r)\in (0,\infty)$ is continuous. Choose $\delta_0\gt 0$ such that $g_0(r^\prime)\gt g_{\delta_0}(r^{\prime\prime}) $. Then there exists a compact neighbourhood K of $\text{int}(A)\times \text{int}(A)$ containing $\big((t_0,r^\prime),(t_0+\delta_0,r^{\prime\prime})\big)$ such that

\begin{equation*}t\lt t^\prime\ \text{and }g_{t}(r)\gt g_{t^\prime}(s) \quad \text{for every }\;(B(r)+tv_i,B(s)+t^\prime v_i)\in K.\end{equation*}

This implies that $\varepsilon^{(2)}_{v_i}\neq \varepsilon^{(2)}_{v_d}$ on K. This is a contradiction to Proposition 6. Hence, $g_0(.)$ is a constant function.

Without loss of generality, we assume that $\partial (A)\ni 0$. Then $\varphi(0)=0$. Using this equality and the fact that $g_0(.)$ is a constant function, we see that for every i with $1\leq i\leq d-1$, $\varphi(r+s_0e_i)=\varphi(r)+\varphi(s_0e_i)$ for $r\in \mathbb{R}^{d-1}$ and $s_0\gt 0$. Consequently, we deduce that $\varphi(r)=\varphi(r+c)-\varphi(c)$ for every $r\in \mathbb{R}^{d-1}$ and $c=(c_1,c_2,\ldots,c_{d-1})$ with $c_i\gt 0$. Let $r,r^\prime \in \mathbb{R}^{d-1}$ be given. Choose $c=(c_1,c_2,\ldots,c_{d-1}),c^\prime=(c_1^\prime,c_2^\prime,\ldots,c^\prime_{d-1})\in \mathbb{R}^{d-1}$ with $c_i,c_i^\prime\gt 0$ and $ c_i+r_i,c_i^\prime+r_i^\prime\gt 0$ for each $1\leq i\leq d-1$. Then we have,

\begin{align*} \varphi(r)+\varphi(r^\prime)&=\varphi(r+c)-\varphi(c)+\varphi(r^\prime+c^\prime)-\varphi(c^\prime)\\ &=\varphi(r+c)+\varphi(r^\prime+c^\prime)-(\varphi(c)+\varphi(c^\prime))\\ &=\varphi(r+r^\prime+c+c^\prime)-\varphi(c+c^\prime)=\varphi(r+r^\prime). \end{align*}

Since φ is continuous, there exists a unique $\mu\in \mathbb{R}^{d-1}$ such that $\varphi(r)=\langle r|\mu\rangle$ for every $r\in \mathbb{R}^{d-1}$. By Lemma 7(2), $r_d-\varphi(r_1,r_2,\ldots,r_{d-1})=r_d-\langle r|\mu\rangle=\psi_{v_d}(r_1v_1+r_2v_2+\cdots+r_dv_d)\geq 0$ for the points of A. Therefore $A=\{x\in \mathbb{R}^d: \langle x|\lambda\rangle \geq 0\}$ for some $\lambda\in \mathbb{R}^{d}$. Since $P\subseteq A$, $\lambda\in P^*$. This completes the proof.

Recall that a P-space A is proper if the isometric representation $V^{(A,\mathbb{C})}$ associated to A is proper. In other words, A is proper if there exist $x,y\in P$ such that $(A+x)\cap(A\setminus A+y)\neq\emptyset$ and $(A+y)\cap(A\setminus A+x)\neq\emptyset$. We remark here that any P-space A of the form $A=\{x\in \mathbb{R}^d: \langle x|\lambda\rangle \geq 0\}$, for some $\lambda\in P^*$, is not proper. For, let $x,y\in P$. Note that $A+x=\{z\in \mathbb{R}^d: \langle z-x|\lambda\rangle\geq 0 \}$ and $A\setminus A+y=\{z\in \mathbb{R}^d: \langle z|\lambda\rangle\geq 0\ \text{and } \langle z-y|\lambda\rangle\gt0\}$. Then we have

\begin{align*} (A+x)\cap(A\setminus A+y)&=\{z\in \mathbb{R}^d: \langle z|\lambda\rangle\geq 0,\; \langle z-x|\lambda\rangle\geq 0\quad \text{and } \langle z-y|\lambda\rangle\lt0\}, \text{and }\\ (A+y)\cap(A\setminus A+x)&=\{z\in \mathbb{R}^d: \langle z|\lambda\rangle\geq 0,\; \langle z-y|\lambda\rangle\geq 0\quad \text{and } \langle z-x|\lambda\rangle\lt0\}. \end{align*}

Suppose there exist $x,y\in P$ such that $(A+x)\cap(A\setminus A+y)\neq \emptyset$. Then there exists $z_0\in A$ such that

(4.1)\begin{equation} \langle z_0-x|\lambda\rangle\geq 0\ \text{and }\langle z_0-y|\lambda\rangle\lt0. \end{equation}

Let $z\in A$ be any element such that

(4.2)\begin{equation} \langle z-y|\lambda\rangle \geq 0. \end{equation}

Then we have

\begin{align*} \langle z-x,\lambda\rangle &=\langle z-y|\lambda\rangle+ \langle y-z_0|\lambda\rangle+ \langle z_0-x|\lambda\rangle \quad \quad ( \text{By inequalities } (4.1)\ \text{and}\ (4.2))\\ &\lt0. \end{align*}

This implies that $(A+y)\cap(A\setminus A+x)=\emptyset$. Similarly, if $(A+y)\cap(A\setminus A+x)\neq\emptyset$, then we have $(A+x)\cap(A\setminus A+y)=\emptyset$. Therefore, either $(A+x)\cap(A\setminus A+y)=\emptyset$ or $(A+y)\cap(A\setminus A+x)=\emptyset$ for every $x,y\in P$. Hence, any half space is not proper.

Proposition 8. Let $v_1,v_2,\ldots,v_d$ be a linearly independent set in $\mathbb{R}^d$ and let $Q:=\{r_1v_1+r_2v_2+\cdots+r_dv_d: \text{each }r_i\geq 0\}$. Let $E=\{E(x)\}_{x\in Q}$ be a product system over Q. For $1\leq i\leq d$, set $E^{(i)}=\{E^{(i)}(r)=E(rv_i): r\geq 0\}$ and denote the set of all decomposable vectors in $E^{(i)}(r)$ by $D^{(i)}(r)$ for $r\geq 0$. Suppose for $1\leq i,j\leq d$ and $r,s\geq 0$, $D^{(i)}(r)D^{(j)}(s)=D^{(j)}(s)D^{(i)}(r)$. Then E is decomposable over Q if and only if each $E^{(i)}$ is decomposable over $\mathbb{R}_+$.

Proof. We only prove for d = 2 and the proof for general d is similar. Assume that E is a decomposable product system. Then each $E^{(i)}$ is a decomposable product system over $\mathbb{R}_+$. Conversely, assume that each $E^{(i)}$ is decomposable over $\mathbb{R}_+$ and $D^{(1)}(r)D^{(2)}(s)=D^{(2)}(s)D^{(1)}(r)$ for every $r,s\gt 0$. We claim that $D(rv_1+sv_2)= D^{(1)}(r)D^{(2)}(s)$, for every $r,s\gt 0$. Clearly, $D(rv_1+sv_2)\subseteq D^{(1)}(r)D^{(2)}(s)$. On the other hand, let $u\in D^{(1)}(r)$ and $v\in D^{(2)}(s)$. Let $r^\prime v_1+s^\prime v_2\in Q$ be such that $r^\prime v_1+s^\prime v_2\lt rv_1+sv_2.$ Then $(r-r^\prime)v_1+(s-s^\prime)v_2\in \text{int}(Q)$, that is, $r\gt r^\prime$ and $s\gt s^\prime$. Write $u=u^\prime u^{\prime\prime}$ for some $u^\prime \in E^{(1)}(r^\prime)$ and $u^{\prime\prime} \in E^{(1)}(r-r^\prime)$. Then we have $u^\prime \in D^{(1)}(r^\prime)$ and $u^{\prime\prime} \in D^{(1)}(r-r^\prime)$. Similarly we have $v=v^\prime v^{\prime\prime} \in D^{(2)}(s^\prime)D^{(2)}(s-s^\prime)$. By using the relation $D^{(1)}(r-r^\prime)D^{(2)}(s^\prime)=D^{(2)}(s^\prime)D^{(1)}(r-r^\prime)$, we have $u^{\prime\prime} v^\prime=\tilde{v}\tilde{u}$ for some $\tilde{v}\in D^{(2)}(s^\prime)$ and $\tilde{u}\in D^{(1)}(r-r^\prime)$. Now we have

\begin{equation*}uv=u^\prime u^{\prime\prime} v^\prime v^{\prime\prime}=u\tilde{v}\tilde{u}v^{\prime\prime} \in E(r^\prime v_1+s^\prime v_2)E((r-r^\prime)v_1+(s-s^\prime)v_2).\end{equation*}

This implies that $uv\in D(rv_1+sv_2)$, and hence $D(rv_1+sv_2)= D^{(1)}(r)D^{(2)}(s)$. Clearly, $D(rv_1+sv_2)$ is total in $E(rv_1+sv_2)$. Now for any $r,r^\prime,s,s^\prime\gt 0$, we have

\begin{align*} D(rv_1+sv_2)D(r^\prime v_1+s^\prime v_2)&=D^{(1)}(r)D^{(2)}(s)D^{(1)}(r^\prime)D^{(2)}(s^\prime)\\ &=D^{(1)}(r)D^{(1)}(r^\prime)D^{(2)}(s)D^{(2)}(s^\prime)\\ &=D^{(1)}(r+r^\prime)D^{(2)}(s+s^\prime)\\ &= D((r+r^\prime)v_1+(s+s^\prime)v_2) \end{align*}

Therefore, E is a decomposable product system over Q.

Proposition 9. Let $A=\{x\in \mathbb{R}^d: \langle x|\lambda\rangle \geq 0\}$ for some $\lambda\in P^*$ and let E be the product system for the CAR flow over P associated to the P-space A of multiplicity k. Then E is decomposable over P.

Proof. Since $P\subseteq A$, choose a linearly independent set $\{v_1,v_2,\ldots,v_d\}$ in A such that $v_1,v_2,\ldots,v_{d-1}\in \partial A$ and $P\subseteq Q=\{r_1v_1+r_2v_2+\cdots+r_dv_d: \text{each }r_i\geq 0\}$. Note that $\partial A$ is a d − 1 vector space over $\mathbb{R}$. Observe that A is also a Q-space. Let $F=\{F(x)\}_{x\in Q}$ be the product system for the CAR flow over Q associated to A of multiplicity k. Note that $E=\{F(x)\}_{x\in P}$ and for $1\leq i\leq d-1$, $D^{(i)}(r)=F^{(i)}(r)=\{\lambda \Gamma_a(V_{rv_i}): \lambda\in \mathbb{C}\setminus\{0\}\}$. By Proposition 5, $D^{(d)}(r)=\{\lambda T^{rv_d}_{e^{\varepsilon_d}(\xi)}: \lambda\in \mathbb{C}\setminus\{0\}\ \text{and } \xi\in \text{Ker}(V_{rv_d}^*)\}$. Clearly, $D^{(i)}(r)D^{(j)}(s)=D^{(j)}(s)D^{(i)}(r)$ for each $1\leq i,j\leq d$ and $r,s\geq 0$. By Proposition 8, F is decomposable over Q. This implies that E is decomposable over P.

Theorem 4. Let A be a P-space and let E be the product system over P for the CAR flow associated to A of multiplicity k. Then E is decomposable over P if and only if $A=\{x\in \mathbb{R}^d: \langle x|\lambda\rangle \geq 0\}$ for some $\lambda\in P^*$.

Proof. Proof follows from the Theorem 3 and the Proposition 9.

When a P-space A is proper, then the CCR flow and the CAR flow associated to $V^{(A,\mathbb{C})}$ are not cocycle conjugate. Since any P-space of the form $A=\{x\in \mathbb{R}^d: \langle x|\lambda\rangle \geq 0\}$, for some $\lambda\in P^*$, is not proper, so we cannot apply the results of [1]. In fact, we have the following result.

Corollary 2. There are an uncountable many CCR flows cocycle conjugate to CAR flows over P.

Proof. Let $A=\{x\in \mathbb{R}^d: \langle x|\lambda\rangle \geq 0\}$ and $B=\{x\in \mathbb{R}^d: \langle x|\mu\rangle \geq 0\}$ for some $\lambda,\mu \in P^*$. Let α be the CCR flow associated to A with multiplicity k and let β be the CAR flow associated to B with multiplicity l.

We claim that α is cocycle conjugate to β if and only if A = B and k = l. Let E and F be the product systems associated to α and β, respectively. Assume that α is cocycle conjugate to β. Then by [7, Theorem 2.9], E is isomorphic to F as product systems. Observe that the product systems E and F are embeddable and its corresponding isometric representations of P are unitary equivalent to $V^{(A,K)}$ and $V^{(B,L)}$, respectively (see [10, Definition 3.3] and the discussion following that). Since E is isomorphic to F, $V^{(A,K)}$ is unitary equivalent to $V^{(B,L)}$. Hence, A = B and k = l. Conversely, let A = B and k = l. Since F is decomposable and has a unit, by Theorem 1, there exists an isometric representation V of P such that the product system F is isometric to the product system associated to the CCR flow α ^V. Since the isometric representation constructed out of F is $V^{(A,K)}$, V is unitary equivalent to $V^{(A,K)}$. Hence, E is isomorphic to F. Since there are uncountable many P-spaces of the form $A=\{x\in \mathbb{R}^d: \langle x|\lambda\rangle \geq 0\}$ for $\lambda\in P^*$, the proof is complete.