The importance of population density and inter-individual distance in conserving the European oyster Ostrea edulis

Abstract

Fertilization success will determine the rate at which a population can expand and is especially important when considering small, establishing or enduring communities. Introduced species frequently fail to establish reproductively functional populations due to strong Allee effects associated with low densities. The native European oyster, Ostrea edulis broods its fertilized eggs in the pallial cavity for a period of 8–10 days before releasing the larvae. It is considered a partial broadcast spawner and was used as a model species to assess the importance of Allee effects such as inter-individual distance on reproductive success. Distances between individual oysters within test plots in areas of known oyster density were used in conjunction with standardized brood size (n larvae g⁻¹ total wet weight) to assess fertilization success. A significant, positive relationship was observed between brood size and oyster density. Oysters with a nearest neighbour ≤1.5 m were found to brood significantly more larvae than individuals with nearest neighbours ≥1.5 m. Therefore, high density sites need to be maintained to ensure the recovery and enhancement of this OSPAR Convention recognized species in decline.

INTRODUCTION

The procreative success of sexually reproducing species is determined by a number of factors. Two of the key influences are maternal size and population density (Beukema, 1982). The concept that small populations may suffer declines in relation to per capita growth as a result of their size or low densities was first suggested by Allee in 1931. This idea was further explored in his subsequent book on animal ecology in 1949 (Allee et al., 1949). The concept of the Allee effect has been a widely accepted theory adopted by researchers investigating species facing the threat of extinction as a consequence of low population densities and low fecundity (Stephens & Sutherland, 1999; Barnett, 2001). However, it is equally important when examining species which are recovering from population crashes or ones which have only recently become established (Arim et al., 2006). By its nature, an invading species is either on the very edge of its native range and colonizing new areas naturally or it has been translocated and is in a low density founding population (Hayes & Barry, 2008). The same scenario can be anthropogenically induced in species, whereby the wild population or its associated habitat is overexploited as a resource to such an extent that decreases in densities resemble those of an invading species (Smyth et al., 2009; Campos-Silva et al., 2017; Sullivan et al., 2017). Once species reach critical densities that make biological functionality a challenge conditions are ideal for the initiation of the Allee effect, which can have major impacts on subsequent population dynamics (Taylor & Hastings, 2005). With regards to invasive species biology, the Allee effect can be considered beneficial as it slows population density increase through the introduction of a prolonged lag phase in population growth. This phenomenon affords researchers time to acquire information and initiate decisive management control measures. The Allee effect may even be severe enough in some cases to prevent population expansion beyond the initial cohort of introduced individuals (Tobin et al., 2011; Fauvergue et al., 2012).

There are numerous influencing parameters which have been associated with the Allee effect but one of the major components is the availability of a mate, an important requirement in the perseverance of a fecund population (Wells et al., 1998; Boukal & Berec, 2002; Kramer et al., 2009).

Many broadcast spawning marine invertebrate species exhibit traits which serve to maximize fertilization success (Gosling, 2003). In sessile species chemically induced gregarious settlements are common (Tamburri et al., 2008). In vagile species external environmental factors such as the lunar cycle (Rudloe, 2008) or temperature (Mann, 1979) are inclined to trigger a mass movement to ensure the close proximity of potential mates and synchronicity in spawning (Yund, 2000). The distance between potential mates, the number of gametes produced and the longevity of gametes in the water column plus localized dilution factors can all have major implications for successful fertilization in broadcast spawners (Breen & Adkins, 1980; Shepherd, 1986; Gascoigne & Lipcius, 2004). Population densities of sedentary sessile species can therefore be considered as fundamental determinants of fitness since individuals cannot move to find a mate (Cruz-Rivera & Hay, 2000).

The European flat oyster Ostrea edulis is a sequentially alternating species, whereby individuals develop firstly as males and after spawning switch sex to produce eggs (Korringa, 1952). This alternation between sexes is thought to continue throughout the lifetime of the oyster (Orton, 1922; Walne, 1974). It may be possible that some overlap in the presence of spermatozoa and eggs exists, however there have been no documented reports of O. edulis functioning as both sexes simultaneously. Females are larviparous and extrude their eggs into their mantle space where they are retained until fertilized by conspecific sperm acquired from the water column (Orton, 1937). Post-fertilization, the eggs are moved to the inhalant chamber where they develop for ~1 week (Orton, 1937). After 3–4 days the larvae develop shells and cilia to facilitate swimming (Walne, 1974). During this period larvae change from a translucent whitish colour to miniature, dark grey shelled veligers (Korringa, 1952). Within ~10 days the larvae are expelled from the maternal mantle cavity into the plankton where they undergo further development. Spawning takes place from June to mid-September and spat are visible from late summer onwards (Kennedy & Roberts, 1999). The fact that O. edulis females retain larvae in the mantle makes it possible to gauge fertilization success (Adams et al., 2008; Lallias et al., 2010) and it was this reproductive trait which enabled size–fecundity relationships in the European oyster to be established over 25 years ago (Korringa, 1952; Walne, 1974).

The present study is the first to explore the effects of population density and spatial distribution on fertilization success in the European oyster, O. edulis in Strangford Lough, Northern Ireland. This study was undertaken in an attempt to model fertilization success in oysters with the ultimate aim of designing strategies to enhance local range and population size of the native O. edulis. It is envisaged that the findings will have a broader application in similar global scenarios.

MATERIALS AND METHODS

Study area

Strangford Lough is a designated UK Marine Nature Reserve located on the north-east coast of Ireland and lies between 54^o35–54^o20N and 5^o41–5^o34W enclosing an area of 150 km²(Figure 1). The average depth of the lough is ~30 m but depths range from 14–60 m, with substrate types varying from bedrock to fine sediments winnowed out by a gradient of tidal water movement (Erwin, 1976). The lough can be divided into northern and southern basins. Tidal currents are weak in the north and strong (~3.5 m s⁻¹) in the bottleneck entrance in the south (Kregting & Elsäer, 2014).

Fig. 1. Strangford Lough Northern Ireland and the three survey sites of Newtownards Sailing Club (NSC), Greyabbey and Kircubbin.

Density and inter-individual distance

The three survey sites, Newtownards Sailing Club (NSC), Greyabbey and Kircubbin (Figure 1), were selected as they represented high, medium and low density O. edulis populations, as acknowledged in Guy & Roberts (2010) and Smyth et al. (2018). Sampling was undertaken during July and August in 2009 when O. edulis females were most likely to be brooding (Orton, 1937; Korringa, 1952; Walne, 1974). All sites were visited on spring tides with a predicted low water of <0.5 m below chart datum with sampling conducted during the 30 min before and after low water.

At each site, five 36 m² plots were selected parallel to the low water mark. Within each 36 m² plot, six 1 m² quadrats were randomly positioned as described by Low (2006). A thorough visual census within each quadrat was carried out and the location of all O. edulis recorded diagrammatically and in digital images. The first three O. edulis found within a quadrat were used for subsequent measurements. The three nearest conspecifics to each individual inside the quadrat were located and the inter-individual distances recorded using a Bosch Lazer Rule^©. The length of each oyster was also noted alongside the inter-individual distance. Each oyster was assigned a unique reference code before being collected and stored in a correspondingly labelled container.

Patterns of dispersion

Inter-individual distances between O. edulis at the three survey sites were used to assess how spatial distribution patterns of the species varied depending on the overall mean oyster density of the site. Morisita's index of dispersion (I _d) and the standardized Morisita index of dispersion were calculated for each of the three sites as per Krebs (1989).

First, the Morisita index of dispersion (I _d) was calculated by:

(1)$$I_{\rm d} = n\left[ {\displaystyle{{\Sigma {\rm \chi} ^2 - \Sigma {\rm \chi}} \over {\{ \Sigma {\rm \chi} ^2 \} - \Sigma {\rm \chi}}}} \right]$$

Where: I _d = Morisita's index of dispersion; n = sample size; Σ_χ = sum of the quadrat counts; Σχ² = sum of the quadrat counts squared.

Additions were made to this equation by Smith-Gill (1975) to implement an absolute scale from −1 to + 1 resulting in the Standardized Morisita index of dispersion (I _p) (Krebs, 1989). I _p is derived by calculating the uniform index (M _u) (equation (2)) and the clumped index (M _c) (equation (3)).

(2)$$M_{\rm u} = \displaystyle{{{\rm \chi} ^2 \times .97_5 - {\frak n} + \Sigma {\rm \chi} i} \over {\{ \Sigma {\rm \chi} i\} - 1}}$$

Where: χ² × .97₅ = Value of the chi-square from table with n − 1 degrees of freedom with 97.5% of area to the right; χi = number of organisms in quadrat i.

(3)$$M_{\rm c} = \displaystyle{{{\rm \chi} ^2 \times 02_5 - {\frak n} + \Sigma {\rm \chi} i} \over {\{ \Sigma {\rm \chi} i\} - 1}} $$

Where: χ² × 02₅ = Value of the chi-square from table with n − 1 degrees of freedom with 2.5% of area to the right.

The final calculation to ascertain the standardized Morisita index (I_p) was chosen dependent on the outcomes of equations (2) and (3).

When I _d > M _c ≥ 1.0:

(4)$$I_{\rm p} = 0.5 + 0.5\left( {\displaystyle{{I_{\rm d} - M_{\rm c}} \over {{\frak n} - M_{\rm c}}}} \right) $$

When M _c > I _d > 1.0:

(5)$$I_{\rm p} = 0.5\left( {\displaystyle{{I_d - 1} \over {M_c - 1}}} \right) $$

When 1.0 > I _d > M _u

(6)$$I_{\rm p} = - 0.5\left( {\displaystyle{{I_{\rm d} - 1} \over {M_{\rm u} - 1}}} \right)$$

When 1.0 > M _u > I _d

(7)$$ I_{\rm p} = - 0.5 + 0.5\left( {\displaystyle{{I_d - M_{\rm u}} \over {M_{\rm u}}}} \right) $$

I _p ranges from −1.0 to + 1.0 with 96% confidence limits at + 0.5 and −0.5. Populations with an I _p result of zero are classed as randomly distributed, a positive I _p result suggests clumped distribution patterns and negative I _p results suggest uniform distribution.

Brood density analysis

Presence and density of brooded larvae were used to assess the impact of oyster density and inter-individual distance on fertilization success in O. edulis. The collected oysters were brought back to the laboratory for immediate processing. The initial weight of the oyster was recorded prior to the removal of the left valve. The mantle cavity was then checked for evidence of larval brooding. Brooding individuals could be clearly distinguished as they had masses of white/grey/black particles in the gill area, visible to the naked eye. The mantle of all oysters was then carefully rinsed using a measured amount of filtered seawater to remove all larvae which were collected in a glass beaker for subsequent analysis (Utting et al., 1991). Beakers containing washings from brooding oysters were gently agitated to ensure larvae were in an even suspension. An aliquot of liquid (5 ml) was then removed from the beaker and placed into a Bogorov tray. The Bogorov tray was divided into 17 equal portions, of which five were randomly selected for larval counts. Each portion contained 0.29 ml of liquid and therefore the total density of larvae being brooded could be back-calculated taking the dilution factors into account (Utting et al., 1991). As brood size is positively related to the size of the brooding oyster (Walne, 1974) brood-size data were standardized to n larvae g⁻¹ total wet weight.

RESULTS

Density, inter-individual distance and patterns of dispersion

The distribution patterns of O. edulis were assessed at NSC, Greyabbey and Kircubbin which correspondingly displayed high, medium and low density populations (Table 1). The O. edulis population at NSC was found to have a standardized Morisita index of dispersion (I _p) of −0.56 which denotes a uniform pattern of dispersion. Greyabbey was found to have a clumped O. edulis population distribution (I _p = 0.149) and Kircubbin was found to have a randomly distributed population of O. edulis (I _p = 0).

Table 1. Mean density, inter-individual distance (cm) and dispersion indices of O. edulis across the three sample sites.

Brood densities

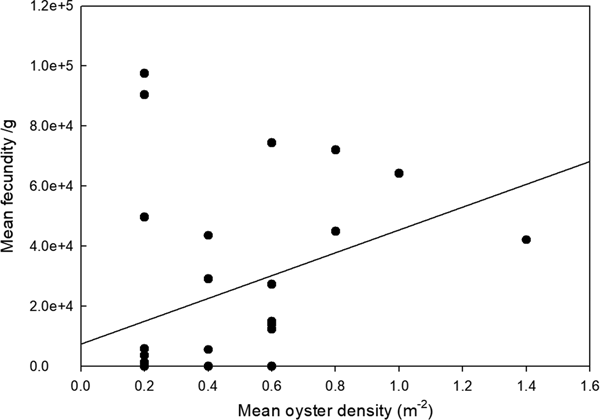
To allow for variation in oyster size and weight, larval brooding was expressed as n g⁻¹ total wet (flesh) weight. Number of brooded larvae showed a significant positive relationship with oyster density (ANOVA, F _1,28 = 4.445, P = 0.044) (Figure 1). The percentage of population found to be brooding larvae was considered positively orientated, but not significantly (ANOVA, F _2,164 = 0.078, NS), related to oyster density (Figure 2).

Fig. 2. Relationship between mean fecundity of larvae (n g⁻¹ wet tissue weight) and oyster density (pooled data – all sites).

As oyster densities and distribution patterns differed between sites (Table 1) the data were investigated further to assess if inter-site differences in distribution pattern were reflected in brood densities. The data were then subdivided into ≤1.5 m and >1.5 m to take into account the distance to the nearest neighbour.

Although differences were present in the distribution patterns and density of O. edulis at the three sites, there was no significant variation in the mean inter-individual distances between sites (ANOVA, F _2,164 = 0.030, NS). However, within each site, oysters which were ≤1.5 m from their nearest neighbour were brooding larvae in significantly higher densities than those which were >1.5 m from their nearest neighbour (ANOVA, F _1,37 = 6.730, P = 0.014) (Figure 3).

Fig. 3. Relationship between percentage of brooding oysters and oyster density (pooled data – all sites).

The three sites revealed a gradual weakening of the Allee effect and brood density (Figure 4) as oyster density per m² decreased from the northerly high density site of NSC through to the low density site of Kircubbin (Table 1). It was also evident that a linkage existed between oyster settlement patterns indicated by the Morisita index and the strength of the Allee effect. As oyster settlements changed from grouped attachments at high density assemblages through to single settlements at low density the Allee effect strengthened.

Fig. 4. Mean brood density (n larvae g⁻¹ wet tissue weight) per n × 17 samples at three different sites where the nearest neighbour was ≤1.5 m (open) and >1.5 m (shaded). Error bars represent the standard deviation of the mean.

DISCUSSION

Fertilization success for broadcast spawning bivalves such as O. edulis increases with the age and size of individuals; as the oyster becomes larger it produces greater quantities of eggs and sperm (Walne, 1974). A major influence in limiting fertilization success in O. edulis is gamete dilution: a parameter which is more pronounced in fragmented and low-density populations (Oliver & Babcock, 1992; Farley & Levitan, 2001; Vitiello et al., 2011). This scenario existed between the three survey sites examined during this research. Gamete dilution is also a factor in mobile broadcast spawning species such as polychaete worms. However, reduced fertilization success at low population densities of these mobile species can be partially or at least offset by behavioural adaptations such as the formation of synchronized spawning swarms (Coma & Lasker, 1997; Santu et al., 2016). Such strategies are not possible for sessile broadcast spawners. Monitoring fertilization success of broadcast spawners in the field would necessitate detailed plankton studies with a low likelihood of success. Ostrea edulis provides an ideal model for alternatives to this research approach as it is a partial broadcast spawner in which females retain eggs within the mantle cavity while they undergo post-fertilization development (Walne, 1974).

This study examined the fertilization success of O. edulis over a range of low density sites which are representative of many of the wild standing stock levels in Europe at the present time (Bostock et al., 2016; Smyth et al., 2018). The research revealed that the O. edulis brooding larvae percentage was positively but not significantly related to density (Figure 3). The weak relationship between percentage brooding and density was not unexpected as brooding percentage simply demonstrates that females within the population have been fertilized and not the rate of fertilization. However, the density of brooded larvae (n larvae g⁻¹ wet tissue weight) was significantly higher in oysters which were <1.5 m away from their nearest conspecific (Figure 4). Although no significant variation was observed between the inter-individual distances at the three sites there was variation in fertilization success between < and >1.5 m. Consequently the findings suggest that it is a combination of the overall numbers of individuals (Figure 2) and the inter-individual distances (Figure 4) which are governing the fertilization success rate.

The significance of inter-individual distance with regards to the reproductive success of O. edulis reiterates the importance of maintaining high density populations in a recovering oyster stock as a means of ensuring sustainability (Kennedy & Roberts, 1999). Safeguarding a high density stock of an economically important shellfish such as O. edulis is extremely difficult unless sites are closed and policed (Beck et al., 2011; Selkoe et al., 2015). Smyth et al. (2009) highlighted the detrimental impact illegal harvesting could have on a recovering O. edulis population. Decreases of >500,000 were recorded within the commercially marketable 3–5 years cohort between 2004 and 2006 and as result wild stocks have never recovered to the pre-2004 densities (Smyth et al., 2016). These large-scale removals of larger, fecund individuals greatly reduce the number of gametes released and increases inter-individual distance which consequently reduces fertilization success. It was unregulated harvesting such as this that led to the collapse of the O. edulis fishery throughout Europe in the mid-1800s (Hiscock et al., 2013; Thurstan et al., 2013). It is possible that population distributional changes in undisturbed recovering populations could shift randomly at low densities through to intermediate and high densities as a result of larval attraction to conspecifics and naturally occurring abiotic factors. However, the introduction of illegal harvesting into the scenario would reverse this hypothetical trend and natural density patterns would be compromised (Smyth et al., 2016).

The European oyster, Ostrea edulis, has been listed by the OSPAR Convention for the Protection of the Marine Environment of the North-East Atlantic as a threatened species in decline since 2003 and was included within the remit of the UK Biodiversity Plan (OSPAR Commission, 2009; UKBAP, 2009). These environmental advisory concords encourage the maintenance and expansion of existing O. edulis assemblages within their native European range (Hiscock et al., 2013). If the signatories of these agreements are to meet their obligations the illegal harvesting of O. edulis should be prevented. As the continued removal of wild stocks increases inter-individual distances it will in turn reduce fertilization success and generate a strong Allee effect which could eventually drive populations below recruitment thresholds, resulting in extinction (Liebhold & Tobin, 2010).

Species such as O. edulis which are considered as brooding or partial broadcast spawners have a limited dispersal time, producing larvae which spend a relatively short period of time in a planktonic phase and therefore tend to be more aggregated around the parent population. Species which have a longer planktonic juvenile stage such as Crassostrea gigas are more likely to settle diffusely apart from the parent population due to the effect of broadcast spawning events, larval swimming time, settlement behaviour and ultimately adult distribution (Carlon & Olson, 1993). The Pacific oyster C. gigas has spread from its introduced aquaculture sites throughout Europe creating concern that its establishment within the low-mid intertidal zone could threaten the existence of indigenous species (Melo et al., 2010). In Strangford Lough C. gigas is beginning to settle among O. edulis on the lower intertidal and potential for settlements to have detrimental impacts on the small recovering assemblages of native oysters is worrying (Zwershke et al., 2016; Smyth et al., 2018).

At present C. gigas can be considered in the lag phase of population growth in Strangford Lough and this offers concerned habitat managers a time lapse whereby they could accentuate any associated Allee effect. The implementation of anti-invasive control measures such as culling and sacrificial spat collector deployment during this period could hinder the development of large C. gigas assemblages like those currently reported from the Wadden Sea (Nehls et al., 2006). The findings of this research into the Allee effect have identified the appropriate action to take in Strangford Lough, first to sustain the tentative recovering O. edulis assemblages and second to possibly offer a means of coping with a future C. gigas infestation. If these are executed in conjunction with the comprehensive understanding of the hydrodynamic regime (Kregting & Elsäer, 2014) of the Lough, a maximum cull effect on C. gigas could be achieved while a high density O. edulis population is protected. This represents a blueprint which could be repeated on a global scale for similar invasive species which have formed feral populations.