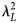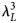Highly miniaturized wideband angular stable linear–circular and linear–cross polarization converter for Ka-band satellite applications

Abstract

This work proposes a novel, extremely miniaturized wideband linear–circular and linear–cross metasurface-enabled reflective polarizer. This design comprises a Meander-line structure with horizontal dipoles as the top frequency selective surface, printed on a 1 mm thin-grounded FR-4 substrate. The x/y-polarized incident electromagnetic wave is converted as circularly polarized from 10.60 to 10.92 GHz (LHCP/RHCP), 12.12 to 17.32 GHz (RHCP/LHCP), and 22.72 to 37.76 GHz (LHCP/RHCP) during reflection with 2.97, 35.33, and 49.74% Fractional Bandwidth (FBW). In addition, this design shows linear–cross conversion with a minimum 90% polarization conversion ratio from 11.41 to 12, 19.01 to 22.34, and 40.74 to 46.82 GHz with an FBW of 5.04, 16.11, and 13.89%. The device performance is considerably stable under different oblique incidences, and the polarizer's unitcell is compact with a structural periodicity of 0.089 × 0.064 $\lambda _L^2$, and ultra-thin low-profile substrate thickness of 0.035λ_L. The proposed prototype is fabricated, and the measured results are in good agreement with the simulated one. This article also mentions how this polarizer could be tuned for dual-band K- and Ka-band satellite applications. The authors believe that the design's novelty lies in the multiband conversion with circular-polarization orthogonality, a highly miniaturized unitcell's volume of 0.199 $\lambda _L^3$/1000, and better angular stability made this design a potential candidate for real-time satellite applications.

Introduction

Wave polarization is an essential feature, regulating the polarization state of the electromagnetic (EM) wave is used in various applications such as polarization manipulation [1], wavefront controlling [2], and optical communication [3]. birefringent-based wave plates, crystalline solids, and liquids modify EM wave polarization [4]. In order to accommodate the hundreds of times greater thickness required by the weak anisotropic natural crystals, these converters are incredibly bulky and consumes more power. The ever-more miniaturized systems necessitate the development of low-profile devices [5].

The development of frequency selective surface (FSS) technology [6, 7] has boosted the researcher's community in the design of different two-dimensional polarizers for advanced wireless applications [8]. Note that circular polarization (CP) is impervious to the effects like Faraday rotation, multipath fading, and polarization mismatch compared to linear polarization [9]. But the inherent drawbacks of circularly polarized antennas like narrow bandwidth, complex feeding, and polarization orthogonality made FSS-based linear-to-circular polarization converters (LCPCs) compulsory candidates to exploit all features like wideband and so on, which are lacking and difficult to implement with direct CP antennas [10].

An H-shaped dipole-based linear–cross and circular–circular reflective polarizer is exhibited for K-and Ka-band applications [11]. Since the circularly polarized wave's rotation sense changes after encountering any barrier, maintaining the wave handiness is also critical in many applications. Michele et al. proposed the optimal design of miniaturized wideband angular stable polarization conversion using the genetic algorithm approach [12]. Zhao et al. proposed a compact and high efficient linear–cross miniaturized w-shaped asymmetric FSS with a polarization conversion ratio (PCR) above 90% from 8.44 to 24.96 GHz [13]. The concentric rectangular pattern-based metasurface is presented to regulate linear and circularly polarized waves in dual bands from 4.40 to 5.30 GHz and 9.45 to 13.60 GHz [14]. Two linear–circular polarizers were presented, i.e. one operating from 6 to 6.5 GHz, and the second being operating in the dual band from 4.58 to 5.13 and 6.0 to 6.4 GHz with bandwidths of 550 and 400 MHz, respectively [15]. The proposed research provides a new rationale for favoring the meta-concrete definition with potential future radome designs. The research on metasurface will focus on developing new fiber-based microwave shielding and cloaking technologies. A broadband ultrathin (~λ/70) linear–circular polarizer with an invertibility feature is demonstrated in [16]. The induced anisotropic optical resonances made this single-layered substrate design able to convert linearly polarized waves into circular ones and the other way around. In addition, broadband LCPCs are implemented in two different modes using solid-state plasma metasurface with a minimum 3 dB axial ratio (AR) bandwidth ranging from 14.34 to 19.61 GHz (mode-1) and 8.81 to 14.34 GHz (mode-2) [17]. The transmission line equivalent circuit approach is detailed for designing dual-band CP converters [18]. Lin et al. proposed an ultra-wideband LCPC with 112% fractional bandwidth (FBW) (5.8–20.4 GHz) utilizing a corner-truncated circular patch [19]. A reflective polarizer that can convert linearly polarized wave into its orthogonal in the lower band (6.53–12.07 GHz) and circularly polarized in the upper band (13.70–15.60 GHz) is presented in [20]. This simple design comprises a 45° tilted dipole separated by two meander-line-like structures. Also, flexible substrate-based linear–cross reflective polarizers for conformal application exhibit an average of 85% PCR in X and Ku-bands [21]. Various polarizers like metallic three-dimensional FSS-based [22] were also introduced, from microwave [23] to optical regimes [24], which are reported in the literature.

In Satcom applications, along with the CP, it is preferred that antennas are required to operate at two distinct, non-adjacent frequency bands in orthogonal polarizations to further enhance the isolation between transmitted and received signals [9]. Due to extremely low power flux densities, the system environment is highly susceptible to interference in real time [8]. Dual-band, dual-CP patch antennas can be used as the terminal antenna in a phased array [25]. However, at Ka-band frequencies, these solutions are either cumbersome and expensive or tiny but inefficient and difficult to make. There is a need for low-profile, low-cost, and easily fabricated devices in the millimeter-wave frequency range and beyond. LCPCs mounted with antennas were used to overcome the abovementioned limitations [26]. In addition, the polarizer metasurface should perform at greater oblique angles since there is no guarantee that the incident wave will always be in the normal direction in real time. As a result, destructive interference occurs at increasingly larger oblique angles and higher frequency ranges due to the increased path taken by the EM wave within a dielectric spacer [11].

By considering all these, a simple reflective polarizer is proposed in this work which performs LCPC in three different bands with a bandwidth of 0.32 GHz (LHCP/RHCP in X-band), 5.2 GHz (RHCP/LHCP in Ku), and 15.04 GHz (LHCP/RHCP in K- and Ka-bands). In addition, it can also perform linear–cross polarization conversion having bandwidths like 0.59 GHz (X-band), 3.33 GHz (K-band), and 6.08 GHz (V-band). The design is highly compacted (unitcell area of 0.569 $\lambda _L^2$/100) with considerable angular stability up to 45° for transverse electric (TE) and transverse magnetic (TM) modes along with the CP orthogonality.

Conversely, a dedicated frequency spectrum is used in Ka-band for downlink (D/L) at 17.7–20.2 GHz and uplink (U/L) at 27–30 GHz for high-speed satellite communications. After receiving a left-handed CP field, the user's ground terminal should broadcast an orthogonal right-handed CP (RHCP) field back to the sender opposite to the received D/L signal. For this purpose, the proposed design's central portion is connected with a metallic strip of a particular width such that the orthogonal LCPC bands shift to 17.73–19.35 GHz (LHCP/RHCP) and 27.02–36.20 GHz (RHCP/LHCP). This design shows its capability to work for dedicated Satcom application frequencies and other bands where the linear–circular (with orthogonality) and linear–cross conversion is the primary concern.

The paper is organized as follows. The design of the unitcell and simulation results are discussed in Section “Unitcell design and the simulation results.” A transfer matrix method (TMM) is employed for analysis, and the extracted equivalent circuit is discussed in Section “Analysis and discussion.” The design can be transformed to work for K- and Ka-band real-time satellite communication frequencies. The device is fabricated, and the obtained result is compared with the simulated one in Section “Prototype fabrication and measured results.” The proposed design performance is compared with the other state-of-the-art designs reported in the literature in Table 3, and finally, the conclusion is drawn in the final section.

Unitcell design and the simulation results

The unitcell of the multiband linear–circular and linear–cross reflective type polarizer is shown in Fig. 1. The top FSS and ground plane are sandwiched on either side of a dielectric spacer. The top FSS constitutes a meander-line-based inductor separated by orthogonal dipoles that generate the capacitance effect. The commercially available FR-4 substrate (relative permittivity $\varepsilon _r$ = 4.4, and loss-tangent tan δ = 0.02) is used as a dielectric spacer, and a 0.035 mm (=t) thin copper film with conductivity σ = 5.8 × 10⁷ S/m is utilized as the metallic layer. Finite-element method-based solver ANSYS HFSS is used to optimize the final unitcell's dimensions for the desired performance by applying appropriate master–slave periodic boundary conditions, mimicking the infinite array. The final unitcell dimensions are: p_x = 1.8 mm, p_y = 2.52 mm, h = 1 mm, s = 2.28 mm, l ₁ = 0.3 mm, l ₂ = 0.36 mm, l ₃ = 0.3 mm, l ₄ = 0.4 mm, w = 0.12 mm, and g = 0.12 mm, as shown in Fig. 1(a). Here, p_x, p_y denote the unitcell periodicity along the x and y axes, h is the substrate thickness, l_i (i = 1–4) are the lengths, w is the line's trace width, and g represents the gap. The reflective type polarization converter is opted for the inherent broadband performance to serve the purpose.

Fig. 1. (a) Top view and (b) side view of the proposed reflective polarizer.

For the y-polarized EM wave incident on the polarizer's metasurface (see Fig. 1), the co-pol r _yy = |E _yr|/|E _yi|, and the cross-pol r _xy = |E _xr|/|E _yi| reflectances are defined. The terms E _i, and E _r refer to the incident and reflected electrical field components, and the indices x, and y represent field oscillating directions.

The simulated reflectances of the polarizer for the y-polarized incidence are shown in Fig. 2. The response in Fig. 2(a) depicts that co-pol reflectance approaches minimum, i.e. −17.88, −39.93, and −12.94 dB at three different resonant frequencies, 11.4, 19.54, and 44.3 GHz. However, the cross-pol reflectance approaches near-unity (≈0 dB), illustrating perfect linear–cross conversion in these three bands. For any reflective polarizer, $PCR = r_{xy}^2 /( {r_{xy}^2 + r_{yy}^2 } )$ defines the efficiency of linear–cross conversion, and the simulated PCR of the proposed design is shown in Fig. 3(a). It shows PCR of above 90% from 11.41–12 GHz (X-band), 19.01–22.34 GHz (K-band), 40.74–46.82 GHz (V-band) with an FBW of 5.04, 16.11, and 13.89%, respectively.

Fig. 2. Simulated (a) magnitudes of r_yy and r_xy. (b) Relative phase (Δϕ).

Fig. 3. Simulated (a) PCR. (b) Absorption and ECR of the reflective polarizer.

In the reflective polarizer's design, it is necessary to know the total amount of the incident energy dissipating in the substrate along with its conversion efficiency. The absorptivity, A(ω) = 1 −|r _yy|² − |r _xy|², and energy conversion ratio (ECR), ECR = |r _yy|² +|r _xy|² are calculated, and the results are depicted in Fig. 3(b). Almost 90% of incident energy is transformed into different polarizations, and the remaining portion is accounted for as the dielectric losses over the operating frequency range. In addition to the linear–cross conversion, the design also demonstrates linear–circular conversion characteristics at other frequency bands. From Fig. 2, the magnitudes of the co- and cross-pol reflectances are almost equal (crossing each other around 3 dB) in three frequency bands (see Fig. 2(a)). The relative phase (Δϕ) between the co- and cross-pol reflectances is calculated, and it is ±90° in those frequency bands, as shown in Fig. 2(b). Since the CP is the ideal case, the efficiency of the linear–circular conversion is measured by the term AR which has been calculated, as in [20]:

(1)$$R = \displaystyle{{r_{xy}} \over {r_{yy}}}$$

(2)$$Schi, \;\;\chi = 0.5\sin ^{{-}1}\left({\displaystyle{{2R\sin ( {\Delta \phi } ) } \over {1 + R^2}}} \right)$$

(3)$$Axial\;Ratio, \;\;AR = \vert {1/\tan ( \chi ) } \vert $$

From Fig. 4(a), it is clear that the proposed converter results in the AR ≤ 3 dB response from 10.60 to 10.92 GHz (X-band), 12.12 to 17.32 GHz (Ku-band), and 22.72 to 37.76 GHz (Ka-band) with 2.97, 35.33, and 49.74% FBW, respectively. In order to detrmine the CP handedbness, the expression for the ellipticity (e) is given below:

(4)$${\rm Ellipticity}, \,e = \displaystyle{{2\vert {r_{xy}} \vert \vert {r_{yy}} \vert \sin ( {\Delta \phi } ) } \over {{\vert {r_{xy}} \vert }^2 + {\vert {r_{yy}} \vert }^2}}$$

Fig. 4. Simulated (a) AR. (b) Ellipticity (e) of the proposed reflective polarizer.

The ellipticity ranges from −1 (RHCP) to +1 (LHCP). Different circular senses of rotation in the consecutive bands are observed from simulated ellipticity, as shown in Fig. 4(b). It is noteworthy that the polarizer is dual-polarized. The handedness of CP interchanges if the y-polarized incidence is changed with the x-polarized.

Next, the performance of the proposed polarizer is subjected to different incident angles from 0° to 45°, in steps of 15° for both TE and TM cases. The simulated PCR and AR (dB) for TE and TM incidences are shown in Figs 5 and 6, respectively. The PCR computation deals with reflectance magnitudes, but the reflection phases explain its behavior better at higher oblique angles [27]. The PCR bandwidth is reduced when the incidence angle is shifted away from the normal. It is also noticed that the cross-pol (r_xy) is nearly unaltered in amplitude and phase, while the co-pol (r_yy) reflectance is highly dependent on the incidence angle. As a result, the extra traveling path between the FSS and ground at higher angles results in a more r_yy phase. This extra phase would deteriorate the PCR performance. It can be calculated by the Bragg diffraction formula [21] as below:

(5)$$\Delta \phi = \phi _{oblique}-\phi _{normal} = 2\sqrt {\varepsilon _r} kd\left({\displaystyle{1 \over {\sqrt {1-( {{\sin }^2\theta /\varepsilon_r} ) } }}-1} \right)$$

Here, θ denotes the incident angle concerning normal, d is the substrate thickness, and k is the wave vector within the substrate. From Fig. 5, the PCR response is almost stable up to 45°, but a small disturbance is observed at a higher frequency region for the TM case at higher oblique angles, as in [11], and the reduction in PCR level is due to weak field couplings at higher angles. The AR (dB) is almost stable up to 45° with a slight reduction of AR (≤3 dB) bandwidth in the higher frequency band (see Fig. 6). The detailed working analysis of the reflective polarizer is included in the subsequent section.

Fig. 5. Simulated PCR for different incident angles under TE for (a) X, Ku, and (b) K, Ka-bands. Under TM incidence of the proposed reflective polarizer for (c) X, Ku, and (d) K, Ka-bands.

Fig. 6. Simulated AR for different incident angles under TE for (a) X, Ku, and (b) K, Ka-bands. Under TM incidence of the proposed reflective polarizer for (c) X, Ku, and (d) K, Ka-bands.

Analysis and discussion

Analysis using TMM

Two axes, u- and v-, are defined at 45° counterclockwise to the x–y to gain insight into the physical mechanism behind the polarization conversion (see Fig. 1). The incident electric field for the wave propagating along the negative z-direction can be written as $\overrightarrow {E_i} = ( E_i^u \tilde{u} + E_i^v \tilde{v}) e^{jkz}$, where $E_i^u$, and $E_i^v$ are the components along u and v-axes. From the TMM technique, the reflected field components can be written as $[ {\overrightarrow {E_r} } ] = [ {R_{lin}} ] [ {\overrightarrow {E_i} } ]$. Here [R _lin] is the reflection matrix as given below:

(6)$$\left[{\matrix{ {E_r^u } \cr {E_r^v } \cr } } \right] = \left[{\matrix{ {r_{uu}} & {r_{uv}} \cr {r_{vu}} & {r_{vv}} \cr } } \right]\left[{\matrix{ {E_i^u } \cr {E_i^v } \cr } } \right]$$

Since the unitcell design is symmetric about uv-axes, the effect of the cross-pol reflectance is minimal and can be neglected. Then, the reflected field component can be written as

(7)$$\overrightarrow {E_r} = ( r_{uu}E_i^u \tilde{u} + r_{vv}E_i^v \tilde{v}) e^{{-}jkz}$$

The simulated magnitudes and phases of the r _uu and r _vv are shown in Fig. 7. From the response shown in Fig. 7(a), the magnitudes of r _uu and r _vv are almost unity over the band with a small dip at frequencies 11.5, 20.3, and 45.6 GHz. The eigenmodes at frequencies 11.5 and 45.6 GHz is stronger (higher Q-factor) than the mode at 20.3 GHz. If the loss of substrate is neglected, then $r_{uu} = e^{j\phi _u}$, $r_{vv} = e^{j\phi _v}$, and Δϕ = ϕ _u − ϕ _v, where Δϕ is the relative phase. The different possible conversions for different magnitudes and phases of r _uu and r _vv are summarized in Table 1. The equivalent circuit of the polarizer is extracted and is detailed in the next section.

Fig. 7. Simulated (a) magnitudes of r_uu and r_vv. (b) Relative phase (Δϕ_uv).

Table 1. Summary of polarization conversion for different magnitudes and relative phases

Equivalent circuit modeling of the proposed converter

The polarizer's transmission line equivalent circuit model for a better understanding of the polarization conversion phenomena is extracted for u- and v-polarized incidences (see Fig. 1), as shown in Fig. 8. A similar procedure is used for the extraction, as detailed in [28]. The top FSS is modeled as a combination of series and parallel LC circuit elements. Accordingly, the metallic conductor and dielectric losses are modeled as lumped resistors. The short circuit is taken to mimic the perfect ground (for reflection type). The transmission line of length “h” with respective characteristic impedance is chosen for the dielectric substrate. Primarily, the inductances and capacitances values are calculated first from the expressions below [29]:

(8)$$L_i = \displaystyle{{\mu _oL} \over {2\pi }}\ln \left({\sin {\left({\displaystyle{{\pi m} \over {2L}}} \right)}^{{-}1}} \right)\;\quad {\rm for}\,i = 1, \;\,2, \;\,3.$$

(9)$$C_j = \displaystyle{{2\varepsilon _o\varepsilon _{eff}L} \over \pi }\ln \left({\sin {\left({\displaystyle{{\pi n} \over {2L}}} \right)}^{{-}1}} \right)\quad {\rm for}\,j = 1, \;\,2, \;\,3.$$

Fig. 8. Equivalent circuit model of the proposed polarizer for (a) u- and (b) v-polarized incidence.

Here, the coupling between the orthogonal components is negligible [9]. The top FSS is modeled series RLC for the u-polarization excitation. Series and parallel LC circuits were combined for v-excitation (see Fig. 8(b)). Here μ _o, $\varepsilon _o$ are the permittivity and permeability of the free space (or vacuum). The $\varepsilon _{eff} = \sqrt {( \varepsilon _r + 1) /2}$ is considered as effective permittivity. The terms m and n are calculated as detailed in [9]. Initially, the calculated values are used in the Keysight ADS circuit simulator, and the curve fitting technique is used to extract the final values of the lumped components, as tabulated in Table 2. The simulated magnitude response of r_uu and r_vv extracted from the circuit simulator is compared with the EM solver response, and a good agreement is observed, as shown in Fig. 9. The surface currents are field distribution for different resonant frequencies are investigated in the next section.

Fig. 9. Reflectances extracted from circuit simulator with EM solver.

Table 2. Lumped components values of the equivalent circuit model

Note: The units for R, L, and C are in Ω, nH, and fF, respectively.

Surface current and electric field distribution analysis

In this section, the surface current distribution of the proposed polarizer of the top FSS and ground are analyzed for a better understanding of the physics lying behind the polarization conversion phenomena. When a polarizer excites at 45°, the symmetric and asymmetric coupling of the electric and magnetic fields induces currents in both top polarizer FSS and ground. From Fig. 10, the response induces in the FSS and ground are antiparallel (180° out of phase) for both the resonant frequencies 11.2 and 19.1 GHz, as shown in Fig. 10.

Fig. 10. Simulated surface distributions of the (a) top FSS, (b) ground at 11.2 GHz, and for the (c) top FSS, (d) ground at 19.1 GHz.

Since the design performs linear–circular conversion along with linear–cross, the electric field distribution profiles have been investigated at 12.6 GHz at different incident phases, as shown in Fig. 11. A plane has been assigned near the proposed polarizer FSS. The simulated electric field pattern depicts that the design converts the y-polarized linear incidence to the RHCP wave. Similar analysis can be carried out for different frequencies to verify the handedness of CP. Next, the proposed design analysis for the transformation to work for the satellite application frequencies is presented.

Fig. 11. Simulated electric field patterns at the incident phase: (a) 0°, (b) 90°, (c) 180°, and (d) 270° for 12.6 GHz.

Transforming the proposed polarizer for K- and Ka-band satellite communication

In the future of wireless applications, satellite communications will be increasingly significant. A dual-band dual-polarization in adjacent mode CP antenna is required for K/Ka-band satellite communications. As a result, it should be capable of operating from 17.7 to 20.2 and 27 to 30 GHz simultaneously with an improved polarization mismatch ratio [26]. The highly miniaturized multiband linear–circular with CP orthogonality in the consecutive bands reflective polarizer is demonstrated in the proposed work (see Fig. 1). This design shows linear–circular polarization in operational bands, i.e. 10.60–10.92, 12.12–17.32, and 22.72–37.76 GHz. But the design is easily configurable to resonate in the Satcom application frequencies by simply connecting the two dipoles with the metallic strip of width w ₁, as shown in Fig. 12(a). The value of w ₁ was found to be 0.14 mm by parametric optimization. The simulated AR of the modified cell is compared with the earlier response without a metallic strip connected between the two dipoles, as shown in Fig. 12(b). It shows 3 dB AR in the frequency range from 17.73 to 19.35 and 27.02 to 36.20 GHz covering the K- and Ka-bands of the satellite applications.

Fig. 12. Comparison of the AR variation for different configurations of the proposed cell.

Prototype fabrication and measured results

For the validation of the simulated results, the prototype of a 30 × 40 array (75.6 × 72 mm²) is fabricated using conventional printed circuit board technology (see Fig. 13(a)). The free-space measurement method is adopted to extract the fabricated sample's reflectances [27], as depicted in Fig. 13(b). This setup consists of two high-gain horn antennas (transmitting and receiving), connected to the Keysight Power Network Analyzer (PNA) N5224B through high-end RF connecting probes operating from 10 MHz to 43.5 GHz. The sample is placed in the far-field zone of the horn antennas and is aligned at 45° (see Fig. 13(b)), at a distance of 30 cm from the antennas. These antennas were separated by 5° to ensure maximum power incidence from the transmitter and reflected to the receiver under normal incidence. The PNA calibration is done by a perfect electric conductor having similar dimensions as the prototype. The receiver antenna is tilted orthogonally by 90° with reference to the transmitter for the cross-pol measurement. For the co-pol measurement, antennas are aligned in the same polarization plane (see Fig. 13(b)), and responses are depicted in Fig. 14. The measured PCR and AR are depicted in Figs 15 and 16, respectively. The performance of the polarizer for different oblique angles under TE and TM modes is investigated. For the TE case, antennas were placed and moved away in steps of 15° from 0° to 45°, such that the incident electric field from the transmitter is always tangential to the metasurface. The antennas were orthogonally oriented for the TM mode of incidences, and the same steps were repeated. The PCR and AR (dB) results for TE and TM are plotted in Figs 17 and 18, respectively. The measured results are in a good agreement with the simulated one. From the authors’ knowledge, the proposed design is ultra-compact, with low-profile thickness, showing multi-polarization conversion of both linear–circular (with a different sense of CP), and linear–cross with considerable angular stability. These features make this design suitable for real-time sub-miniaturized applications. It will be clear by comparing the proposed polarizer performance with other recently reported state-of-the-art designs reported in the literature (see Table 3).

Fig. 13. (a) The top-view of the fabricated prototype. (b) Free-space measurement experimental setup.

Fig. 14. Comparison of simulated versus measured reflectances (r_yy and r_xy) for (a) X, Ku, and (b) K, Ka-bands.

Fig. 15. Comparison of simulated versus measured PCR for (a) X, Ku, and (b) K, Ka-bands.

Fig. 16. Comparison of simulated versus measured AR (dB) for (a) X, Ku, and (b) K, Ka-bands.

Fig. 17. Comparison of the measured PCR with simulated one under TE for (a) X, Ku, and (b) K, Ka-bands. Under TM incidence of the proposed reflective polarizer for (c) X, Ku, and (d) K, Ka-bands.

Fig. 18. Comparison of the measured AR (dB) with simulated one under TE for (a) X, Ku, and (b) K, Ka-bands. Under TM incidence of the proposed reflective polarizer for (c) X, Ku, and (d) K, Ka-bands.

Table 3. Performance comparison of the proposed design with other recently reported state-of-the-art reflective type polarizers

Conclusion

This article proposes a multiband linear–circular and linear–cross reflective type polarization converter for different X–Ka band applications. This simple design comprises a meander-line connected to horizontally oriented dipoles on a dielectric substrate. The performance is stable for the oblique incidences in TE and TM cases. Multiple plasmonic resonances are the reason behind the wideband characteristics for different polarization conversions. This structure's novelties lie in the orthogonal circular rotation in consecutive bands, highly miniaturized unitcell architecture possesses more than one polarization conversion in different bands with better angular stability. To the best of the authors' knowledge, these attractive features make the design suitable for satellite communication applications.