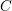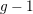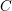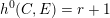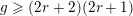Tangent cones to generalised theta divisors and generic injectivity of the theta map

Abstract

Let $C$ be a Petri general curve of genus $g$ and $E$ a general stable vector bundle of rank $r$ and slope $g-1$ over $C$ with $h^{0}(C,E)=r+1$. For $g\geqslant (2r+2)(2r+1)$, we show how the bundle $E$ can be recovered from the tangent cone to the generalised theta divisor $\unicode[STIX]{x1D6E9}_{E}$ at ${\mathcal{O}}_{C}$. We use this to give a constructive proof and a sharpening of Brivio and Verra’s theorem that the theta map $\mathit{SU}_{C}(r){\dashrightarrow}|r\unicode[STIX]{x1D6E9}|$ is generically injective for large values of $g$.

1 Introduction

Let $C$ be a nonhyperelliptic curve of genus $g$ and $L\in \operatorname{Pic}^{g-1}(C)$ a line bundle with $h^{0}(C,L)=2$ corresponding to a general double point of the Riemann theta divisor $\unicode[STIX]{x1D6E9}$ . It is well known that the projectivised tangent cone to $\unicode[STIX]{x1D6E9}$ at $L$ is a quadric hypersurface $R_{L}$ of rank less than or equal to $4$ in the canonical space $|K_{C}|^{\ast }$ , which contains the canonically embedded curve.

Quadrics arising from tangent cones in this way have been much studied: Green [Gre84] showed that the $R_{L}$ span the space of all quadrics in $|K_{C}|^{\ast }$ containing $C$ ; and both Kempf and Schreyer [KS88] and Ciliberto and Sernesi [CS92] have used the quadrics $R_{L}$ in various ways to give new proofs of Torelli’s theorem.

In another direction: via the Riemann–Kempf singularity theorem [Kem73], one sees that the rulings on $R_{L}$ cut out the linear series $|L|$ and $|K_{C}L^{-1}|$ on the canonical curve. Thus the data of the tangent cone and the canonical curve allow one to reconstruct the line bundle $L$ . In this paper we study a related construction for vector bundles of higher rank.

Let $V\rightarrow C$ be a semistable vector bundle of rank $r$ and integral slope $h$ . We consider the set

(1) $$\begin{eqnarray}\{M\in \operatorname{Pic}^{g-1-h}(C):h^{0}(C,V\otimes M)\geqslant 1\}.\end{eqnarray}$$

It is by now well known that for general $V$ , this is the support of a divisor $\unicode[STIX]{x1D6E9}_{V}$ algebraically equivalent to a translate of $r\cdot \unicode[STIX]{x1D6E9}$ . If $V$ has trivial determinant, then in fact $\unicode[STIX]{x1D6E9}_{V}$ , when it exists, belongs to $|r\unicode[STIX]{x1D6E9}|$ .

For general $V$ , the projectivised tangent cone ${\mathcal{T}}_{M}(\unicode[STIX]{x1D6E9}_{V})$ to $\unicode[STIX]{x1D6E9}_{V}$ at a point $M$ of multiplicity $r+1$ is a determinantal hypersurface of degree $r+1$ in $|K_{C}|^{\ast }$ (see, for example, Casalaina-Martin and Teixidor i Bigas [CT11]). Our first main result (§ 3.2) is a construction which from ${\mathcal{T}}_{M}(\unicode[STIX]{x1D6E9}_{V})$ recovers the bundle $V\otimes M$ , up to the involution $V\otimes M\mapsto K_{C}\otimes M^{-1}\otimes V^{\ast }$ . This is valid whenever $V\otimes M$ and $K_{C}\otimes M^{-1}\otimes V^{\ast }$ are globally generated.

We apply this construction to give an improvement of a result of Brivio and Verra [BV12]. To describe this application, we need to recall some more objects. Write $\mathit{SU}_{C}(r)$ for the moduli space of semistable bundles of rank $r$ and trivial determinant over $C$ . The association $V\mapsto \unicode[STIX]{x1D6E9}_{V}$ defines a map

(2) $$\begin{eqnarray}{\mathcal{D}}:\mathit{SU}_{C}(r){\dashrightarrow}|r\unicode[STIX]{x1D6E9}|=\mathbb{P}^{r^{g}-1},\end{eqnarray}$$

called the theta map. Drezet and Narasimhan [DN89] showed that the line bundle associated to the theta map is the ample generator of the Picard group of $\mathit{SU}_{C}(r)$ . Moreover, the indeterminacy locus of ${\mathcal{D}}$ consists of those bundles $V\in \mathit{SU}_{C}(r)$ for which (1) is the whole Picard variety. This has been much studied; see, for example, Pauly [Pau10], Popa [Pop99] and Raynaud [Ray82].

Brivio and Verra [BV12] showed that ${\mathcal{D}}$ is generically injective for a general curve of genus $g\geqslant \binom{3r}{r}-2r-1$ , partially answering a conjecture of Beauville [Bea06, §6]. We apply the aforementioned construction to give the following sharpening of Brivio and Verra’s result.

Theorem 1.1. For $r\geqslant 2$ and $C$ a Petri general curve of genus $g\geqslant (2r+2)(2r+1)$ , the theta map (2) is generically injective.

In addition to giving the statement for several new values of $g$ when $r\geqslant 3$ (our lower bound for $g$ depends quadratically on $r$ rather than exponentially), our proof is constructive, based on the method mentioned above for explicitly recovering the bundle $V$ from the tangent cone to the theta divisor at a point of multiplicity $r+1$ . This gives a new example, in the context of vector bundles, of the principle apparent in [KS88] and [CS92] that the geometry of a theta divisor at a sufficiently singular point can encode essentially all the information of the bundle and/or the curve.

Our method works for $r=2$ , but in this case much more is already known: Narasimhan and Ramanan [NR69] showed, for $g=2$ and $r=2$ , that ${\mathcal{D}}$ is an isomorphism $\mathit{SU}_{C}(2)\xrightarrow[{}]{{\sim}}\mathbb{P}^{3}$ , and van Geemen and Izadi [vGI01] generalised this statement to nonhyperelliptic curves of higher genus. Note that our proof of Theorem 4.1 is not valid for hyperelliptic curves (see Remark 4.3).

Here is a more detailed summary of the paper. In § 2, we study semistable bundles $E$ of slope $g-1$ for which the Petri trace map

$$\begin{eqnarray}\bar{\unicode[STIX]{x1D707}}:H^{0}(C,E)\otimes H^{0}(C,K_{C}\otimes E^{\ast })\rightarrow H^{0}(C,K_{C})\end{eqnarray}$$

is injective. A bundle $E$ with this property will be called Petri trace injective. We prove that for large enough genus, the theta divisor of a generic $V\in \mathit{SU}_{C}(r)$ contains a point $M$ of multiplicity $r+1$ such that $V\otimes M$ and $K_{C}\otimes M^{-1}\otimes V^{\ast }$ are Petri trace injective and globally generated.

Now suppose that $E$ is a vector bundle of slope $g-1$ with $h^{0}(C,E)\geqslant r+1$ . If $\unicode[STIX]{x1D6E9}_{E}$ is defined, then the tangent cone to $\unicode[STIX]{x1D6E9}_{E}$ at ${\mathcal{O}}_{C}$ is a determinantal hypersurface in $|K_{C}|^{\ast }=\mathbb{P}^{g-1}$ containing the canonical embedding of  $C$ . We prove (Proposition 3.3 and Corollary 3.5) that if $C$ is a general curve of genus $g\geqslant (2r+2)(2r+1)$ , and $E$ a globally generated Petri trace injective bundle of rank $r$ and slope $g-1$ with $h^{0}(C,E)=r+1$ , then the bundle $E$ can be reconstructed up to the involution $E\mapsto K_{C}\otimes E^{\ast }$ from a certain determinantal representation of the tangent cone to $\unicode[STIX]{x1D6E9}_{E}$ at ${\mathcal{O}}_{C}$ . By a classical result of Frobenius (whose proof we sketch in Proposition 3.7), any two such representations are equivalent up to transpose. The generic injectivity of the theta map for a Petri general curve (Theorem 4.1) can then be deduced by combining these facts and the statement in § 2 that the theta divisor of a general $V\in \mathit{SU}_{C}(r)$ contains a suitable point of multiplicity $r+1$ .

We assume throughout that the ground field is $\mathbb{C}$ . The reconstruction of $E$ from its tangent cone in § 3.2 is valid for an algebraically closed field of characteristic zero or $p>0$ not dividing $r+1$ .

2 Singularities of theta divisors of vector bundles

2.1 Petri trace injective bundles

Let $C$ be a projective smooth curve of genus $g\geqslant 2$ . Let $V\rightarrow C$ be a stable vector bundle of rank $r\geqslant 2$ and integral slope $h$ , and consider the locus

(3) $$\begin{eqnarray}\{M\in \operatorname{Pic}^{g-1-h}(C):h^{0}(C,V\otimes M)\geqslant 1\}.\end{eqnarray}$$

If this is not the whole of $\operatorname{Pic}^{g-1-h}(C)$ , then it is the support of the theta divisor $\unicode[STIX]{x1D6E9}_{V}$ .

The theta divisor of a vector bundle is a special case of a twisted Brill–Noether locus

(4) $$\begin{eqnarray}B_{1,g-1-h}^{n}(V):=\{M\in \operatorname{Pic}^{g-1-h}(C):h^{0}(C,V\otimes M)\geqslant n\}.\end{eqnarray}$$

The following is central in the study of these loci (see, for example, Teixidor i Bigas [TiB14, §1]): For $E\rightarrow C$ a stable vector bundle, we consider the Petri trace map:

(5) $$\begin{eqnarray}\bar{\unicode[STIX]{x1D707}}:H^{0}(C,E)\otimes H^{0}(C,K_{C}\otimes E^{\ast })\stackrel{\unicode[STIX]{x1D707}}{\rightarrow }H^{0}(C,K_{C}\otimes \operatorname{End}E)\stackrel{\operatorname{tr}}{\rightarrow }H^{0}(C,K_{C}).\end{eqnarray}$$

Then for $E=V\otimes M$ and $M\in B_{1,g-1-h}^{n}(V)\backslash B_{1,g-1-h}^{n+1}(V)$ , the Zariski tangent space to the twisted Brill–Noether locus $B_{1,g-1-h}^{n}(V)$ at $M$ is exactly $\operatorname{Im}(\bar{\unicode[STIX]{x1D707}})^{\bot }$ . This motivates the following definition.

Definition 2.1. Suppose $E\rightarrow C$ is a vector bundle with $h^{0}(C,E)=n\geqslant 1$ . If the map $\unicode[STIX]{x1D707}$ above is injective, we will say that $E$ is Petri injective. If the composed map $\bar{\unicode[STIX]{x1D707}}$ is injective, we will say that $E$ is Petri trace injective.

Remark 2.2. (1) Clearly, a Petri trace injective bundle is Petri injective. For line bundles, the two notions coincide.

(2) Suppose $V\in \mathit{U}_{C}(r,d)$ where $\mathit{U}_{C}(r,d)$ is the moduli space of semistable rank $r$ vector bundles of degree $d$ . If $E=V\otimes M$ is Petri trace injective for $M\in \operatorname{Pic}^{e}(C)$ , then $B_{1,e}^{n}(V)$ is smooth at $M$ and of the expected dimension

$$\begin{eqnarray}h^{1}(C,{\mathcal{O}}_{C})-h^{0}(C,V\otimes M)\cdot h^{1}(C,V\otimes M).\end{eqnarray}$$

(3) We will also need to refer to the usual generalised Brill–Noether locus

$$\begin{eqnarray}B_{r,d}^{n}=\{E\in \mathit{U}_{C}(r,d):h^{0}(C,E)\geqslant n\}.\end{eqnarray}$$

If $E$ is Petri injective then this is smooth and of the expected dimension

$$\begin{eqnarray}h^{1}(C,\operatorname{End}E)-h^{0}(C,E)\cdot h^{1}(C,E)\end{eqnarray}$$

at $E$ . See, for example, Grzegorczyk and Teixidor i Bigas [GT09, §2].

(4) Petri injectivity and Petri trace injectivity are open conditions on families of bundles ${\mathcal{E}}\rightarrow C\times B$ with $h^{0}(C,{\mathcal{E}}_{b})$ constant. Later, we will discuss the sense in which these properties are ‘open’ when $h^{0}(C,{\mathcal{E}}_{b})$ may vary.

We will also need the notion of a Petri general curve.

Definition 2.3. A curve $C$ is called Petri general if every line bundle on $C$ is Petri injective.

By [Gie82], the locus of curves which are not Petri general is a proper subset of the moduli space $M_{g}$ of curves of genus $g$ , the so-called Gieseker–Petri locus. The hyperelliptic locus is contained in the Gieseker–Petri locus. Apart from this, in general not much is known about the components of the Gieseker–Petri locus and their dimensions. For an overview of known results, we refer to [TiB88, Far05, BS11] and the references cited therein.

Proposition 2.4. Suppose $V$ is a stable bundle of rank $r$ and integral slope $h$ . Suppose $M_{0}\in \operatorname{Pic}^{g-1-h}(C)$ satisfies $h^{0}(C,V\otimes M_{0})\geqslant 1$ , and furthermore that $V\otimes M_{0}$ is Petri trace injective. Then the theta divisor $\unicode[STIX]{x1D6E9}_{V}\subset \operatorname{Pic}^{g-1-h}(C)$ is defined. Furthermore, we have equality $\operatorname{mult}_{M_{0}}\unicode[STIX]{x1D6E9}_{V}=h^{0}(C,V\otimes M_{0})$ .

Proof. Write $E:=V\otimes M_{0}$ . It is well known that via Serre duality, $\bar{\unicode[STIX]{x1D707}}$ is dual to the cup product map

$$\begin{eqnarray}\bigcup :H^{1}(C,{\mathcal{O}}_{C})\rightarrow \operatorname{Hom}(H^{0}(C,E),H^{1}(C,E)).\end{eqnarray}$$

By hypothesis, therefore, $\bigcup$ is surjective. Since $E$ has Euler characteristic zero, $h^{0}(C,E)=h^{1}(C,E)$ . Hence there exists $b\in H^{1}(C,{\mathcal{O}}_{C})$ such that $\cdot \cup b:H^{0}(C,E)\rightarrow H^{1}(C,E)$ is injective. The tangent vector $b$ induces a deformation of $M_{0}$ and hence of $E$ , which does not preserve any nonzero section of $E$ . Therefore, the locus

$$\begin{eqnarray}\{M\in \operatorname{Pic}^{g-1-h}(C):h^{0}(C,V\otimes M)\geqslant 1\}\end{eqnarray}$$

is a proper sublocus of $\operatorname{Pic}^{g-1-h}(C)$ , so $\unicode[STIX]{x1D6E9}_{V}$ is defined. Now we can apply Casalaina-Martin and Teixidor i Bigas [CT11, Proposition 4.1] to obtain the desired equality $\operatorname{mult}_{M_{0}}\unicode[STIX]{x1D6E9}_{V}=h^{0}(C,V\otimes M_{0})$ .◻

2.2 Existence of good singular points

In this section, we study global generatedness and Petri trace injectivity of the bundles $V\otimes M$ for $M\in B_{1,g-1}^{r+1}(V)$ for general $C$ and $V$ . The main result of this section is the following theorem.

Theorem 2.5. Suppose $C$ is a Petri general curve of genus $g\geqslant (2r+2)(2r+1)$ and $V\in \mathit{SU}_{C}(r)$ a general bundle. Then there exists $M\in \unicode[STIX]{x1D6E9}_{V}$ such that $h^{0}(C,V\otimes M)=r+1$ , and both $V\otimes M$ and $K_{C}\otimes M^{-1}\otimes V^{\ast }$ are globally generated and Petri trace injective.

The proof of this theorem has several ingredients. We begin by constructing a stable bundle $E_{0}$ with some of the properties we are interested in. Let $F$ be a semistable bundle of rank $r-1$ and degree $(r-1)(g-1)-1$ , and let $N$ be a line bundle of degree $g$ .

Lemma 2.6. A general extension $0\rightarrow F\rightarrow E\rightarrow N\rightarrow 0$ is a stable vector bundle.

Proof. Any subbundle $G$ of $E$ fits into an exact diagram

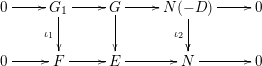
where $D$ is an effective divisor on $C$ . If $\unicode[STIX]{x1D704}_{2}=0$ , then $\unicode[STIX]{x1D707}(G)=\unicode[STIX]{x1D707}(G_{1})\leqslant \unicode[STIX]{x1D707}(F)<\unicode[STIX]{x1D707}(E)$ . Suppose $\unicode[STIX]{x1D704}_{2}\neq 0$ , and write $s:=\operatorname{rk}(G_{1})$ . If $s\neq 0$ , the semistability of $F$ implies that

$$\begin{eqnarray}\deg (G_{1})\leqslant s(g-1)-\frac{s}{r-1},\end{eqnarray}$$

so in fact $\deg (G_{1})\leqslant s(g-1)-1$ . As $\deg (N)=g$ , we have $\deg (G)\leqslant (s+1)(g-1)$ . Thus we need only exclude the case where $\deg (G_{1})=s(g-1)-1$ and $D=0$ , so $\unicode[STIX]{x1D704}_{2}=\operatorname{Id}_{N}$ . In this case, the existence of the above diagram is equivalent to $[E]=(\unicode[STIX]{x1D704}_{1})_{\ast }[G]$ for some extension $G$ , that is, $[E]\in \operatorname{Im}((\unicode[STIX]{x1D704}_{1})_{\ast })$ .

It therefore suffices to check that

$$\begin{eqnarray}(\unicode[STIX]{x1D704}_{1})_{\ast }:H^{1}(C,\operatorname{Hom}(N,G_{1}))\rightarrow H^{1}(C,\operatorname{Hom}(N,F))\end{eqnarray}$$

is not surjective. This follows from the fact, easily shown by a Riemann–Roch calculation, that $h^{1}(C,\operatorname{Hom}(N,F/G_{1}))>0$ .

If $s=0$ , then we need to exclude the lifting of $G=N(-p)$ for all $p\in C$ , that is,

$$\begin{eqnarray}[E]\not \in \mathop{\bigcup }_{p\in C}(\operatorname{Ker}(H^{1}(C,\operatorname{Hom}(N,F))\rightarrow H^{1}(C,\operatorname{Hom}(N(-p),F)))).\end{eqnarray}$$

A dimension count shows that this locus is not dense in $H^{1}(C,\operatorname{Hom}(N,F))$ .◻

Lemma 2.7. Suppose $h^{0}(C,N)\geqslant h^{1}(C,F)$ . Then for a general extension $0\rightarrow F\rightarrow E\rightarrow N\rightarrow 0$ , the coboundary map is surjective.

Proof. Clearly it suffices to exhibit one extension $E_{0}$ with the required property. We write $n:=h^{1}(C,F)$ for brevity.

Let $0\rightarrow F\rightarrow \tilde{F}\rightarrow \unicode[STIX]{x1D70F}\rightarrow 0$ be an elementary transformation with $\deg (\unicode[STIX]{x1D70F})=n$ and such that the image of $\unicode[STIX]{x1D6E4}(C,\unicode[STIX]{x1D70F})$ generates $H^{1}(C,F)$ . We may assume that $\unicode[STIX]{x1D70F}$ is supported along $n$ general points $p_{1},\ldots ,p_{n}$ of $C$ which are not base points of $|N|$ . Then $\unicode[STIX]{x1D70F}_{p_{i}}$ is generated by an element

$$\begin{eqnarray}\unicode[STIX]{x1D719}_{i}\in \biggl(\frac{F(p_{i})}{F}\biggr)_{p_{i}}\end{eqnarray}$$

defined up to nonzero scalar multiple. We write $[\unicode[STIX]{x1D719}_{i}]$ for the class in $H^{1}(C,F)$ defined by $\unicode[STIX]{x1D719}_{i}$ .

Now $h^{0}(C,N)\geqslant n$ and the image of $C$ is nondegenerate in $|N|^{\ast }$ . As the $p_{i}$ can be assumed to be general, they impose independent conditions on sections of $N$ . We choose sections $s_{1},\ldots ,s_{n}\in H^{0}(C,N)$ such that $s_{i}(p_{i})\neq 0$ but $s_{i}(p_{j})=0$ for $j\neq i$ . For $1\leqslant i\leqslant n$ , let $\unicode[STIX]{x1D702}_{i}$ be a local section of $N^{-1}$ such that $\unicode[STIX]{x1D702}_{i}(s_{i}(p_{i}))=1$ .

Let $0\rightarrow F\rightarrow E_{0}\rightarrow N\rightarrow 0$ be the extension with class $[E_{0}]$ defined by the image of

$$\begin{eqnarray}(\unicode[STIX]{x1D702}_{1}\otimes \unicode[STIX]{x1D719}_{1},\ldots ,\unicode[STIX]{x1D702}_{n}\otimes \unicode[STIX]{x1D719}_{n})\end{eqnarray}$$

by the coboundary map $\unicode[STIX]{x1D6E4}(C,N^{-1}\otimes \unicode[STIX]{x1D70F})\rightarrow H^{1}(C,N^{-1}\otimes F)$ . Then $s_{i}\cup [E_{0}]=[\unicode[STIX]{x1D719}_{i}]$ for $1\leqslant i\leqslant n$ . Hence the image of $\cdot \cup [E_{0}]$ spans $H^{1}(C,F)$ .◻

We now make further assumptions on $F$ and $N$ . If $r=2$ , then $g\geqslant (2r+2)(2r+1)=30$ . Hence by the Brill–Noether theory of line bundles on $C$ , we may choose a line bundle $F$ of degree $g-2$ with $h^{0}(C,F)=2$ and $|F|$ base point free. If $r\geqslant 3$ : since $g\geqslant 3$ , we have $(r-1)(g-1)-1\geqslant r$ . Therefore, by [BBN15, Theorem 5.1] we may choose a semistable bundle $F$ of rank $r-1$ and degree $(r-1)(g-1)-1$ which is globally generated and satisfies $h^{0}(C,F)=r$ , so $h^{1}(C,F)=r+1$ .

Furthermore, again by Brill–Noether theory, since $g\geqslant (2r+2)(2r+1)$ we may choose $N\in \operatorname{Pic}^{g}(C)$ such that $h^{0}(C,N)=2r+2$ and $|N|$ is base point free. By Lemma 2.7, we may choose an $(r+1)$ -dimensional subspace $\unicode[STIX]{x1D6F1}\subset H^{0}(C,E)$ lifting from $H^{0}(C,N)$ .

Proposition 2.8. Let $F$ , $N$ and $\unicode[STIX]{x1D6F1}$ be as above, and let $0\rightarrow F\rightarrow E\rightarrow N\rightarrow 0$ be a general extension. Then the restricted Petri trace map $\unicode[STIX]{x1D6F1}\otimes H^{0}(C,K_{C}\otimes E^{\ast })\rightarrow H^{0}(C,K_{C})$ is injective.

Proof. Choose a basis $\unicode[STIX]{x1D70E}_{1},\ldots ,\unicode[STIX]{x1D70E}_{r+1}$ for $\unicode[STIX]{x1D6F1}$ . For each $i$ , write $\widetilde{\unicode[STIX]{x1D70E}_{i}}$ for the image of $\unicode[STIX]{x1D70E}_{i}$ in $H^{0}(C,N)$ .

By Lemma 2.7, there is an isomorphism $H^{1}(C,E)\xrightarrow[{}]{{\sim}}H^{1}(C,N)$ . Hence, by Serre duality, the injection $K_{C}\otimes N^{-1}{\hookrightarrow}K_{C}\otimes E^{\ast }$ induces an isomorphism on global sections. Choose a basis $\unicode[STIX]{x1D70F}_{1},\ldots ,\unicode[STIX]{x1D70F}_{2r+1}$ for $H^{0}(C,K_{C}\otimes E^{\ast })$ . For each $j$ , write $\widetilde{\unicode[STIX]{x1D70F}_{j}}$ for the preimage of $\unicode[STIX]{x1D70F}_{j}$ by the aforementioned isomorphism.

For each $i$ and $j$ we have a commutative diagram

where the top row defines the twisted endomorphism

$$\begin{eqnarray}\unicode[STIX]{x1D707}(\unicode[STIX]{x1D70E}_{i}\otimes \unicode[STIX]{x1D70F}_{j})\in H^{0}(C,K_{C}\otimes \operatorname{End}E^{\ast })=H^{0}(C,K_{C}\otimes \operatorname{End}E).\end{eqnarray}$$

Clearly this has rank one. As it factorises via $K_{C}\otimes N^{-1}$ , at a general point of $C$ the eigenspace corresponding to the single nonzero eigenvalue is identified with the fibre of $N^{-1}$ in $E^{\ast }$ . Hence the Petri trace $\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D70E}_{i}\otimes \unicode[STIX]{x1D70F}_{j})$ may be identified with the restriction to $N^{-1}$ . By the diagram, we may identify this restriction with

$$\begin{eqnarray}\unicode[STIX]{x1D707}_{N}(\widetilde{\unicode[STIX]{x1D70E}_{i}}\otimes \widetilde{\unicode[STIX]{x1D70F}_{j}})\in H^{0}(C,K_{C}),\end{eqnarray}$$

where $\unicode[STIX]{x1D707}_{N}$ is the Petri map of the line bundle $N$ . Since $C$ is Petri, $\unicode[STIX]{x1D707}_{N}$ is injective. Hence the elements $\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D70E}_{i}\otimes \unicode[STIX]{x1D70F}_{j})=\unicode[STIX]{x1D707}_{N}(\widetilde{\unicode[STIX]{x1D70E}_{i}}\otimes \widetilde{\unicode[STIX]{x1D70F}_{j}})$ are independent in $H^{0}(C,K_{C})$ . This proves the statement.◻

Before proceeding, we need to recall some background on coherent systems (see [BBN08, §2] for an overview and [BGMN03] for more detail). We recall that a coherent system of type $(r,d,k)$ is a pair $(W,\unicode[STIX]{x1D6F1})$ where $W$ is a vector bundle of rank $r$ and degree $d$ over $C$ , and $\unicode[STIX]{x1D6F1}\subseteq H^{0}(C,W)$ is a subspace of dimension $k$ . There is a stability condition for coherent systems depending on a real parameter $\unicode[STIX]{x1D6FC}$ , and a moduli space $G(\unicode[STIX]{x1D6FC};r,d,k)$ for equivalence classes of $\unicode[STIX]{x1D6FC}$ -semistable coherent systems of type $(r,d,k)$ . If $k\geqslant r$ , then by [BGMN03, Proposition 4.6] there exists $\unicode[STIX]{x1D6FC}_{L}\in \mathbb{R}$ such that $G(\unicode[STIX]{x1D6FC};r,d,k)$ is independent of $\unicode[STIX]{x1D6FC}$ for $\unicode[STIX]{x1D6FC}>\unicode[STIX]{x1D6FC}_{L}$ . This ‘terminal’ moduli space is denoted $G_{L}$ . Moreover, the locus

$$\begin{eqnarray}U(r,d,k):=\{(W,\unicode[STIX]{x1D6F1})\in G_{L}:W\text{ is a stable vector bundle}\}\end{eqnarray}$$

is an open subset of $G_{L}$ . For us, $d=r(g-1)$ and $k=r+1$ . To ease notation, we write $U:=U(r,r(g-1),r+1)$ .

Now let $N_{1}$ be a line bundle of degree $g$ with $h^{0}(C,N_{1})\geqslant r+2$ . Let $F$ be as above, and let $0\rightarrow F\rightarrow E\rightarrow N_{1}\rightarrow 0$ be a general extension.

Lemma 2.9. For a general subspace $\unicode[STIX]{x1D6F1}\subset H^{0}(C,E)$ of dimension $r+1$ , the coherent system $(E,\unicode[STIX]{x1D6F1})$ defines an element of $U$ .

Proof. Recall the bundle $E_{0}$ defined in Lemma 2.7, which clearly is generically generated. Let us describe the subsheaf $E_{0}^{\prime }$ generated by $H^{0}(C,E_{0})$ .

Write $p_{1}+\cdots +p_{r+1}=:D$ . Clearly $s\cup [E_{0}]=0$ for any $s\in H^{0}(C,N_{1}(-D))$ . Since the $p_{i}$ are general points,

$$\begin{eqnarray}h^{0}(C,N_{1}(-D))=h^{0}(C,N_{1})-(r+1)=\dim (\operatorname{Ker}(\cdot \cup [E_{0}]:H^{0}(C,N_{1})\rightarrow H^{1}(C,F))).\end{eqnarray}$$

Therefore, the image of $H^{0}(C,E_{0})$ in $H^{0}(C,N_{1})$ is exactly $H^{0}(C,N_{1}(-D))$ . As the subbundle $F\subset E_{0}$ is globally generated, $E_{0}^{\prime }$ is an extension $0\rightarrow F\rightarrow E_{0}^{\prime }\rightarrow N_{1}(-D)\rightarrow 0$ . Dualising and taking global sections, we obtain

$$\begin{eqnarray}0\rightarrow H^{0}(C,N_{1}^{-1}(D))\rightarrow H^{0}(C,(E_{0}^{\prime })^{\ast })\rightarrow H^{0}(C,F^{\ast })\rightarrow \cdots\end{eqnarray}$$

Since both $N_{1}^{-1}(D)$ and $F^{\ast }$ are semistable of negative degree, $h^{0}(C,N_{1}^{-1}(-D))=h^{0}(C,F^{\ast })=0$ , so $h^{0}(C,(E_{0}^{\prime })^{\ast })=0$ .

Now let $\unicode[STIX]{x1D6F1}_{1}\subset H^{0}(C,E_{0})$ be any subspace of dimension $r+1$ generically generating $E_{0}$ . Since $h^{0}(C,(E_{0}^{\prime })^{\ast })=0$ , by [BBN08, Theorem 3.1(3)] the coherent system $(E_{0},\unicode[STIX]{x1D6F1}_{1})$ defines a point of $G_{L}$ . Since generic generatedness and vanishing of $h^{0}(C,(E^{\prime })^{\ast })$ are open conditions on families of bundles with a fixed number of sections, the same is true for a generic $(E,\unicode[STIX]{x1D6F1})$ where $E$ is an extension $0\rightarrow F\rightarrow E\rightarrow N_{1}\rightarrow 0$ . By Lemma 2.6, in fact $(E,\unicode[STIX]{x1D6F1})$ belongs to $U$ .◻

Lemma 2.10. For generic $E$ represented in $U$ , we have $h^{0}(C,E)=h^{0}(C,K_{C}\otimes E^{\ast })=r+1$ .

Proof. Since $C$ is Petri general, $B_{1,g}^{r+2}$ is irreducible in $\operatorname{Pic}^{g}(C)$ . Thus there exists an irreducible family parametrising extensions of the form $0\rightarrow F\rightarrow E\rightarrow N_{1}\rightarrow 0$ where $F$ is as above and $N_{1}$ ranges over $B_{1,g}^{r+2}$ . This contains the extension $E_{0}$ constructed above. By Lemma 2.7, a general element $E_{1}$ of the family satisfies $h^{0}(C,E_{1})=r+1$ . By semicontinuity, the same is true for general $E$ represented in $U$ .◻

Now by [BBN08, Theorem 3.1(4) and Remark 6.2], the locus $U$ is irreducible. Write $B$ for the component of $B_{r,r(g-1)}^{r+1}$ containing the image of $U$ , and $B^{\prime }$ for the sublocus $\{E\in B:h^{0}(C,E)=r+1\}$ . Set $U^{\prime }:=U\times _{B}B^{\prime }$ ; clearly $U^{\prime }\cong B^{\prime }$ .

Let $\tilde{B}\rightarrow B$ be an étale cover such that there is a Poincaré bundle ${\mathcal{E}}\rightarrow \tilde{B}\times C$ . In a natural way we obtain a commutative cube

where all faces are fibre product diagrams. By a standard construction, we can find a complex of bundles $\unicode[STIX]{x1D6FC}:K^{0}\rightarrow K^{1}$ over $\tilde{B}$ such that $\operatorname{Ker}(\unicode[STIX]{x1D6FC}_{b})\cong H^{0}(C,K_{C}\otimes {\mathcal{E}}_{b}^{\ast })$ for each $b\in \tilde{B}$ . Following [ACGH85, ch. IV], we consider the Grassmann bundle $\operatorname{Gr}(r+1,K^{0})$ over $\tilde{B}$ and the sublocus

$$\begin{eqnarray}{\mathcal{G}}:=\{\unicode[STIX]{x1D6EC}\in \operatorname{Gr}(r+1,K^{0}):\unicode[STIX]{x1D6FC}|_{\unicode[STIX]{x1D6EC}}=0\}.\end{eqnarray}$$

Write ${\mathcal{G}}_{1}:={\mathcal{G}}\times _{\tilde{B}}\tilde{U}$ . The fibre of ${\mathcal{G}}_{1}$ over $({\mathcal{E}}_{b},\unicode[STIX]{x1D6F1})\in \tilde{U}$ is then $\operatorname{Gr}(r+1,H^{0}(C,K_{C}\otimes {\mathcal{E}}_{b}^{\ast }))$ .

Now let $E_{0}$ be a bundle as constructed in Lemma 2.7 with $h^{0}(C,E_{0})=2r+2$ , and let $\unicode[STIX]{x1D6F1}_{0}$ be a generic choice of $(r+1)$ -dimensional subspace of $H^{0}(C,E_{0})$ . We may assume $\tilde{U}$ is irreducible since $U$ is. Since $\tilde{U} \rightarrow U$ is étale, by Lemma 2.8 in fact $U$ is also smooth at $(E_{0},\unicode[STIX]{x1D6F1}_{0})$ (cf. [BGMN03, Proposition 3.10]). Therefore, we may choose a one-parameter family $\{(E_{t},\unicode[STIX]{x1D6F1}_{t}):t\in T\}$ in $\tilde{U}$ such that $(E_{t_{0}},\unicode[STIX]{x1D6F1}_{t_{0}})=(E_{0},\unicode[STIX]{x1D6F1}_{0})$ while $(E_{t},\unicode[STIX]{x1D6F1}_{t})$ belongs to $\tilde{U} ^{\prime }$ for generic $t\in T$ . Since the bundles have Euler characteristic zero, for generic $t\in T$ there is exactly one choice of $\unicode[STIX]{x1D6EC}\in {\mathcal{G}}_{1}|_{(E_{t},\unicode[STIX]{x1D6F1}_{t})}$ . Thus we obtain a section $T\backslash \{0\}\rightarrow {\mathcal{G}}_{1}$ . As $\dim T=1$ , this section can be extended uniquely to $0$ . We thus obtain a triple $(E_{0},\unicode[STIX]{x1D6F1}_{0},\unicode[STIX]{x1D6EC}_{0})$ where $\unicode[STIX]{x1D6EC}\subset H^{0}(C,K_{C}\otimes E_{0}^{\ast })$ has dimension $r+1$ . By Lemma 2.8, this triple is Petri trace injective. Hence

$$\begin{eqnarray}(E_{t},\unicode[STIX]{x1D6F1}_{t},\unicode[STIX]{x1D6EC}_{t})=(E,H^{0}(C,E),H^{0}(C,K_{C}\otimes E^{\ast }))\end{eqnarray}$$

is Petri trace injective for generic $t\in T$ . Thus a general bundle $E$ represented in $\tilde{U} ^{\prime }$ is Petri trace injective.

Furthermore, by [BBN08, Theorem 3.1(4)], a general $(E,\unicode[STIX]{x1D6F1})\in U$ is globally generated (not just generically). Thus we obtain the following proposition.

Proposition 2.11. A general element $E$ of the irreducible component $B\subseteq B_{r,r(g-1)}^{r+1}$ is Petri trace injective and globally generated with $h^{0}(C,E)=r+1$ .

We can now prove the theorem.

Proof of Theorem 2.5.

Consider the map $a:\mathit{SU}_{C}(r)\times \operatorname{Pic}^{g-1}(C)\rightarrow \mathit{U}_{C}(r,r(g-1))$ given by $(V,M)\mapsto V\otimes M$ . This is an étale cover of degree $r^{2g}$ . We write $\overline{B}$ for the inverse image $a^{-1}(B)$ . Since $a$ is étale, we have $T_{(V,M)}\overline{B}\cong T_{V\otimes M}B$ for each $(V,M)\in B$ . In particular,

(6) $$\begin{eqnarray}\dim \overline{B}=\dim B=\dim \mathit{U}_{C}(r,r(g-1))-(r+1)^{2}.\end{eqnarray}$$

We write $p$ for the projection $\mathit{SU}_{C}(r)\times \operatorname{Pic}^{g-1}(C)\rightarrow \mathit{SU}_{C}(r)$ , and $p_{1}$ for the restriction $p|_{\overline{B}}:\overline{B}\rightarrow \mathit{SU}_{C}(r)$ .

Claim. $p_{1}$ is dominant.

To see this: for $(V,M)\in \overline{B}$ , the locus $p_{1}^{-1}(V)$ is identified with an open subset of the twisted Brill–Noether locus

$$\begin{eqnarray}B_{1,g-1}^{r+1}(V)=\{M\in \operatorname{Pic}^{g-1}(C):h^{0}(C,V\otimes M)\geqslant r+1\}\subseteq \operatorname{Pic}^{g-1}(C).\end{eqnarray}$$

Moreover, for each such $(V,M)$ , we have

$$\begin{eqnarray}\dim _{M}(p_{1}^{-1}(V))=\dim (T_{M}(B_{1,g-1}^{r+1}(V)))=\dim \operatorname{Im}(\bar{\unicode[STIX]{x1D707}})^{\bot }.\end{eqnarray}$$

Since $V\otimes M$ is Petri trace injective, this dimension is $g-(r+1)^{2}$ . By semicontinuity, a general fibre of $p_{1}$ has dimension at most $g-(r+1)^{2}$ . Therefore, in view of (6), the image of $p_{1}$ has dimension at least

$$\begin{eqnarray}(\dim \mathit{U}_{C}(r,r(g-1))-(r+1)^{2})-(g-(r+1)^{2})=\dim \mathit{U}_{C}(r,r(g-1))-g=\dim \mathit{SU}_{C}(r).\end{eqnarray}$$

As $\mathit{SU}_{C}(r)$ is irreducible, the claim follows.

We can now conclude the proof. Let $V\in \mathit{SU}_{C}(r)$ be general. By the claim, we can find $(V,M)\in \tilde{P}$ such that $h^{0}(C,V\otimes M)=r+1$ and $V\otimes M$ is globally generated and Petri trace injective. By Proposition 2.4, the theta divisor $\unicode[STIX]{x1D6E9}_{V}$ exists and satisfies $\operatorname{mult}_{M}\unicode[STIX]{x1D6E9}_{V}=h^{0}(C,V\otimes M)=r+1$ . Finally, by considering a suitable sum of line bundles, we see that the involution $E\mapsto K_{C}\otimes E^{\ast }$ preserves the component $\overline{B}$ . Since a general element of $\overline{B}$ is globally generated, in general both $V\otimes M$ and $K_{C}\otimes M^{-1}\otimes V^{\ast }$ are globally generated. ◻

3 Reconstruction of bundles from tangent cones to theta divisors

3.1 Tangent cones

Let $Y$ be a normal variety and $Z\subset Y$ a divisor. Let $p$ be a smooth point of $Y$ which is a point of multiplicity $n\geqslant 1$ of $Z$ . A local equation $f$ for $Z$ near $p$ has the form $f_{n}+f_{n+1}+\cdots \,$ , where the $f_{i}$ are homogeneous polynomials of degree $i$ in local coordinates centred at $p$ . The projectivised tangent cone ${\mathcal{T}}_{p}(Z)$ to $Z$ at $p$ is the hypersurface in $\mathbb{P}T_{p}Y$ defined by the first nonzero component $f_{n}$ of $f$ . (For a more intrinsic description, see [ACGH85, ch. II.1].)

Now let $C$ be a curve of genus $g\geqslant (r+1)^{2}$ . Let $E$ be a Petri trace injective bundle of rank $r$ and degree $r(g-1)$ , with $h^{0}(C,E)=r+1$ . By Proposition 2.4 (with $h=g-1$ ), the theta divisor $\unicode[STIX]{x1D6E9}_{E}$ is defined and contains the origin ${\mathcal{O}}_{C}$ of $\text{Pic}^{0}(C)$ with multiplicity $h^{0}(C,E)=r+1$ .

By [CT11, Theorem 3.4 and Remark 3.8] (see also Kempf [Kem73]), the tangent cone ${\mathcal{T}}_{{\mathcal{O}}_{C}}(\unicode[STIX]{x1D6E9}_{E})$ to $\unicode[STIX]{x1D6E9}_{E}$ at ${\mathcal{O}}_{C}$ is given by the determinant of an $(r+1)\times (r+1)$ matrix $\unicode[STIX]{x1D6EC}=(l_{ij})$ of linear forms $l_{ij}$ on $H^{1}(C,{\mathcal{O}}_{C})$ , which is related to the multiplication map $\bar{\unicode[STIX]{x1D707}}$ as follows: in appropriate bases $(s_{i})$ and $(t_{j})$ of $H^{0}(C,E)$ and $H^{0}(C,K_{C}\otimes E^{\ast })$ respectively, $\unicode[STIX]{x1D6EC}$ is given by

$$\begin{eqnarray}(l_{ij})=(\bar{\unicode[STIX]{x1D707}}(s_{i}\otimes t_{j})).\end{eqnarray}$$

Hence, via Serre duality, $\unicode[STIX]{x1D6EC}$ coincides with the cup product map

$$\begin{eqnarray}\bigcup :H^{0}(C,E)\otimes H^{1}(C,{\mathcal{O}}_{C})\rightarrow H^{1}(C,E).\end{eqnarray}$$

Thus the matrix $\unicode[STIX]{x1D6EC}=(l_{ij})$ is a matrix of linear forms on the canonical space $|K_{C}|^{\ast }$ .

In the following two subsections, we will show on the one hand that one can recover the bundle $E$ from the determinantal representation of the tangent cone ${\mathcal{T}}_{{\mathcal{O}}_{C}}(\unicode[STIX]{x1D6E9}_{E})$ given by the matrix $\unicode[STIX]{x1D6EC}$ . On the other hand, up to changing bases in $H^{0}(C,E)$ and $H^{1}(C,E)$ there are only two determinantal representations of the tangent cone, namely $\unicode[STIX]{x1D6EC}$ and $\unicode[STIX]{x1D6EC}^{t}$ . Thus the tangent cone determines $E$ up to an involution.

We will denote by $\mathbb{P}=|K_{C}|^{\ast }$ the canonical space and by $\unicode[STIX]{x1D711}$ the canonical embedding $C{\hookrightarrow}\mathbb{P}$ .

3.2 Reconstruction of the bundle from the tangent cone

As above, let $\unicode[STIX]{x1D6EC}=(l_{ij})$ be the determinantal representation of the tangent cone given by the cup product mapping. We identify the source of $\unicode[STIX]{x1D6EC}$ with $H^{0}(C,E)$ and the target with $H^{1}(C,E)$ :

$$\begin{eqnarray}H^{0}(C,E)\otimes {\mathcal{O}}_{\mathbb{ P}}(-1)\stackrel{\unicode[STIX]{x1D6EC}}{\longrightarrow }H^{1}(C,E)\otimes {\mathcal{O}}_{\mathbb{ P}}.\end{eqnarray}$$

We recall that the Serre duality isomorphism sends $b\in H^{1}(C,E)$ to the linear form

$$\begin{eqnarray}\cdot \,\cup \,b:H^{0}(C,K_{C}\otimes E^{\ast })\rightarrow H^{1}(C,K_{C})=\mathbb{C}.\end{eqnarray}$$

In the following proofs, we will use principal parts in order to represent cohomology classes of certain bundles. We refer to [Kem83] or [Pau03, §3.2] for the necessary background. See also Kempf and Schreyer [KS88].

Lemma 3.1. Suppose that $h^{0}(C,E)=r+1$ and $E$ and $K_{C}\otimes E^{\ast }$ are globally generated. Then the rank of $\unicode[STIX]{x1D6EC}|_{C}=\unicode[STIX]{x1D711}^{\ast }\unicode[STIX]{x1D6EC}$ is equal to $r=\operatorname{rk}\,E$ at all points of $C$ . In particular, the canonical curve is contained in ${\mathcal{T}}_{{\mathcal{O}}_{C}}(\unicode[STIX]{x1D6E9}_{E})$ .

Proof. For each $p\in C$ , write $\unicode[STIX]{x1D6FD}_{p}$ for a principal part with a simple pole supported at $p$ . Then (see [KS88]) the cohomology class $[\unicode[STIX]{x1D6FD}_{p}]$ is identified with the image of $p$ by $\unicode[STIX]{x1D711}$ . Therefore, at $p\in C$ , the pullback $\unicode[STIX]{x1D6EC}|_{C}$ is identified with the cup product map

$$\begin{eqnarray}[\unicode[STIX]{x1D6FD}_{p}]\otimes s\mapsto [\unicode[STIX]{x1D6FD}_{p}]\cup s.\end{eqnarray}$$

The kernel of $[\unicode[STIX]{x1D6FD}_{p}]\cup \cdot$ contains the subspace $H^{0}(C,E(-p))$ , which is one-dimensional since $E$ is globally generated and $h^{0}(C,E)=r+1$ . If $\operatorname{Ker}([\unicode[STIX]{x1D6FD}_{p}]\cup \cdot )$ has dimension greater than 1, then there is a section $s^{\prime }\in H^{0}(C,E)$ not vanishing at $p$ such that

$$\begin{eqnarray}[\unicode[STIX]{x1D6FD}_{p}\cdot s^{\prime }]=[\unicode[STIX]{x1D6FD}_{p}]\cup s^{\prime }=0\in H^{1}(C,E).\end{eqnarray}$$

By Serre duality, this means that

$$\begin{eqnarray}[\unicode[STIX]{x1D6FD}_{p}\cdot \langle s^{\prime }(p),t(p)\rangle ]\end{eqnarray}$$

is zero in $H^{1}(C,K_{C})$ for all $t\in H^{0}(C,K_{C}\otimes E^{\ast })$ . Hence the values at $p$ of all global sections of $K_{C}\otimes E^{\ast }$ belong to the hyperplane in $(K_{C}\otimes E^{\ast })|_{p}$ defined by contraction with the nonzero vector $s^{\prime }(p)\in E|_{p}$ . Thus $K_{C}\otimes E^{\ast }$ is not globally generated, contrary to our hypothesis.◻

Remark 3.2. Casalaina-Martin and Teixidor i Bigas in [CT11, §6] prove more generally that if $E$ is a general vector bundle with $h^{0}(C,E)>kr$ , then the $k$ th secant variety of the canonical image $\unicode[STIX]{x1D711}(C)$ of $C$ is contained in ${\mathcal{T}}_{{\mathcal{O}}_{C}}(\unicode[STIX]{x1D6E9}_{E})$ .

Proposition 3.3. Let $E$ be a vector bundle with $h^{0}(C,E)=r+1$ , such that both $E$ and $K_{C}\otimes E^{\ast }$ are globally generated. Then the image of $\unicode[STIX]{x1D6EC}|_{C}$ is isomorphic to $T_{C}\otimes E$ .

Proof. As $\unicode[STIX]{x1D711}^{\ast }{\mathcal{O}}_{\mathbb{P}^{g-1}}(-1)\cong T_{C}$ , the pullback $\unicode[STIX]{x1D711}^{\ast }\unicode[STIX]{x1D6EC}=\unicode[STIX]{x1D6EC}|_{C}$ is a map

$$\begin{eqnarray}\unicode[STIX]{x1D6EC}|_{C}:T_{C}\otimes H^{0}(C,E)\rightarrow {\mathcal{O}}_{C}\otimes H^{1}(C,E).\end{eqnarray}$$

Write $L:=\det (E)$ , a line bundle of degree $r(g-1)$ . Then $\det (K_{C}\otimes E^{\ast })=K_{C}^{r}\otimes L^{-1}$ . As $K_{C}\otimes E^{\ast }$ is globally generated, the evaluation sequence

$$\begin{eqnarray}0\rightarrow K_{C}^{-r}\otimes L\rightarrow {\mathcal{O}}_{C}\otimes H^{0}(C,K_{C}\otimes E^{\ast })\rightarrow K_{C}\otimes E^{\ast }\rightarrow 0\end{eqnarray}$$

is exact. For each $p\in C$ , the image of $(K_{C}^{-r}\otimes L)|_{p}$ in $H^{0}(C,K_{C}\otimes E^{\ast })$ is exactly $\mathbb{C}\cdot t_{p}$ , where $t_{p}$ is the unique section, up to scalar, of $K_{C}\otimes E^{\ast }$ vanishing at $p$ .

Dualising, we obtain a diagram

Here $e_{p}$ can be identified up to scalar with the map $f\mapsto f(t_{p})$ where $t_{p}$ is as above.

Now for each $p\in C$ , the image

$$\begin{eqnarray}[\unicode[STIX]{x1D6FD}_{p}]\cup H^{0}(C,E)\subset H^{1}(C,E)\cong H^{0}(C,K_{C}\otimes E^{\ast })^{\ast }\end{eqnarray}$$

annihilates $t_{p}\in H^{0}(C,K_{C}\otimes E^{\ast })$ , since the principal part $\unicode[STIX]{x1D6FD}_{p}\cdot t_{p}$ is everywhere regular. Therefore, $\unicode[STIX]{x1D6EC}|_{C}$ factorises via $\operatorname{Ker}(e)=T_{C}\otimes E$ . Since $\operatorname{rk}(\unicode[STIX]{x1D6EC}|_{C})\equiv r$ by Lemma 3.1, we have $\operatorname{Im}(\unicode[STIX]{x1D6EC}|_{C})\cong T_{C}\otimes E$ .◻

Remark 3.4. A straightforward computation shows also that

$$\begin{eqnarray}\operatorname{Ker}(\unicode[STIX]{x1D6EC}|_{C})\cong T_{C}\otimes L^{-1}\quad \text{and}\quad \operatorname{Coker}(\unicode[STIX]{x1D6EC}|_{C})\cong K_{C}^{r}\otimes L^{-1}.\end{eqnarray}$$

We will also want to study the transpose $\unicode[STIX]{x1D6EC}^{t}$ , which we will consider as a map

$$\begin{eqnarray}\unicode[STIX]{x1D6EC}^{t}:{\mathcal{O}}_{\mathbb{ P}}(-1)\otimes H^{0}(C,K_{C}\otimes E^{\ast })\rightarrow {\mathcal{O}}_{\mathbb{ P}}\otimes H^{1}(C,K_{C}\otimes E^{\ast }).\end{eqnarray}$$

The proof of Proposition 3.3 also shows the following corollary.

Corollary 3.5. Let $E$ and $\unicode[STIX]{x1D6EC}$ be as above. Then the image of $\unicode[STIX]{x1D6EC}^{t}|_{C}$ is isomorphic to $E^{\ast }$ .

Remark 3.6. In order to describe the cokernel of $\unicode[STIX]{x1D6EC}|_{C}$ , it is also enough to know at which points of $C$ a row of $\unicode[STIX]{x1D6EC}|_{C}$ vanishes. Dualising the sequence

$$\begin{eqnarray}0\rightarrow K_{C}^{r}\otimes L^{-1}\rightarrow {\mathcal{O}}_{C}\otimes H^{0}(C,K_{C}\otimes E^{\ast })\rightarrow K_{C}\otimes E^{\ast }\rightarrow 0,\end{eqnarray}$$

we see that $H^{0}(C,K_{C}\otimes E^{\ast })^{\ast }$ is canonically identified with a subspace of $H^{0}(C,K_{C}^{r}\otimes L^{-1})$ . Using the description of

$$\begin{eqnarray}T_{C}\otimes H^{0}(C,E)\stackrel{\unicode[STIX]{x1D6EC}|_{C}}{\longrightarrow }{\mathcal{O}}_{C}\otimes H^{1}(C,E)\stackrel{{\sim}}{\longrightarrow }{\mathcal{O}}_{C}\otimes H^{0}(C,K_{C}\otimes E^{\ast })^{\ast }\end{eqnarray}$$

as in the above proof, we see that a row vanishes exactly in a divisor associated to $K_{C}^{r}\otimes L^{-1}$ . Hence, the cokernel is isomorphic to $K_{C}^{r}\otimes L^{-1}$ .

3.3 Uniqueness of the linear determinantal representation of the tangent cone

In order to show the desired uniqueness of the determinantal representation of the tangent cone, we use a classical result of Frobenius. See [Fro97] and also for a modern proof [Die49, Wat87] and the references therein. For the sake of completeness we will give a sketch of a proof following Frobenius.

Proposition 3.7. Suppose $r\geqslant 1$ . Let $A$ and $B$ be $(r+1)\times (r+1)$ matrices of independent linear forms, such that the entries of $A$ are linear combinations of the entries of $B$ and $\det (A)=c\cdot \det (B)$ for a nonzero constant $k\in \mathbb{C}$ . Then, there exist invertible matrices $S,T\in \operatorname{Gl}(r+1,\mathbb{C})$ , unique up to scalar, such that $A=S\cdot B\cdot T$ or $A=S\cdot B^{t}\cdot T$ .

Proof by Frobenius [Fro97, pp. 1011–1013].

Note that for $r\geqslant 1$ only one of the above cases can occur and the matrices $S$ and $T$ are unique up to scalar. Indeed, let $A=SBT=S^{\prime }BT^{\prime }$ and set $b_{ii}=1$ and $b_{ij}=0$ if $i\neq j$ ; then $ST=S^{\prime }T^{\prime }$ . Set $U=T({T^{\prime }}^{-1})=S({S^{\prime }}^{-1})$ ; thus $UB=BU$ . Since $U$ commutes with every matrix, we have $U=k\cdot E_{r}$ and hence $S^{\prime }=k\cdot S$ and $T^{\prime }=1/k\cdot T$ . Similarly, one can show that $B^{t}$ is not equivalent to $B$ . Note also that there is no relation between any minors of $A$ or $B$ .

For $l=0,\ldots ,r$ , let $c_{ij}^{l}$ be the coefficient of $b_{ll}$ in $a_{ij}$ and let $y$ be a new variable. We substitute $b_{ll}$ with $b_{ll}+y$ in $A$ and $B$ and get new matrices, denoted by $(a_{ij}+y\cdot c_{ij}^{l})$ and $B^{l}$ , respectively. Since $\det B^{l}$ is linear in $y$ , the coefficient of $y^{2}$ in $\det (a_{ij}+y\cdot c_{ij}^{l})=\det B^{l}$ has to vanish. But the coefficient is the sum of products of $2\times 2$ minors of $(c_{ij}^{l})$ and $(r-1)\times (r-1)$ minors of $A$ . Since there are no relations between any minors of $A$ , all $2\times 2$ minors of $(c_{ij}^{l})$ vanish. Hence, $(c_{ij}^{l})$ has rank one for any $l$ and we can write $c_{ij}^{l}=p_{i}^{l}q_{j}^{l}$ where $p^{l}$ and $q^{l}$ are column and row vectors, respectively.

Let $B_{0}=B|_{\{b_{ij}=0,i\neq j\}}$ and $A_{0}=A|_{\{b_{ij}=0,i\neq j\}}$ . Then

$$\begin{eqnarray}A_{0}=PB_{0}Q,\end{eqnarray}$$

where $P=(p_{i}^{l})_{0\leqslant i,l\leqslant r}$ and $Q=(q_{j}^{l})_{0\leqslant l,j\leqslant r}$ . Since $\det (A_{0})=c\cdot \det (B_{0})=c\cdot b_{00}\cdot \ldots \cdot b_{rr}$ , we get $\det (P)\cdot \det (Q)=c$ , hence $P$ and $Q$ are invertible.

Let $\widetilde{B}=P^{-1}AQ^{-1}$ . By definition $\widetilde{B}|_{\{b_{ij}=0,i\neq j\}}=B_{0}$ . Thus, the entries $\widetilde{b_{ij}}$ for $i\neq j$ and $v_{i}=\widetilde{b_{ii}}-b_{ii}$ are linear functions in $b_{ij}$ for $i\neq j$ . Furthermore, we have

$$\begin{eqnarray}\det (\widetilde{B})=\det (P^{-1}AQ^{-1})=\det (P^{-1}Q^{-1})\cdot \det (A)=\frac{1}{c}\cdot c\cdot \det (B)=\det (B).\end{eqnarray}$$

Comparing the coefficients of $b_{11}b_{22}\cdots b_{rr}$ in $\det (\widetilde{B})$ and $\det (B)$ , we get $v_{0}=0$ . Similarly, $v_{i}=0$ for $0\leqslant i\leqslant r$ . Comparing the coefficients of $b_{22}\cdots b_{rr}$ , we get $b_{12}b_{21}=\widetilde{b_{12}}\widetilde{b_{21}}$ and in general

$$\begin{eqnarray}b_{ij}b_{ji}=\widetilde{b_{ij}}\widetilde{b_{ji}},\quad i\neq j.\end{eqnarray}$$

Comparing the coefficients of $b_{33}\cdots b_{rr}$ , we get $b_{12}b_{23}b_{31}+b_{21}b_{13}b_{32}=\widetilde{b_{12}}\widetilde{b_{23}}\widetilde{b_{31}}+\widetilde{b_{21}}\widetilde{b_{13}}\widetilde{b_{32}}$ and in general

$$\begin{eqnarray}b_{ij}b_{jk}b_{ki}+b_{ji}b_{ik}b_{kj}=\widetilde{b_{ij}}\widetilde{b_{jk}}\widetilde{b_{ki}}+\widetilde{b_{ji}}\widetilde{b_{ik}}\widetilde{b_{kj}},\quad i\neq j\neq k\neq i.\end{eqnarray}$$

A careful study of these equations shows that either

$$\begin{eqnarray}\widetilde{b_{ij}}=\frac{k_{i}}{k_{j}}b_{ij}\quad \text{and}\quad \widetilde{B}=KBK^{-1}\quad \text{or}\quad \widetilde{b_{ij}}=\frac{k_{i}}{k_{j}}b_{ji}\quad \text{and}\quad \widetilde{B}=KB^{t}K^{-1},\end{eqnarray}$$

where $K=(k_{i}\unicode[STIX]{x1D6FF}_{ij})_{0\leqslant i,j\leqslant r}$ . The claim follows.◻

We now assume that $E$ is a Petri trace injective bundle. Let $\unicode[STIX]{x1D6EC}=(l_{ij})$ be a determinantal representation of the tangent cone ${\mathcal{T}}_{{\mathcal{O}}_{C}}(\unicode[STIX]{x1D6E9}_{E})$ as above. By Petri trace injectivity, the matrix $\unicode[STIX]{x1D6EC}$ is $(r+1)$ -generic (see [Eis88] for a definition), that is, there are no relations between the entries $l_{ij}$ or any subminors of $\unicode[STIX]{x1D6EC}$ .

Corollary 3.8. For a curve of genus $g\geqslant (r+1)^{2}$ and a Petri trace injective bundle $E$ with $r+1$ global sections of degree $r(g-1)$ , any determinantal representation of the tangent cone ${\mathcal{T}}_{{\mathcal{O}}_{C}}(\unicode[STIX]{x1D6E9}_{E})\subset |K_{C}|^{\ast }$ is equivalent to $\unicode[STIX]{x1D6EC}$ or $\unicode[STIX]{x1D6EC}^{t}$ .

Proof. Let $\unicode[STIX]{x1D6FC}$ be any determinantal representation of the tangent cone ${\mathcal{T}}_{{\mathcal{O}}_{C}}(\unicode[STIX]{x1D6E9}_{E})$ in $|K_{C}|^{\ast }$ . Then, $\unicode[STIX]{x1D6FC}$ is an $(r+1)\times (r+1)$ matrix of linear entries, since the degree of the tangent cone is $r+1$ . Furthermore, the entries $\unicode[STIX]{x1D6FC}_{ij}$ of $\unicode[STIX]{x1D6FC}$ are linear combinations of the entries $l_{ij}$ of $\unicode[STIX]{x1D6EC}$ . Indeed, assume for some $k,l$ that $\unicode[STIX]{x1D6FC}_{kl}$ is not a linear combination of the $l_{ij}$ . Then ${\mathcal{T}}_{{\mathcal{O}}_{C}}(\unicode[STIX]{x1D6E9}_{E})$ would be the cone over $V(\unicode[STIX]{x1D6FC}_{kl})\cap {\mathcal{T}}_{{\mathcal{O}}_{C}}(\unicode[STIX]{x1D6E9}_{E})$ . Hence, the vertex of ${\mathcal{T}}_{{\mathcal{O}}_{C}}(\unicode[STIX]{x1D6E9}_{E})$ defined by the entries $l_{ij}$ would have codimension strictly less than $(r+1)^{2}$ , a contradiction to the independence of the $l_{ij}$ . The corollary follows from Proposition 3.7.◻

4 Injectivity of the theta map

Theorem 4.1. Suppose $r\geqslant 2$ . Let $C$ be a Petri general curve of genus $g\geqslant (2r+2)(2r+1)$ . Then the theta map ${\mathcal{D}}:\mathit{SU}_{C}(r){\dashrightarrow}|r\unicode[STIX]{x1D6E9}|$ is generically injective.

Proof. Let $V\in \mathit{SU}_{C}(r)$ be a general stable bundle. By Theorem 2.5, there exists $M\in \unicode[STIX]{x1D6E9}_{V}$ such that $h^{0}(C,V\otimes M)=r+1$ , the bundle $V\otimes M=:E$ is Petri trace injective, and $E$ and $K_{C}\otimes E^{\ast }$ are globally generated.

Note that tensoring by $M^{-1}$ defines an isomorphism $\unicode[STIX]{x1D6E9}_{V}\xrightarrow[{}]{{\sim}}\unicode[STIX]{x1D6E9}_{E}$ inducing an isomorphism ${\mathcal{T}}_{M}(\unicode[STIX]{x1D6E9}_{V})\xrightarrow[{}]{{\sim}}{\mathcal{T}}_{{\mathcal{O}}_{C}}(\unicode[STIX]{x1D6E9}_{E})$ . In order to use the results of the previous sections, we will work with $\unicode[STIX]{x1D6E9}_{E}$ .

Now let

$$\begin{eqnarray}\unicode[STIX]{x1D6FC}:{\mathcal{O}}_{\mathbb{P}^{g-1}}(-1)\otimes \mathbb{C}^{r+1}\rightarrow {\mathcal{O}}_{\mathbb{ P}^{g-1}}\otimes \mathbb{C}^{r+1}\end{eqnarray}$$

be a map of bundles of rank $r+1$ over $\mathbb{P}^{g-1}$ whose determinant defines the tangent cone ${\mathcal{T}}_{{\mathcal{O}}_{C}}(\unicode[STIX]{x1D6E9}_{E})$ . By Corollary 3.8, the map $\unicode[STIX]{x1D6FC}$ is equivalent either to $\unicode[STIX]{x1D6EC}$ or $\unicode[STIX]{x1D6EC}^{t}$ , where $\unicode[STIX]{x1D6EC}$ is the representation given by the cup product mapping as defined in § 3. Therefore, by Proposition 3.3 and Corollary 3.5, the image $E^{\prime }$ of $\unicode[STIX]{x1D6FC}|_{C}$ is isomorphic either to $T_{C}\otimes E=V\otimes M\otimes T_{C}$ or to $E^{\ast }=V^{\ast }\otimes M^{-1}$ . Thus $V$ is isomorphic either to

(7) $$\begin{eqnarray}E^{\prime }\otimes K_{C}\otimes M^{-1}\quad \text{or to}\quad (E^{\prime })^{\ast }\otimes M^{-1}.\end{eqnarray}$$

Now since in particular $g>(r+1)^{2}$ , the open subset $\{M\in \operatorname{Pic}^{g-1}(C):h^{0}(C,V\otimes M)=r+1\}\subseteq B_{1,g-1}^{r+1}(V)$ has a component of dimension $g-(r+1)^{2}\geqslant 1$ . Therefore, we may assume that $M^{2r}\not \cong K_{C}^{r}$ . Thus only one of the bundles in (7) can have trivial determinant. Hence there is only one possibility for $V$ .

In summary, the data of the tangent cone ${\mathcal{T}}_{M}(\unicode[STIX]{x1D6E9}_{V})$ and the point $M$ , together with the property $\det (V)={\mathcal{O}}_{C}$ , determine the bundle $V$ up to isomorphism. In particular, $\unicode[STIX]{x1D6E9}_{V}$ determines  $V$ .◻

Remark 4.2. The involution $M\mapsto K_{C}\otimes M^{-1}$ defines an isomorphism of varieties $\unicode[STIX]{x1D6E9}_{V}\xrightarrow[{}]{{\sim}}\unicode[STIX]{x1D6E9}_{V^{\ast }}$ . We observe that the transposed map $\unicode[STIX]{x1D6EC}^{t}$ occurs naturally as the cup product map defining the tangent cone ${\mathcal{T}}_{K_{C}\otimes M^{-1}}(\unicode[STIX]{x1D6E9}_{V^{\ast }})$ .

Remark 4.3. If $C$ is hyperelliptic, then the canonical map factorises via the hyperelliptic involution $\unicode[STIX]{x1D704}$ . Thus the construction in Proposition 3.3 can never give bundles over $C$ which are not $\unicode[STIX]{x1D704}$ -invariant. We note that Beauville [Bea88] showed that in rank two, if $C$ is hyperelliptic then the bundles $V$ and $\unicode[STIX]{x1D704}^{\ast }V$ have the same theta divisor.