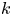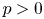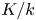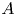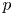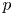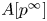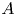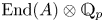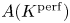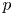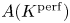1. Introduction
Let ![]() $p$ be a prime number and
$p$ be a prime number and ![]() $k$ a finite field of characteristic
$k$ a finite field of characteristic ![]() $p>0$. Let
$p>0$. Let ![]() $K/k$ be a finitely generated extension of fields (e.g.
$K/k$ be a finitely generated extension of fields (e.g. ![]() $\mathbb {F}_p(t)/\mathbb {F}_p$), fix an algebraic closure
$\mathbb {F}_p(t)/\mathbb {F}_p$), fix an algebraic closure ![]() $K\subseteq \overline {K}$ and write
$K\subseteq \overline {K}$ and write ![]() $K\subseteq K^{\mathrm {perf}}$ for the perfect closure of
$K\subseteq K^{\mathrm {perf}}$ for the perfect closure of ![]() $K$, i.e. the smallest perfect field containing
$K$, i.e. the smallest perfect field containing ![]() $K$ (or, equivalently, the field obtained adding to
$K$ (or, equivalently, the field obtained adding to ![]() $K$ all the
$K$ all the ![]() $p^{n}$-roots of its elements). Let
$p^{n}$-roots of its elements). Let ![]() $A$ be a
$A$ be a ![]() $K$-abelian variety. Motivated by applications to the ‘full’ Mordell–Lang conjecture, in this paper we study the structure of
$K$-abelian variety. Motivated by applications to the ‘full’ Mordell–Lang conjecture, in this paper we study the structure of ![]() $A(K^{\mathrm {perf}})$ using
$A(K^{\mathrm {perf}})$ using ![]() $p$-adic cohomology. The main novelty of our approach is the use of ‘mixed’
$p$-adic cohomology. The main novelty of our approach is the use of ‘mixed’ ![]() $p$-divisible groups and overconvergent
$p$-divisible groups and overconvergent ![]() $F$-isocrystals associated to elements in
$F$-isocrystals associated to elements in ![]() $A(K^{\mathrm {perf}})$.
$A(K^{\mathrm {perf}})$.
1.1 Motivation
In recent years there has been a remarkable interest in the study of the group ![]() $A(K^{\mathrm {perf}})$, see, e.g., [Reference Ambrosi and D'AddezioAD22, Reference Bragg and LieblichBL22, Reference D'AddezioD'A23, Reference Ghioca and MoosaGM06, Reference GhiocaGhi10, Reference RösslerRös15, Reference RösslerRös20, Reference XinyiXin21].
$A(K^{\mathrm {perf}})$, see, e.g., [Reference Ambrosi and D'AddezioAD22, Reference Bragg and LieblichBL22, Reference D'AddezioD'A23, Reference Ghioca and MoosaGM06, Reference GhiocaGhi10, Reference RösslerRös15, Reference RösslerRös20, Reference XinyiXin21].
This interest is mainly motivated by its relation with the ‘full’ Mordell–Lang conjecture (see, e.g., [Reference Ghioca and MoosaGM06, Conjecture 1.2]). Roughly, this conjecture states that if ![]() $\Gamma \subseteq A(\overline K)$ is a finite rank subgroup and
$\Gamma \subseteq A(\overline K)$ is a finite rank subgroup and ![]() $X\subseteq A_{\overline K}$ is an irreducible
$X\subseteq A_{\overline K}$ is an irreducible ![]() $\overline K$-subvariety, then
$\overline K$-subvariety, then ![]() $X(\overline K) \cap \Gamma$ is not Zariski dense, unless
$X(\overline K) \cap \Gamma$ is not Zariski dense, unless ![]() $X$ is a ‘special’ (e.g. the translate of an abelian subvariety of
$X$ is a ‘special’ (e.g. the translate of an abelian subvariety of ![]() $A$).
$A$).
The characteristic zero version of the Mordell–Lang conjecture ![]() $\mathrm {ML}$ is a celebrated theorem of Faltings [Reference FaltingsFal91] for finitely generated subgroups, extended to the finite rank ones by Hindry [Reference HindryHin88]. In our positive characteristic setting, the conjecture has been proved in [Reference HrushovskiHru96] under the extra assumption that
$\mathrm {ML}$ is a celebrated theorem of Faltings [Reference FaltingsFal91] for finitely generated subgroups, extended to the finite rank ones by Hindry [Reference HindryHin88]. In our positive characteristic setting, the conjecture has been proved in [Reference HrushovskiHru96] under the extra assumption that ![]() $\Gamma \otimes \mathbb {Z}_p$ is a finitely generated
$\Gamma \otimes \mathbb {Z}_p$ is a finitely generated ![]() $\mathbb {Z}_p$-module. However, the case of arbitrary subgroups of finite rank has proven to be more elusive and few results are known.
$\mathbb {Z}_p$-module. However, the case of arbitrary subgroups of finite rank has proven to be more elusive and few results are known.
In [Reference Ghioca and MoosaGM06], Ghioca and Moosa reduced the ‘full’ conjecture to the case in which the subgroup ![]() $\Gamma$ is included
$\Gamma$ is included ![]() $A(L^{\mathrm {perf}})$, for
$A(L^{\mathrm {perf}})$, for ![]() $K\subseteq L$ a finite field extension. Combining this with the fact that the conjecture is known when
$K\subseteq L$ a finite field extension. Combining this with the fact that the conjecture is known when ![]() $\Gamma$ is finitely generated, the following question arises naturally.
$\Gamma$ is finitely generated, the following question arises naturally.
Question 1.1.1 When is ![]() $A(K^{\mathrm {perf}})$ finitely generated? What is the structure of
$A(K^{\mathrm {perf}})$ finitely generated? What is the structure of ![]() $A(K^{\mathrm {perf}})$?
$A(K^{\mathrm {perf}})$?
Our main result (Theorem 1.3.1.2) roughly states that whether ![]() $A(K^{\mathrm {perf}})$ is finitely generated or not depends only on the action of
$A(K^{\mathrm {perf}})$ is finitely generated or not depends only on the action of ![]() $\mathrm {End}(A)\otimes \mathbb {Q}_p$ on the
$\mathrm {End}(A)\otimes \mathbb {Q}_p$ on the ![]() $p$-divisible group of
$p$-divisible group of ![]() $A$ and on the
$A$ and on the ![]() $p$-rank of the isogeny factors of
$p$-rank of the isogeny factors of ![]() $A$. As a corollary of our result, one gets the Mordell–Lang conjecture for a sufficiently generic abelian variety with Newton polygon of positive
$A$. As a corollary of our result, one gets the Mordell–Lang conjecture for a sufficiently generic abelian variety with Newton polygon of positive ![]() $p$-rank. To simplify the exposition, we assume for the rest of the introduction that
$p$-rank. To simplify the exposition, we assume for the rest of the introduction that ![]() $A$ is simple and we refer the reader to main text (and, in particular, to Theorem 3.1.1) for the general case.
$A$ is simple and we refer the reader to main text (and, in particular, to Theorem 3.1.1) for the general case.
1.2 Perfect points
Let us recall that, while ![]() $A(K)$ is finitely generated by the Lang–Néron theorem [Reference Lang and NéronLN59], it is well known that
$A(K)$ is finitely generated by the Lang–Néron theorem [Reference Lang and NéronLN59], it is well known that ![]() $A(K^{\mathrm {perf}})$ is not always finitely generated. For example, if
$A(K^{\mathrm {perf}})$ is not always finitely generated. For example, if ![]() $A(K)$ contains a non-torsion element and
$A(K)$ contains a non-torsion element and ![]() $A$ is defined up to isogeny over
$A$ is defined up to isogeny over ![]() $k$ or
$k$ or ![]() $A$ is of
$A$ is of ![]() $p$-rank
$p$-rank ![]() $0$, then
$0$, then ![]() $A(K^{\mathrm {perf}})$ is not finitely generated. Even worst, Helm constructed in [Reference HelmHel22] an ordinary abelian variety without isotrivial isogeny factors such that
$A(K^{\mathrm {perf}})$ is not finitely generated. Even worst, Helm constructed in [Reference HelmHel22] an ordinary abelian variety without isotrivial isogeny factors such that ![]() $A(K^{\mathrm {perf}})$ is not finitely generated. Thus, to have finite generation, one has to impose further conditions.
$A(K^{\mathrm {perf}})$ is not finitely generated. Thus, to have finite generation, one has to impose further conditions.
On the positive side, it is well known that the torsion subgroup ![]() $A(K^{\mathrm {perf}})_{\mathrm {tors}}\subseteq A(K^{\mathrm {perf}})$ is finite (see, for example, [Reference Ghioca and MoosaGM06, p. 7]), so that the interesting part to study is its torsion free quotient
$A(K^{\mathrm {perf}})_{\mathrm {tors}}\subseteq A(K^{\mathrm {perf}})$ is finite (see, for example, [Reference Ghioca and MoosaGM06, p. 7]), so that the interesting part to study is its torsion free quotient ![]() $A(K^{\mathrm {perf}})_{\mathrm {tf}}:=A(K^{\mathrm {perf}})/A(K^{\mathrm {perf}})_{\mathrm {tors}}$. Since the
$A(K^{\mathrm {perf}})_{\mathrm {tf}}:=A(K^{\mathrm {perf}})/A(K^{\mathrm {perf}})_{\mathrm {tors}}$. Since the ![]() $i$th-power Frobenius
$i$th-power Frobenius ![]() $F^i:A\rightarrow A^{(p^i)}$ and the Verschiebung
$F^i:A\rightarrow A^{(p^i)}$ and the Verschiebung ![]() $V^i:A^{(p^i)}\rightarrow A$ induce a factorization
$V^i:A^{(p^i)}\rightarrow A$ induce a factorization

where the union is taken along the injections ![]() $F^i:A(K)\hookrightarrow A^{(p^i)}(K)$, one has that
$F^i:A(K)\hookrightarrow A^{(p^i)}(K)$, one has that
Hence, to study ![]() $A(K^{\mathrm {perf}})$, one is reduced to understand how much the non-torsion elements of
$A(K^{\mathrm {perf}})$, one is reduced to understand how much the non-torsion elements of ![]() $A(K)$ become
$A(K)$ become ![]() $p^n$-divisible in
$p^n$-divisible in ![]() $A(K^{\mathrm {perf}})$. There are essentially two phenomena that can make
$A(K^{\mathrm {perf}})$. There are essentially two phenomena that can make ![]() $A(K^{\mathrm {perf}})$ not finitely generated:
$A(K^{\mathrm {perf}})$ not finitely generated:
(a) there might be a sequence
 $\{x_n\}_{n\in \mathbb {N}}$ of non-torsion elements
$\{x_n\}_{n\in \mathbb {N}}$ of non-torsion elements  $x_n\in A(K)$ such that
$x_n\in A(K)$ such that  $x_n$ becomes
$x_n$ becomes  $p^n$-divisible but not
$p^n$-divisible but not  $p^{n+1}$-divisible; or
$p^{n+1}$-divisible; or(b) there might be a non-torsion element
 $x\in A(K)$ that becomes infinitely
$x\in A(K)$ that becomes infinitely  $p$-divisible in
$p$-divisible in  $A(K^{\mathrm {perf}})$.
$A(K^{\mathrm {perf}})$.
Both cases can happen and our main result says that the occurring of phenomenon (a) depends only on the action of ![]() $\mathrm {End}(A)\otimes \mathbb {Q}_p$ on the
$\mathrm {End}(A)\otimes \mathbb {Q}_p$ on the ![]() $p$-divisible group of
$p$-divisible group of ![]() $A$ and the occurring of phenomenon (b) only on the
$A$ and the occurring of phenomenon (b) only on the ![]() $p$-rank of
$p$-rank of ![]() $A$.
$A$.
1.3 Main results
1.3.1 Finite generation of perfect points
To state our main result, recall that the ![]() $p$-divisible group
$p$-divisible group ![]() $A[p^{\infty }]$ of
$A[p^{\infty }]$ of ![]() $A$ fits into a canonical connected-étale exact sequence
$A$ fits into a canonical connected-étale exact sequence
with ![]() $A[p^{\infty }]^0$ (respectively,
$A[p^{\infty }]^0$ (respectively, ![]() $A[p^{\infty }]^{ \unicode{x00E9}{\textrm{t}}}$) a connected (respectively, étale)
$A[p^{\infty }]^{ \unicode{x00E9}{\textrm{t}}}$) a connected (respectively, étale) ![]() $p$-divisible group. Then we prove the following.
$p$-divisible group. Then we prove the following.
Theorem 1.3.1.2 Assume that ![]() $A(K)\otimes \mathbb {Q}\neq 0$ (and recall that
$A(K)\otimes \mathbb {Q}\neq 0$ (and recall that ![]() $A$ is assumed to be simple). Then:
$A$ is assumed to be simple). Then:
(i)
 $A(K^{\mathrm {perf}})$ is not finitely generated if and only if and there exists an idempotent
$A(K^{\mathrm {perf}})$ is not finitely generated if and only if and there exists an idempotent  $0\neq e\in \mathrm {End}(A)\otimes \mathbb {Q}_p$ (i.e.
$0\neq e\in \mathrm {End}(A)\otimes \mathbb {Q}_p$ (i.e.  $e^2=e$) that acts as
$e^2=e$) that acts as  $0$ on (the isogeny class of)
$0$ on (the isogeny class of)  $A[p^{\infty }]^{ \unicode{x00E9}{\textrm{t}}}$;
$A[p^{\infty }]^{ \unicode{x00E9}{\textrm{t}}}$;(ii) every infinitely
 $p$-divisible point is torsion if and only if
$p$-divisible point is torsion if and only if  $A$ is of positive
$A$ is of positive  $p$-rank.
$p$-rank.
Remark 1.3.1.3 Let us recall that, since ![]() $A$ is simple,
$A$ is simple, ![]() $\mathrm {End}(A)\otimes \mathbb {Q}$ is a division algebra, hence the idempotent appearing in Theorem 1.3.1.2(i) has to live in
$\mathrm {End}(A)\otimes \mathbb {Q}$ is a division algebra, hence the idempotent appearing in Theorem 1.3.1.2(i) has to live in ![]() $\mathrm {End}(A)\otimes \mathbb {Q}_{p}\setminus \mathrm {End}(A)\otimes \mathbb {Q}$. As often happens, it is much easier to construct
$\mathrm {End}(A)\otimes \mathbb {Q}_{p}\setminus \mathrm {End}(A)\otimes \mathbb {Q}$. As often happens, it is much easier to construct ![]() $\mathbb {Q}_p$-linear combination of endomorphisms of
$\mathbb {Q}_p$-linear combination of endomorphisms of ![]() $A$ (i.e. elements in
$A$ (i.e. elements in ![]() $\mathrm {End}(A)\otimes \mathbb {Q}_p$) than actual endomorphisms of
$\mathrm {End}(A)\otimes \mathbb {Q}_p$) than actual endomorphisms of ![]() $A$ (i.e. elements in
$A$ (i.e. elements in ![]() $\mathrm {End}(A)$). This kind of phenomena appears, for example, in the proof of the Tate conjecture for endomorphism of abelian varieties over finite fields [Reference TateTat66].
$\mathrm {End}(A)$). This kind of phenomena appears, for example, in the proof of the Tate conjecture for endomorphism of abelian varieties over finite fields [Reference TateTat66].
Beyond the ordinary case, these seem to be the first general results towards the understanding of the torsion free part of ![]() $A(K^{\mathrm {perf}})$. Coming back to phenomena (a) and (b) of § 1.2, Theorem 1.3.1.2 says that case (a) happens if and only if there exists an idempotent as in Theorem 1.3.1.2(i) and case (b) happens if and only if the
$A(K^{\mathrm {perf}})$. Coming back to phenomena (a) and (b) of § 1.2, Theorem 1.3.1.2 says that case (a) happens if and only if there exists an idempotent as in Theorem 1.3.1.2(i) and case (b) happens if and only if the ![]() $p$-rank of
$p$-rank of ![]() $A$ is
$A$ is ![]() $0$. As an immediate corollary we get the following.
$0$. As an immediate corollary we get the following.
Corollary 1.3.1.4 If ![]() $A$ has positive
$A$ has positive ![]() $p$-rank and
$p$-rank and ![]() $\mathrm {End}(A)\otimes \mathbb {Q}_p$ is a simple algebra, then
$\mathrm {End}(A)\otimes \mathbb {Q}_p$ is a simple algebra, then ![]() $A(K^{\mathrm {perf}})$ is finitely generated.
$A(K^{\mathrm {perf}})$ is finitely generated.
Since for every Newton stratum of positive ![]() $p$-rank of the moduli space of abelian varieties of fixed dimension the generic member has
$p$-rank of the moduli space of abelian varieties of fixed dimension the generic member has ![]() $\mathrm {End}(A_{\overline K})\simeq \mathbb {Z}$, Corollary 1.3.1.4, together with the main results of [Reference HrushovskiHru96] and [Reference Ghioca and MoosaGM06], implies the Mordell–Lang conjecture for such a generic abelian variety.
$\mathrm {End}(A_{\overline K})\simeq \mathbb {Z}$, Corollary 1.3.1.4, together with the main results of [Reference HrushovskiHru96] and [Reference Ghioca and MoosaGM06], implies the Mordell–Lang conjecture for such a generic abelian variety.
1.3.2 Comparison with previous results
We compare Theorem 1.3.1.2 with some of the previously known results, assuming that (![]() $A$ is simple and)
$A$ is simple and) ![]() $A(K)\otimes \mathbb {Q}\neq 0$.
$A(K)\otimes \mathbb {Q}\neq 0$.
As already mentioned, if ![]() $A$ is isogenous to an abelian variety defined over
$A$ is isogenous to an abelian variety defined over ![]() $k$,
$k$, ![]() $A(K^{\mathrm {perf}})$ is not finitely generated. This is coherent with Theorem 1.3.1.2(i), since in this case the sequence (1.3.1.1) splits canonically up to isogeny and this splitting is induced, by the
$A(K^{\mathrm {perf}})$ is not finitely generated. This is coherent with Theorem 1.3.1.2(i), since in this case the sequence (1.3.1.1) splits canonically up to isogeny and this splitting is induced, by the ![]() $p$-adic Tate conjecture for abelian varieties, from an idempotent
$p$-adic Tate conjecture for abelian varieties, from an idempotent ![]() $e\in \mathrm {End}(A)\otimes \mathbb {Q}_p$. Similarly, the fact that if
$e\in \mathrm {End}(A)\otimes \mathbb {Q}_p$. Similarly, the fact that if ![]() $A$ is of
$A$ is of ![]() $p$-rank
$p$-rank ![]() $0$ then
$0$ then ![]() $A(K^{\mathrm {perf}})$ is not finitely generated, is coherent with Theorem 1.3.1.2(i), taking
$A(K^{\mathrm {perf}})$ is not finitely generated, is coherent with Theorem 1.3.1.2(i), taking ![]() $e={\rm Id}_A$.
$e={\rm Id}_A$.
When ![]() $A$ is ordinary, Theorem 1.3.1.2 was essentially already known, since part (ii) follows from [Reference RösslerRös20, Theorem 1.4] and part (i) follows from combining [Reference RösslerRös20, Theorem 1.1]) with [Reference D'AddezioD'A23, Theorem 1.1.3] (and their proofs). Always in the ordinary case, if
$A$ is ordinary, Theorem 1.3.1.2 was essentially already known, since part (ii) follows from [Reference RösslerRös20, Theorem 1.4] and part (i) follows from combining [Reference RösslerRös20, Theorem 1.1]) with [Reference D'AddezioD'A23, Theorem 1.1.3] (and their proofs). Always in the ordinary case, if ![]() $\textrm {Dim}(A)\leq 2$, then
$\textrm {Dim}(A)\leq 2$, then ![]() $A(K^{\mathrm {perf}})$ is always finitely generated: this can be either deduced from [Reference RösslerRös20, Theorem 1.2(g)]) or from Theorem 1.3.1.2(ii).
$A(K^{\mathrm {perf}})$ is always finitely generated: this can be either deduced from [Reference RösslerRös20, Theorem 1.2(g)]) or from Theorem 1.3.1.2(ii).
Remark 1.3.2.1 Most of the results recalled in this section also holds replacing ![]() $k$ with
$k$ with ![]() $\overline k$, assuming that
$\overline k$, assuming that ![]() $A_{\overline K}$ is not isogenous to an abelian variety defined over
$A_{\overline K}$ is not isogenous to an abelian variety defined over ![]() $\overline k$. In addition, our Theorem 1.3.1.2 holds replacing
$\overline k$. In addition, our Theorem 1.3.1.2 holds replacing ![]() $k$ with
$k$ with ![]() $\overline k$, as we show in Theorem 3.4.1, by elaborating the arguments used in the proof of Theorem 1.3.1.2.
$\overline k$, as we show in Theorem 3.4.1, by elaborating the arguments used in the proof of Theorem 1.3.1.2.
1.4 Strategy
Our proof is mostly cohomological, in the sense that we work with ![]() $p$-divisible group and crystals. To lift our cohomological results to
$p$-divisible group and crystals. To lift our cohomological results to ![]() $\mathrm {End}(A)$ and
$\mathrm {End}(A)$ and ![]() $\mathrm {End}(A)\otimes \mathbb {Q}_p$, we use the assumption that
$\mathrm {End}(A)\otimes \mathbb {Q}_p$, we use the assumption that ![]() $K$ is finitely generated over a finite field, to be able to apply the
$K$ is finitely generated over a finite field, to be able to apply the ![]() $p$-adic Tate conjecture for abelian varieties.
$p$-adic Tate conjecture for abelian varieties.
1.4.1  $p$-adic Abel–Jacobi maps
$p$-adic Abel–Jacobi maps
To prove Theorem 1.3.1.2, we start, in § 2, considering various Abel–Jacobi maps. By using the short exact sequence ![]() $0\rightarrow A[p^n]\rightarrow A\xrightarrow {p^n} A\rightarrow 0$ one constructs a Abel–Jacobi map
$0\rightarrow A[p^n]\rightarrow A\xrightarrow {p^n} A\rightarrow 0$ one constructs a Abel–Jacobi map
Composing with the quotient map ![]() $A[p^{\infty }]\rightarrow A[p^{\infty }]^{ \unicode{x00E9}{\textrm{t}}}$, we get a morphism
$A[p^{\infty }]\rightarrow A[p^{\infty }]^{ \unicode{x00E9}{\textrm{t}}}$, we get a morphism
which we call the étale Abel–Jacobi map, and we consider its ![]() $\mathbb {Q}_p$-linearization
$\mathbb {Q}_p$-linearization
which we call the ![]() $p$-adic étale Abel–Jacobi map. In Proposition 2.1.2.1 we prove that every infinitely
$p$-adic étale Abel–Jacobi map. In Proposition 2.1.2.1 we prove that every infinitely ![]() $p$-divisible element is torsion if and only if
$p$-divisible element is torsion if and only if ![]() $\mathrm {AJ}^{ \unicode{x00E9}{\textrm{t}}}$ is injective and that
$\mathrm {AJ}^{ \unicode{x00E9}{\textrm{t}}}$ is injective and that ![]() $A(K^{\mathrm {perf}})$ is finitely generated if and only if
$A(K^{\mathrm {perf}})$ is finitely generated if and only if ![]() $\mathrm {AJ}^{ \unicode{x00E9}{\textrm{t}}}_p$ is injective. Hence, we can translate the two statements of Theorem 1.3.1.2 into two statements on ‘mixed’
$\mathrm {AJ}^{ \unicode{x00E9}{\textrm{t}}}_p$ is injective. Hence, we can translate the two statements of Theorem 1.3.1.2 into two statements on ‘mixed’ ![]() $p$-divisible groups associated to elements in
$p$-divisible groups associated to elements in ![]() $A(K)\otimes \mathbb {Q}_p$ and
$A(K)\otimes \mathbb {Q}_p$ and ![]() $A(K)\otimes \mathbb {Q}$.
$A(K)\otimes \mathbb {Q}$.
Remark 1.4.1.1 Since the two properties of having a non torsion infinitely ![]() $p$-divisible point and having a finitely generated group of perfect points are codified by two different maps (one
$p$-divisible point and having a finitely generated group of perfect points are codified by two different maps (one ![]() $\mathbb {Q}_p$-linear and the other
$\mathbb {Q}_p$-linear and the other ![]() $\mathbb {Q}$-linear), it is natural to consider two different statements in Theorem 1.3.1.2. This is slightly different from what one could aspects from apparently similar motivic conjectures (see, e.g., the Jansen injectivity conjecture [Reference JannsenJan94, Conjecture 9.15]). Roughly, this shows that the behavior of
$\mathbb {Q}$-linear), it is natural to consider two different statements in Theorem 1.3.1.2. This is slightly different from what one could aspects from apparently similar motivic conjectures (see, e.g., the Jansen injectivity conjecture [Reference JannsenJan94, Conjecture 9.15]). Roughly, this shows that the behavior of ![]() $\mathrm {AJ}^{ \unicode{x00E9}{\textrm{t}}}_p$ is not motivic, since
$\mathrm {AJ}^{ \unicode{x00E9}{\textrm{t}}}_p$ is not motivic, since ![]() $\mathrm {AJ}^{ \unicode{x00E9}{\textrm{t}}}_p$ might not be injective even when
$\mathrm {AJ}^{ \unicode{x00E9}{\textrm{t}}}_p$ might not be injective even when ![]() $\mathrm {AJ}^{ \unicode{x00E9}{\textrm{t}}}$ is.
$\mathrm {AJ}^{ \unicode{x00E9}{\textrm{t}}}$ is.
1.4.2  $p$-divisible groups and crystals
$p$-divisible groups and crystals
For ![]() $x\in A(K)\otimes \mathbb {Q}_p$, let
$x\in A(K)\otimes \mathbb {Q}_p$, let
be the exact sequences of ![]() $p$-divisible groups representing
$p$-divisible groups representing ![]() $\mathrm {AJ}_p(x)$ and
$\mathrm {AJ}_p(x)$ and ![]() $\mathrm {AJ}^{ \unicode{x00E9}{\textrm{t}}}_p(x)$. By the finite generation of
$\mathrm {AJ}^{ \unicode{x00E9}{\textrm{t}}}_p(x)$. By the finite generation of ![]() $A(K)$, we know that first does not split and we want to understand when and why second splits. To do this, we spread out
$A(K)$, we know that first does not split and we want to understand when and why second splits. To do this, we spread out ![]() $A\rightarrow K$ to an abelian scheme
$A\rightarrow K$ to an abelian scheme ![]() $\mathcal {A}\rightarrow X$ over some smooth connected
$\mathcal {A}\rightarrow X$ over some smooth connected ![]() $k$-variety
$k$-variety ![]() $X$ with function field
$X$ with function field ![]() $K$ and we consider the category
$K$ and we consider the category ![]() $\mathbf {F\textrm {-}Isoc}(X)$ of
$\mathbf {F\textrm {-}Isoc}(X)$ of ![]() $F$-isocrystals and the fully faithful contravariant Dieudonné functor [Reference Berthelot, Breen and MessingBBM82]
$F$-isocrystals and the fully faithful contravariant Dieudonné functor [Reference Berthelot, Breen and MessingBBM82]
By fully faithfulness, we translate the splitting properties of (1.4.2.1) into analogous splitting properties of an exact sequence of ![]() $F$-isocrystals. As in [Reference Ambrosi and D'AddezioAD22], the advantage of doing this is that we can prove in Proposition 3.3.3.1 that the image via
$F$-isocrystals. As in [Reference Ambrosi and D'AddezioAD22], the advantage of doing this is that we can prove in Proposition 3.3.3.1 that the image via ![]() $\mathbb {D}:\mathbf {pDiv}(X)_{\mathbb {Q}}\rightarrow \mathbf {F\textrm {-}Isoc}(X),$ of the first sequence in (1.4.2.1) lies inside the much better behaved subcategory
$\mathbb {D}:\mathbf {pDiv}(X)_{\mathbb {Q}}\rightarrow \mathbf {F\textrm {-}Isoc}(X),$ of the first sequence in (1.4.2.1) lies inside the much better behaved subcategory ![]() $\mathbf {F\textrm {-}Isoc}^{\dagger}(X)\subseteq \mathbf {F\textrm {-}Isoc}(X)$ of overconvergent
$\mathbf {F\textrm {-}Isoc}^{\dagger}(X)\subseteq \mathbf {F\textrm {-}Isoc}(X)$ of overconvergent ![]() $F$-isocrystals.
$F$-isocrystals.
Since ![]() $\mathbb {D}(A[p^{\infty }])$ is semisimple in
$\mathbb {D}(A[p^{\infty }])$ is semisimple in ![]() $\mathbf {F\textrm {-}Isoc}^{\dagger}(X)$, we can apply recent advances in
$\mathbf {F\textrm {-}Isoc}^{\dagger}(X)$, we can apply recent advances in ![]() $p$-adic cohomology (see [Reference TsuzukiTsu23] and its improvement done in [Reference D'AddezioD'A23]) to construct, from the splitting of
$p$-adic cohomology (see [Reference TsuzukiTsu23] and its improvement done in [Reference D'AddezioD'A23]) to construct, from the splitting of ![]() $\mathrm {AJ}^{ \unicode{x00E9}{\textrm{t}}}_p$, an idempotent in
$\mathrm {AJ}^{ \unicode{x00E9}{\textrm{t}}}_p$, an idempotent in ![]() $\mathrm {End}(A[p^{\infty }])\otimes \mathbb {Q}_p$ with the desired properties, which, since
$\mathrm {End}(A[p^{\infty }])\otimes \mathbb {Q}_p$ with the desired properties, which, since ![]() $K$ is finitely generated over a finite field, lifts to
$K$ is finitely generated over a finite field, lifts to ![]() $\mathrm {End}(A)\otimes \mathbb {Q}_p$, by the
$\mathrm {End}(A)\otimes \mathbb {Q}_p$, by the ![]() $p$-adic Tate conjecture for abelian varieties.
$p$-adic Tate conjecture for abelian varieties.
This is enough to conclude the proof of Theorem 1.3.1.2(i), but to complete the proof of Theorem 1.3.1.2(ii) one needs to show that such a splitting cannot exist if the sequence (1.4.2.1) comes from an ![]() $x\in A(K)\otimes \mathbb {Q}$ and not from a random
$x\in A(K)\otimes \mathbb {Q}$ and not from a random ![]() $x\in A(K)\otimes \mathbb {Q}_p$. This follows from Lemma 2.2.3.2 which shows that even if
$x\in A(K)\otimes \mathbb {Q}_p$. This follows from Lemma 2.2.3.2 which shows that even if ![]() $\mathrm {End}(A[p^{\infty }])$ can be big and with lots of idempotency, one always has that
$\mathrm {End}(A[p^{\infty }])$ can be big and with lots of idempotency, one always has that ![]() $\mathrm {End}(M_x[p^{\infty }])\otimes \mathbb {Q}_p\simeq \mathbb {Q}_p$ if
$\mathrm {End}(M_x[p^{\infty }])\otimes \mathbb {Q}_p\simeq \mathbb {Q}_p$ if ![]() $x\in A(K)\otimes \mathbb {Q}$. This is essentially due to the geometric origin of
$x\in A(K)\otimes \mathbb {Q}$. This is essentially due to the geometric origin of ![]() $M_x[p^{\infty }]$, which makes
$M_x[p^{\infty }]$, which makes ![]() $M_x[p^{\infty }]$ much more rigid for a
$M_x[p^{\infty }]$ much more rigid for a ![]() $x\in A(K)\otimes \mathbb {Q}$ than for a random
$x\in A(K)\otimes \mathbb {Q}$ than for a random ![]() $x\in A(K)\otimes \mathbb {Q}_p$. This extra rigidity is the reason for difference between the two different parts of Theorem 1.3.1.2.
$x\in A(K)\otimes \mathbb {Q}_p$. This extra rigidity is the reason for difference between the two different parts of Theorem 1.3.1.2.
1.5 Organization of the paper
In § 2 we study various ![]() $p$-adic Kummer and Abel–Jacobi maps, their relation with the group of perfect points and with the extensions of
$p$-adic Kummer and Abel–Jacobi maps, their relation with the group of perfect points and with the extensions of ![]() $p$-divisible groups. In § 3 we use this to prove Theorem 1.3.1.2 assuming the overconvergence result Proposition 4.1.2. Finally, in § 4 we prove this overconvergence result.
$p$-divisible groups. In § 3 we use this to prove Theorem 1.3.1.2 assuming the overconvergence result Proposition 4.1.2. Finally, in § 4 we prove this overconvergence result.
2. Abel–Jacobi and étale Abel–Jacobi maps
Let ![]() $S$ be a noetherian
$S$ be a noetherian ![]() $\mathbb {F}_p$-scheme and let
$\mathbb {F}_p$-scheme and let ![]() $A\rightarrow S$ be an abelian scheme. We write
$A\rightarrow S$ be an abelian scheme. We write ![]() $\mathbf {SH}_{\mathrm {fppf}}(S)$ for the category of fppf sheaves in abelian groups on
$\mathbf {SH}_{\mathrm {fppf}}(S)$ for the category of fppf sheaves in abelian groups on ![]() $S$. Write
$S$. Write ![]() $A(S)_{\mathrm {tors}}\subseteq A(S)$ for the torsion subgroup of
$A(S)_{\mathrm {tors}}\subseteq A(S)$ for the torsion subgroup of ![]() $A(S)$,
$A(S)$, ![]() $A(S)_{\mathrm {tf}}:=A(S)/A(S)_{\mathrm {tors}}$ for its torsion free quotient and
$A(S)_{\mathrm {tf}}:=A(S)/A(S)_{\mathrm {tors}}$ for its torsion free quotient and
for its subgroup of infinitely ![]() $p$-divisible elements.
$p$-divisible elements.
2.1 Kummer maps
2.1.1 Kummer map
For every ![]() $n\in \mathbb {N}$, the exact sequence
$n\in \mathbb {N}$, the exact sequence
in ![]() $\mathbf {SH}_{\mathrm {fppf}}(S)$, induces an injective morphism
$\mathbf {SH}_{\mathrm {fppf}}(S)$, induces an injective morphism
and taking the projective limit and tensoring with ![]() $\mathbb {Q}$, we get the following commutative diagram.
$\mathbb {Q}$, we get the following commutative diagram.

We call ![]() $\mathrm {Kum}$:
$\mathrm {Kum}$: ![]() $A(S)\otimes \mathbb {Q}\rightarrow (\varprojlim _n H^1_{\mathrm {fl}}(S,A[p^n]))\otimes \mathbb {Q}$ the Kummer map and
$A(S)\otimes \mathbb {Q}\rightarrow (\varprojlim _n H^1_{\mathrm {fl}}(S,A[p^n]))\otimes \mathbb {Q}$ the Kummer map and ![]() $\mathrm {Kum}_p$:
$\mathrm {Kum}_p$: ![]() $A(S)\otimes \mathbb {Q}_p\rightarrow (\varprojlim _n H^1_{\mathrm {fl}}(S,A[p^n]))\otimes \mathbb {Q}$ the
$A(S)\otimes \mathbb {Q}_p\rightarrow (\varprojlim _n H^1_{\mathrm {fl}}(S,A[p^n]))\otimes \mathbb {Q}$ the ![]() $p$-adic Kummer map. By construction, one has the following lemma, which we state for further reference.
$p$-adic Kummer map. By construction, one has the following lemma, which we state for further reference.
Lemma 2.1.1.1
(i) The Kummer map
 $\mathrm {Kum}$ is injective if and only if
$\mathrm {Kum}$ is injective if and only if  $A(S)_{p^{\infty }}\subseteq A(S)_{\mathrm {tors}}$;
$A(S)_{p^{\infty }}\subseteq A(S)_{\mathrm {tors}}$;(ii) If
 $A(S)_{\mathrm {tf}}$ is finitely generated, then
$A(S)_{\mathrm {tf}}$ is finitely generated, then  $\mathrm {Kum}_p$ is injective.
$\mathrm {Kum}_p$ is injective.
Proof. Statement (i) follows by tensoring with ![]() $\mathbb {Q}$ the short exact sequence
$\mathbb {Q}$ the short exact sequence
For statement (ii), one uses that if ![]() $A(S)_{\mathrm {tf}}$ is finitely generated, then the kernel of
$A(S)_{\mathrm {tf}}$ is finitely generated, then the kernel of ![]() $A(S)\otimes \mathbb {Z}_p\rightarrow \varprojlim _n A(S)/p^n$ is torsion, so that the map
$A(S)\otimes \mathbb {Z}_p\rightarrow \varprojlim _n A(S)/p^n$ is torsion, so that the map ![]() $A(S)\otimes \mathbb {Q}_p\rightarrow (\varprojlim _n A(S)/p^n)\otimes \mathbb {Q}$ is injective.
$A(S)\otimes \mathbb {Q}_p\rightarrow (\varprojlim _n A(S)/p^n)\otimes \mathbb {Q}$ is injective.
2.1.2 Étale Kummer maps
Assume now that ![]() $S=\mathrm {Spec}(K)$ is the spectrum of a field and write
$S=\mathrm {Spec}(K)$ is the spectrum of a field and write ![]() $K^{\mathrm {perf}}$ for the perfection of
$K^{\mathrm {perf}}$ for the perfection of ![]() $K$. Then, the quotient maps
$K$. Then, the quotient maps ![]() $A[p^n]\rightarrow A[p^n]^{ \unicode{x00E9}{\textrm{t}}}$ induce a commutative diagram
$A[p^n]\rightarrow A[p^n]^{ \unicode{x00E9}{\textrm{t}}}$ induce a commutative diagram

where ![]() $T_p(A):=\varprojlim _n A(\overline K)[p^n]$ is the
$T_p(A):=\varprojlim _n A(\overline K)[p^n]$ is the ![]() $p$-adic étale module of
$p$-adic étale module of ![]() $A$ and
$A$ and ![]() $H^1_{ \unicode{x00E9}{\textrm{t}}}(K,T_p(A))$ is its first continuous étale cohomology group. We call
$H^1_{ \unicode{x00E9}{\textrm{t}}}(K,T_p(A))$ is its first continuous étale cohomology group. We call ![]() $\mathrm {Kum}^{ \unicode{x00E9}{\textrm{t}}}$:
$\mathrm {Kum}^{ \unicode{x00E9}{\textrm{t}}}$: ![]() $A(K)\otimes \mathbb {Q}\rightarrow H^1_{ \unicode{x00E9}{\textrm{t}}}(K,T_p(A))\otimes \mathbb {Q}$ the étale Kummer map and
$A(K)\otimes \mathbb {Q}\rightarrow H^1_{ \unicode{x00E9}{\textrm{t}}}(K,T_p(A))\otimes \mathbb {Q}$ the étale Kummer map and ![]() $\mathrm {Kum}^{ \unicode{x00E9}{\textrm{t}}}_p$:
$\mathrm {Kum}^{ \unicode{x00E9}{\textrm{t}}}_p$: ![]() $A(K)\otimes \mathbb {Q}_p\rightarrow H^1_{ \unicode{x00E9}{\textrm{t}}}(K,T_p(A))\otimes \mathbb {Q}$ the
$A(K)\otimes \mathbb {Q}_p\rightarrow H^1_{ \unicode{x00E9}{\textrm{t}}}(K,T_p(A))\otimes \mathbb {Q}$ the ![]() $p$-adic étale Kummer map. The following proposition links the properties of
$p$-adic étale Kummer map. The following proposition links the properties of ![]() $\mathrm {Kum}^{ \unicode{x00E9}{\textrm{t}}}$ and
$\mathrm {Kum}^{ \unicode{x00E9}{\textrm{t}}}$ and ![]() $\mathrm {Kum}^{ \unicode{x00E9}{\textrm{t}}}_p$ with the study of
$\mathrm {Kum}^{ \unicode{x00E9}{\textrm{t}}}_p$ with the study of ![]() $A(K^{\mathrm {perf}})$.
$A(K^{\mathrm {perf}})$.
Proposition 2.1.2.1 We have:
(i)
 $A(K^{\mathrm {perf}})_{p^{\infty }}\subseteq A(K^{\mathrm {perf}})_{\mathrm {tors}}$ if and only if
$A(K^{\mathrm {perf}})_{p^{\infty }}\subseteq A(K^{\mathrm {perf}})_{\mathrm {tors}}$ if and only if  $\mathrm {Kum}^{ \unicode{x00E9}{\textrm{t}}}$ is injective;
$\mathrm {Kum}^{ \unicode{x00E9}{\textrm{t}}}$ is injective;(ii)
 $A(K^{\mathrm {perf}})_{\mathrm {tf}}$ is finitely generated if and only if
$A(K^{\mathrm {perf}})_{\mathrm {tf}}$ is finitely generated if and only if  $A(K)_{\mathrm {tf}}$ is finitely generated and
$A(K)_{\mathrm {tf}}$ is finitely generated and  $\mathrm {Kum}^{ \unicode{x00E9}{\textrm{t}}}_p$ is injective.
$\mathrm {Kum}^{ \unicode{x00E9}{\textrm{t}}}_p$ is injective.
Proof. Let us recall that:
(a) since
 $K\subseteq K^{\mathrm {perf}}$ is purely inseparable, for every finite étale group scheme
$K\subseteq K^{\mathrm {perf}}$ is purely inseparable, for every finite étale group scheme  $G$ the natural map
$G$ the natural map  $H^1(K,G)\rightarrow H^1(K^{\mathrm {perf}},G)$ is an isomorphism (see, e.g., [Sta20, Tag 04DZ]);
$H^1(K,G)\rightarrow H^1(K^{\mathrm {perf}},G)$ is an isomorphism (see, e.g., [Sta20, Tag 04DZ]);(b) if
 $L$ is a perfect field, then
$L$ is a perfect field, then  $H^1_{\mathrm {fl}}(L,H)\rightarrow H^1_{\mathrm {fl}}(L,H^{ \unicode{x00E9}{\textrm{t}}})$ is injective for every finite group scheme
$H^1_{\mathrm {fl}}(L,H)\rightarrow H^1_{\mathrm {fl}}(L,H^{ \unicode{x00E9}{\textrm{t}}})$ is injective for every finite group scheme  $H$ over
$H$ over  $L$, since
$L$, since  $H^1_{\mathrm {fl}}(L,G)=0$ for every finite connected group scheme
$H^1_{\mathrm {fl}}(L,G)=0$ for every finite connected group scheme  $G$ (see, e.g., [Reference Česnavic〶iusČes15, Lemma 2.7(a)]).
$G$ (see, e.g., [Reference Česnavic〶iusČes15, Lemma 2.7(a)]).
Hence, part (i) and the only if part of item (ii) follow from Lemma 2.1.1.1 and the commutative diagram for ![]() $?\in \{\emptyset, p\}$:
$?\in \{\emptyset, p\}$:

where the left vertical isomorphism follows from (1.2.1), the right vertical isomorphism from part (a) and the bottom right injection from part (b).
Thus, we are left to prove that if ![]() $A(K)_{\mathrm {tf}}$ is finitely generated and
$A(K)_{\mathrm {tf}}$ is finitely generated and ![]() $\mathrm {Kum}^{ \unicode{x00E9}{\textrm{t}}}_p$ is injective, then
$\mathrm {Kum}^{ \unicode{x00E9}{\textrm{t}}}_p$ is injective, then ![]() $A(K^{\mathrm {perf}})_{\mathrm {tf}}$ is finitely generated. Since
$A(K^{\mathrm {perf}})_{\mathrm {tf}}$ is finitely generated. Since ![]() $A(K)_{\mathrm {tf}}[1/p]=A(K^{\mathrm {perf}})_{\mathrm {tf}}[1/p]$ is a finitely generated
$A(K)_{\mathrm {tf}}[1/p]=A(K^{\mathrm {perf}})_{\mathrm {tf}}[1/p]$ is a finitely generated ![]() $\mathbb {Z}[1/p]$-module, it is enough to show that
$\mathbb {Z}[1/p]$-module, it is enough to show that ![]() $A(K^{\mathrm {perf}})_{\mathrm {tf}}\otimes \mathbb {Z}_p$ is a finitely generated
$A(K^{\mathrm {perf}})_{\mathrm {tf}}\otimes \mathbb {Z}_p$ is a finitely generated ![]() $\mathbb {Z}_p$-module. Since the kernel of
$\mathbb {Z}_p$-module. Since the kernel of ![]() $\mathrm {Kum}_p^{ \unicode{x00E9}{\textrm{t}}}$ is a torsion group by assumption and
$\mathrm {Kum}_p^{ \unicode{x00E9}{\textrm{t}}}$ is a torsion group by assumption and ![]() $A(K)\otimes \mathbb {Q}=A(K^{\mathrm {perf}})\otimes \mathbb {Q}$, the group
$A(K)\otimes \mathbb {Q}=A(K^{\mathrm {perf}})\otimes \mathbb {Q}$, the group ![]() $A(K^{\mathrm {perf}})_{\mathrm {tf}}\otimes \mathbb {Z}_p$ injects in the torsion free quotient of the image of
$A(K^{\mathrm {perf}})_{\mathrm {tf}}\otimes \mathbb {Z}_p$ injects in the torsion free quotient of the image of ![]() $\mathrm {Kum}_p^{ \unicode{x00E9}{\textrm{t}}}$. Hence, it is enough to show that the image of
$\mathrm {Kum}_p^{ \unicode{x00E9}{\textrm{t}}}$. Hence, it is enough to show that the image of ![]() $A(K^{\mathrm {perf}})\otimes \mathbb {Z}_p$ in
$A(K^{\mathrm {perf}})\otimes \mathbb {Z}_p$ in ![]() $H^1(K^{\mathrm {perf}},T_p(A))\simeq H^1(K,T_p(A))$ lies in a finitely generated sub-
$H^1(K^{\mathrm {perf}},T_p(A))\simeq H^1(K,T_p(A))$ lies in a finitely generated sub-![]() $\mathbb {Z}_p$-module.
$\mathbb {Z}_p$-module.
Since ![]() $A(K)_{\mathrm {tf}}$ is finitely generated, we can choose a set
$A(K)_{\mathrm {tf}}$ is finitely generated, we can choose a set ![]() $x_1,\ldots x_r\in A(K)$ which generates
$x_1,\ldots x_r\in A(K)$ which generates ![]() $A(K)_{\mathrm {tf}}$ and write
$A(K)_{\mathrm {tf}}$ and write ![]() $T_p(M_{x_i})$ for the
$T_p(M_{x_i})$ for the ![]() $\mathbb {Z}_p$-linear
$\mathbb {Z}_p$-linear ![]() $\pi _1(K)$-representation corresponding to the exact sequence
$\pi _1(K)$-representation corresponding to the exact sequence ![]() $\mathrm {Kum}^{ \unicode{x00E9}{\textrm{t}}}(x_i)$
$\mathrm {Kum}^{ \unicode{x00E9}{\textrm{t}}}(x_i)$
Let
be the image of ![]() $\pi _1(K^{\mathrm {perf}})$ acting on
$\pi _1(K^{\mathrm {perf}})$ acting on ![]() $T_p(M_{x_1})\times \cdots \times T_p(M_{x_r})$ and write
$T_p(M_{x_1})\times \cdots \times T_p(M_{x_r})$ and write ![]() $K^{\mathrm {perf}}\subseteq L$ for the Galois extension corresponding to the closed subgroup
$K^{\mathrm {perf}}\subseteq L$ for the Galois extension corresponding to the closed subgroup ![]() $\mathrm {Ker}(\pi _1(K^{\mathrm {perf}})\twoheadrightarrow \Pi )$.
$\mathrm {Ker}(\pi _1(K^{\mathrm {perf}})\twoheadrightarrow \Pi )$.
Since ![]() $\Pi$ is a closed subgroup of
$\Pi$ is a closed subgroup of ![]() $\mathrm {GL}(T_p(A))$, it is a compact
$\mathrm {GL}(T_p(A))$, it is a compact ![]() $p$-adic Lie group by [Reference Dixon, du Sautoy, Mann and SegalDdSMS91, Corollary 9.36]. In particular, by [Reference SerreSer64, Prop. 9],
$p$-adic Lie group by [Reference Dixon, du Sautoy, Mann and SegalDdSMS91, Corollary 9.36]. In particular, by [Reference SerreSer64, Prop. 9], ![]() $H^1(\Pi,T_p(A))\subseteq H^1(K^{\mathrm {perf}},T_p(A))$ is a finitely generated
$H^1(\Pi,T_p(A))\subseteq H^1(K^{\mathrm {perf}},T_p(A))$ is a finitely generated ![]() $\mathbb {Z}_p$-module. We are left to show that the image of
$\mathbb {Z}_p$-module. We are left to show that the image of ![]() $A(K^{\mathrm {perf}})\otimes \mathbb {Z}_p$ in
$A(K^{\mathrm {perf}})\otimes \mathbb {Z}_p$ in ![]() $H^1(K^{\mathrm {perf}},T_p(A))$ lies in
$H^1(K^{\mathrm {perf}},T_p(A))$ lies in ![]() $H^1(\Pi,T_p(A))$. Since
$H^1(\Pi,T_p(A))$. Since ![]() $H^1(\Pi,T_p(A))$ is a sub-
$H^1(\Pi,T_p(A))$ is a sub-![]() $\mathbb {Z}_p$-module of
$\mathbb {Z}_p$-module of ![]() $H^1(K^{\mathrm {perf}},T_p(A))$, it is enough to show that the image of
$H^1(K^{\mathrm {perf}},T_p(A))$, it is enough to show that the image of ![]() $A(K^{\mathrm {perf}})$ lies in
$A(K^{\mathrm {perf}})$ lies in ![]() $H^1(\Pi,T_p(A))$.
$H^1(\Pi,T_p(A))$.
The inflation–restriction exact sequence
reduces us to show that the composition
is the zero map. Since ![]() $\pi _1(L)$ acts trivially on
$\pi _1(L)$ acts trivially on ![]() $T_p(M_{x_i})$, it acts trivially
$T_p(M_{x_i})$, it acts trivially ![]() $T_p(A)$, so that
$T_p(A)$, so that
is torsion free, hence it is enough to show that for every non-torsion ![]() $x\in A(K^{\mathrm {perf}})$, there exists an
$x\in A(K^{\mathrm {perf}})$, there exists an ![]() $n$ such that
$n$ such that ![]() $\phi (p^nx)=0$. Since, by (1.2.1), for every
$\phi (p^nx)=0$. Since, by (1.2.1), for every ![]() $x\in A(K^{\mathrm {perf}})$, there exists an
$x\in A(K^{\mathrm {perf}})$, there exists an ![]() $n$ such that
$n$ such that ![]() $p^nx\in A(K)$, it is enough to show that the map
$p^nx\in A(K)$, it is enough to show that the map
is zero.
Since ![]() $A(K)_{\mathrm {tf}}$ is generated by
$A(K)_{\mathrm {tf}}$ is generated by ![]() $x_1,\ldots, x_r$, it is enough to show that
$x_1,\ldots, x_r$, it is enough to show that ![]() $\phi '(x_i)=0$ for every
$\phi '(x_i)=0$ for every ![]() $1\leq i\leq r$. However, the exact sequence corresponding to
$1\leq i\leq r$. However, the exact sequence corresponding to ![]() $\phi '(x_i)$ is the restriction of the exact sequence (2.1.2.2) to
$\phi '(x_i)$ is the restriction of the exact sequence (2.1.2.2) to ![]() $\pi _1(L)$. By construction, this sequence is an exact sequence of trivial
$\pi _1(L)$. By construction, this sequence is an exact sequence of trivial ![]() $\pi _1(L)$-representations, hence it splits as a
$\pi _1(L)$-representations, hence it splits as a ![]() $\pi _1(L)$-module for all the
$\pi _1(L)$-module for all the ![]() $x_i\in A(K)$. Hence,
$x_i\in A(K)$. Hence, ![]() $\phi '(x_i)=0$ and this concludes the proof.
$\phi '(x_i)=0$ and this concludes the proof.
2.2 Interpretation in terms of Abel–Jacobi maps
In this section, we compare the Kummer map with an Abel–Jacobi map constructed via ![]() $p$-divisible groups and 1-motives.
$p$-divisible groups and 1-motives.
Write ![]() $\mathbf {pDiv}(S)$ for the category of
$\mathbf {pDiv}(S)$ for the category of ![]() $p$-divisible group over
$p$-divisible group over ![]() $S$ and
$S$ and ![]() $\mathbf {pDiv}(S)\otimes \mathbb {Q}$ for its isogeny category.
$\mathbf {pDiv}(S)\otimes \mathbb {Q}$ for its isogeny category.
2.2.1  $p$-divisible group associate to a point
$p$-divisible group associate to a point
Let ![]() $s\in A(S)$ be a section. Since
$s\in A(S)$ be a section. Since ![]() $s:S\rightarrow A$ corresponds to a morphism of fppf
$s:S\rightarrow A$ corresponds to a morphism of fppf ![]() $S$-groups schemes
$S$-groups schemes ![]() $s:\mathbb {Z}\rightarrow A$, we can consider the 1-motive
$s:\mathbb {Z}\rightarrow A$, we can consider the 1-motive ![]() $[s:\mathbb {Z}\rightarrow A]$. We now recall how to associate to
$[s:\mathbb {Z}\rightarrow A]$. We now recall how to associate to ![]() $[s:\mathbb {Z}\rightarrow A]$ a
$[s:\mathbb {Z}\rightarrow A]$ a ![]() $p$-divisible group
$p$-divisible group ![]() $M_s[p^{\infty }]$ over
$M_s[p^{\infty }]$ over ![]() $S$ (see, for example, [Reference Andreatta and Barbieri-VialeAB05, § 1.3] for more details). Define
$S$ (see, for example, [Reference Andreatta and Barbieri-VialeAB05, § 1.3] for more details). Define
so that there is an exact sequence
of finite flat ![]() $S$-group schemes. Define
$S$-group schemes. Define
so that ![]() $M_{s}[p^{\infty }]$ is a
$M_{s}[p^{\infty }]$ is a ![]() $p$-divisible group fitting into an exact sequence
$p$-divisible group fitting into an exact sequence
We let ![]() $[M_s[p^{\infty }]]$ be the corresponding class in
$[M_s[p^{\infty }]]$ be the corresponding class in ![]() $\mathrm {Ext}^1(\mathbb {Q}_p/\mathbb {Z}_p, A[p^\infty ])$.
$\mathrm {Ext}^1(\mathbb {Q}_p/\mathbb {Z}_p, A[p^\infty ])$.
2.2.2 Comparison with the Kummer class
Since ![]() $H^1_{\mathrm {fl}}(S,A[p^n])\simeq \mathrm {Ext}^1(\mathbb {Z}/p^{n}\mathbb {Z}, A[p^n])$, where the latter is the group of extension
$H^1_{\mathrm {fl}}(S,A[p^n])\simeq \mathrm {Ext}^1(\mathbb {Z}/p^{n}\mathbb {Z}, A[p^n])$, where the latter is the group of extension ![]() $A[p^n]$ by
$A[p^n]$ by ![]() $\mathbb {Z}/p^n\mathbb {Z}$ as
$\mathbb {Z}/p^n\mathbb {Z}$ as ![]() $\mathbb {Z}/p^n\mathbb {Z}$-sheaf, the Kummer map can be interpreted as a morphism
$\mathbb {Z}/p^n\mathbb {Z}$-sheaf, the Kummer map can be interpreted as a morphism
On the other hand, since ![]() $\mathrm {Hom}(\mathbb {Z}/p^{n}\mathbb {Z}, A[p^n])$ is finite, taking
$\mathrm {Hom}(\mathbb {Z}/p^{n}\mathbb {Z}, A[p^n])$ is finite, taking ![]() $p^n$-torsion we get a natural injective morphism
$p^n$-torsion we get a natural injective morphism
In the next lemma, which follows essentially from the constructions involved, we prove that ![]() $\varphi ([M_s[p^{\infty }])$ and
$\varphi ([M_s[p^{\infty }])$ and ![]() $\mathrm {Kum}(s)$ represent the same class.
$\mathrm {Kum}(s)$ represent the same class.
Lemma 2.2.2.1 There is an equality ![]() $\mathrm {Kum}(s)=\varphi ([M_s[p^{\infty }]])$.
$\mathrm {Kum}(s)=\varphi ([M_s[p^{\infty }]])$.
Proof. It is enough to show that, for every ![]() $n$, the sequence (2.2.1.1) identifies with the class of
$n$, the sequence (2.2.1.1) identifies with the class of ![]() $\mathrm {Kum}(s)\in H^1_{\mathrm {fl}}(S,A[p^n])\simeq \mathrm {Ext}^1(\mathbb {Z}/p^{n}\mathbb {Z}, A[p^n])$. By definition, the
$\mathrm {Kum}(s)\in H^1_{\mathrm {fl}}(S,A[p^n])\simeq \mathrm {Ext}^1(\mathbb {Z}/p^{n}\mathbb {Z}, A[p^n])$. By definition, the ![]() $A[p^n]$-torsor
$A[p^n]$-torsor ![]() $\mathrm {Kum}(s)\in H^1_{\mathrm {fl}}(S,A[p^n])$ is the pullback of the inclusion of
$\mathrm {Kum}(s)\in H^1_{\mathrm {fl}}(S,A[p^n])$ is the pullback of the inclusion of ![]() $s\hookrightarrow A$ along the multiplication by
$s\hookrightarrow A$ along the multiplication by ![]() $p^n:A\rightarrow A$.
$p^n:A\rightarrow A$.
Let ![]() $\mathbb {Z}/p^n\mathbb {Z}[\mathrm {Kum}(s)]$ be the free
$\mathbb {Z}/p^n\mathbb {Z}[\mathrm {Kum}(s)]$ be the free ![]() $\mathbb {Z}/p^n\mathbb {Z}$-sheaf on
$\mathbb {Z}/p^n\mathbb {Z}$-sheaf on ![]() $[\mathrm {Kum}(s)]$, let
$[\mathrm {Kum}(s)]$, let ![]() $\deg :\mathbb {Z}/p^n\mathbb {Z}[\mathrm {Kum}(s)]\rightarrow \mathbb {Z}/p^n\mathbb {Z}$ be the ‘degree’ map sending
$\deg :\mathbb {Z}/p^n\mathbb {Z}[\mathrm {Kum}(s)]\rightarrow \mathbb {Z}/p^n\mathbb {Z}$ be the ‘degree’ map sending ![]() $\sum n_iz_i$ to
$\sum n_iz_i$ to ![]() $\sum n_i$ and write
$\sum n_i$ and write ![]() $B:=\mathrm {Ker}(\deg )$.
$B:=\mathrm {Ker}(\deg )$.
By construction (see, e.g., [Sta20, 03AJ]), the sequence
in ![]() $\mathrm {Ext}^1(\mathbb {Z}/p^{n}\mathbb {Z}, A[p^n])$ corresponding to
$\mathrm {Ext}^1(\mathbb {Z}/p^{n}\mathbb {Z}, A[p^n])$ corresponding to ![]() $\mathrm {Kum}(s)$, is obtained by pushing out the exact sequence
$\mathrm {Kum}(s)$, is obtained by pushing out the exact sequence
along the map ![]() $B\rightarrow A[p^n]$ sending the generators of the form
$B\rightarrow A[p^n]$ sending the generators of the form ![]() $x-x'$ to the unique
$x-x'$ to the unique ![]() $a$ such that
$a$ such that ![]() $x+a=x'$. The isomorphism of the sequence (2.2.2.2) with the sequence (2.2.1.1) is then induced by the map
$x+a=x'$. The isomorphism of the sequence (2.2.2.2) with the sequence (2.2.1.1) is then induced by the map ![]() $\widetilde {\mathrm {Kum}(s)}\rightarrow M_x[p^n]$ obtained by the universal property of pushout using the natural inclusion
$\widetilde {\mathrm {Kum}(s)}\rightarrow M_x[p^n]$ obtained by the universal property of pushout using the natural inclusion ![]() $A[p^n]\subseteq \{0\}\times A\subseteq \mathbb {Z}\times A$ and the map
$A[p^n]\subseteq \{0\}\times A\subseteq \mathbb {Z}\times A$ and the map ![]() $\mathbb {Z}[\mathrm {Kum}(s)]\rightarrow A$ sending
$\mathbb {Z}[\mathrm {Kum}(s)]\rightarrow A$ sending ![]() $s\in \mathrm {Kum}(s)$ to
$s\in \mathrm {Kum}(s)$ to ![]() $(1,-s)\in \mathbb {Z}\times A$.
$(1,-s)\in \mathbb {Z}\times A$.
Hence, from now on, if ![]() $S=\mathrm {Spec}(K)$ is the spectrum of a field, we interpret, for
$S=\mathrm {Spec}(K)$ is the spectrum of a field, we interpret, for ![]() $?\in \{\emptyset, p\}$ and
$?\in \{\emptyset, p\}$ and ![]() $\Delta \in \{\emptyset, { \unicode{x00E9}{\textrm{t}}}\}$ the Kummer maps as (
$\Delta \in \{\emptyset, { \unicode{x00E9}{\textrm{t}}}\}$ the Kummer maps as (![]() $p$-adic, étale) Abel–Jacobi maps
$p$-adic, étale) Abel–Jacobi maps
We can then rephrase the work done in this section in the following corollary, which is a direct consequence of Proposition 2.1.2.1 and Lemma 2.2.2.1.
Corollary 2.2.2.3 We have:
(i)
 $A(K^{\mathrm {perf}})_{\mathrm {tf}}$ is finitely generated if and only if
$A(K^{\mathrm {perf}})_{\mathrm {tf}}$ is finitely generated if and only if  $A(K)_{\mathrm {tf}}$ is finitely generated and
$A(K)_{\mathrm {tf}}$ is finitely generated and  $\mathrm {AJ}^{ \unicode{x00E9}{\textrm{t}}}_{p}$ is injective;
$\mathrm {AJ}^{ \unicode{x00E9}{\textrm{t}}}_{p}$ is injective;(ii)
 $A(K^{\mathrm {perf}})_{p^{\infty }}\subseteq A(K^{\mathrm {perf}})_{\mathrm {tors}}$ if and only if
$A(K^{\mathrm {perf}})_{p^{\infty }}\subseteq A(K^{\mathrm {perf}})_{\mathrm {tors}}$ if and only if  $\mathrm {AJ}^{ \unicode{x00E9}{\textrm{t}}}$ is injective.
$\mathrm {AJ}^{ \unicode{x00E9}{\textrm{t}}}$ is injective.
2.2.3 Rigidity of the Abel–Jacobi extension
Suppose that ![]() $S=Spec(K)$ for a finitely generated field
$S=Spec(K)$ for a finitely generated field ![]() $K$ over
$K$ over ![]() $\mathbb {F}_p$. We give a first application of the interpretation of
$\mathbb {F}_p$. We give a first application of the interpretation of ![]() $\mathrm {Kum}$ in terms of
$\mathrm {Kum}$ in terms of ![]() $p$-divisible groups, proving that the extensions in the image of
$p$-divisible groups, proving that the extensions in the image of ![]() $\mathrm {AJ}$ are more rigid than a general extension in the image of
$\mathrm {AJ}$ are more rigid than a general extension in the image of ![]() $\mathrm {AJ}_p$. This sets an important difference between the maps
$\mathrm {AJ}_p$. This sets an important difference between the maps ![]() $\mathrm {AJ}$ and
$\mathrm {AJ}$ and ![]() $\mathrm {AJ}_p$ and it is the reason why one has to consider two different statements in Theorem 1.3.1.2.
$\mathrm {AJ}_p$ and it is the reason why one has to consider two different statements in Theorem 1.3.1.2.
We begin with an easy but important lemma, which is the only place in which some assumption on the geometry of ![]() $A$ is used.
$A$ is used.
Lemma 2.2.3.1 Assume that ![]() $A$ is simple and
$A$ is simple and ![]() $x\in A(K)$ is a non-torsion point. Then the map
$x\in A(K)$ is a non-torsion point. Then the map
sending ![]() $f$ to
$f$ to ![]() $f(x)$ is injective.
$f(x)$ is injective.
Proof. Take any morphism ![]() $f:A\rightarrow A$ such that
$f:A\rightarrow A$ such that ![]() $f(x)=0$. If
$f(x)=0$. If ![]() $f:A\rightarrow A$ is not the zero map, then, since
$f:A\rightarrow A$ is not the zero map, then, since ![]() $A$ is simple,
$A$ is simple, ![]() $\mathrm {Ker}(f)$ is finite. On the other hand,
$\mathrm {Ker}(f)$ is finite. On the other hand, ![]() $x$ is in
$x$ is in ![]() $\mathrm {Ker}(f)$ which is a contradiction with the fact that
$\mathrm {Ker}(f)$ which is a contradiction with the fact that ![]() $x$ is not torsion.
$x$ is not torsion.
Then one has the following result, which is a consequence of the Tate conjecture for abelian varieties and a concrete incarnation of the Tate conjecture for 1-motives.
Lemma 2.2.3.2 If ![]() $A$ is simple and
$A$ is simple and ![]() $x\in A(K)$ is not torsion, then
$x\in A(K)$ is not torsion, then ![]() $\mathrm {End}_{\mathbf {pDiv}(K)}(M_{x}[p^{\infty }])\simeq \mathbb {Z}_p$.
$\mathrm {End}_{\mathbf {pDiv}(K)}(M_{x}[p^{\infty }])\simeq \mathbb {Z}_p$.
Proof. Applying the functor ![]() $\mathrm {Hom}_{\mathbf {pDiv}(K)}(M_x[p^{\infty }],-)$ to the exact sequence (2.2.1.2) we get an exact sequence
$\mathrm {Hom}_{\mathbf {pDiv}(K)}(M_x[p^{\infty }],-)$ to the exact sequence (2.2.1.2) we get an exact sequence
Since ![]() $\mathrm {Hom}_{\mathbf {pDiv}(K)}(A[p^{\infty }],\mathbb {Q}_p/\mathbb {Z}_p)=0$, applying the functor
$\mathrm {Hom}_{\mathbf {pDiv}(K)}(A[p^{\infty }],\mathbb {Q}_p/\mathbb {Z}_p)=0$, applying the functor ![]() $\mathrm {Hom}_{\mathbf {pDiv}(K)}(-,\mathbb {Q}_p/\mathbb {Z}_p)$ to (2.2.1.2) one sees that
$\mathrm {Hom}_{\mathbf {pDiv}(K)}(-,\mathbb {Q}_p/\mathbb {Z}_p)$ to (2.2.1.2) one sees that ![]() $\mathrm {Hom}_{\mathbf {pDiv}(K)}(M_x[p^{\infty }],\mathbb {Q}_p/\mathbb {Z}_p)\simeq \mathrm {End}_{\mathbf {pDiv}(K)}(\mathbb {Q}_p/\mathbb {Z}_p)\simeq \mathbb {Z}_p$. Hence, it is then enough to prove that
$\mathrm {Hom}_{\mathbf {pDiv}(K)}(M_x[p^{\infty }],\mathbb {Q}_p/\mathbb {Z}_p)\simeq \mathrm {End}_{\mathbf {pDiv}(K)}(\mathbb {Q}_p/\mathbb {Z}_p)\simeq \mathbb {Z}_p$. Hence, it is then enough to prove that
Since ![]() $\mathrm {Hom}_{\mathbf {pDiv}(K)}(\mathbb {Q}_p/\mathbb {Z}_p,A[p^{\infty }])=0$, applying the functor
$\mathrm {Hom}_{\mathbf {pDiv}(K)}(\mathbb {Q}_p/\mathbb {Z}_p,A[p^{\infty }])=0$, applying the functor ![]() $\mathrm {Hom}_{\mathbf {pDiv}(K)}(-,A[p^{\infty }])$ to the exact sequence (2.2.1.2) we get an exact sequence
$\mathrm {Hom}_{\mathbf {pDiv}(K)}(-,A[p^{\infty }])$ to the exact sequence (2.2.1.2) we get an exact sequence
Since ![]() $\mathrm {Hom}_{\mathbf {pDiv}(K)}(M[p^{\infty }],A[p^{\infty }])$ is torsion free, we are left to show that the natural map
$\mathrm {Hom}_{\mathbf {pDiv}(K)}(M[p^{\infty }],A[p^{\infty }])$ is torsion free, we are left to show that the natural map
is injective.
Consider the commutative diagram

where ![]() $\psi _x\otimes \text {Id}$ is induced by the map
$\psi _x\otimes \text {Id}$ is induced by the map ![]() $\psi _x:\mathrm {End}(A)\rightarrow A(K)$ sending a morphism
$\psi _x:\mathrm {End}(A)\rightarrow A(K)$ sending a morphism ![]() $f$ to
$f$ to ![]() $f(x)$. Since
$f(x)$. Since ![]() $K$ if finitely generated,
$K$ if finitely generated, ![]() $A(K)$ is a finitely generated group, hence by Lemma 2.1.1.1
$A(K)$ is a finitely generated group, hence by Lemma 2.1.1.1 ![]() $\mathrm {AJ}_p$ is injective. By the
$\mathrm {AJ}_p$ is injective. By the ![]() $p$-adic Tate conjecture for abelian varieties proved in [Reference de JongdJ98, Theorem 2.6], the left vertical map is an isomorphism. Thus, since
$p$-adic Tate conjecture for abelian varieties proved in [Reference de JongdJ98, Theorem 2.6], the left vertical map is an isomorphism. Thus, since ![]() $A$ is simple, we conclude by using Lemma 2.2.3.1.
$A$ is simple, we conclude by using Lemma 2.2.3.1.
2.2.4 Kummer class and semiabelian schemes
Let ![]() $s\in A(S)$. As a second application of the interpretation of
$s\in A(S)$. As a second application of the interpretation of ![]() $\mathrm {Kum}$ in terms of
$\mathrm {Kum}$ in terms of ![]() $p$-divisible groups we give a geometric interpretation of the Cartier dual of the class of
$p$-divisible groups we give a geometric interpretation of the Cartier dual of the class of ![]() $[M_x[p^{\infty }]]$. This will be important to prove Proposition 3.3.3.1. The dual of the 1-motive
$[M_x[p^{\infty }]]$. This will be important to prove Proposition 3.3.3.1. The dual of the 1-motive ![]() $[\mathbb {Z}\rightarrow A]$ is a semiabelian scheme
$[\mathbb {Z}\rightarrow A]$ is a semiabelian scheme
where ![]() $A^{\vee }$ is the dual abelian variety, and the
$A^{\vee }$ is the dual abelian variety, and the ![]() $p$-divisible group
$p$-divisible group ![]() $G_s[p^{\infty }]$ of
$G_s[p^{\infty }]$ of ![]() $G_s$ is the Cartier dual
$G_s$ is the Cartier dual ![]() $M_{s}[p^{\infty }]^{\vee }$ of
$M_{s}[p^{\infty }]^{\vee }$ of ![]() $M_{s}[p^{\infty }]$ (see, for example, [Reference Andreatta and Barbieri-VialeAB05, § 1.3]). Hence, the class of the dual of the extension (2.2.1.2) in
$M_{s}[p^{\infty }]$ (see, for example, [Reference Andreatta and Barbieri-VialeAB05, § 1.3]). Hence, the class of the dual of the extension (2.2.1.2) in ![]() $\mathrm {Ext}^1(A^{\vee }[p^{\infty }],\mu _{p^{\infty }})$ is the extension
$\mathrm {Ext}^1(A^{\vee }[p^{\infty }],\mu _{p^{\infty }})$ is the extension
associated to the ![]() $p$-divisible group of a semi-abelian
$p$-divisible group of a semi-abelian ![]() $S$-scheme
$S$-scheme ![]() $G_s\rightarrow S$.
$G_s\rightarrow S$.
3. On the injectivity of the étale Abel–Jacobi map
In this section, we prove the main theorem of the paper (Theorem 3.1.1) and its geometric variant (Theorem 3.4.1) assuming an overconvergence result (Proposition 4.1.2) which will be proved in the next § 4 (since it relies on different techniques).
3.1 Notation and statements
We assume that ![]() $k$ is a finite field,
$k$ is a finite field, ![]() $K/k$ is a finitely generated field extension and
$K/k$ is a finitely generated field extension and ![]() $A$ a
$A$ a ![]() $K$-abelian variety. Write
$K$-abelian variety. Write ![]() $p(A)$ (respectively,
$p(A)$ (respectively, ![]() $r(A)$) for the
$r(A)$) for the ![]() $p$-rank of
$p$-rank of ![]() $A$ (respectively, the rank of
$A$ (respectively, the rank of ![]() $A(K)$, which is finite by the Lang–Néron theorem) and if
$A(K)$, which is finite by the Lang–Néron theorem) and if ![]() $A_1,\ldots, A_n$ are the simple isogeny factors of
$A_1,\ldots, A_n$ are the simple isogeny factors of ![]() $A$, set
$A$, set ![]() $p(A)^{\mathrm {min}}$ (respectively,
$p(A)^{\mathrm {min}}$ (respectively, ![]() $r(A)^{\mathrm {min}}$) as the minimum of
$r(A)^{\mathrm {min}}$) as the minimum of ![]() $p(A_i)$ (respectively, of
$p(A_i)$ (respectively, of ![]() $r(A_i)$). If
$r(A_i)$). If ![]() $e\in \mathrm {End}(A)\otimes \mathbb {Q}_p$, we write
$e\in \mathrm {End}(A)\otimes \mathbb {Q}_p$, we write ![]() $e[p^{\infty }]\in \mathrm {End}(A[p^{\infty }])\otimes \mathbb {Q}_p$ (respectively,
$e[p^{\infty }]\in \mathrm {End}(A[p^{\infty }])\otimes \mathbb {Q}_p$ (respectively, ![]() $e[p^{\infty }]^{ \unicode{x00E9}{\textrm{t}}}\in \mathrm {End}(A[p^{\infty }]^{ \unicode{x00E9}{\textrm{t}}})\otimes \mathbb {Q}$) for the induced morphism. Finally, set
$e[p^{\infty }]^{ \unicode{x00E9}{\textrm{t}}}\in \mathrm {End}(A[p^{\infty }]^{ \unicode{x00E9}{\textrm{t}}})\otimes \mathbb {Q}$) for the induced morphism. Finally, set
In this section, we prove the following.
Theorem 3.1.1 Assume that ![]() $r(A)^{\mathrm {min}}>0$. Then:
$r(A)^{\mathrm {min}}>0$. Then:
(i)
 $A(K^{\mathrm {perf}})$ is not finitely generated if and only if and there exists an idempotent
$A(K^{\mathrm {perf}})$ is not finitely generated if and only if and there exists an idempotent  $0\neq e\in \mathrm {End}(A)\otimes \mathbb {Q}_p$ (i.e.
$0\neq e\in \mathrm {End}(A)\otimes \mathbb {Q}_p$ (i.e.  $e^2=e$) such that
$e^2=e$) such that  $0=e[p^{\infty }]^{ \unicode{x00E9}{\textrm{t}}}\in \mathrm {End}(A[p^{\infty }]^{ \unicode{x00E9}{\textrm{t}}})\otimes \mathbb {Q}_p$;
$0=e[p^{\infty }]^{ \unicode{x00E9}{\textrm{t}}}\in \mathrm {End}(A[p^{\infty }]^{ \unicode{x00E9}{\textrm{t}}})\otimes \mathbb {Q}_p$;(ii)
 $A(K^{\mathrm {perf}})_{p^{\infty }}\subseteq A(K^{\mathrm {perf}})_{\mathrm {tors}}$ if and only if
$A(K^{\mathrm {perf}})_{p^{\infty }}\subseteq A(K^{\mathrm {perf}})_{\mathrm {tors}}$ if and only if  $p(A)^{\mathrm {min}}>0$.
$p(A)^{\mathrm {min}}>0$.
Since ![]() $A(K^{\mathrm {perf}})_{\mathrm {tors}}$ is finite by [Reference Ghioca and MoosaGM06, p. 7], thanks to Corollary 2.2.2.3, Theorem 1.3.1.2 is equivalent to the following.
$A(K^{\mathrm {perf}})_{\mathrm {tors}}$ is finite by [Reference Ghioca and MoosaGM06, p. 7], thanks to Corollary 2.2.2.3, Theorem 1.3.1.2 is equivalent to the following.
Theorem 3.1.2 Assume that ![]() $r(A)^{\mathrm {min}}>0$. Then:
$r(A)^{\mathrm {min}}>0$. Then:
(i) the morphism
is not injective if and only there exists an idempotent \[ \mathrm{AJ}^{ \unicode{x00E9}{\textrm{t}}}_p: A(K)\otimes \mathbb{Q}_p\rightarrow \mathrm{Ext}^1_{\mathbf{pDiv}(K)}(\mathbb{Q}_p/\mathbb{Z}_p, A[p^{\infty}]^{ \unicode{x00E9}{\textrm{t}}})\otimes\mathbb{Q} \]
\[ \mathrm{AJ}^{ \unicode{x00E9}{\textrm{t}}}_p: A(K)\otimes \mathbb{Q}_p\rightarrow \mathrm{Ext}^1_{\mathbf{pDiv}(K)}(\mathbb{Q}_p/\mathbb{Z}_p, A[p^{\infty}]^{ \unicode{x00E9}{\textrm{t}}})\otimes\mathbb{Q} \]
 $0\neq e\in \mathrm {End}(A)\otimes \mathbb {Q}_p$ such that
$0\neq e\in \mathrm {End}(A)\otimes \mathbb {Q}_p$ such that  $0=e[p^{\infty }]^{ \unicode{x00E9}{\textrm{t}}}\in \mathrm {End}(A[p^{\infty }]^{ \unicode{x00E9}{\textrm{t}}})\otimes \mathbb {Q}$;
$0=e[p^{\infty }]^{ \unicode{x00E9}{\textrm{t}}}\in \mathrm {End}(A[p^{\infty }]^{ \unicode{x00E9}{\textrm{t}}})\otimes \mathbb {Q}$;(ii) the morphism
is not injective if and only if \[ \mathrm{AJ}^{ \unicode{x00E9}{\textrm{t}}}: A(K)\otimes \mathbb{Q}\rightarrow \mathrm{Ext}^1_{\mathbf{pDiv}(K)}(\mathbb{Q}_p/\mathbb{Z}_p, A[p^{\infty}]^{ \unicode{x00E9}{\textrm{t}}})\otimes \mathbb{Q} \]
\[ \mathrm{AJ}^{ \unicode{x00E9}{\textrm{t}}}: A(K)\otimes \mathbb{Q}\rightarrow \mathrm{Ext}^1_{\mathbf{pDiv}(K)}(\mathbb{Q}_p/\mathbb{Z}_p, A[p^{\infty}]^{ \unicode{x00E9}{\textrm{t}}})\otimes \mathbb{Q} \]
 $p(A)^{\mathrm {min}}=0$.
$p(A)^{\mathrm {min}}=0$.
3.2 Preliminaries and the first implication
3.2.1 Reduction to  $A$ simple
$A$ simple
Since the assumptions and the conclusions are stable under products and isogenies of abelian varieties, we can assume that ![]() $A$ is simple (that will be used to apply Lemmas 2.2.3.1 and 2.2.3.2) and
$A$ is simple (that will be used to apply Lemmas 2.2.3.1 and 2.2.3.2) and ![]() $r(A)>0$. Since the statements with
$r(A)>0$. Since the statements with ![]() $p(A)=0$ are trivial, we can assume that
$p(A)=0$ are trivial, we can assume that ![]() $p(A)>0$.
$p(A)>0$.
3.2.2 First implication
We first prove the if part of Theorem 3.1.2(i). Assume that there exists an idempotent ![]() $0\neq e\in \mathrm {End}(A)\otimes \mathbb {Q}_p$ such that
$0\neq e\in \mathrm {End}(A)\otimes \mathbb {Q}_p$ such that ![]() $e[p^{\infty }]^{ \unicode{x00E9}{\textrm{t}}}=0$ in
$e[p^{\infty }]^{ \unicode{x00E9}{\textrm{t}}}=0$ in ![]() $\mathrm {End}(A[p^{\infty }]^{ \unicode{x00E9}{\textrm{t}}})\otimes \mathbb {Q}_p$. Choose an
$\mathrm {End}(A[p^{\infty }]^{ \unicode{x00E9}{\textrm{t}}})\otimes \mathbb {Q}_p$. Choose an ![]() $n$ such that
$n$ such that ![]() $p^ne=:u\in \mathrm {End}(A)\otimes \mathbb {Z}_p$. Since
$p^ne=:u\in \mathrm {End}(A)\otimes \mathbb {Z}_p$. Since ![]() $e[p^{\infty }]^{ \unicode{x00E9}{\textrm{t}}}=0$ and
$e[p^{\infty }]^{ \unicode{x00E9}{\textrm{t}}}=0$ and ![]() $\mathrm {End}(A[p^{\infty }]^{ \unicode{x00E9}{\textrm{t}}})$ is torsion free, also
$\mathrm {End}(A[p^{\infty }]^{ \unicode{x00E9}{\textrm{t}}})$ is torsion free, also ![]() $u[p^{\infty }]^{ \unicode{x00E9}{\textrm{t}}}=0$. Take a non-torsion
$u[p^{\infty }]^{ \unicode{x00E9}{\textrm{t}}}=0$. Take a non-torsion ![]() $x\in A(K)$ (which exists by assumption). Since
$x\in A(K)$ (which exists by assumption). Since ![]() $A$ is simple, by Lemma 2.2.3.1, the map
$A$ is simple, by Lemma 2.2.3.1, the map
is injective, where ![]() $\psi _x:\mathrm {End}(A)\rightarrow A(K)$ is the map sending
$\psi _x:\mathrm {End}(A)\rightarrow A(K)$ is the map sending ![]() $f$ to
$f$ to ![]() $f(x)$. Hence,
$f(x)$. Hence, ![]() $e(x)\neq 0$, therefore
$e(x)\neq 0$, therefore ![]() $u(x)\neq 0$. The commutative diagram
$u(x)\neq 0$. The commutative diagram

shows that ![]() $u(x)$ goes to zero in
$u(x)$ goes to zero in ![]() $\mathrm {Ext}_{\mathbf {pDiv}(K)}^{1}(\mathbb {Q}_p/\mathbb {Z}_p,A[p^{\infty }]^{ \unicode{x00E9}{\textrm{t}}})\otimes \mathbb {Q}$. This concludes the proof of the if part of Theorem 3.1.2(i).
$\mathrm {Ext}_{\mathbf {pDiv}(K)}^{1}(\mathbb {Q}_p/\mathbb {Z}_p,A[p^{\infty }]^{ \unicode{x00E9}{\textrm{t}}})\otimes \mathbb {Q}$. This concludes the proof of the if part of Theorem 3.1.2(i).
3.2.3 Reduction to Proposition 3.2.3.1
We are left to prove the only if part of Theorem 3.1.2(i) and 3.1.2(ii). We first show that the following Proposition 3.2.3.1 implies Theorem 3.1.2.
Proposition 3.2.3.1 Let ![]() $x\in A(K)\otimes \mathbb {Q}_p$ be such that
$x\in A(K)\otimes \mathbb {Q}_p$ be such that ![]() $\mathrm {AJ}_p(x)=0$. Then there exists an idempotent
$\mathrm {AJ}_p(x)=0$. Then there exists an idempotent ![]() $0\neq e\in \mathrm {End}(M_x[p^{\infty }])\otimes \mathbb {Q}_p$ which preserves the sub-
$0\neq e\in \mathrm {End}(M_x[p^{\infty }])\otimes \mathbb {Q}_p$ which preserves the sub-![]() $p$-divisible group
$p$-divisible group ![]() $A[p^{\infty }]\subseteq M_x[p^{\infty }]$ and it induces a non-zero idempotent
$A[p^{\infty }]\subseteq M_x[p^{\infty }]$ and it induces a non-zero idempotent ![]() $e[p^{\infty }]\in \mathrm {End}(A[p^{\infty }])\otimes \mathbb {Q}_p$ acting as
$e[p^{\infty }]\in \mathrm {End}(A[p^{\infty }])\otimes \mathbb {Q}_p$ acting as ![]() $0$ on
$0$ on ![]() $A_x[p^{\infty }]^{ \unicode{x00E9}{\textrm{t}}}$.
$A_x[p^{\infty }]^{ \unicode{x00E9}{\textrm{t}}}$.
Assume that Proposition 3.2.3.1 holds. Then Theorem 3.1.2(ii) follows from it and Lemma 2.2.3.1. To deduce Theorem 3.1.2(i), we use that, by the ![]() $p$-adic Tate conjecture for abelian varieties proved in [Reference de JongdJ98, Theorem 2.6], the natural map
$p$-adic Tate conjecture for abelian varieties proved in [Reference de JongdJ98, Theorem 2.6], the natural map
is an isomorphism, so that ![]() $e[p^{\infty }]$ is induced by a non-zero idempotent in
$e[p^{\infty }]$ is induced by a non-zero idempotent in ![]() $\mathrm {End}(A)\otimes \mathbb {Q}_p$ acting as
$\mathrm {End}(A)\otimes \mathbb {Q}_p$ acting as ![]() $0$ on
$0$ on ![]() $A_x[p^{\infty }]^{ \unicode{x00E9}{\textrm{t}}}$. Hence, we are left to prove Proposition 3.2.3.1.
$A_x[p^{\infty }]^{ \unicode{x00E9}{\textrm{t}}}$. Hence, we are left to prove Proposition 3.2.3.1.
3.3 Proof of Proposition 3.2.3.1
3.3.1 Spreading out
Let ![]() $x\in A(K)\otimes \mathbb {Q}_p$ be such that
$x\in A(K)\otimes \mathbb {Q}_p$ be such that ![]() $\mathrm {AJ}_p(x)=0$. To prove Proposition 3.2.3.1 we can replace
$\mathrm {AJ}_p(x)=0$. To prove Proposition 3.2.3.1 we can replace ![]() $x$ with
$x$ with ![]() $p^nx$, hence we may and do assume that
$p^nx$, hence we may and do assume that ![]() $x\in A(K)\otimes \mathbb {Z}_p$ is not torsion. Let
$x\in A(K)\otimes \mathbb {Z}_p$ is not torsion. Let
and
be the extensions associated to ![]() $\mathrm {AJ}_p(x)$ and
$\mathrm {AJ}_p(x)$ and ![]() $AJ^{ \unicode{x00E9}{\textrm{t}}}_p(x)$, respectively. Since
$AJ^{ \unicode{x00E9}{\textrm{t}}}_p(x)$, respectively. Since ![]() $AJ^{ \unicode{x00E9}{\textrm{t}}}_p(x)=0$, the exact sequence (3.3.1.2) splits. Replacing
$AJ^{ \unicode{x00E9}{\textrm{t}}}_p(x)=0$, the exact sequence (3.3.1.2) splits. Replacing ![]() $k$ with a finite field extension, we can assume that
$k$ with a finite field extension, we can assume that ![]() $k$ is algebraically closed in
$k$ is algebraically closed in ![]() $K$ and take an affine smooth geometrically connected
$K$ and take an affine smooth geometrically connected ![]() $k$-variety
$k$-variety ![]() $X$ with function field
$X$ with function field ![]() $K$. Replacing
$K$. Replacing ![]() $X$ with a dense open subset, we can assume that
$X$ with a dense open subset, we can assume that ![]() $A$ extends to an abelian scheme
$A$ extends to an abelian scheme ![]() $\mathcal {A}\rightarrow X$ with constant Newton polygon and that, since
$\mathcal {A}\rightarrow X$ with constant Newton polygon and that, since ![]() $A(K)$ is finitely generated, the natural map
$A(K)$ is finitely generated, the natural map ![]() $\mathcal {A}(X)\otimes \mathbb {Z}_p\rightarrow A(K)\otimes \mathbb {Z}_p$ is an isomorphism. In particular,
$\mathcal {A}(X)\otimes \mathbb {Z}_p\rightarrow A(K)\otimes \mathbb {Z}_p$ is an isomorphism. In particular, ![]() $x$ extends to a non-torsion element
$x$ extends to a non-torsion element ![]() $\mathfrak t\in \mathcal {A}(X)\otimes \mathbb {Z}_p$. By [Reference de JongdJ95], the natural functor
$\mathfrak t\in \mathcal {A}(X)\otimes \mathbb {Z}_p$. By [Reference de JongdJ95], the natural functor
is fully faithful, so that our assumption is equivalent to the fact that the sequence
splits in ![]() $\mathbf {pDiv}(X)\otimes \mathbb {Q}$ and we know (by Lemma 2.1.1.1) that the exact sequence
$\mathbf {pDiv}(X)\otimes \mathbb {Q}$ and we know (by Lemma 2.1.1.1) that the exact sequence
does not split.
3.3.2  $F$-isocrystals
$F$-isocrystals
Let ![]() $\mathbf {F\textrm {-}Isoc}(X)$ be the category of
$\mathbf {F\textrm {-}Isoc}(X)$ be the category of ![]() $F$-isocrystals over
$F$-isocrystals over ![]() $X$ (as defined for example in [Reference MorrowMor19, § A.1]). By [Reference KedlayaKed22, Corollary 4.2], every
$X$ (as defined for example in [Reference MorrowMor19, § A.1]). By [Reference KedlayaKed22, Corollary 4.2], every ![]() $F$-isocrystal
$F$-isocrystal ![]() $\mathcal {E}$ with constant Newton polygon admits a slope filtration
$\mathcal {E}$ with constant Newton polygon admits a slope filtration
such that ![]() $\mathcal {E}_i/\mathcal {E}_{i-1}$ is isoclinic of some slope
$\mathcal {E}_i/\mathcal {E}_{i-1}$ is isoclinic of some slope ![]() $s_i\in \mathbb {Q}$ with
$s_i\in \mathbb {Q}$ with ![]() $s_i< s_{i+1}$. By [Reference Berthelot, Breen and MessingBBM82], there is a fully faithful contravariant functor
$s_i< s_{i+1}$. By [Reference Berthelot, Breen and MessingBBM82], there is a fully faithful contravariant functor ![]() $\mathbb {D}: \mathbf {pDiv}(X)\otimes \mathbb {Q}\rightarrow \mathbf {F\textrm {-}Isoc}(X)$. Write
$\mathbb {D}: \mathbf {pDiv}(X)\otimes \mathbb {Q}\rightarrow \mathbf {F\textrm {-}Isoc}(X)$. Write
so that ![]() $\mathcal {E}$ and
$\mathcal {E}$ and ![]() $\mathcal {E}_{\mathfrak t}$ have constant Newton polygon by the preliminary reduction. Recall that the slopes appearing in an
$\mathcal {E}_{\mathfrak t}$ have constant Newton polygon by the preliminary reduction. Recall that the slopes appearing in an ![]() $F$-isocrystal associated to a
$F$-isocrystal associated to a ![]() $p$-divisible group are between
$p$-divisible group are between ![]() $0$ and
$0$ and ![]() $1$ and that a
$1$ and that a ![]() $p$-divisible group is étale if and only after applying
$p$-divisible group is étale if and only after applying ![]() $\mathbb {D}$ has constant slope
$\mathbb {D}$ has constant slope ![]() $0$. Hence,
$0$. Hence,
are the sub-![]() $F$-isocrystals of minimal slope of
$F$-isocrystals of minimal slope of ![]() $\mathcal {E}$ and
$\mathcal {E}$ and ![]() $\mathcal {E}_{\mathfrak t}$, respectively. Then the sequences (3.3.1.3) and (3.3.1.4) are sent to exact sequences
$\mathcal {E}_{\mathfrak t}$, respectively. Then the sequences (3.3.1.3) and (3.3.1.4) are sent to exact sequences
and
By fully faithfulness of ![]() $\mathbb {D}:\mathbf {pDiv}(X)\otimes \mathbb {Q}\rightarrow \mathbf {F\textrm {-}Isoc}(X)$ and the assumption, the sequence (3.3.2.2) splits and (3.3.2.1) does not split.
$\mathbb {D}:\mathbf {pDiv}(X)\otimes \mathbb {Q}\rightarrow \mathbf {F\textrm {-}Isoc}(X)$ and the assumption, the sequence (3.3.2.2) splits and (3.3.2.1) does not split.
3.3.3 Overconvergence
Let ![]() $\mathbf {F\textrm {-}Isoc}^{\dagger}(X)$ be the category of overconvergent
$\mathbf {F\textrm {-}Isoc}^{\dagger}(X)$ be the category of overconvergent ![]() $F$-isocrystals over
$F$-isocrystals over ![]() $X$ (see, for example, [Reference BerthelotBer96, Definition 2.3.6]). By [Reference BerthelotBer96, Theorem 2.4.2], every
$X$ (see, for example, [Reference BerthelotBer96, Definition 2.3.6]). By [Reference BerthelotBer96, Theorem 2.4.2], every ![]() $F$-isocrystals is convergent, hence there is a natural functor
$F$-isocrystals is convergent, hence there is a natural functor ![]() $\Phi :\mathbf {F\textrm {-}Isoc}^{\dagger}(X)\rightarrow \mathbf {F\textrm {-}Isoc}(X)$.
$\Phi :\mathbf {F\textrm {-}Isoc}^{\dagger}(X)\rightarrow \mathbf {F\textrm {-}Isoc}(X)$.
Recall that, by [Reference KedlayaKed04], the functor ![]() $\Phi :\mathbf {F\textrm {-}Isoc}^{\dagger}(X)\rightarrow \mathbf {F\textrm {-}Isoc}(X)$ is fully faithful, so that we can identify
$\Phi :\mathbf {F\textrm {-}Isoc}^{\dagger}(X)\rightarrow \mathbf {F\textrm {-}Isoc}(X)$ is fully faithful, so that we can identify ![]() $\mathbf {F\textrm {-}Isoc}^{\dagger}(X)$ with a full subcategory of
$\mathbf {F\textrm {-}Isoc}^{\dagger}(X)$ with a full subcategory of ![]() $\mathbf {F\textrm {-}Isoc}(X)$. If
$\mathbf {F\textrm {-}Isoc}(X)$. If ![]() $\mathcal {G}$ in
$\mathcal {G}$ in ![]() $\mathbf {F\textrm {-}Isoc}(X)$ is in the essential image of
$\mathbf {F\textrm {-}Isoc}(X)$ is in the essential image of ![]() $\Phi :\mathbf {F\textrm {-}Isoc}^{\dagger}(X)\rightarrow \mathbf {F\textrm {-}Isoc}(X)$ we say that it is overconvergent and we write
$\Phi :\mathbf {F\textrm {-}Isoc}^{\dagger}(X)\rightarrow \mathbf {F\textrm {-}Isoc}(X)$ we say that it is overconvergent and we write ![]() $\mathcal {G}^{{\dagger} }$ for its (unique) overconvergent extension. By [Reference ÉtesseÉte02],
$\mathcal {G}^{{\dagger} }$ for its (unique) overconvergent extension. By [Reference ÉtesseÉte02], ![]() $\mathcal {E}$ is overconvergent. As a consequence of Proposition 4.1.2, that will be proved in § 4, and the geometric interpretation of
$\mathcal {E}$ is overconvergent. As a consequence of Proposition 4.1.2, that will be proved in § 4, and the geometric interpretation of ![]() $\mathcal {E}_{\mathfrak t}$ given in § 2.2.4, we can show that
$\mathcal {E}_{\mathfrak t}$ given in § 2.2.4, we can show that ![]() $\mathcal {E}_{\mathfrak t}$ is also overconvergent.
$\mathcal {E}_{\mathfrak t}$ is also overconvergent.
Proposition 3.3.3.1 The ![]() $F$-isocrystal
$F$-isocrystal ![]() $\mathcal {E}_{\mathfrak t}$ is overconvergent.
$\mathcal {E}_{\mathfrak t}$ is overconvergent.
Proof. Since inside ![]() $\mathrm {Ext}^1_{\mathbf {F\textrm {-}Isoc}(X)}(\mathcal {E}, \mathcal {O}^{\mathrm {crys}}_X)$ the class of
$\mathrm {Ext}^1_{\mathbf {F\textrm {-}Isoc}(X)}(\mathcal {E}, \mathcal {O}^{\mathrm {crys}}_X)$ the class of ![]() $\mathcal {E}_{\mathfrak t}$ is a
$\mathcal {E}_{\mathfrak t}$ is a ![]() $\mathbb {Q}_p$-linear combination of classes
$\mathbb {Q}_p$-linear combination of classes ![]() $\mathcal {E}_{\mathfrak v}$ with
$\mathcal {E}_{\mathfrak v}$ with ![]() $\mathfrak v\in \mathcal {A}(X)$ and the morphism
$\mathfrak v\in \mathcal {A}(X)$ and the morphism ![]() $\mathrm {Ext}^1_{\mathbf {F\textrm {-}Isoc}^{\dagger}(X)}(\mathcal {E}^{{\dagger} }, \mathcal {O}^{{\dagger} }_X)\rightarrow \mathrm {Ext}^1_{\mathbf {F\textrm {-}Isoc}(X)}(\mathcal {E}, \mathcal {O}^{\mathrm {crys}}_X)$ is
$\mathrm {Ext}^1_{\mathbf {F\textrm {-}Isoc}^{\dagger}(X)}(\mathcal {E}^{{\dagger} }, \mathcal {O}^{{\dagger} }_X)\rightarrow \mathrm {Ext}^1_{\mathbf {F\textrm {-}Isoc}(X)}(\mathcal {E}, \mathcal {O}^{\mathrm {crys}}_X)$ is ![]() $\mathbb {Q}_p$-linear, we can assume that
$\mathbb {Q}_p$-linear, we can assume that ![]() $\mathfrak t\in \mathcal {A}(X)$. It is then enough to show that
$\mathfrak t\in \mathcal {A}(X)$. It is then enough to show that ![]() $\mathcal {E}^{\vee }_{\mathfrak t}(1)$ (where
$\mathcal {E}^{\vee }_{\mathfrak t}(1)$ (where ![]() $(-)^{\vee }$ is the dual
$(-)^{\vee }$ is the dual ![]() $F$-isocrystals and
$F$-isocrystals and ![]() $(-)(1)$ is the Tate twist) is overconvergent. By § 2.2.4 and the compatibility of the functor
$(-)(1)$ is the Tate twist) is overconvergent. By § 2.2.4 and the compatibility of the functor ![]() $\mathbb {D}$ with dualities ([Reference Berthelot, Breen and MessingBBM82, (5.3.3.1)]), one has that
$\mathbb {D}$ with dualities ([Reference Berthelot, Breen and MessingBBM82, (5.3.3.1)]), one has that ![]() $\mathcal {E}^{\vee }_{\mathfrak t}(1)$ identifies with
$\mathcal {E}^{\vee }_{\mathfrak t}(1)$ identifies with ![]() $\mathbb {D}(G[p^{\infty }])$, where
$\mathbb {D}(G[p^{\infty }])$, where ![]() $G[p^{\infty }]$ is the
$G[p^{\infty }]$ is the ![]() $p$-divisible group of an algebraic group
$p$-divisible group of an algebraic group ![]() $G$ which is an extension
$G$ which is an extension
of an abelian variety and a ![]() $\mathbb {G}_m$. Then the overconvergence of
$\mathbb {G}_m$. Then the overconvergence of ![]() $\mathcal {E}^{\vee }_{\mathfrak t}(1)$ follows from Proposition 4.1.2, that we will prove in § 4.
$\mathcal {E}^{\vee }_{\mathfrak t}(1)$ follows from Proposition 4.1.2, that we will prove in § 4.
Since ![]() $\mathcal {E}$ and
$\mathcal {E}$ and ![]() $\mathcal {E}_{\mathfrak t}$ are overconvergent and the functor
$\mathcal {E}_{\mathfrak t}$ are overconvergent and the functor ![]() $\Phi :\mathbf {F\textrm {-}Isoc}^{\dagger}(X)\rightarrow \mathbf {F\textrm {-}Isoc}(X)$ is fully faithful, the non-split exact sequence (3.3.2.1) lifts to a non-split exact sequence
$\Phi :\mathbf {F\textrm {-}Isoc}^{\dagger}(X)\rightarrow \mathbf {F\textrm {-}Isoc}(X)$ is fully faithful, the non-split exact sequence (3.3.2.1) lifts to a non-split exact sequence
On the other hand, by construction, the exact sequence (3.3.2.2) is obtained by applying ![]() $\Phi :\mathbf {F\textrm {-}Isoc}^{\dagger}(X)\rightarrow \mathbf {F\textrm {-}Isoc}(X)$ to (3.3.3.2) and then base changing it along
$\Phi :\mathbf {F\textrm {-}Isoc}^{\dagger}(X)\rightarrow \mathbf {F\textrm {-}Isoc}(X)$ to (3.3.3.2) and then base changing it along ![]() $\mathcal {E}_1\rightarrow \mathcal {E}$.
$\mathcal {E}_1\rightarrow \mathcal {E}$.
3.3.4 Minimal slope conjecture
Chose a splitting ![]() $s:\mathcal {E}_1\rightarrow \mathcal {E}_{\mathfrak t,1}$ of the sequence (3.3.2.2). Consider the smallest overconvergent object
$s:\mathcal {E}_1\rightarrow \mathcal {E}_{\mathfrak t,1}$ of the sequence (3.3.2.2). Consider the smallest overconvergent object ![]() $\widetilde {\mathcal {E}}^{{\dagger} }$ contained in
$\widetilde {\mathcal {E}}^{{\dagger} }$ contained in ![]() $\mathcal {E}^{{\dagger} }_{\mathfrak t}$ and containing
$\mathcal {E}^{{\dagger} }_{\mathfrak t}$ and containing ![]() $s(\mathcal {E}_1)$.
$s(\mathcal {E}_1)$.
Since ![]() $p(A)>0$, we have
$p(A)>0$, we have ![]() $\widetilde {\mathcal {E}}^{{\dagger} }\neq 0$. By the recent work [Reference TsuzukiTsu23] and its improvement done in [Reference D'AddezioD'A23, Theorem 4.1.3], one has
$\widetilde {\mathcal {E}}^{{\dagger} }\neq 0$. By the recent work [Reference TsuzukiTsu23] and its improvement done in [Reference D'AddezioD'A23, Theorem 4.1.3], one has ![]() $s(\mathcal {E}_1)=\widetilde {\mathcal {E}}_1$ so that
$s(\mathcal {E}_1)=\widetilde {\mathcal {E}}_1$ so that ![]() $\widetilde {\mathcal {E}}^{{\dagger} }\cap \mathcal {O}^{\mathrm {crys}}_{X}=0$. Hence, the natural composite map
$\widetilde {\mathcal {E}}^{{\dagger} }\cap \mathcal {O}^{\mathrm {crys}}_{X}=0$. Hence, the natural composite map
is injective and it induces an isomorphism ![]() $\pi : \widetilde {\mathcal {E}}^{{\dagger} }\xrightarrow {\simeq } \pi (\widetilde {\mathcal {E}}^{{\dagger} })$. By construction, the sequence (3.3.3.2) splits after base change along
$\pi : \widetilde {\mathcal {E}}^{{\dagger} }\xrightarrow {\simeq } \pi (\widetilde {\mathcal {E}}^{{\dagger} })$. By construction, the sequence (3.3.3.2) splits after base change along ![]() $\pi (\widetilde {\mathcal {E}}^{{\dagger} })\subseteq \mathcal {E}^{{\dagger} }$.
$\pi (\widetilde {\mathcal {E}}^{{\dagger} })\subseteq \mathcal {E}^{{\dagger} }$.
Since the sequence (3.3.3.2) does not split, ![]() $\pi (\widetilde {\mathcal {E}}^{{\dagger} })\neq \mathcal {E}^{{\dagger} }$. By a result of Pál [Reference PálPál22, Theorem 1.2], the overconvergent
$\pi (\widetilde {\mathcal {E}}^{{\dagger} })\neq \mathcal {E}^{{\dagger} }$. By a result of Pál [Reference PálPál22, Theorem 1.2], the overconvergent ![]() $F$-isocrystal
$F$-isocrystal ![]() $\mathcal {E}^{{\dagger} }$ is semisimple, hence there is a projection
$\mathcal {E}^{{\dagger} }$ is semisimple, hence there is a projection ![]() $\widetilde e:\mathcal {E}^{{\dagger} }\rightarrow \mathcal {E}^{{\dagger} }$ onto
$\widetilde e:\mathcal {E}^{{\dagger} }\rightarrow \mathcal {E}^{{\dagger} }$ onto ![]() $\pi (\widetilde {\mathcal {E}}^{{\dagger} })$. Since
$\pi (\widetilde {\mathcal {E}}^{{\dagger} })$. Since ![]() $\widetilde {\mathcal {E}}$ contains
$\widetilde {\mathcal {E}}$ contains ![]() $s(\mathcal {E}_1)$, the non-zero idempotent
$s(\mathcal {E}_1)$, the non-zero idempotent ![]() $1-\widetilde e$ acts as zero on
$1-\widetilde e$ acts as zero on ![]() $\mathcal {E}_1$. By the faithfulness of the composite functor
$\mathcal {E}_1$. By the faithfulness of the composite functor ![]() $\mathbf {F\textrm {-}Isoc}^{\dagger}(X)\xrightarrow {\Phi } \mathbf {F\textrm {-}Isoc}(X)\xrightarrow {\mathbb {D}} \mathbf {pDiv}(X)\otimes \mathbb {Q}$, we get a non-zero idempotent
$\mathbf {F\textrm {-}Isoc}^{\dagger}(X)\xrightarrow {\Phi } \mathbf {F\textrm {-}Isoc}(X)\xrightarrow {\mathbb {D}} \mathbf {pDiv}(X)\otimes \mathbb {Q}$, we get a non-zero idempotent ![]() $e[p^{\infty }]$ in
$e[p^{\infty }]$ in ![]() $\mathrm {End}(A[p^{\infty }])\otimes \mathbb {Q}_p$ acting as zero on
$\mathrm {End}(A[p^{\infty }])\otimes \mathbb {Q}_p$ acting as zero on ![]() $A[p^{\infty }]^{ \unicode{x00E9}{\textrm{t}}}$. Observe that the composite map
$A[p^{\infty }]^{ \unicode{x00E9}{\textrm{t}}}$. Observe that the composite map
is a projection onto ![]() $\widetilde {\mathcal {E}}^{{\dagger} }$. Hence, there exists a non-zero idempotent
$\widetilde {\mathcal {E}}^{{\dagger} }$. Hence, there exists a non-zero idempotent ![]() $e\in \mathrm {End}(\mathcal {E}_{\mathfrak t}^{{\dagger} })\simeq \mathrm {End}_{\mathbf {pDiv}(K)}(M_x[p^{\infty }])\otimes \mathbb {Q}_p$ which induces the non-zero idempotent in
$e\in \mathrm {End}(\mathcal {E}_{\mathfrak t}^{{\dagger} })\simeq \mathrm {End}_{\mathbf {pDiv}(K)}(M_x[p^{\infty }])\otimes \mathbb {Q}_p$ which induces the non-zero idempotent in ![]() $e[p^{\infty }]\in \mathrm {End}(A[p^{\infty }])\otimes \mathbb {Q}_p$ acting as
$e[p^{\infty }]\in \mathrm {End}(A[p^{\infty }])\otimes \mathbb {Q}_p$ acting as ![]() $0$ on
$0$ on ![]() $A_x[p^{\infty }]^{ \unicode{x00E9}{\textrm{t}}}$. This concludes the proof of Proposition 3.2.3.1.
$A_x[p^{\infty }]^{ \unicode{x00E9}{\textrm{t}}}$. This concludes the proof of Proposition 3.2.3.1.
3.4 Geometric variant
Write ![]() $L:=\overline kK\subseteq \overline K$ for the field generated by
$L:=\overline kK\subseteq \overline K$ for the field generated by ![]() $\overline k$ and
$\overline k$ and ![]() $K$ in
$K$ in ![]() $\overline K$. Let
$\overline K$. Let ![]() $\mathrm {Tr}_{\overline K/\overline k}(A)$ be the
$\mathrm {Tr}_{\overline K/\overline k}(A)$ be the ![]() $(\overline K/\overline k)$-trace of
$(\overline K/\overline k)$-trace of ![]() $A_{\overline K}$ (i.e. the biggest
$A_{\overline K}$ (i.e. the biggest ![]() $\overline k$-isotrivial quotient
$\overline k$-isotrivial quotient ![]() $A_{\overline K}\rightarrow \mathrm {Tr}_{\overline K/\overline k}(A)$ of
$A_{\overline K}\rightarrow \mathrm {Tr}_{\overline K/\overline k}(A)$ of ![]() $A_{\overline K}$). A modification of the previous arguments gives us the following geometric variant.
$A_{\overline K}$). A modification of the previous arguments gives us the following geometric variant.
Theorem 3.4.1 Assume that ![]() $r(A)^{\mathrm {min}}>0$. Then:
$r(A)^{\mathrm {min}}>0$. Then:
(i) if
 $\mathrm {Tr}_{\overline K/\overline k}(A)=0$, then
$\mathrm {Tr}_{\overline K/\overline k}(A)=0$, then  $A(L^{\mathrm {perf}})$ is not finitely generated if and only if there exists an idempotent
$A(L^{\mathrm {perf}})$ is not finitely generated if and only if there exists an idempotent  $0\neq e\in \mathrm {End}(A_{L})\otimes \mathbb {Q}_p$ such that
$0\neq e\in \mathrm {End}(A_{L})\otimes \mathbb {Q}_p$ such that  $0=e[p^{\infty }]^{ \unicode{x00E9}{\textrm{t}}}\in \mathrm {End}(A_L[p^{\infty }]^{ \unicode{x00E9}{\textrm{t}}})\otimes \mathbb {Q}_p$;
$0=e[p^{\infty }]^{ \unicode{x00E9}{\textrm{t}}}\in \mathrm {End}(A_L[p^{\infty }]^{ \unicode{x00E9}{\textrm{t}}})\otimes \mathbb {Q}_p$;(ii)
 $A(L^{\mathrm {perf}})_{p^{\infty }}\subseteq A(L^{\mathrm {perf}})_{\mathrm {tors}}$ if and only if
$A(L^{\mathrm {perf}})_{p^{\infty }}\subseteq A(L^{\mathrm {perf}})_{\mathrm {tors}}$ if and only if  $p(A)^{\mathrm {min}}>0$.
$p(A)^{\mathrm {min}}>0$.
Proof. Since ![]() $A(L)_{\mathrm {tf}}$ is finitely generated by the Lang–Néron theorem and the action of
$A(L)_{\mathrm {tf}}$ is finitely generated by the Lang–Néron theorem and the action of ![]() $\pi _1(K)$ on
$\pi _1(K)$ on ![]() $\mathrm {End}(A)$ factors through a finite quotient, there exists a finite extension
$\mathrm {End}(A)$ factors through a finite quotient, there exists a finite extension ![]() $K\subseteq K'\subseteq L$ such that
$K\subseteq K'\subseteq L$ such that ![]() $A(K')\otimes \mathbb {Q}=A(L)\otimes \mathbb {Q}$ and
$A(K')\otimes \mathbb {Q}=A(L)\otimes \mathbb {Q}$ and ![]() $\mathrm {End}(A_{K'})=\mathrm {End}(A_L)$. For
$\mathrm {End}(A_{K'})=\mathrm {End}(A_L)$. For ![]() $?\in \{\emptyset, p\}$ we consider the following commutative diagrams.
$?\in \{\emptyset, p\}$ we consider the following commutative diagrams.

Moreover, if ![]() $\mathrm {Tr}(A)=0$ then
$\mathrm {Tr}(A)=0$ then ![]() $A(L^{\mathrm {perf}})_{\mathrm {tors}}$ is finite by [Reference Ambrosi and D'AddezioAD22]. Since
$A(L^{\mathrm {perf}})_{\mathrm {tors}}$ is finite by [Reference Ambrosi and D'AddezioAD22]. Since ![]() $A(L)_{\mathrm {tf}}$ is finitely generated, by Corollary 2.2.2.3 and Theorem 3.1.2 it is enough to show that
$A(L)_{\mathrm {tf}}$ is finitely generated, by Corollary 2.2.2.3 and Theorem 3.1.2 it is enough to show that ![]() $\phi$ is injective. Since
$\phi$ is injective. Since ![]() $\pi _1(L)\subseteq \pi _1(K')$ is an normal subgroup, the Hochschild–Serre spectral sequence gives us an exact sequence
$\pi _1(L)\subseteq \pi _1(K')$ is an normal subgroup, the Hochschild–Serre spectral sequence gives us an exact sequence
Since ![]() $\pi _1(K')/\pi _1(L)$ is pro-cyclic, one has
$\pi _1(K')/\pi _1(L)$ is pro-cyclic, one has
where the last terms are the coinvariants. However, since ![]() $A(K^{\mathrm {perf}})[p^{\infty }]$ is finite, one has
$A(K^{\mathrm {perf}})[p^{\infty }]$ is finite, one has
and this concludes the proof.
4. Overconvergence
4.1 Statement
Let ![]() $X$ be a smooth geometrically connected variety over a finite field
$X$ be a smooth geometrically connected variety over a finite field ![]() $k$ of characteristic
$k$ of characteristic ![]() $p$ and let
$p$ and let
be an extension of an abelian ![]() $X$-scheme
$X$-scheme ![]() $A$ by a torus
$A$ by a torus ![]() $W$ over
$W$ over ![]() $X$. By applying the Dieudonné functor
$X$. By applying the Dieudonné functor ![]() $\mathbb {D}:\mathbf {pDiv}(X)\otimes \mathbb {Q}\rightarrow \mathbf {F\textrm {-}Isoc}(X)$ to the exact sequence
$\mathbb {D}:\mathbf {pDiv}(X)\otimes \mathbb {Q}\rightarrow \mathbf {F\textrm {-}Isoc}(X)$ to the exact sequence ![]() $0\rightarrow W[p^{\infty }]\rightarrow G[p^{\infty }]\rightarrow A[p^{\infty }]\rightarrow 0$, we get an exact sequence
$0\rightarrow W[p^{\infty }]\rightarrow G[p^{\infty }]\rightarrow A[p^{\infty }]\rightarrow 0$, we get an exact sequence
The main result of this section is the following.
Proposition 4.1.2 The ![]() $F$-isocrystal
$F$-isocrystal ![]() $\mathbb {D}(G[p^{\infty }])$ is overconvergent.
$\mathbb {D}(G[p^{\infty }])$ is overconvergent.
To prove Proposition 4.1.2, we reduce to the case in which ![]() $X$ is a curve and the abelian scheme has everywhere semistable reduction. Then, in § 4.3, we use a result of Trihan [Reference TrihanTri08] to reduce to prove a semistability result for
$X$ is a curve and the abelian scheme has everywhere semistable reduction. Then, in § 4.3, we use a result of Trihan [Reference TrihanTri08] to reduce to prove a semistability result for ![]() $G[p^{\infty }]$. We conclude the proof in §§ 4.4 and 4.5, proving this semistability.
$G[p^{\infty }]$. We conclude the proof in §§ 4.4 and 4.5, proving this semistability.
4.2 Preliminary reductions
By [Reference Grub, Kedlaya and UptonGKU21, Lemma 4.2], to prove overconvergence, we can freely replace ![]() $X$ with a smooth variety
$X$ with a smooth variety ![]() $Y$ admitting a dominant morphism
$Y$ admitting a dominant morphism ![]() $Y\rightarrow X$. Thus, we can assume that
$Y\rightarrow X$. Thus, we can assume that ![]() $W\simeq \mathbb {G}_{m,X}^m$ and that
$W\simeq \mathbb {G}_{m,X}^m$ and that ![]() $A(X)[n]\simeq (\mathbb {Z}/n\mathbb {Z})^{2g}$ for some fixed
$A(X)[n]\simeq (\mathbb {Z}/n\mathbb {Z})^{2g}$ for some fixed ![]() $n\geq 3$ coprime with
$n\geq 3$ coprime with ![]() $p$. By [Reference Deligne and KatzDK73, Proposition 4.7, Exposé IX, p. 48], this last condition implies that, for every smooth curve
$p$. By [Reference Deligne and KatzDK73, Proposition 4.7, Exposé IX, p. 48], this last condition implies that, for every smooth curve ![]() $C$ and every morphism
$C$ and every morphism ![]() $C\rightarrow X$, the abelian scheme
$C\rightarrow X$, the abelian scheme ![]() $A\times _X C$ has everywhere semistable reduction. Moreover, by de Jong's alteration theorem [Reference de JongdJ98], we can assume that
$A\times _X C$ has everywhere semistable reduction. Moreover, by de Jong's alteration theorem [Reference de JongdJ98], we can assume that ![]() $X$ admits a compactification whose complementary is a normal crossing divisor. In this situation, by [Reference de JongdJ98, 2.5] and [Reference TrihanTri08, Corollary 3.14], for every smooth curve
$X$ admits a compactification whose complementary is a normal crossing divisor. In this situation, by [Reference de JongdJ98, 2.5] and [Reference TrihanTri08, Corollary 3.14], for every smooth curve ![]() $C$ and every morphism
$C$ and every morphism ![]() $f:C\rightarrow X$, the
$f:C\rightarrow X$, the ![]() $F$-isocrystals
$F$-isocrystals ![]() $f^*\mathbb {D}(A[p^{\infty }])\simeq \mathbb {D}(A\times _XC[p^{\infty }])$ has everywhere semistable reduction. Therefore, we can apply the cut by curve criterion for overconvergence proved in [Reference Grub, Kedlaya and UptonGKU21, Lemma 6.7] to reduce to the case in which
$f^*\mathbb {D}(A[p^{\infty }])\simeq \mathbb {D}(A\times _XC[p^{\infty }])$ has everywhere semistable reduction. Therefore, we can apply the cut by curve criterion for overconvergence proved in [Reference Grub, Kedlaya and UptonGKU21, Lemma 6.7] to reduce to the case in which ![]() $X$ is a curve. Thus, from now we assume that
$X$ is a curve. Thus, from now we assume that ![]() $X$ is a curve with smooth compactification
$X$ is a curve with smooth compactification ![]() $\overline X$ and
$\overline X$ and ![]() $A$ has every everywhere semistable reduction.
$A$ has every everywhere semistable reduction.
4.3 Passing to  $p$-divisible groups
$p$-divisible groups
For every ![]() $x\in \overline X-X$ we let
$x\in \overline X-X$ we let ![]() $S_x$ be the spectrum of the completion of
$S_x$ be the spectrum of the completion of ![]() $\overline X$ in
$\overline X$ in ![]() $x$ and
$x$ and ![]() $\eta _x$ the generic point of
$\eta _x$ the generic point of ![]() $S_x$. Write
$S_x$. Write ![]() $A_{\eta _x}$ and
$A_{\eta _x}$ and ![]() $G_{\eta _x}$ for the base change of
$G_{\eta _x}$ for the base change of ![]() $A\rightarrow X$ and
$A\rightarrow X$ and ![]() $G \rightarrow X$ trough
$G \rightarrow X$ trough ![]() $\eta _x \rightarrow X$. By [Reference TrihanTri08, Theorem 4.5], to prove Proposition 4.1.2, it is enough to show that for every
$\eta _x \rightarrow X$. By [Reference TrihanTri08, Theorem 4.5], to prove Proposition 4.1.2, it is enough to show that for every ![]() $x\in \overline X-X$, the
$x\in \overline X-X$, the ![]() $p$-divisible group
$p$-divisible group ![]() $G_{\eta _x}[p^{\infty }]$ is semistable, i.e. that there exists a filtration
$G_{\eta _x}[p^{\infty }]$ is semistable, i.e. that there exists a filtration
such that:
(i)
 $G_{\eta _x}[p^{\infty }]^{\mathrm {f}}$ and
$G_{\eta _x}[p^{\infty }]^{\mathrm {f}}$ and  $G_{\eta _x}[p^{\infty }]/G_{\eta _x}[p^{\infty }]^{\mathrm {t}}$ extend to
$G_{\eta _x}[p^{\infty }]/G_{\eta _x}[p^{\infty }]^{\mathrm {t}}$ extend to  $p$-divisible groups
$p$-divisible groups  $G[p^{\infty }]_{x,1}$ and
$G[p^{\infty }]_{x,1}$ and  $G[p^{\infty }]_{x,2}$ over
$G[p^{\infty }]_{x,2}$ over  $S_x$; in this case, by [Reference de JongdJ98], the natural map
$S_x$; in this case, by [Reference de JongdJ98], the natural map  $G_{\eta _x}[p^{\infty }]^{\mathrm {f}}\rightarrow G_{\eta _x}[p^{\infty }]/G_{\eta _x}[p^{\infty }]^{\mathrm {t}}$ extends to a map
$G_{\eta _x}[p^{\infty }]^{\mathrm {f}}\rightarrow G_{\eta _x}[p^{\infty }]/G_{\eta _x}[p^{\infty }]^{\mathrm {t}}$ extends to a map  $G[p^{\infty }]_{x,1}\rightarrow G[p^{\infty }]_{x,2}$;
$G[p^{\infty }]_{x,1}\rightarrow G[p^{\infty }]_{x,2}$;(ii)
 $\mathrm {Ker}(G[p^{\infty }]_{x,1}\rightarrow G[p^{\infty }]_{x,2})$ is a multiplicative
$\mathrm {Ker}(G[p^{\infty }]_{x,1}\rightarrow G[p^{\infty }]_{x,2})$ is a multiplicative  $p$-divisible group and
$p$-divisible group and  $\mathrm {Coker}(G[p^{\infty }]_{x,1}\rightarrow G[p^{\infty }]_{x,2})$ is an étale
$\mathrm {Coker}(G[p^{\infty }]_{x,1}\rightarrow G[p^{\infty }]_{x,2})$ is an étale  $p$-divisible group.
$p$-divisible group.
Since the situation is now entirely local, we drop the subscript ![]() $x$ from the notation.
$x$ from the notation.
4.4 Construction of the filtration
By [Reference Bosch, Lutkebohmert and RaynaudBLR90, Proposition 7, p. 292] and its proof, there exists an exact sequence of smooth group ![]() $S$-schemes with connected fibers
$S$-schemes with connected fibers
where ![]() $\mathcal {A}\rightarrow S$ is the Néron model of
$\mathcal {A}\rightarrow S$ is the Néron model of ![]() $A_\eta$ and
$A_\eta$ and ![]() $\mathcal {A}^0\rightarrow S$ is its connected component of the identity, having as a generic fiber the sequence
$\mathcal {A}^0\rightarrow S$ is its connected component of the identity, having as a generic fiber the sequence
Since ![]() $A_{\eta }$ has semistable reduction, the special fiber
$A_{\eta }$ has semistable reduction, the special fiber ![]() $\mathcal {A}^0_s$ fits into an exact sequence
$\mathcal {A}^0_s$ fits into an exact sequence
with ![]() $T$ a
$T$ a ![]() $k$-torus and
$k$-torus and ![]() $B$ a
$B$ a ![]() $k$-abelian variety. Let
$k$-abelian variety. Let ![]() $\mathcal {A}^0[p^n]^{\mathrm {f}}\subseteq \mathcal {A}^0[p^n]$ be the maximal subgroup which is finite over
$\mathcal {A}^0[p^n]^{\mathrm {f}}\subseteq \mathcal {A}^0[p^n]$ be the maximal subgroup which is finite over ![]() $S$ and
$S$ and ![]() $\mathcal {A}^0[p^n]^{\mathrm {t}}\subseteq \mathcal {A}^0[p^n]^{\mathrm {f}}$ be the unique lifting of the finite subgroup
$\mathcal {A}^0[p^n]^{\mathrm {t}}\subseteq \mathcal {A}^0[p^n]^{\mathrm {f}}$ be the unique lifting of the finite subgroup ![]() $T[p^n]\subseteq \mathcal {A}^0_{s}[p^n]$ to
$T[p^n]\subseteq \mathcal {A}^0_{s}[p^n]$ to ![]() $\mathcal {A}^0[p^n]^{\mathrm {f}}$. For
$\mathcal {A}^0[p^n]^{\mathrm {f}}$. For ![]() $?\in \{\mathrm {t},\mathrm {f}\}$, we define
$?\in \{\mathrm {t},\mathrm {f}\}$, we define ![]() $\mathcal {G}^0[p^n]^{?}\subseteq \mathcal {G}^0[p^n]$ via the following cartesian diagram with exact rows.
$\mathcal {G}^0[p^n]^{?}\subseteq \mathcal {G}^0[p^n]$ via the following cartesian diagram with exact rows.

Taking the direct limit with ![]() $n$ and applying [Reference Deligne and KatzDK73, Proposition 5.6, Exposé IX, p. 180] and [Reference MessingMes72, (2.4.3)], we get a filtration
$n$ and applying [Reference Deligne and KatzDK73, Proposition 5.6, Exposé IX, p. 180] and [Reference MessingMes72, (2.4.3)], we get a filtration ![]() $\mathcal {G}^0[p^{\infty }]^{\mathrm {t}}\subseteq \mathcal {G}^0[p^{\infty }]^{\mathrm {f}}\subseteq \mathcal {G}^0[p^{\infty }],$ of
$\mathcal {G}^0[p^{\infty }]^{\mathrm {t}}\subseteq \mathcal {G}^0[p^{\infty }]^{\mathrm {f}}\subseteq \mathcal {G}^0[p^{\infty }],$ of ![]() $p$-divisible groups. Set
$p$-divisible groups. Set
so that there are filtrations
4.5 End of the proof
By [Reference Deligne and KatzDK73, Exposé IX], the inclusions ![]() $A[p^{\infty }]_{\eta }^{\mathrm {t}}\subseteq A[p^{\infty }]_{\eta }^{\mathrm {f}}\subseteq A_{\eta }[p^{\infty }]$ produce a filtration of
$A[p^{\infty }]_{\eta }^{\mathrm {t}}\subseteq A[p^{\infty }]_{\eta }^{\mathrm {f}}\subseteq A_{\eta }[p^{\infty }]$ produce a filtration of ![]() $A[p^{\infty }]_{\eta }$ giving semistable reduction for
$A[p^{\infty }]_{\eta }$ giving semistable reduction for ![]() $A_{\eta }[p^{\infty }]$, in the sense that:
$A_{\eta }[p^{\infty }]$, in the sense that:
(i)
 $A[p^{\infty }]_{\eta }^{\mathrm {f}}$ and
$A[p^{\infty }]_{\eta }^{\mathrm {f}}$ and  $A_{\eta }[p^{\infty }]/A[p^{\infty }]^{\mathrm {t}}_{\eta }$ extend to
$A_{\eta }[p^{\infty }]/A[p^{\infty }]^{\mathrm {t}}_{\eta }$ extend to  $p$-divisible groups
$p$-divisible groups  $A[p^{\infty }]_{1}$ and
$A[p^{\infty }]_{1}$ and  $A[p^{\infty }]_{2}$ over
$A[p^{\infty }]_{2}$ over  $S$ (see [Reference Deligne and KatzDK73, Proposition 5.6, p. 380, Exposé IX]);
$S$ (see [Reference Deligne and KatzDK73, Proposition 5.6, p. 380, Exposé IX]);(ii) if
 $f_A:A[p^{\infty }]_{1}\rightarrow A[p^{\infty }]_{2}$ denotes the natural induced map, then
$f_A:A[p^{\infty }]_{1}\rightarrow A[p^{\infty }]_{2}$ denotes the natural induced map, then  $\mathrm {Ker}(f_A)$ is a multiplicative
$\mathrm {Ker}(f_A)$ is a multiplicative  $p$-divisible group and
$p$-divisible group and  $\mathrm {Coker}(f_A)$ is an étale
$\mathrm {Coker}(f_A)$ is an étale  $p$-divisible group (this follows from the orthogonality theorem [Reference Deligne and KatzDK73, Proposition 5.2, p. 372, Exposé IX], which implies that
$p$-divisible group (this follows from the orthogonality theorem [Reference Deligne and KatzDK73, Proposition 5.2, p. 372, Exposé IX], which implies that  $\mathrm {Ker}(f_A)\simeq \mathcal {A}^0[p^{\infty }]^{\mathrm {t}}$ and
$\mathrm {Ker}(f_A)\simeq \mathcal {A}^0[p^{\infty }]^{\mathrm {t}}$ and  $\mathrm {Coker}(f_A)\simeq (\mathcal (\mathcal {A}^{\vee })^0[p^{\infty }]^{\mathrm {t}})^{\vee }$, where
$\mathrm {Coker}(f_A)\simeq (\mathcal (\mathcal {A}^{\vee })^0[p^{\infty }]^{\mathrm {t}})^{\vee }$, where  $\mathcal {A}^{\vee }$ is Néron-model of the dual abelian
$\mathcal {A}^{\vee }$ is Néron-model of the dual abelian  $A_\eta ^\vee$ and
$A_\eta ^\vee$ and  $(\mathcal {A}^{\vee }[p^{\infty }]^{\mathrm {t}})^{\vee }$ is the Cartier dual of
$(\mathcal {A}^{\vee }[p^{\infty }]^{\mathrm {t}})^{\vee }$ is the Cartier dual of  $\mathcal {A}^{\vee }[p^{\infty }]^{\mathrm {t}}$).
$\mathcal {A}^{\vee }[p^{\infty }]^{\mathrm {t}}$).
To conclude the proof we now deduce for (i) and (ii) above that the same properties holds for the filtration ![]() $G[p^{\infty }]_{\eta }^{\mathrm {t}}\subseteq G[p^{\infty }]_{\eta }^{\mathrm {f}}\subseteq G_{\eta }[p^{\infty }]$.
$G[p^{\infty }]_{\eta }^{\mathrm {t}}\subseteq G[p^{\infty }]_{\eta }^{\mathrm {f}}\subseteq G_{\eta }[p^{\infty }]$.
(i) By construction,
 $G[p^{\infty }]_{\eta }^{\mathrm {f}}$ extends over
$G[p^{\infty }]_{\eta }^{\mathrm {f}}$ extends over  $S$ to the
$S$ to the  $p$-divisible group
$p$-divisible group  $G[p^{\infty }]_1:=\mathcal {G}^0[p^{\infty }]^{\mathrm {f}}$. On the other hand, the diagram (4.4.1) shows that
$G[p^{\infty }]_1:=\mathcal {G}^0[p^{\infty }]^{\mathrm {f}}$. On the other hand, the diagram (4.4.1) shows that  $G_{\eta }[p^{\infty }]/G[p^{\infty }]_{\eta }^{\mathrm {t}}\simeq A_{\eta }[p^{\infty }]/A[p^{\infty }]_{\eta }^{\mathrm {t}}$, so that we can set
$G_{\eta }[p^{\infty }]/G[p^{\infty }]_{\eta }^{\mathrm {t}}\simeq A_{\eta }[p^{\infty }]/A[p^{\infty }]_{\eta }^{\mathrm {t}}$, so that we can set  $G[p^{\infty }]_2:=A[p^{\infty }]_2$.
$G[p^{\infty }]_2:=A[p^{\infty }]_2$.(ii) Let
 $f:G[p^{\infty }]_1\rightarrow G[p^{\infty }]_2$ be the induced morphism. We are left to prove that
$f:G[p^{\infty }]_1\rightarrow G[p^{\infty }]_2$ be the induced morphism. We are left to prove that  $\mathrm {Ker}(f)$ and
$\mathrm {Ker}(f)$ and  $\mathrm {Coker}(f)$ are
$\mathrm {Coker}(f)$ are  $p$-divisible groups and
$p$-divisible groups and  $\mathrm {Ker}(f)$ is multiplicative and
$\mathrm {Ker}(f)$ is multiplicative and  $\mathrm {Coker}(f)$ is étale. This can be deduced from the analogues properties for
$\mathrm {Coker}(f)$ is étale. This can be deduced from the analogues properties for  $A[p^{\infty }]_1$ and
$A[p^{\infty }]_1$ and  $A[p^{\infty }]_2$, thanks to the following commutative diagram with exact rows and columns.
$A[p^{\infty }]_2$, thanks to the following commutative diagram with exact rows and columns.

Conflicts of Interest
None.