Published online by Cambridge University Press: 15 November 2021
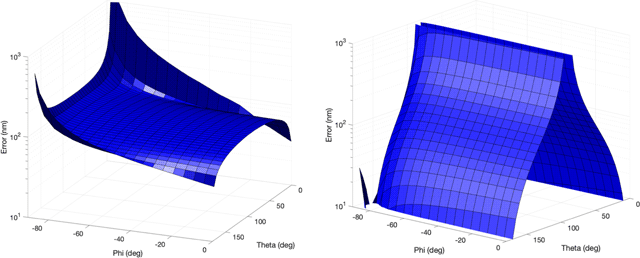
As the feature size of crystalline materials gets smaller, the ability to correctly interpret geometrical sample information from electron backscatter diffraction (EBSD) data becomes more important. This paper uses the notion of transition curves, associated with line scans across grain boundaries (GBs), to correctly account for the finite size of the excitation volume (EV) in the determination of the geometry of the boundary. Various metrics arising from the EBSD data are compared to determine the best experimental proxy for actual numbers of backscattered electrons that are tracked in a Monte Carlo simulation. Consideration of the resultant curves provides an accurate method of determining GB position (at the sample surface) and indicates a significant potential for error in determining GB position using standard EBSD software. Subsequently, simple criteria for comparing experimental and simulated transition curves are derived. Finally, it is shown that the EV is too shallow for the curves to reveal subsurface geometry of the GB (i.e., GB inclination angle) for most values of GB inclination.