Note on the generalized Mehler transform
Published online by Cambridge University Press: 24 October 2008
Extract
1. The integral transform. One result of recent studies of boundary-value problems of the wave and diffusion equations involving wedge- or conically-shaped boundaries has been the interest shown in integrals in which the variable of integration appears as the order of Bessel or Legendre functions. An integral of this type occurs as the inversion formula for the generalized Mehler transform which is defined by
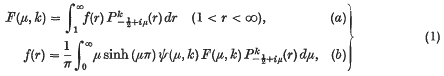
where ψ(μ, k) = Γ(½ − k + iμ)Γ(½ − k − iμ) and 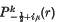 is the associated Legendre function of the first kind. Oberhettinger and Higgins (4) have given a table of transform pairs corresponding to the above transform.
is the associated Legendre function of the first kind. Oberhettinger and Higgins (4) have given a table of transform pairs corresponding to the above transform.
- Type
- Research Article
- Information
- Mathematical Proceedings of the Cambridge Philosophical Society , Volume 60 , Issue 1 , January 1964 , pp. 57 - 59
- Copyright
- Copyright © Cambridge Philosophical Society 1964
References
REFERENCES
- 4
- Cited by


