Hostname: page-component-cd9895bd7-gvvz8 Total loading time: 0 Render date: 2024-12-25T13:19:55.404Z Has data issue: false hasContentIssue false
  • English
  • Français

Limit laws for the averages of exponential random variables

Published online by Cambridge University Press:  24 October 2008

Lajos Horváth
Lajos Horváth
Affiliation:
Department of Statistics, University of Wisconsin-Madison, Madison, Wisconsin 53706-1693, U.S.A.
Get access Rights & Permissions [Opens in a new window]

Extract

Let {Xi. i ≥1} be a sequence of independent exponential random variables with mean 1. Setting S(n) = X1 +…+ Xn, S(0) = 0, we define

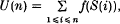
where f is a real-valued, measurable function satisfying

.

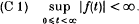Type
Research Article
Information
Mathematical Proceedings of the Cambridge Philosophical Society , Volume 105 , Issue 2 , March 1989 , pp. 381 - 388
Copyright
Copyright © Cambridge Philosophical Society 1989

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[1]Bingham, N. H. and Goldie, C. M.. Probabilistic and deterministic averaging. Trans. Amer. Math. Soc. 269 (1982), 453–480.Google Scholar
[2]Horváth, L.. Strong approximation of renewal processes. Stochastic Process. Appl. 18 (1984), 127–138.CrossRefGoogle Scholar
[3]Horváth, L.. Strong approximation of extended renewal processes. Ann. Probab. 12 (1984), 1149–1166.Google Scholar
[4]Meilijson, I.. The average of the values of a function at random points. Israel J. Math. 15 (1973), 193–203.CrossRefGoogle Scholar
[5]Petrov, V. V.. Sums of Independent Random Variables (Springer-Verlag, 1975).Google Scholar
[6]Stam, A. J.. Laws of large numbers for functions of random walks with positive drift. Compositio Math. 19 (1968), 299–333.Google Scholar