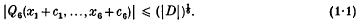Published online by Cambridge University Press: 24 October 2008
The famous conjecture of Watson(11) on the minima of indefinite quadratic forms in n variables has been proved for n ≤ 5, n ≥ 21 and for signatures 0 and ± 1. For the details and history of the conjecture the reader is referred to the author's paper(8). In the succeeding paper (9), we prove Watson's conjecture for signature ± 2 and ± 3 and for all n. Thus only one case for n = 6 (i.e. forms of type (1, 5) or (5, 1)) remains to he proved which we do here; thereby completing the case n = 6. This result is also used in (9) for proving the conjecture for all quadratic forms of signature ± 4. More precisely, here we prove:
Theorem 1. Let Q6(x1, …, x6) be a real indefinite quadratic form in six variables of determinant D ( < 0) and of type (5, 1) or (1, 5). Then given any real numbers ci, 1 ≤ i ≤ 6, there exist integers x1,…, x6such that