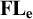 $\textbf{FL}_{\textbf{e}}$ ARE UNDECIDABLE
$\textbf{FL}_{\textbf{e}}$ ARE UNDECIDABLEPublished online by Cambridge University Press: 10 June 2021
All known structural extensions of the substructural logic 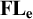 $\textbf{FL}_{\textbf{e}}$
, the Full Lambek calculus with exchange/commutativity (corresponding to subvarieties of commutative residuated lattices axiomatized by
$\textbf{FL}_{\textbf{e}}$
, the Full Lambek calculus with exchange/commutativity (corresponding to subvarieties of commutative residuated lattices axiomatized by 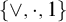 $\{\vee , \cdot , 1\}$
-equations), have decidable theoremhood; in particular all the ones defined by knotted axioms enjoy strong decidability properties (such as the finite embeddability property). We provide infinitely many such extensions that have undecidable theoremhood, by encoding machines with undecidable halting problem. An even bigger class of extensions is shown to have undecidable deducibility problem (the corresponding varieties of residuated lattices have undecidable word problem); actually with very few exceptions, such as the knotted axioms and the other prespinal axioms, we prove that undecidability is ubiquitous. Known undecidability results for non-commutative extensions use an encoding that fails in the presence of commutativity, so and-branching counter machines are employed. Even these machines provide encodings that fail to capture proper extensions of commutativity, therefore we introduce a new variant that works on an exponential scale. The correctness of the encoding is established by employing the theory of residuated frames.
$\{\vee , \cdot , 1\}$
-equations), have decidable theoremhood; in particular all the ones defined by knotted axioms enjoy strong decidability properties (such as the finite embeddability property). We provide infinitely many such extensions that have undecidable theoremhood, by encoding machines with undecidable halting problem. An even bigger class of extensions is shown to have undecidable deducibility problem (the corresponding varieties of residuated lattices have undecidable word problem); actually with very few exceptions, such as the knotted axioms and the other prespinal axioms, we prove that undecidability is ubiquitous. Known undecidability results for non-commutative extensions use an encoding that fails in the presence of commutativity, so and-branching counter machines are employed. Even these machines provide encodings that fail to capture proper extensions of commutativity, therefore we introduce a new variant that works on an exponential scale. The correctness of the encoding is established by employing the theory of residuated frames.