Published online by Cambridge University Press: 13 March 2009
A general formalism for describing two-time fluctuations in magnetized plasma is presented. Two-time expectations of one-body operators (phase functions) are written in terms of the phase space density autocorrelation function

where δN is the fluctuation in the singular Klimontovich microdensity. It is shown that  is the first member of a set of two-time quantities
is the first member of a set of two-time quantities
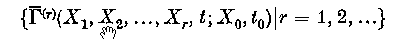
which collectively obeys the linearized BBGKY cumulant hierarchy in the (Xi, t) variables, with initial conditions successively smaller in the plasma parameter  . We study in detail the case of fluctuations in thermal equilibrium, although the general formalism holds also for the non-equilibrium case. To lowest order in εP, Γ obeys the linearized Vlasov equation. From this are recovered all of Rostoker's results for fluctuations excited by Cherenkov emission and absorbed by Landau damping, as well as a constructive proof of the test particle superposition principle. To first order, Γ obeys (in the Markovian approximation) the linearized Balescu-Guernsey-Lenard equation. For frequencies and wavenumbers in the hydrodynamic regime, the velocity moments of Γ obey linearized fluid equations with classical transport coefficients (i.e. essentially those computed by Braginskii in the 3-D case). It has been found that the classical theory is in disagreement with certain computer and laboratory experiments performed in strong magnetic fields. This defect is attributed to the absence in the classical theory of contributions to the collision operator, hence transport coefficients, of fluctuations long-lived on the Vlasov scale. Analogous difficulties arise in the theory of hydrodynamics in neutral fluids. To improve the plasma theory, a renormalization of the two-time hierarchy is proposed which sums selected terms from all orders in εP and thus treats the hydrodynamic fluctuations self-consistently. The resulting theory retains appropriate fluid conservation laws, thereby avoiding erroneous results encountered in certain diffusing orbit theories, when the fluid viscosity is indiscriminantly replaced by the test particle diffusion coefficient. In order to explain the results of the computer simulations, the theory is applied in part 2 to the problem of anomalous hydrodynamic contributions to the transport coefficients.
. We study in detail the case of fluctuations in thermal equilibrium, although the general formalism holds also for the non-equilibrium case. To lowest order in εP, Γ obeys the linearized Vlasov equation. From this are recovered all of Rostoker's results for fluctuations excited by Cherenkov emission and absorbed by Landau damping, as well as a constructive proof of the test particle superposition principle. To first order, Γ obeys (in the Markovian approximation) the linearized Balescu-Guernsey-Lenard equation. For frequencies and wavenumbers in the hydrodynamic regime, the velocity moments of Γ obey linearized fluid equations with classical transport coefficients (i.e. essentially those computed by Braginskii in the 3-D case). It has been found that the classical theory is in disagreement with certain computer and laboratory experiments performed in strong magnetic fields. This defect is attributed to the absence in the classical theory of contributions to the collision operator, hence transport coefficients, of fluctuations long-lived on the Vlasov scale. Analogous difficulties arise in the theory of hydrodynamics in neutral fluids. To improve the plasma theory, a renormalization of the two-time hierarchy is proposed which sums selected terms from all orders in εP and thus treats the hydrodynamic fluctuations self-consistently. The resulting theory retains appropriate fluid conservation laws, thereby avoiding erroneous results encountered in certain diffusing orbit theories, when the fluid viscosity is indiscriminantly replaced by the test particle diffusion coefficient. In order to explain the results of the computer simulations, the theory is applied in part 2 to the problem of anomalous hydrodynamic contributions to the transport coefficients.