Hostname: page-component-586b7cd67f-2brh9 Total loading time: 0 Render date: 2024-11-28T07:58:54.978Z Has data issue: false hasContentIssue false
  • English
  • Français

Sparsifying the resolvent forcing mode via gradient-based optimisation

Published online by Cambridge University Press:  06 July 2022

Calum S. Skene Open the ORCID record for Calum S. Skene [Opens in a new window] ,
Chi-An Yeh Open the ORCID record for Chi-An Yeh [Opens in a new window] ,
Peter J. Schmid Open the ORCID record for Peter J. Schmid [Opens in a new window]  and
Kunihiko Taira Open the ORCID record for Kunihiko Taira [Opens in a new window]
Calum S. Skene*
Affiliation:
Department of Mechanical and Aerospace Engineering, University of California, Los Angeles, CA 90095, USA
Chi-An Yeh
Affiliation:
Department of Mechanical and Aerospace Engineering, University of California, Los Angeles, CA 90095, USA
Peter J. Schmid
Affiliation:
Department of Mathematics, Imperial College London, London SW7 2AZ, UK
Kunihiko Taira
Affiliation:
Department of Mechanical and Aerospace Engineering, University of California, Los Angeles, CA 90095, USA
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

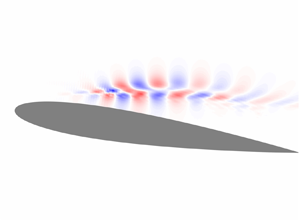
We consider the use of sparsity-promoting norms in obtaining localised forcing structures from resolvent analysis. By formulating the optimal forcing problem as a Riemannian optimisation, we are able to maximise cost functionals whilst maintaining a unit-energy forcing. Taking the cost functional to be the energy norm of the driven response results in a traditional resolvent analysis and is solvable by a singular value decomposition (SVD). By modifying this cost functional with the $L_1$-norm, we target spatially localised structures that provide an efficient amplification in the energy of the response. We showcase this optimisation procedure on two flows: plane Poiseuille flow at Reynolds number $Re=4000$, and turbulent flow past a NACA 0012 aerofoil at $Re=23\,000$. In both cases, the optimisation yields sparse forcing modes that maintain important features of the structures arising from an SVD in order to provide a gain in energy. These results showcase the benefits of utilising a sparsity-promoting resolvent formulation to uncover sparse forcings, specifically with a view to using them as actuation locations for flow control.

JFM classification

Instability: Shear-flow instability Flow Control: Instability control
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 944 , 10 August 2022 , A52
Check for updates
Copyright
© The Author(s), 2022. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

‡

Present address: Department of Applied Mathematics, University of Leeds, Leeds LS2 9JT, UK.

§

Present address: Department of Mechanical and Aerospace Engineering, North Carolina State University, NC 27695, USA.

Present address: Physical Science and Engineering Division, King Abdullah University of Science and Technology, Thuwal 23955-6900, Kingdom of Saudi Arabia.

References

Absil, P.-A., Mahony, R. & Sepulchre, R. 2007 Optimization Algorithms on Matrix Manifolds. Princeton University Press.Google Scholar
Amestoy, P.R., Buttari, A., L'Excellent, J.-Y. & Mary, T. 2019 Performance and scalability of the block low-rank multifrontal factorization on multicore architectures. ACM Trans. Math. Softw. 45, 1–26.CrossRefGoogle Scholar
Amestoy, P.R., Duff, I.S., Koster, J. & L'Excellent, J.-Y. 2001 A fully asynchronous multifrontal solver using distributed dynamic scheduling. SIAM J. Matrix Anal. Applics. 23 (1), 15–41.CrossRefGoogle Scholar
Balay, S. et al. 2021 a PETSc users manual. Tech. Rep. ANL-95/11 – Revision 3.15. Argonne National Laboratory.Google Scholar
Balay, S. et al. 2021 b PETSc Web page. https://petsc.org/.Google Scholar
Balay, S., Gropp, W.D., McInnes, L.C. & Smith, B.F. 1997 Efficient management of parallelism in object oriented numerical software libraries. In Modern Software Tools in Scientific Computing (ed. E. Arge, A.M. Bruaset & H.P. Langtangen), pp. 163–202. Birkhauser.CrossRefGoogle Scholar
Boumal, N. & Absil, P.-A. 2015 Low-rank matrix completion via preconditioned optimization on the Grassmann manifold. Linear Algebr. Applics. 475, 200–239.CrossRefGoogle Scholar
Boumal, N., Mishra, B., Absil, P.-A. & Sepulchre, R. 2014 Manopt, a Matlab toolbox for optimization on manifolds. J. Mach. Learn Res. 15, 1455–1459.Google Scholar
Brès, G.A., Ham, F.E., Nichols, J.W. & Lele, S.K. 2017 Unstructured large-eddy simulations of supersonic jets. AIAA J. 55 (4), 1164–1184.CrossRefGoogle Scholar
Bube, K.P. & Langan, R.T. 1997 Hybrid $\ell 1 / \ell 2$ minimization with applications to tomography. Geophysics 62 (4), 1183–1195.CrossRefGoogle Scholar
Bube, K.P. & Nemeth, T. 2007 Fast line searches for the robust solution of linear systems in the hybrid $\ell 1 / \ell 2$ and Huber norms. Geophysics 72 (2), A13–A17.CrossRefGoogle Scholar
Cattafesta, L.N. & Sheplak, M. 2011 Actuators for active flow control. Annu. Rev. Fluid Mech. 43, 247–272.CrossRefGoogle Scholar
Chu, B.T. 1965 On the energy transfer to small disturbances in fluid flow (part I). Acta Mechanica 1 (3), 215–234.CrossRefGoogle Scholar
Dalcin, L.D., Paz, R.R., Kler, P.A. & Cosimo, A. 2011 Parallel distributed computing using Python. Adv. Water Resour. 34 (9), 1124–1139, New Computational Methods and Software Tools.CrossRefGoogle Scholar
Douglas, S.C., Amari, S. & Kung, S.-Y. 1998 Gradient adaptation under unit-norm constraints. In Ninth IEEE Signal Processing Workshop on Statistical Signal and Array Processing (Cat. No.98TH8381), pp. 144–147. IEEE.Google Scholar
Farrell, B.F. & Ioannou, P.J. 1993 Stochastic forcing of the linearized Navier–Stokes equations. Phys. Fluids A: Fluid Dyn. 5 (11), 2600–2609.CrossRefGoogle Scholar
Fosas de Pando, M. 2020 IBMOS: immersed boundary method optimization and stability. https://doi.org/10.5281/zenodo.3757783.CrossRefGoogle Scholar
Fosas de Pando, M. & Schmid, P.J. 2017 Optimal frequency-response sensitivity of compressible flow over roughness elements. J. Turbul. 18 (4), 338–351.CrossRefGoogle Scholar
Fosas de Pando, M., Schmid, P.J. & Lele, S.K. 2014 Parametric sensitivity for large-scale aeroacoustic flows. In Proceedings of the 2014 Summer Program, pp. 365–374. Center for Turbulence Research, Stanford University.Google Scholar
Foures, D.P.G., Caulfield, C.P. & Schmid, P.J. 2013 Localization of flow structures using $\infty$-norm optimization. J. Fluid Mech. 729, 672–701.CrossRefGoogle Scholar
Giannetti, F. & Luchini, P. 2007 Structural sensitivity of the first instability of the cylinder wake. J. Fluid Mech. 581, 167–197.CrossRefGoogle Scholar
Hernandez, V., Roman, J.E. & Vidal, V. 2005 SLEPc: a scalable and flexible toolkit for the solution of eigenvalue problems. ACM Trans. Math. Softw. 31 (3), 351–362.CrossRefGoogle Scholar
Huang, W., Absil, P.-A. & Gallivan, K.A. 2016 A Riemannian BFGS method for nonconvex optimization problems. In Numerical Mathematics and Advanced Applications ENUMATH 2015 (ed. B. Karasözen, M. Manguoğlu, M. Tezer-Sezgin, S. Göktepe & Ö. Uğur), Lecture Notes in Computational Science and Engineering, vol. 112, pp. 627–634. Springer.CrossRefGoogle Scholar
Jeun, J., Nichols, J.W. & Jovanović, M.R. 2016 Input–output analysis of high-speed axisymmetric isothermal jet noise. Phys. Fluids 28 (4), 047101.CrossRefGoogle Scholar
Jovanović, M.R. 2004 Modeling, analysis, and control of spatially distributed systems. PhD thesis, University of California, Santa Barbara.Google Scholar
Jovanović, M.R. & Bamieh, B. 2005 Componentwise energy amplification in channel flows. J. Fluid Mech. 534, 145–183.CrossRefGoogle Scholar
Khalighi, Y., Nichols, J.W., Ham, F., Lele, S.K. & Moin, P. 2011 Unstructured large eddy simulation for prediction of noise issued from turbulent jets in various configurations. 17th AIAA/CEAS Aeroacoustics Conference (32nd AIAA Aeroacoustics Conference). AIAA Paper 2011-2886.CrossRefGoogle Scholar
Liu, Q., Sun, Y., Yeh, C.-A., Ukeiley, L.S., Cattafesta, L.N. & Taira, K. 2021 Unsteady control of supersonic turbulent cavity flow based on resolvent analysis. J. Fluid Mech. 925, A5.CrossRefGoogle Scholar
Luhar, M., Sharma, A.S. & McKeon, B.J. 2014 Opposition control within the resolvent analysis framework. J. Fluid Mech. 749, 597–626.CrossRefGoogle Scholar
McKeon, B.J. & Sharma, A.S. 2010 A critical-layer framework for turbulent pipe flow. J. Fluid Mech. 658, 336–382.CrossRefGoogle Scholar
Padovan, A., Otto, S.E. & Rowley, C.W. 2020 Analysis of amplification mechanisms and cross-frequency interactions in nonlinear flows via the harmonic resolvent. J. Fluid Mech. 900, A14.CrossRefGoogle Scholar
Paladini, E., Beneddine, S., Dandois, J., Sipp, D. & Robinet, J.-C. 2019 Transonic buffet instability: from two-dimensional airfoils to three-dimensional swept wings. Phys. Rev. Fluids 4, 103906.CrossRefGoogle Scholar
Pringle, C.C.T., Willis, A.P. & Kerswell, R.R. 2012 Minimal seeds for shear flow turbulence: using nonlinear transient growth to touch the edge of chaos. J. Fluid Mech. 702, 415–443.CrossRefGoogle Scholar
Qadri, U.A. & Schmid, P.J. 2017 Frequency selection mechanisms in the flow of a laminar boundary layer over a shallow cavity. Phys. Rev. Fluids 2, 013902.CrossRefGoogle Scholar
Ribeiro, J.H.M., Yeh, C.-A. & Taira, K. 2020 Randomized resolvent analysis. Phys. Rev. Fluids 5, 033902.CrossRefGoogle Scholar
Rigas, G., Sipp, D. & Colonius, T. 2021 Nonlinear input/output analysis: application to boundary layer transition. J. Fluid Mech. 911, A15.CrossRefGoogle Scholar
Rosenberg, K., Symon, S. & McKeon, B.J. 2019 Role of parasitic modes in nonlinear closure via the resolvent feedback loop. Phys. Rev. Fluids 4, 052601.CrossRefGoogle Scholar
Schmid, P.J. & Brandt, L. 2014 Analysis of fluid systems: stability, receptivity, sensitivity: lecture notes from the FLOW-NORDITA summer school on advanced instability methods for complex flows, Stockholm, Sweden, 2013. Appl. Mech. Rev. 66 (2), 024803.CrossRefGoogle Scholar
Schmid, P.J. & Henningson, D.S. 2001 Stability and Transition in Shear Flows, Applied Mathematical Sciences, vol. 142. Springer.CrossRefGoogle Scholar
Skene, C.S., Ribeiro, J.H.M. & Taira, K. 2022 csskene/linear-analysis-tools: initial release. https://doi.org/10.5281/zenodo.6550726.CrossRefGoogle Scholar
Sun, Y., Taira, K., Cattafesta, L.N. & Ukeiley, L.S. 2017 Biglobal instabilities of compressible open-cavity flows. J. Fluid Mech. 826, 270–301.CrossRefGoogle Scholar
Taira, K., Brunton, S.L., Dawson, S.T.M., Rowley, C.W., Colonius, T., McKeon, B.J., Schmidt, O.T., Gordeyev, S., Theofilis, V. & Ukeiley, L.S. 2017 Modal analysis of fluid flows: an overview. AIAA J. 55 (12), 4013–4041.CrossRefGoogle Scholar
Taira, K. & Colonius, T. 2007 The immersed boundary method: a projection approach. J. Comput. Phys. 225 (2), 2118–2137.CrossRefGoogle Scholar
Taira, K., Hemati, M.S., Brunton, S.L., Sun, Y., Duraisamy, K., Bagheri, S., Dawson, S. & Yeh, C.-A. 2020 Modal analysis of fluid flows: applications and outlook. AIAA J. 58 (3), 998–1022.CrossRefGoogle Scholar
Toedtli, S.S., Luhar, M. & McKeon, B.J. 2019 Predicting the response of turbulent channel flow to varying-phase opposition control: resolvent analysis as a tool for flow control design. Phys. Rev. Fluids 4, 073905.CrossRefGoogle Scholar
Townsend, J., Koep, N. & Weichwald, S. 2016 Pymanopt: a Python toolbox for optimization on manifolds using automatic differentiation. J. Mach. Learn Res. 17 (137), 1–5.Google Scholar
Trefethen, L.N., Trefethen, A.E., Reddy, S.C. & Driscoll, T.A. 1993 Hydrodynamic stability without eigenvalues. Science 261 (5121), 578–584.CrossRefGoogle ScholarPubMed
Vreman, A.W. 2004 An eddy-viscosity subgrid-scale model for turbulent shear flow: algebraic theory and applications. Phys. Fluids 16 (10), 3670–3681.CrossRefGoogle Scholar
Yeh, C.-A., Benton, S.I., Taira, K. & Garmann, D.J. 2020 Resolvent analysis of an airfoil laminar separation bubble at $Re=500\,000$. Phys. Rev. Fluids 5 (8), 083906.CrossRefGoogle Scholar
Yeh, C.-A. & Taira, K. 2019 Resolvent-analysis-based design of airfoil separation control. J. Fluid Mech. 867, 572–610.CrossRefGoogle Scholar