Hostname: page-component-586b7cd67f-dlnhk Total loading time: 0 Render date: 2024-11-28T13:20:35.244Z Has data issue: false hasContentIssue false
  • English
  • Français

Receptivity characteristics of under-expanded supersonic impinging jets

Published online by Cambridge University Press:  26 February 2020

Shahram Karami Open the ORCID record for Shahram Karami [Opens in a new window] ,
Paul C. Stegeman ,
Andrew Ooi Open the ORCID record for Andrew Ooi [Opens in a new window] ,
Vassilis Theofilis Open the ORCID record for Vassilis Theofilis [Opens in a new window]  and
Julio Soria Open the ORCID record for Julio Soria [Opens in a new window]
Shahram Karami*
Affiliation:
Laboratory for Turbulence Research in Aerospace and Combustion (LTRAC), Department of Mechanical and Aerospace Engineering, Monash University, Melbourne3800, Australia
Paul C. Stegeman
Affiliation:
Laboratory for Turbulence Research in Aerospace and Combustion (LTRAC), Department of Mechanical and Aerospace Engineering, Monash University, Melbourne3800, Australia
Andrew Ooi
Affiliation:
Department of Mechanical Engineering, University of Melbourne, Victoria3010, Australia
Vassilis Theofilis
Affiliation:
School of Engineering, University of Liverpool, LiverpoolL69 7ZX, UK Department of Mechanical Engineering, University of São Paulo, Av. Prof. Mello Moraes, 2231,São Paulo, Brazil
Julio Soria
Affiliation:
Laboratory for Turbulence Research in Aerospace and Combustion (LTRAC), Department of Mechanical and Aerospace Engineering, Monash University, Melbourne3800, Australia Department of Aeronautical Engineering, King Abdulaziz University, Jeddah21589, Kingdom of Saudi Arabia
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

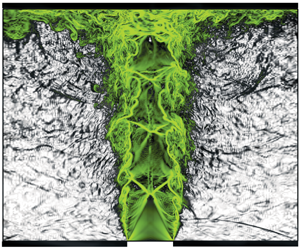
The receptivity of an under-expanded supersonic impinging jet flow at a sharp nozzle lip to acoustic impulse disturbances is investigated as a function of geometric and flow parameters. The under-expanded supersonic jets emanate from an infinite-lipped nozzle, i.e. the nozzle exit is a circular hole in a flat plate. Two specific cases have been investigated corresponding to nozzle-to-wall distances of $h=2d$ and $5d$, where $d$ is the jet diameter, at a nozzle pressure ratio of 3.4 and a Reynolds number of 50 000. Receptivity in this study is defined as originally coined by Morkovin (Tech. Rep. AFFDL TR, 1969, pp. 68–149; see also Reshotko, AGARD Special Course on Stability and Transition of Laminar Flow, N84-33757 23-34) as the internalisation of an external disturbance into the initial condition that either initiates or sustains a vortical fluid dynamic instability. Notionally, receptivity can be considered as a transfer function between the external disturbance and the initial conditions of the vortical instability. In the case of under-expanded supersonic impinging jet flow subjected to an acoustic disturbance, this transfer function is located at the nozzle lip and, thus, is amenable to an impulse response analysis using the linearised compressible three-dimensional Navier–Stokes equations. In this study, the transfer function at the nozzle lip is defined as the ratio of the output flow energy to the input acoustic energy of the acoustic disturbance. The sensitivity of this transfer function to the angular acoustic disturbance location, its azimuthal mode number and Strouhal number has been investigated for the two under-expanded supersonic impinging jet flow cases. It is found that for both the $h=2d$ and $5d$ cases, acoustic disturbances located at angles greater than $80^{\circ }$ from the jet centreline, with Strouhal numbers in the range between 0.7 and 6.5, have the highest receptivity for all azimuthal mode numbers investigated, except the azimuthal mode number 2 in the case of $h=5d$. The case with $h=5d$ is found to also have high receptivity to acoustic disturbances located at angles between $15^{\circ }$ and $50^{\circ }$ from the jet centreline for acoustic disturbances of all azimuthal mode numbers.

JFM classification

Turbulent Flows: Compressible turbulence Turbulent Flows: Shear layer turbulence Flow Control: Instability control
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 889 , 25 April 2020 , A27
Copyright
© The Author(s), 2020. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Ackeret, J. 1927 Gasdynamik. In Mechanik der Flüssigen und Gasförmigen Körper (ed. Ackeret, J., Betz, A., Forchheimer, P., Gyemant, A., Hopf, L., Lagally, M. & Grammel, R.), pp. 289–342. Springer.CrossRefGoogle Scholar
Amili, O., Edgington-Mitchell, D., Honnery, D. & Soria, J. 2016 Interaction of a supersonic underexpanded jet with a flat plate. In Fluid–Structure–Sound Interactions and Control, pp. 247–251. Springer.CrossRefGoogle Scholar
Barkley, D. 2006 Linear analysis of the cylinder wake mean flow. Europhys. Lett. 75 (5), 750.CrossRefGoogle Scholar
Barone, M. F. & Lele, S. K. 2005 Receptivity of the compressible mixing layer. J. Fluid Mech. 540, 301–335.CrossRefGoogle Scholar
Bechert, D. W. 1988 Excitation of instability waves in free shear layers. Part 1. Theory. J. Fluid Mech. 186, 47–62.CrossRefGoogle Scholar
Bechert, D. W. & Stahl, B. 1988 Excitation of instability waves in free shear layers. Part 2. Experiments. J. Fluid Mech. 186, 63–84.CrossRefGoogle Scholar
Bendat, J. S. & Piersol, A. G. 2011 Random Data: Analysis and Measurement Procedures, vol. 729. Wiley.Google Scholar
Beneddine, S., Sipp, D., Arnault, A., Dandois, J. & Lesshafft, L. 2016 Conditions for validity of mean flow stability analysis. J. Fluid Mech. 798, 485–504.CrossRefGoogle Scholar
Bodony, D. J. & Lele, S. K. 2005 On using large-eddy simulation for the prediction of noise from cold and heated turbulent jets. Phys. Fluids 17 (8), 085103.Google Scholar
Bogey, C., Marsden, O. & Bailly, C. 2011 Large-eddy simulation of the flow and acoustic fields of a Reynolds number 105 subsonic jet with tripped exit boundary layers. Phys. Fluids 23 (3), 035104.CrossRefGoogle Scholar
Dauptain, A., Cuenot, B. & Gicquel, L. Y. M. 2010 Large eddy simulation of stable supersonic jet impinging on flat plate. AIAA J. 48 (10), 2325–2338.CrossRefGoogle Scholar
Donaldson, C. & Snedeker, R. S. 1971 A study of free jet impingement. Part 1. Mean properties of free and impinging jets. J. Fluid Mech. 45 (2), 281–319.CrossRefGoogle Scholar
Edgington-Mitchell, D., Honnery, D. R. & Soria, J. 2014a The underexpanded jet Mach disk and its associated shear layer. Phys. Fluids 26 (9), 096101.CrossRefGoogle Scholar
Edgington-Mitchell, D., Oberleithner, K., Honnery, D. R. & Soria, J. 2014b Coherent structure and sound production in the helical mode of a screeching axisymmetric jet. J. Fluid Mech. 748, 822–847.CrossRefGoogle Scholar
Emden, R. 1899 Ueber die Ausströmungserscheinungen permanenter Gase. Ann. Phys. 305 (9), 264–289.CrossRefGoogle Scholar
Erturk, E. & Corke, T. C. 2001 Boundary layer leading-edge receptivity to sound at incidence angles. J. Fluid Mech. 444, 383–407.CrossRefGoogle Scholar
Gojon, R. & Bogey, C. 2017 Flow structure oscillations and tone production in underexpanded impinging round jets. AIAA J. 55 (6), 1792–1805.CrossRefGoogle Scholar
Gojon, R., Bogey, C. & Marsden, O.2015 Large-eddy simulation of underexpanded round jets impinging on a flat plate 4 to 9 radii downstream from the nozzle. AIAA Paper 2210, 2015.Google Scholar
Haddad, O. M. & Corke, T. C. 1998 Boundary layer receptivity to free-stream sound on parabolic bodies. J. Fluid Mech. 368, 1–26.CrossRefGoogle Scholar
Hammond, D. A. & Redekopp, L. G. 1997 Global dynamics of symmetric and asymmetric wakes. J. Fluid Mech. 331, 231–260.CrossRefGoogle Scholar
Henderson, B., Bridges, J. & Wernet, M. 2005 An experimental study of the oscillatory flow structure of tone-producing supersonic impinging jets. J. Fluid Mech. 542, 115–137.CrossRefGoogle Scholar
Henderson, B. & Powell, A. 1993 Experiments concerning tones produced by an axisymmetric choked jet impinging on flat plates. J. Sound Vib. 168 (2), 307–326.CrossRefGoogle Scholar
Henderson, L. F. 1966 Experiments on the impingement of a supersonic jet on a flat plate. Z. Angew. Math. Phys. 17 (5), 553–569.CrossRefGoogle Scholar
Ho, C.-M. & Nosseir, N. S. 1981 Dynamics of an impinging jet. Part 1. The feedback phenomenon. J. Fluid Mech. 105, 119–142.CrossRefGoogle Scholar
Illingworth, S. J., Monty, J. P. & Marusic, I. 2018 Estimating large-scale structures in wall turbulence using linear models. J. Fluid Mech. 842, 146–162.CrossRefGoogle Scholar
Jones, D. S. & Morgan, J. D. 1973 The instability due to acoustic radiation striking a vortex sheet on a supersonic stream. Proc. R. Soc. Edin. A 71 (2), 121–140.Google Scholar
Jones, D. S. & Morgan, J. P. 1972 The instability of a vortex sheet on a subsonic stream under acoustic radiation. In Mathematical Proceedings of the Cambridge Philosophical Society, vol. 72, pp. 465–488. Cambridge University Press.Google Scholar
Karami, S., Edgington-Mitchell, D. & Soria, J. 2018a Large eddy simulation of supersonic under-expanded jets impinging on a flat plate. In Proceedings of the 11th Australasian Heat and Mass Transfer Conference, p. 12. Australasian Fluid and Thermal Engineering Society (AFTES).Google Scholar
Karami, S. & Soria, J. 2018 Analysis of coherent structures in an under-expanded supersonic impinging jet using spectral proper orthogonal decomposition (SPOD). Aerospace 5 (3), 73.CrossRefGoogle Scholar
Karami, S., Stegeman, P. C., Ooi, A. & Soria, J. 2019 High-order accurate large-eddy simulations of compressible viscous flow in cylindrical coordinates. Comput. Fluids 191, 104241.CrossRefGoogle Scholar
Karami, S., Stegeman, P. C., Theofilis, V., Schmid, P. J. & Soria, J. 2018b Linearised dynamics and non-modal instability analysis of an impinging under-expanded supersonic jet. In Journal of Physics: Conference Series, vol. 1001, 012019. IOP Publishing.Google Scholar
Kawai, S. & Lele, S. K. 2010 Large-eddy simulation of jet mixing in supersonic crossflows. AIAA J. 48 (9), 2063–2083.CrossRefGoogle Scholar
Kennedy, C. A. & Carpenter, M. H. 1994 Several new numerical methods for compressible shear-layer simulations. Appl. Numer. Maths 14 (4), 397–433.CrossRefGoogle Scholar
Kennedy, C. A., Carpenter, M. H. & Lewis, R. M. 2000 Low-storage, explicit Runge–Kutta schemes for the compressible Navier–Stokes equations. Appl. Numer. Maths 35 (3), 177–219.CrossRefGoogle Scholar
Kerschen, E. 1996 Receptivity of shear layers to acoustic disturbances. In Theroretical Fluid Mechanics Conference, p. 2135. American Institute of Aeronautics and Astronautics (AIAA).Google Scholar
Li, S., Muddle, B., Jahedi, M. & Soria, J. 2012 A numerical investigation of the cold spray process using underexpanded and overexpanded jets. J. Therm. Spray Technol. 21, 108–120.CrossRefGoogle Scholar
Lilly, D. K. 1992 A proposed modification of the Germano subgrid-scale closure method. Phys. Fluids A 4 (3), 633–635.CrossRefGoogle Scholar
Mason-Smith, N., Edgington-Mitchell, D., Buchmann, N. A., Honnery, D. R. & Soria, J. 2015 Shock structures and instabilities formed in an underexpanded jet impinging on to cylindrical sections. Shock Waves 25 (6), 611–622.CrossRefGoogle Scholar
Mittal, S. 2008 Global linear stability analysis of time-averaged flows. Intl J. Numer. Meth. Fluids 58 (1), 111–118.CrossRefGoogle Scholar
Morgan, J. D. 1974 The interaction of sound with a semi-infinite vortex sheet. Q. J. Mech. Appl. Maths 27 (4), 465–487.CrossRefGoogle Scholar
Morkovin, M. V.1969 Critical evaluation of transition from laminar to turbulent shear layers with emphasis on hypersonically traveling bodies. Tech. Rep. AFFDL TR, 68–149.Google Scholar
Oberleithner, K., Rukes, L. & Soria, J. 2014 Mean flow stability analysis of oscillating jet experiments. J. Fluid Mech. 757, 1–32.CrossRefGoogle Scholar
Pier, B. 2002 On the frequency selection of finite-amplitude vortex shedding in the cylinder wake. J. Fluid Mech. 458, 407–417.CrossRefGoogle Scholar
Poinsot, T. & Lele, S. K. 1992 Boundary conditions for direct simulations of compressible viscous flows. J. Comput. Phys. 101 (1), 104–129.CrossRefGoogle Scholar
Powell, A. 1988 The sound-producing oscillations of round underexpanded jets impinging on normal plates. J. Acoust. Soc. Am. 83 (2), 515–533.CrossRefGoogle Scholar
Prandtl, L. 1904 Über die stationären Wellen in einem Gasstrahl. Phys. Z. 5, 599–6010.Google Scholar
Prandtl, L. 1907 Neue Untersuchungen über die strömende Bewegung der Gase und Dämpfe. Phys. Z. 8, 23–30.Google Scholar
Prandtl, L. 1913 Gasbewegung. Handwörterbuch der Naturwissenschaften 4, 544–560.Google Scholar
Raman, G. 1997 Cessation of screech in underexpanded jets. J. Fluid Mech. 336, 69–90.CrossRefGoogle Scholar
Raman, G. & Srinivasan, K. 2009 The powered resonance tube: from Hartmann’s discovery to current active flow control applications. Prog. Aerosp. Sci. 45 (4-5), 97–123.CrossRefGoogle Scholar
Reshotko, E. 1976 Boundary-layer stability and transition. Annu. Rev. Fluid Mech. 8 (1), 311–349.CrossRefGoogle Scholar
Reshotko, E. 1984 Environment and receptivity. In AGARD Special Course on Stability and Transition of Laminar Flow (N84-33757 23-34).Google Scholar
Risborg, A. & Soria, J. 2009 High-speed optical measurements of an underexpanded supersonic jet impinging on an inclined plate. In 28th International Congress on High-Speed Imaging and Photonics (ed. Kleine, H. & Guillen, M. P. B.), vol. 7126, pp. 477–487. International Society for Optics and Photonics, SPIE.Google Scholar
Rogler, H. L. & Reshotko, E. 1975 Disturbances in a boundary layer introduced by a low intensity array of vortices. SIAM J. Appl. Maths 28 (2), 431–462.CrossRefGoogle Scholar
Rossiter, J. E.1964 Wind tunnel experiments on the flow over rectangular cavities at subsonic and transonic speeds. Tech. Rep. Ministry of Aviation; Royal Aircraft Establishment; RAE Farnborough.Google Scholar
Rowley, C. W.2002 Modeling, simulation, and control of cavity flow oscillations. PhD thesis, California Institute of Technology, Pasadena, CA.Google Scholar
Rowley, C. W., Colonius, T. & Basu, A. J. 2002 On self-sustained oscillations in two-dimensional compressible flow over rectangular cavities. J. Fluid Mech. 455, 315–346.CrossRefGoogle Scholar
Ruban, A. I., Bernots, T. & Kravtsova, M. A. 2016 Linear and nonlinear receptivity of the boundary layer in transonic flows. J. Fluid Mech. 786, 154–189.CrossRefGoogle Scholar
Sartor, F., Mettot, C., Bur, R. & Sipp, D. 2015 Unsteadiness in transonic shock-wave/boundary-layer interactions: experimental investigation and global stability analysis. J. Fluid Mech. 781, 550–577.CrossRefGoogle Scholar
Sasaki, K., Vinuesa, R., Cavalieri, A. V. G., Schlatter, P. & Henningson, D. S. 2019 Transfer functions for flow predictions in wall-bounded turbulence. J. Fluid Mech. 864, 708–745.CrossRefGoogle Scholar
Sipp, D. & Lebedev, A. 2007 Global stability of base and mean flows: a general approach and its applications to cylinder and open cavity flows. J. Fluid Mech. 593, 333–358.CrossRefGoogle Scholar
Soria, J. & Risborg, A.2019 High-speed optical measurements of an under-expanded supersonic jet impinging on an inclined plate. Available at: https://doi.org/10.26180/5db3cea26f7b3.CrossRefGoogle Scholar
Stegeman, P. C., Ooi, A. & Soria, J. 2015 Proper orthogonal decomposition and dynamic mode decomposition of under-expanded free-jets with varying nozzle pressure ratios. In Instability and Control of Massively Separated Flows, pp. 85–90. Springer.CrossRefGoogle Scholar
Stegeman, P. C., Pèrez, J. M., Soria, J. & Theofilis, V. 2016a Inception and evolution of coherent structures in under-expanded supersonic jets. In Journal of Physics: Conference Series, vol. 708, 012015.Google Scholar
Stegeman, P. C., Soria, J. & Ooi, A. 2016b Interaction of shear layer coherent structures and the stand-off shock of an under-expanded circular impinging jet. In Fluid–Structure–Sound Interactions and Control, pp. 241–245. Springer.CrossRefGoogle Scholar
Tam, C. K. W. 1986 Excitation of instability waves by sound A physical interpretation. J. Sound Vib. 105 (1), 169–172.CrossRefGoogle Scholar
Tam, C. K. W. & Ahuja, K. K. 1990 Theoretical model of discrete tone generation by impinging jets. J. Fluid Mech. 214, 67–87.CrossRefGoogle Scholar
Turton, S. E., Tuckerman, L. S. & Barkley, D. 2015 Prediction of frequencies in thermosolutal convection from mean flows. Phys. Rev. E 91 (4), 043009.Google ScholarPubMed
Wanderley, J. B. V. & Corke, T. C. 2001 Boundary layer receptivity to free-stream sound on elliptic leading edges of flat plates. J. Fluid Mech. 429, 1–21.CrossRefGoogle Scholar
Weightman, J. L., Amili, O., Honnery, D., Edgington-Mitchell, D. & Soria, J. 2019 Nozzle external geometry as a boundary condition for the azimuthal mode selection in an impinging underexpanded jet. J. Fluid Mech. 862, 421–448.CrossRefGoogle Scholar

Karami et al. supplementary movie 1

The density gradient for the under-expanded supersonic impinging jets with the nozzle-pressure ratio of 3.4 and the nozzle-to-wall distance of 2d.

Download Karami et al. supplementary movie 1(Video)
Video 9.9 MB

Karami et al. supplementary movie 2

The density gradient for the under-expanded supersonic impinging jets with the nozzle-pressure ratio of 3.4 and the nozzle-to-wall distance of 5d.

Download Karami et al. supplementary movie 2(Video)
Video 46.8 MB