Hostname: page-component-586b7cd67f-t7fkt Total loading time: 0 Render date: 2024-12-01T07:20:16.974Z Has data issue: false hasContentIssue false
  • English
  • Français

Quasistatic magnetoconvection: heat transport enhancement and boundary layer crossing

Published online by Cambridge University Press:  14 May 2019

Zi Li Lim ,
Kai Leong Chong ,
Guang-Yu Ding  and
Ke-Qing Xia Open the ORCID record for Ke-Qing Xia [Opens in a new window]
Zi Li Lim
Affiliation:
Department of Physics, The Chinese University of Hong Kong, Shatin, Hong Kong, China
Kai Leong Chong
Affiliation:
Department of Physics, The Chinese University of Hong Kong, Shatin, Hong Kong, China
Guang-Yu Ding
Affiliation:
Department of Physics, The Chinese University of Hong Kong, Shatin, Hong Kong, China
Ke-Qing Xia*
Affiliation:
Department of Physics, The Chinese University of Hong Kong, Shatin, Hong Kong, China Center for Complex Flows and Soft Matter Research and Department of Mechanics and Aerospace Engineering, Southern University of Science and Technology, Shenzhen, 518055, China
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

We present a numerical study of quasistatic magnetoconvection in a cubic Rayleigh–Bénard (RB) convection cell subjected to a vertical external magnetic field. For moderate values of the Hartmann number 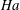$Ha$ (characterising the strength of the stabilising Lorentz force), we find an enhancement of heat transport (as characterised by the Nusselt number 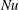$Nu$). Furthermore, a maximum heat transport enhancement is observed at certain optimal 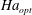$Ha_{opt}$. The enhanced heat transport may be understood as a result of the increased coherence of the thermal plumes, which are elementary heat carriers of the system. To our knowledge this is the first time that a heat transfer enhancement by the stabilising Lorentz force in quasistatic magnetoconvection has been observed. We further found that the optimal enhancement may be understood in terms of the crossing of the thermal and the momentum boundary layers (BL) and the fact that temperature fluctuations are maximum near the position where the BLs cross. These findings demonstrate that the heat transport enhancement phenomenon in the quasistatic magnetoconvection system belongs to the same universality class of stabilising–destabilising (S–D) turbulent flows as the systems of confined Rayleigh–Bénard (CRB), rotating Rayleigh–Bénard (RRB) and double-diffusive convection (DDC). This is further supported by the findings that the heat transport, boundary layer ratio and temperature fluctuations in magnetoconvection at the boundary layer crossing point are similar to the other three cases. A second type of boundary layer crossing is also observed in this work. In the limit of 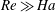$Re\gg Ha$, the (traditionally defined) viscous boundary 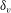$\unicode[STIX]{x1D6FF}_{v}$ is found to follow a Prandtl–Blasius-type scaling with the Reynolds number 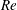$Re$ and is independent of 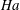$Ha$. In the other limit of 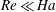$Re\ll Ha$, $\unicode[STIX]{x1D6FF}_{v}$ exhibits an approximate 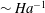${\sim}Ha^{-1}$ dependence, which has been predicted for a Hartmann boundary layer. Assuming the inertial term in the momentum equation is balanced by both the viscous and Lorentz terms, we derived an expression 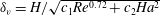$\unicode[STIX]{x1D6FF}_{v}=H/\sqrt{c_{1}Re^{0.72}+c_{2}Ha^{2}}$ (where 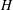$H$ is the height of the cell) for all values of 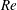$Re$ and 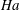$Ha$, which fits the obtained viscous boundary layer well.

JFM classification

MHD and Electrohydrodynamics: Magneto convection Convection: Plumes/thermals
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 870 , 10 July 2019 , pp. 519 - 542
Copyright
© 2019 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Ahlers, G., Grossmann, S. & Lohse, D. 2009 Heat transfer and large-scale dynamics in turbulent Rayleigh–Bénard convection. Rev. Mod. Phys. 81 (2), 503–537.Google Scholar
Aurnou, J. M. & Olson, P. L. 2001 Experiments on Rayleigh–Bénard convection, magnetoconvection and rotating magnetoconvection in liquid gallium. J. Fluid Mech. 430, 283–307.Google Scholar
Burr, U. & Müller, U. 2002 Rayleigh–Bénard convection in liquid metal layers under the influence of a horizontal magnetic field. J. Fluid Mech. 453, 345–369.Google Scholar
Chakraborty, S. 2008 On scaling laws in turbulent magnetohydrodynamic Rayleigh–Bénard convection. Physica D 237 (24), 3233–3236.Google Scholar
Chandrasekhar, S. 2013 Hydrodynamic and Hydromagnetic Stability. Courier Corporation.Google Scholar
Chillà, F. & Schumacher, J. 2012 New perspectives in turbulent Rayleigh–Bénard convection. Eur. Phys. J. E 35, 58.Google Scholar
Chong, K. L., Ding, G. & Xia, K.-Q. 2018 Multiple-resolution scheme in finite-volume code for active or passive scalar turbulence. J. Comput. Phys. 375, 1045–1058.Google Scholar
Chong, K. L., Huang, S.-D., Kaczorowski, M. & Xia, K.-Q. 2015 Condensation of coherent structures in turbulent flows. Phys. Rev. Lett. 115, 264503.Google Scholar
Chong, K. L., Wagner, S., Kaczorowski, M., Shishkina, O. & Xia, K.-Q. 2018 Effect of Prandtl number on heat transport enhancement in Rayleigh–Bénard convection under geometrical confinement. Phys. Rev. Fluids 3 (1), 013501.Google Scholar
Chong, K. L. & Xia, K.-Q. 2016 Exploring the severely confined regime in Rayleigh–Bénard convection. J. Fluid Mech. 805, R4.Google Scholar
Chong, K. L., Yang, Y., Huang, S.-D., Zhong, J.-Q., Stevens, R. J. A. M., Verzicco, R., Lohse, D. & Xia, K.-Q. 2017 Confined Rayleigh–Bénard, rotating Rayleigh–Bénard, and double diffusive convection: a unifying view on turbulent transport enhancement through coherent structure manipulation. Phys. Rev. Lett. 119, 064501.Google Scholar
Cioni, S., Chaumat, S. & Sommeria, J. 2000 Effect of a vertical magnetic field on turbulent Rayleigh–Bénard convection. Phys. Rev. E 62 (4), R4520.Google Scholar
Grossmann, S. & Lohse, D. 2000 Scaling in thermal convection: a unifying theory. J. Fluid Mech. 407, 27–56.Google Scholar
Huang, S.-D., Kaczorowski, M., Ni, R. & Xia, K.-Q. 2013 Confinement-induced heat-transport enhancement in turbulent thermal convection. Phys. Rev. Lett. 111, 104501.Google Scholar
Hurlburt, N. E., Matthews, P. C. & Rucklidge, A. M. 2000 Solar Magnetoconvection–(invited review). Solar Phys. 192 (1–2), 109–118.Google Scholar
Kaczorowski, M., Chong, K. L. & Xia, K.-Q. 2014 Turbulent flow in the bulk of Rayleigh–Bénard convection:aspect-ratio dependence of the small-scale properties. J. Fluid Mech. 747, 73–102.Google Scholar
Kaczorowski, M. & Xia, K.-Q. 2013 Turbulent flow in the bulk of Rayleigh–Bénard convection: small-scale properties in a cubic cell. J. Fluid Mech. 722, 596–617.Google Scholar
Kadanoff, L. P. 2001 Turbulent heat flow: structures and scaling. Phys. Today 54 (8), 34–39.Google Scholar
Kelley, D. H. & Weier, T. 2018 Fluid mechanics of liquid metal batteries. Appl. Mech. Rev. 70 (2), 020801.Google Scholar
King, E., Stellmach, S., Noir, J., Hansen, U. & Aurnou, J. 2009 Boundary layer control of rotating convection systems. Nature 457 (7227), 301–304.Google Scholar
Kitchatinov, L. L., Jardine, M. & Cameron, A. C. 2001 Pre-main sequence dynamos and relic magnetic fields of solar-type stars. Astron. Astrophys. 374 (1), 250–258.Google Scholar
Knaepen, B. & Moreau, R. 2008 Magnetohydrodynamic turbulence at low magnetic Reynolds number. Annu. Rev. Fluid Mech. 40, 25–45.Google Scholar
Lohse, D. & Xia, K.-Q. 2010 Small-scale properties of turbulent Rayleigh–Bénard convection. Annu. Rev. Fluid Mech. 42, 335–364.Google Scholar
Lui, S.-L. & Xia, K.-Q. 1998 Spatial structure of the thermal boundary layer in turbulent convection. Phys. Rev. E 57 (5), 5494–5503.Google Scholar
MacDonald, J. & Mullan, D. J. 2017 Apparent non-coevality among the stars in Upper Scorpio: resolving the problem using a model of magnetic inhibition of convection. Astrophys. J. 834 (1), 67.Google Scholar
Naffouti, A., Ben-Beya, B. & Lili, T. 2014 Three-dimensional Rayleigh–Bénard magnetoconvection: effect of the direction of the magnetic field on heat transfer and flow patterns. Comptes Rendus Mecanique 342 (12), 714–725.Google Scholar
Schüssler, M. 2012 Solar magneto-convection. Proc. Intl Astron. Union 8 (S294), 95–106.Google Scholar
Shen, Y. & Zikanov, O. 2016 Thermal convection in a liquid metal battery. Theor. Comput. Fluid Dyn. 30 (4), 275–294.Google Scholar
Stevens, R. J. A. M., Clercx, H. J. H. & Lohse, D. 2013 Heat transport and flow structure in rotating Rayleigh–Bénard convection. Eur. J. Mech. (B/Fluids) 40, 41–49.Google Scholar
Stevens, R. J. A. M., Zhong, J. Q., Clercx, H. J. H., Ahlers, G. & Lohse, D. 2009 Transitions between turbulent states in rotating Rayleigh–Bénard convection. Phys. Rev. Lett. 103, 024503.Google Scholar
Sun, C., Cheung, Y.-H. & Xia, K.-Q. 2008 Experimental studies of the viscous boundary layer properties in turbulent Rayleigh–Bénard convection. J. Fluid Mech. 605, 79–113.Google Scholar
Tasaka, Y., Igaki, K., Yanagisawa, T., Vogt, T., Zuerner, T. & Eckert, S. 2016 Regular flow reversals in Rayleigh–Bénard convection in a horizontal magnetic field. Phys. Rev. E 93 (4), 043109.Google Scholar
Tilgner, A., Belmonte, A. & Libchaber, A. 1993 Temperature and velocity profiles of turbulent convection in water. Phys. Rev. E 47 (4), R2253.Google Scholar
de Vaux, S. R., Zamansky, R., Bergez, W., Tordjeman, P. & Haquet, J.-F. 2017 Magnetoconvection transient dynamics by numerical simulation. Eur. Phys. J. E 40 (1), 13.Google Scholar
Wei, P. & Xia, K.-Q. 2013 Viscous boundary layer properties in turbulent thermal convection in a cylindrical cell: the effect of cell tilting. J. Fluid Mech. 720, 140–168.Google Scholar
Weiss, S., Wei, P. & Ahlers, G. 2016 Heat-transport enhancement in rotating turbulent Rayleigh–Bénard convection. Phys. Rev. E 93 (4), 043102.Google Scholar
Xi, H.-D., Lam, S. & Xia, K.-Q. 2004 From laminar plumes to organized flows: the onset of large-scale circulation in turbulent thermal convection. J. Fluid Mech. 503, 47–56.Google Scholar
Xia, K.-Q. 2013 Current trends and future directions in turbulent thermal convection. Theor. Appl. Mech. Lett. 3, 052001.Google Scholar
Xin, Y.-B., Xia, K.-Q. & Tong, P. 1996 Measured velocity boundary layers in turbulent convection. Phys. Rev. Lett. 77 (7), 1266.Google Scholar
Yanagisawa, T., Hamano, Y., Miyagoshi, T., Yamagishi, Y., Tasaka, Y. & Takeda, Y. 2013 Convection patterns in a liquid metal under an imposed horizontal magnetic field. Phys. Rev. E 88 (6), 063020.Google Scholar
Yang, Y., Verzicco, R. & Lohse, D. 2016 From convection rolls to finger convection in double-diffusive turbulence. Proc. Natl Acad. Sci. USA 113, 69–73.Google Scholar
Zhong, J.-Q., Stevens, R. J. A. M., Clercx, H. J. H., Verzicco, R., Lohse, D. & Ahlers, G. 2009 Prandtl-, Rayleigh-, and Rossby-number dependence of heat transport in turbulent rotating Rayleigh–Bénard convection. Phys. Rev. Lett. 102 (4), 044502.Google Scholar
Zürner, T., Liu, W., Krasnov, D. & Schumacher, J. 2016 Heat and momentum transfer for magnetoconvection in a vertical external magnetic field. Phys. Rev. E 94 (4), 043108.Google Scholar
Zwirner, L. & Shishkina, O. 2018 Confined inclined thermal convection in low-Prandtl-number fluids. J. Fluid Mech. 850, 984–1008.Google Scholar