Hostname: page-component-586b7cd67f-l7hp2 Total loading time: 0 Render date: 2024-11-25T00:38:46.189Z Has data issue: false hasContentIssue false
  • English
  • Français

Passive oscillations of inverted flags in a uniform flow

Published online by Cambridge University Press:  17 December 2019

Yao-Wei Hu Open the ORCID record for Yao-Wei Hu [Opens in a new window] ,
Li-Hao Feng Open the ORCID record for Li-Hao Feng [Opens in a new window]  and
Jin-Jun Wang Open the ORCID record for Jin-Jun Wang [Opens in a new window]
Yao-Wei Hu
Affiliation:
Fluid Mechanics Key Laboratory of Education Ministry, Beijing University of Aeronautics and Astronautics, Beijing100191, China
Li-Hao Feng*
Affiliation:
Fluid Mechanics Key Laboratory of Education Ministry, Beijing University of Aeronautics and Astronautics, Beijing100191, China
Jin-Jun Wang
Affiliation:
Fluid Mechanics Key Laboratory of Education Ministry, Beijing University of Aeronautics and Astronautics, Beijing100191, China
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

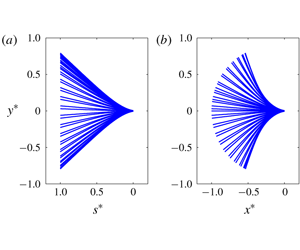
The passive oscillations of inverted flags are investigated both experimentally and theoretically in this paper. First, the force and energy distributions of inverted flags, which contain elastic and inertia components, are analysed based on the experimental data. Two main differences between inverted and conventional flags are found: (1) the elastic energy of a conventional flag is concentrated near the free end, while the fixed end of an inverted flag presents the largest elastic energy; and (2) the elastic component is several orders of magnitude greater than the inertia component for an inverted flag, while they are of the same magnitude for a conventional flag. Second, a linear analysis shows that the critical flow velocities obtained from the experiments at small mass ratios are scattered around the theoretical curve of wavenumber $k=1.875$, which is in contrast with $k=4.694$ of a conventional flag. For large mass ratios, the mass ratio has a certain influence on the critical velocity rather than being irrelevant. For two parallel inverted flags, both the experimental and theoretical results indicate that the range of the in-phase flapping mode becomes smaller with an increase in the separation distance, and a multiple flapping state may occur. For $n\geqslant 2$ parallel inverted flags, the theoretical results show that two of all coupled flapping modes are dominant with most parameters. These findings could contribute to a better understanding of the passive oscillations of inverted flags.

JFM classification

Aerodynamics: Aerodynamics
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 884 , 10 February 2020 , A32
Copyright
© 2019 Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Allen, J. J. & Smits, A. J. 2001 Energy harvesting ell. J. Fluids Struct. 15, 629–640.CrossRefGoogle Scholar
Chen, Y. J., Yu, Y. L., Zhou, W. W., Peng, D. & Liu, Y. Z. 2018 Heat transfer enhancement of turbulent channel flow using tandem self-oscillating inverted flags. Phys. Fluids 30, 075108.CrossRefGoogle Scholar
Fan, B.2015 Fluid-structure Interactions of Inverted Leaves and Flags. BS thesis, California Institute of Technology.Google Scholar
Gibbs, S. C., Sethna, A., Wang, I., Tang, D. & Dowell, E. H. 2014 Aeroelastic stability of a cantilevered plate in yawed subsonic flow. J. Fluids Struct. 49, 450–462.CrossRefGoogle Scholar
Gurugubelli, P. S. & Jaiman, R. K. 2015 Self-induced flapping dynamics of a flexible inverted foil in a uniform flow. J. Fluid Mech. 781, 657–694.CrossRefGoogle Scholar
Hu, Y. W., Wang, J. S., Wang, J. J. & Breitsamter, C. 2019 Flow-structure interaction of an inverted flag in a water tunnel. Sci. China-Phys. Mech. Astron. 62 (12), 124711.CrossRefGoogle Scholar
Huertas-Cerdeira, C., Fan, B. & Gharib, M. 2018 Coupled motion of two side-by-side inverted flags. J. Fluids Struct. 76, 527–535.CrossRefGoogle Scholar
Jia, L. B., Li, F., Yin, X. Z. & Yin, X. Y. 2007 Coupling modes between two flapping filaments. J. Fluid Mech. 581, 199–220.CrossRefGoogle Scholar
Jia, L. B. & Yin, X. Z. 2008 Passive oscillations of two tandem flexible filaments in a flowing soap film. Phys. Rev. Lett. 100, 228104.CrossRefGoogle Scholar
Jia, L. B.2009 The interaction between flexible plates and fluid in two-dimensional flow (in Chinese). PhD thesis, University of Science and Technology of China.Google Scholar
Kim, D., Cossé, J., Huertas-Cerdeira, C. & Gharib, M. 2013 Flapping dynamics of an inverted flag. J. Fluid Mech. 736, R1.CrossRefGoogle Scholar
Kim, H. & Kim, D. 2019 Stability and coupled dynamics of three-dimensional dual inverted flags. J. Fluids Struct. 84, 18–35.CrossRefGoogle Scholar
Kornecki, A., Dowell, E. H. & O’Brien, J. 1976 On the aeroelastic instability of two-dimensional panels in uniform incompressible flow. J. Sound Vib. 47 (2), 163–178.CrossRefGoogle Scholar
Orrego, S., Shoele, K., Ruas, A., Doran, K., Caggiano, B., Mittal, R. & Kang, S. H. 2017 Harvesting ambient wind energy with an inverted piezoelectric flag. Appl. Energy 194, 212–222.CrossRefGoogle Scholar
Park, S. G., Kim, B., Chang, C. B., Ryu, J. & Sung, H. J. 2016 Enhancement of heat transfer by a self-oscillating inverted flag in a Poiseuille channel flow. Intl J. Heat Mass Transfer 96, 362–370.CrossRefGoogle Scholar
Ryu, J., Park, S. G., Kim, B. & Sung, H. J. 2015 Flapping dynamics of an inverted flag in a uniform flow. J. Fluids Struct. 57, 159–169.CrossRefGoogle Scholar
Sader, J. E., Cossé, J., Kim, D., Fan, B. & Gharib, M. 2016 Large-amplitude flapping of an inverted flag in a uniform steady flow-a vortex-induced vibration. J. Fluid Mech. 793, 524–555.CrossRefGoogle Scholar
Schouveiler, L. & Eloy, C. 2009 Coupled flutter of parallel plates. Phys. Fluids 21 (8), 081703.CrossRefGoogle Scholar
Shelley, M., Vandenberghe, N. & Zhang, J. 2005 Heavy flags undergo spontaneous oscillations in flowing water. Phys. Rev. Lett. 94, 094302.CrossRefGoogle ScholarPubMed
Shelley, M. J. & Zhang, J. 2011 Flapping and bending bodies interacting with fluid flows. Annu. Rev. Fluid Mech. 43, 449–465.CrossRefGoogle Scholar
Shoele, K. & Mittal, R. 2016 Energy harvesting by flow-induced flutter in a simple model of an inverted piezoelectric flag. J. Fluid Mech. 790, 582–606.CrossRefGoogle Scholar
Taneda, S. 1968 Waving motion of flags. J. Phys. Soc. Japan 24 (2), 392–401.CrossRefGoogle Scholar
Tang, C., Liu, N. S. & Lu, X. Y. 2015a Dynamics of an inverted flexible plate in a uniform flow. Phys. Fluids 27, 073601.CrossRefGoogle Scholar
Tang, D., Gibbs, S. C. & Dowell, E. H. 2015b Nonlinear aeroelastic analysis with inextensible plate theory including correlation with experiment. AIAA J. 53 (5), 1299–1308.CrossRefGoogle Scholar
Watanabe, Y., Suzuki, S., Sugihara, M. & Sueokam, Y. 2002a An experimental study of paper flutter. J. Fluids Struct. 16 (4), 529–542.CrossRefGoogle Scholar
Watanabe, Y., Isogai, K., Suzuki, S. & Sugihara, M. 2002b A theoretical study of paper flutter. J. Fluids Struct. 16 (4), 543–560.CrossRefGoogle Scholar
Young, D. & Felgar, R. P. 1949 Tables of Characteristic Functions Representing Normal Modes of Vibration of a Beam. p. 4913. The University of Texas Publication.Google Scholar
Yu, Y. L., Liu, Y. Z. & Chen, Y. J. 2017 Vortex dynamics behind a self-oscillating inverted flag placed in a channel flow: time-resolved particle image velocimetry measurements. Phys. Fluids 29, 125104.CrossRefGoogle Scholar
Yu, Y. L., Liu, Y. Z. & Chen, Y. J. 2018 Vortex dynamics and heat transfer behind self-oscillating inverted flags of various lengths in channel flow. Phys. Fluids 30, 045104.CrossRefGoogle Scholar
Zhang, J., Childress, S., Libchaber, A. & Shelley, M. 2000 Flexible filaments in a flowing soap film as a model for one-dimensional flags in a two-dimensional wind. Nature 408, 835–839.CrossRefGoogle Scholar