Hostname: page-component-cd9895bd7-gxg78 Total loading time: 0 Render date: 2024-12-18T22:19:32.807Z Has data issue: false hasContentIssue false
  • English
  • Français

On the role of secondary motions in turbulent square duct flow

Published online by Cambridge University Press:  24 May 2018

Davide Modesti Open the ORCID record for Davide Modesti [Opens in a new window] ,
Sergio Pirozzoli Open the ORCID record for Sergio Pirozzoli [Opens in a new window] ,
Paolo Orlandi  and
Francesco Grasso
Davide Modesti*
Affiliation:
Cnam-Laboratoire DynFluid, 151 Boulevard de L’Hopital, 75013 Paris, France
Sergio Pirozzoli
Affiliation:
Dipartimento di Ingegneria Meccanica e Aerospaziale, Sapienza Università di Roma, Via Eudossiana 18, 00184 Roma, Italy
Paolo Orlandi
Affiliation:
Dipartimento di Ingegneria Meccanica e Aerospaziale, Sapienza Università di Roma, Via Eudossiana 18, 00184 Roma, Italy
Francesco Grasso
Affiliation:
Cnam-Laboratoire DynFluid, 151 Boulevard de L’Hopital, 75013 Paris, France
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

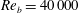We use a direct numerical simulations (DNS) database for turbulent flow in a square duct up to bulk Reynolds number $Re_{b}=40\,000$ to quantitatively analyse the role of secondary motions on the mean flow structure. For that purpose we derive a generalized form of the identity of Fukagata, Iwamoto and Kasagi (FIK), which allows one to quantify the effect of cross-stream convection on the mean streamwise velocity, wall shear stress and bulk friction coefficient. Secondary motions are found to contribute approximately 6 % of the total friction, and to act as a self-regulating mechanism of turbulence whereby wall shear stress non-uniformities induced by corners are equalized, and universality of the wall-normal velocity profiles is established. We also carry out numerical experiments whereby the secondary motions are artificially suppressed, in which case their equalizing role is partially taken by the turbulent stresses.

JFM classification

Turbulent Flows: Turbulence simulation Turbulent Flows: Turbulent Flows
Type
JFM Rapids
Information
Journal of Fluid Mechanics , Volume 847 , 25 July 2018 , R1
Check for updates
Copyright
© 2018 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Brundrett, E. & Baines, W. D. 1964 The production and diffusion of vorticity in duct flow. J. Fluid Mech. 19, 375–394.Google Scholar
Fukagata, K., Iwamoto, K. & Kasagi, N. 2002 Contribution of Reynolds stress distribution to the skin friction in wall-bounded flows. Phys. Fluids 14, L73–L76.Google Scholar
Gavrilakis, S. 1992 Numerical simulation of low-Reynolds-number turbulent flow through a straight square duct. J. Fluid Mech. 244, 101–129.Google Scholar
Gessner, F. B. & Jones, J. B. 1965 On some aspects of fully-developed turbulent flow in rectangular channels. J. Fluid Mech. 23, 689–713.Google Scholar
Jelly, T. O., Jung, S. Y. & Zaki, T. A. 2014 Turbulence and skin friction modification in channel flow with streamwise-aligned superhydrophobic surface texture. Phys. Fluids 26, 095102.Google Scholar
Marin, O., Vinuesa, R., Obabko, A. V. & Schlatter, P. 2016 Characterization of the secondary flow in hexagonal ducts. Phys. Fluids 28, 125101.Google Scholar
Modesti, D. & Pirozzoli, S. 2018 An efficient semi-implicit solver for direct numerical simulation of compressible flows at all speeds. J. Sci. Comput. 75, 308–331.Google Scholar
Nikuradse, J. 1930 Untersuchungen über turbulente Strömung in nicht kreisförmigen Rohren. Ing.-Arch. 1, 306–332.Google Scholar
Peet, Y. & Sagaut, P. 2009 Theoretical prediction of turbulent skin friction on geometrically complex surfaces. Phys. Fluids 21, 105105.CrossRefGoogle Scholar
Pinelli, A., Uhlmann, M., Sekimoto, A. & Kawahara, G. 2010 Reynolds number dependence of mean flow structure in square duct turbulence. J. Fluid Mech. 644, 107–122.Google Scholar
Pirozzoli, S., Modesti, D., Orlandi, P. & Grasso, F. 2018 Turbulence and secondary motions in square duct flow. J. Fluid Mech. 840, 631–655.Google Scholar
Prandtl, L. 1926 Über die ausgebildete Turbulenz. In Int. Congress for Applied Mechanics; also Turbulent flow, NACA-TM 435, 1927.Google Scholar
Sbragaglia, M. & Sugiyama, K. 2007 Boundary induced nonlinearities at small Reynolds numbers. Physica D 228 (2), 140–147.Google Scholar
Shah, R. k. & London, A. L. 2014 Laminar Flow Forced Convection in Ducts: A Source Book for Compact Heat Exchanger Analytical Data. Academic Press.Google Scholar
Spalart, P. R., Garbaruk, A. & Stabnikov, A. 2018 On the skin friction due to turbulence in ducts of various shapes. J. Fluid Mech. 838, 369–378.CrossRefGoogle Scholar
Speziale, C. G. 1982 On turbulent secondary flows in pipes of noncircular cross-section. Intl J. Engng Sci. 20, 863–872.Google Scholar
Townsend, A. A. 1976 The Structure of Turbulent Shear Flow. Cambridge University Press.Google Scholar
Vinuesa, R., Noorani, A., Lozano-Durán, A., El Khoury, G. K., Schlatter, P., Fischer, P. F. & Nagib, H. M. 2014 Aspect ratio effects in turbulent duct flows studied through direct numerical simulation. J. Turbul. 15, 677–706.Google Scholar
Vinuesa, R., Prus, C., Schlatter, P. & Nagib, H. M. 2016 Convergence of numerical simulations of turbulent wall-bounded flows and mean cross-flow structure of rectangular ducts. Meccanica 51, 3025–3042.Google Scholar
Wu, X. & Moin, P. 2008 A direct numerical simulation study on the mean velocity characteristics in turbulent pipe flow. J. Fluid Mech. 608, 81–112.Google Scholar