Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Wunsch, Scott
2018.
Nonlinear harmonic generation by internal waves in a density staircase.
Physical Review Fluids,
Vol. 3,
Issue. 11,
Zaron, Edward D.
2019.
Baroclinic Tidal Sea Level from Exact-Repeat Mission Altimetry.
Journal of Physical Oceanography,
Vol. 49,
Issue. 1,
p.
193.
Thomas, Jim
and
Yamada, Ray
2019.
Geophysical turbulence dominated by inertia–gravity waves.
Journal of Fluid Mechanics,
Vol. 875,
Issue. ,
p.
71.
Safari, Samaneh
and
Jazi, Bahram
2019.
About Generation of Terahertz Radiation Due to the Nonlinear Interaction of Gaussian and Hermite–Cosh–Gaussian Laser Beams in Collisional Plasma Background: Optimization and Field Profile Controlling.
IEEE Transactions on Plasma Science,
Vol. 47,
Issue. 1,
p.
155.
Kar, Subhajit
and
Guha, Anirban
2020.
An inverse technique for reconstructing ocean’s density stratification from surface data.
Ocean Modelling,
Vol. 147,
Issue. ,
p.
101561.
Gururaj, Saranraj
and
Guha, Anirban
2020.
Energy transfer in resonant and near-resonant internal wave triads for weakly non-uniform stratifications. Part 1. Unbounded domain.
Journal of Fluid Mechanics,
Vol. 899,
Issue. ,
Baker, Lois E.
and
Sutherland, Bruce R.
2020.
The evolution of superharmonics excited by internal tides in non-uniform stratification.
Journal of Fluid Mechanics,
Vol. 891,
Issue. ,
Husseini, Pauline
Varma, Dheeraj
Dauxois, Thierry
Joubaud, Sylvain
Odier, Philippe
and
Mathur, Manikandan
2020.
Experimental study on superharmonic wave generation by resonant interaction between internal wave modes.
Physical Review Fluids,
Vol. 5,
Issue. 7,
Varma, Dheeraj
Chalamalla, Vamsi K.
and
Mathur, Manikandan
2020.
Spontaneous superharmonic internal wave excitation by modal interactions in uniform and nonuniform stratifications.
Dynamics of Atmospheres and Oceans,
Vol. 91,
Issue. ,
p.
101159.
Sutherland, Bruce R.
and
Jefferson, Riley
2020.
Triad resonant instability of horizontally periodic internal modes.
Physical Review Fluids,
Vol. 5,
Issue. 3,
Patibandla, Ramana
Mathur, Manikandan
and
Roy, Anubhab
2021.
Triadic resonances in internal wave modes with background shear.
Journal of Fluid Mechanics,
Vol. 929,
Issue. ,
Wang, Yang
Xu, Zhenhua
Hibiya, Toshiyuki
Yin, Baoshu
and
Wang, Fan
2021.
Radiation Path of Diurnal Internal Tides in the Northwestern Pacific Controlled by Refraction and Interference.
Journal of Geophysical Research: Oceans,
Vol. 126,
Issue. 11,
Kim, Yeulwoo
Son, Sangyoung
Jung, Taehwa
and
Gallien, Timu
2021.
An analytical and numerical study of a vertically discretized multi-paddle wavemaker for generating free surface and internal waves.
Coastal Engineering,
Vol. 165,
Issue. ,
p.
103840.
Boury, S.
Peacock, T.
and
Odier, P.
2021.
Experimental generation of axisymmetric internal wave super-harmonics.
Physical Review Fluids,
Vol. 6,
Issue. 6,
Gururaj, Saranraj
and
Guha, Anirban
2022.
Resonant and near-resonant internal wave triads for non-uniform stratifications. Part 2. Vertically bounded domain with mild-slope bathymetry.
Journal of Fluid Mechanics,
Vol. 943,
Issue. ,
Sutherland, Bruce R.
and
Dhaliwal, Maninderpal S.
2022.
The nonlinear evolution of internal tides. Part 1: the superharmonic cascade.
Journal of Fluid Mechanics,
Vol. 948,
Issue. ,
Ivanov, P B
Chernov, S V
and
Barker, A J
2022.
Non-linear tidal excitation of superharmonic gravity waves in main-sequence stars in binary and exoplanetary systems.
Monthly Notices of the Royal Astronomical Society,
Vol. 511,
Issue. 4,
p.
5860.
Varma, Dheeraj
Mathur, Manikandan
and
Dauxois, Thierry
2022.
Instabilities in internal gravity waves.
Mathematics in Engineering,
Vol. 5,
Issue. 1,
p.
1.
Biswas, Lima
and
Shukla, Priyanka
2022.
Resonant triad interactions in a stably stratified uniform shear flow.
Physical Review Fluids,
Vol. 7,
Issue. 2,
Wunsch, Scott
and
Marcellino, F. Joseph
2023.
Estimates of mode-1 internal tide harmonic generation in the global ocean.
Physical Review Fluids,
Vol. 8,
Issue. 12,
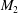 $M_{2}$ baroclinic internal tides, and the results indicate that a rapidly growing harmonic may occur in some environments near the Equator, but is unlikely at higher latitudes. The results are relevant to recent observations of
$M_{2}$ baroclinic internal tides, and the results indicate that a rapidly growing harmonic may occur in some environments near the Equator, but is unlikely at higher latitudes. The results are relevant to recent observations of 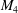 $M_{4}$ (harmonic) internal tides in the South China Sea and elsewhere. More generally, nonlinear self-interaction may contribute to the transfer of energy to smaller scales and the dissipation of baroclinic internal tides, especially in equatorial waters.
$M_{4}$ (harmonic) internal tides in the South China Sea and elsewhere. More generally, nonlinear self-interaction may contribute to the transfer of energy to smaller scales and the dissipation of baroclinic internal tides, especially in equatorial waters.


