Hostname: page-component-586b7cd67f-t8hqh Total loading time: 0 Render date: 2024-11-28T09:43:58.148Z Has data issue: false hasContentIssue false
  • English
  • Français

Effects of distributed roughness on crossflow instability through generalized resonance mechanisms

Published online by Cambridge University Press:  12 November 2018

Jiyang He ,
Adam Butler  and
Xuesong Wu
Jiyang He
Affiliation:
Laboratory of High-Speed Aerodynamics, School of Mechanical Engineering, Tianjin University, Tianjin 300072, PR China
Adam Butler
Affiliation:
Department of Mathematics, Imperial College London, 180 Queen’s Gate, London SW7 2AZ, UK
Xuesong Wu*
Affiliation:
Laboratory of High-Speed Aerodynamics, School of Mechanical Engineering, Tianjin University, Tianjin 300072, PR China Department of Mathematics, Imperial College London, 180 Queen’s Gate, London SW7 2AZ, UK
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Experiments have shown that micron-sized distributed surface roughness can significantly promote transition in a three-dimensional boundary layer dominated by crossflow instability. This sensitive effect has not yet been fully explained physically and mathematically. Past studies focused on surface roughness exciting crossflow vortices and/or changing the local stability characteristics. The present paper seeks possible additional mechanisms by investigating the effects of distributed surface roughness on crossflow instability through resonant interactions with eigenmodes. A key observation is that the perturbation induced by roughness with specific wavenumbers can interact with two eigenmodes (travelling and stationary vortices) through triadic resonance, or interact with one eigenmode (stationary vortices) through Bragg scattering. Unlike the usual triadic resonance of neutral, or nearly neutral, eigenmodes, the present triadic resonance can take place among modes with 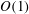$O(1)$ growth rates, provided that these are equal; unlike the usual Bragg scattering involving neutral waves, crossflow stationary vortices can also be unstable. For these amplifying waves, the generalized triadic resonance and Bragg scattering are put forward, and the resulting corrections to the growth rates are derived by a multiple-scale method. The analysis is extended to the case where up to four crossflow vortices interact with each other in the presence of suitable roughness components. The numerical results for Falkner–Skan–Cooke boundary layers show that roughness with a small height (a few percent of the local boundary-layer thickness) can change growth rates substantially (by a more-or-less 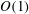$O(1)$ amount). This sensitive effect is attributed to two facts: (i) the resonant nature of the triadic interaction and Bragg scattering, which makes the correction to the growth rate proportional to the roughness height, and (ii) the wavenumbers of the roughness component required for the resonance are close to those of the neutral stationary crossflow modes, as a result of which a small roughness can generate a large response. Another important effect of roughness is that its presence renders the participating eigenmodes, which are otherwise independent, fully coupled. Our theoretical results suggest that micron-sized distributed surface roughness influences significantly both the amplification and spectral composition of crossflow vortices.

JFM classification

Boundary Layers: Boundary layer stability Instability: Transition to turbulence Waves/Free-surface Flows: Wave scattering
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 858 , 10 January 2019 , pp. 787 - 831
Check for updates
Copyright
© 2018 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Bertolotti, F. P. 2000 Receptivity of three-dimensional boundary-layers to localized wall roughness and suction. Phys. Fluids 12 (7), 1799–1809.Google Scholar
Borodulin, V. I., Kachanov, Y. S. & Koptsev, D. B. 2002 Experimental study of resonant interactions of instability waves in a self-similar boundary layer with an adverse pressure gradient: III. Broadband disturbances. J. Turbul. 3 (64), 1–19.Google Scholar
Butler, A. & Wu, X. 2018a Stationary crossflow vortices near the leading edge of three-dimensional boundary layers: the role of non-parallelism and excitation by surface roughness. J. Fluid Mech. 845, 93–140.Google Scholar
Butler, A. & Wu, X.2018b Vortex-roughness interactions in three-dimensional boundary layers (in preparation).Google Scholar
Cabal, A., Szumbarski, J. & Floryan, J. M. 2002 Stability of flow in a wavy channel. J. Fluid Mech. 457, 191–212.Google Scholar
Carpenter, A., Saric, W. S. & Reed, H. L.2008 Laminar flow control on a swept wing with distributed roughness. AIAA Paper 2008-7335.Google Scholar
Carpenter, A. L., Saric, W. S. & Reed, H. L.2009 In-flight receptivity experiments on a 30-degree swept-wing using micron-sized discrete roughness elements. AIAA Paper 2009-0590.Google Scholar
Carrillo, R. B. Jr., Reibert, M. S. & Saric, W. S. 1997 Distributed-roughness effects on stability and transition in swept-wing boundary layers. NASA Tech. Rep. NASA-CR-203580.Google Scholar
Cebeci, T. & Egan, D. A. 1989 Prediction of transition due to isolated roughness. AIAA J. 27 (7), 870–875.Google Scholar
Choudhari, M. 1994 Roughness-induced generation of crossflow vortices in three-dimensional boundary layers. Theor. Comput. Fluid Dyn. 6 (1), 1–30.Google Scholar
Choudhari, M. 1995 Long-wavelength asymptotics of unstable crossflow modes, including the effect of surface curvature. Proc. R. Soc. Lond. A 451, 515–541.Google Scholar
Choudhari, M. & Duck, P. W.1996 Nonlinear excitation of inviscid stationary vortex in a boundary-layer flow. NASA Tech. Rep. NASA-CR-198327.Google Scholar
Collis, S. S. & Lele, S. K. 1999 Receptivity to surface roughness near a swept leading edge. J. Fluid Mech. 380, 141–168.Google Scholar
Corke, T. C., Bar-Server, A. & Morkovin, M. V. 1986 Experiments on transition enhancement by distributed roughness. Phys. Fluids 29 (10), 3199–3213.Google Scholar
Craik, A. D. D. 1971 Non-linear resonant instability in boundary layers. J. Fluid Mech. 50 (2), 393–413.Google Scholar
Crouch, J.1993 Receptivity of three-dimensional boundary layers. AIAA Paper 1993-0074.Google Scholar
Floryan, J. M. 1997 Stability of wall-bounded shear layers in the presence of simulated distributed surface roughness. J. Fluid Mech. 335, 29–55.Google Scholar
Floryan, J. M. 2002 Centrifugal instability of Couette flow over a wavy wall. Phys. Fluids 14 (1), 312–322.Google Scholar
Gao, B., Park, D. H. & Park, S. O. 2011 Stability analysis of a boundary layer over a hump using parabolized stability equations. Fluid Dyn. Res. 43 (5), 055503.Google Scholar
Gaster, M.2016 Understanding the effects of surface roughness on the growth of disturbances. AIAA Paper 2016-4384.Google Scholar
Goldstein, M. E. & Hultgren, L. S. 1989 Boundary-layer receptivity to long-wave free-stream disturbances. Annu. Rev. Fluid Mech. 21 (1), 137–166.Google Scholar
Hamed, A. M., Sadowski, M., Zhang, Z. & Chamorro, L. P. 2016 Transition to turbulence over 2D and 3D periodic large-scale roughnesses. J. Fluid Mech. 804, R6.Google Scholar
Hosseini, S. M., Tempelmann, D., Hanifi, A. & Henningson, D. S. 2013 Stabilization of a swept-wing boundary layer by distributed roughness elements. J. Fluid Mech. 718, R1.Google Scholar
Högberg, M. & Henningson, D. S. 1998 Secondary instability of cross-flow vortices in Falkner–Skan–Cooke boundary layers. J. Fluid Mech. 368, 339–357.Google Scholar
Huang, Z. & Wu, X. 2017 A local scattering approach for the effects of abrupt changes on boundary-layer instability and transition: a finite-Reynolds-number formulation for isolated distortions. J. Fluid Mech. 822, 444–483.Google Scholar
Klebanoff, P. S. & Tidstrom, K. D. 1972 Mechanism by which a two-dimensional roughness element induces boundary-layer transition. Phys. Fluids 15 (7), 1173–1188.Google Scholar
Kurz, H. B. E. & Kloker, M. J. 2014 Receptivity of a swept-wing boundary layer to micron-sized discrete roughness elements. J. Fluid Mech. 755, 62–82.Google Scholar
Lessen, M. & Gangwani, S. T. 1976 Effect of small amplitude wall waviness upon the stability of the laminar boundary layer. Phys. Fluids 19 (4), 510–513.Google Scholar
Li, F., Choudhari, M., Chang, C.-L., Streett, C. & Carpenter, M. 2011 Computational modelling of roughness-based laminar flow control on a subsonic swept wing. AIAA J. 49, 520–529.Google Scholar
Malik, M. R. 1990 Numerical methods for hypersonic boundary layer stability. J. Comput. Phys. 86 (2), 376–413.Google Scholar
Malik, M., Liao, W., Li, F. & Choudhari, M.2013 DRE-enhanced swept-wing natural laminar flow at high Reynolds numbers. AIAA Paper 2013-0412.Google Scholar
Masad, J. A.1996 Effect of surface waviness on transition in three-dimensional boundary-layer flow. NASA Tech. Rep. NASA-CR-201641.Google Scholar
Masad, J. A. & Iyer, V. 1994 Transition prediction and control in subsonic flow over a hump. Phys. Fluids 6 (1), 313–327.Google Scholar
Mamun, M. D., Asai, M. & Inasawa, A. 2014 Effects of surface corrugation on the stability of a zero-pressure-gradient boundary layer. J. Fluid Mech. 741, 228–251.Google Scholar
Medeiros, M. A. F. & Gaster, S. 1999 The production of subharmonic waves in the nonlinear evolution of wavepackets in boundary layers. J. Fluid Mech. 399, 301–318.Google Scholar
Mei, C. C. 1985 Resonant reflection of surface water waves by periodic sandbars. J. Fluid Mech. 152, 315–335.Google Scholar
Mei, C. C., Hara, T. & Naciri, M. 1988 Note on Bragg scattering of water waves by parallel bars on the seabed. J. Fluid Mech. 186, 147–162.Google Scholar
Messing, R. & Kloker, M. J. 2010 Investigation of suction for laminar flow control of three-dimensional boundary layers. J. Fluid Mech. 658, 117–147.Google Scholar
Nayfeh, A. H., Ragab, S. A. & Al-Maaitah, A. A. 1988 Effect of bulges on the stability of boundary layers. Phys. Fluids 31 (4), 796–806.Google Scholar
Ng, L. L. & Crouch, J. D. 1999 Roughness-induced receptivity to crossflow vortices on a swept wing. Phys. Fluids 11 (2), 432–438.Google Scholar
Park, D. & Park, S. O. 2013 Linear and non-linear stability analysis of incompressible boundary layer over a two-dimensional hump. Comput. Fluids 73, 80–96.Google Scholar
Radeztsky, R. H., Reibert, M. S. & Saric, W. S. 1999 Effect of isolated micron-sized roughness on transition in swept-wing flows. AIAA J. 37 (11), 1370–1377.Google Scholar
Reibert, M. S., Saric, W. S., Carrillo, R. B. Jr. & Chapman, K. L. 1996 Experiments in nonlinear saturation of stationary crossflow vortices in a swept-wing boundary layer. AIAA Paper 1996-0184.Google Scholar
Rizzetta, D. P., Visbal, M. R., Reed, H. L. & Saric, W. S. 2010 Direct numerical simulation of discrete roughness on a swept-wing leading edge. AIAA J. 48 (11), 2660–2673.Google Scholar
Saric, W. S., Carrillo, R. B. Jr. & Reibert, M. S. 1998 Leading-edge roughness as a transition control mechanism. AIAA Paper 1998-0781.Google Scholar
Saric, W. S. & Reed, H. L.2003 Crossflow instabilities: theory and technology. AIAA Paper 2003-771.Google Scholar
Saric, W. S., Reed, H. L. & Kerschen, E. J. 2002 Boundary-layer receptivity to freestream disturbances. Annu. Rev. Fluid Mech. 34 (1), 291–319.Google Scholar
Saric, W. S., Reed, H. L. & White, E. B. 2003 Stability and transition of three-dimensional boundary layers. Annu. Rev. Fluid Mech. 35 (1), 413–440.Google Scholar
Schrader, L.-U., Brandt, L. & Henningson, D. S. 2009 Receptivity mechanisms in three-dimensional boundary-layer flows. J. Fluid Mech. 618, 209–241.Google Scholar
Tempelmann, D., Schrader, L.-U., Hanifi, A., Brandt, L. & Henningson, D. S. 2012 Swept wing boundary-layer receptivity to localized surface roughness. J. Fluid Mech. 711, 516–544.Google Scholar
Thomas, C., Mughal, S. & Ashworth, R. 2017 Development of Tollmien–Schlichting disturbances in the presence of laminar separation bubbles on an unswept infinite wavy wing. Phys. Rev. Fluids 2 (4), 043903.Google Scholar
Thomas, C., Mughal, S., Gipon, M., Ashworth, R. & Martinez-Cava, A. 2016 Stability of an infinite swept wing boundary layer with surface waviness. AIAA J. 54 (10), 3024–3038.Google Scholar
Wassermann, P. & Kloker, M. J. 2002 Mechanisms and passive control of crossflow-vortex-induced transition in a three-dimensional boundary layer. J. Fluid Mech. 456, 49–84.Google Scholar
Wie, Y.-S. & Malik, M. R. 1998 Effect of surface waviness on boundary-layer transition in two-dimensional flow. Comput. Fluids 27 (2), 157–181.Google Scholar
Woodruff, M. J., Saric, W. S. & Reed, H. L.2011 Receptivity measurements on a swept-wing model. AIAA Paper 2011-3882.Google Scholar
Wu, X. & Dong, M. 2016 A local scattering theory for the effects of isolated roughness on boundary-layer instability and transition: transmission coefficient as an eigenvalue. J. Fluid Mech. 794, 68–108.Google Scholar