Hostname: page-component-cd9895bd7-fscjk Total loading time: 0 Render date: 2024-12-17T06:31:21.897Z Has data issue: false hasContentIssue false
  • English
  • Français

The dynamics of settling particles in vertical channel flows: gravity, lift and particle clusters

Published online by Cambridge University Press:  14 May 2021

Amir Esteghamatian Open the ORCID record for Amir Esteghamatian [Opens in a new window]  and
Tamer A. Zaki Open the ORCID record for Tamer A. Zaki [Opens in a new window]
Amir Esteghamatian
Affiliation:
Department of Mechanical Engineering, Johns Hopkins University, Baltimore, MD21218, USA
Tamer A. Zaki*
Affiliation:
Department of Mechanical Engineering, Johns Hopkins University, Baltimore, MD21218, USA
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

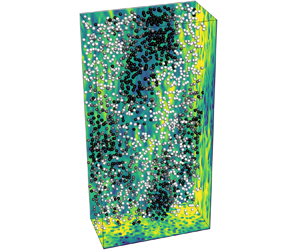
The dynamics of settling finite-size particles in vertical channel flows of Newtonian and viscoelastic carrier fluids is examined using particle resolved simulations. Comparison with neutrally buoyant particles in the same configuration highlights the effect of settling. The particle volume fraction is $5\,\%$, and a gravity field acts counter to the flow direction. Despite a modest density ratio ($\rho _r = 1.15$), qualitative changes arise due to the relative velocity between the particle and fluid phases. While dense particles are homogeneously distributed in the core of the channel, the mean concentration profile peaks at approximately two particle diameters from the wall due to a competition between shear- and rotation-induced lift forces. These forces act in the cross-stream directions, and are analysed by evaluating conditional averages along individual particle trajectories. The correlation between the angular and translational velocities of the particles highlights the significance of the Magnus lift force in both the spanwise and wall-normal directions. The collective behaviour of the particles is also intriguing. Using a Voronoï analysis, strong clustering is identified in dense particles near the wall, which is shown to alter their streamwise velocities. This clustering is attributed to the preferential transport of aggregated particles towards the wall. The practical implication of the non-uniformity of particle distribution is a significant increase in drag. When the carrier fluid is viscoelastic, the particle migration is enhanced which leads to larger stresses, thus negating the capacity of viscoelasticity to reduce turbulent drag.

JFM classification

Non-Newtonian Flows: Polymers Multiphase and Particle-laden Flows: Particle/fluid flow Turbulent Flows: Turbulence simulation
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 918 , 10 July 2021 , A33
Check for updates
Copyright
© The Author(s), 2021. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Agarwal, A., Brandt, L. & Zaki, T.A. 2014 Linear and nonlinear evolution of a localized disturbance in polymeric channel flow. J. Fluid Mech. 760, 278–303.CrossRefGoogle Scholar
Aliseda, A., Cartellier, A., Hainaux, F. & Lasheras, J.C. 2002 Effect of preferential concentration on the settling velocity of heavy particles in homogeneous isotropic turbulence. J. Fluid Mech. 468, 77–105.CrossRefGoogle Scholar
Auton, T.R., Hunt, J.C.R. & Prud'Homme, M. 1988 The force exerted on a body in inviscid unsteady non-uniform rotational flow. J. Fluid Mech. 197, 241–257.CrossRefGoogle Scholar
Bagchi, P. & Balachandar, S. 2002 Effect of free rotation on the motion of a solid sphere in linear shear flow at moderate Re. Phys. Fluids 14 (8), 2719–2737.CrossRefGoogle Scholar
Bobroff, S. & Phillips, R.J. 1998 Nuclear magnetic resonance imaging investigation of sedimentation of concentrated suspensions in non-Newtonian fluids. J. Rheol. 42 (6), 1419–1436.CrossRefGoogle Scholar
Capecelatro, J., Desjardins, O. & Fox, R.O. 2018 On the transition between turbulence regimes in particle-laden channel flows. J. Fluid Mech. 845, 499–519.CrossRefGoogle Scholar
Caporaloni, M., Tampieri, F., Trombetti, F. & Vittori, O. 1975 Transfer of particles in nonisotropic air turbulence. J. Atmos. Sci. 32, 565–568.2.0.CO;2>CrossRefGoogle Scholar
Chhabra, R.P. 2006 Bubbles, Drops, and Particles in Non-Newtonian Fluids. CRC.CrossRefGoogle Scholar
Choueiri, G.H., Lopez, J.M. & Hof, B. 2018 Exceeding the asymptotic limit of polymer drag reduction. Phys. Rev. Lett. 120 (12), 124501.CrossRefGoogle ScholarPubMed
Chouippe, A. & Uhlmann, M. 2019 On the influence of forced homogeneous-isotropic turbulence on the settling and clustering of finite-size particles. Acta Mech. 230 (2), 387–412.CrossRefGoogle Scholar
Cisse, M., Homann, H. & Bec, J. 2013 Slipping motion of large neutrally buoyant particles in turbulence. J. Fluid Mech. 735, R1.CrossRefGoogle Scholar
D'Avino, G. & Maffettone, P.L. 2015 Particle dynamics in viscoelastic liquids. J. Non-Newtonian Fluid Mech. 215, 80–104.CrossRefGoogle Scholar
Ding, E.-J. & Aidun, C.K. 2000 The dynamics and scaling law for particles suspended in shear flow with inertia. J. Fluid Mech. 423, 317–344.CrossRefGoogle Scholar
Dubief, Y., Terrapon, V.E., White, C.M., Shaqfeh, E.S.G., Moin, P. & Lele, S.K. 2005 New answers on the interaction between polymers and vortices in turbulent flows. Flow Turbul. Combust. 74 (4), 311–329.CrossRefGoogle Scholar
Elghobashi, S. 2006 An updated classification map of particle-laden turbulent flows. In IUTAM Symposium on Computational Approaches to Multiphase Flow (ed. S. Balachandar & A. Prosperetti), pp. 3–10. Springer.CrossRefGoogle Scholar
Elghobashi, S. & Truesdell, G.C. 1993 On the two-way interaction between homogeneous turbulence and dispersed solid particles. I. Turbulence modification. Phys. Fluids 5 (7), 1790–1801.CrossRefGoogle Scholar
Esteghamatian, A. & Zaki, T.A. 2019 Dilute suspension of neutrally buoyant particles in viscoelastic turbulent channel flow. J. Fluid Mech. 875, 286–320.CrossRefGoogle Scholar
Esteghamatian, A. & Zaki, T.A. 2020 Viscoelasticity and the dynamics of concentrated particle suspension in channel flow. J. Fluid Mech. 901, A25.CrossRefGoogle Scholar
Fiabane, L., Zimmermann, R., Volk, R., Pinton, J-F & Bourgoin, M. 2012 Clustering of finite-size particles in turbulence. Phys. Rev. E 86 (3), 035301.CrossRefGoogle ScholarPubMed
Fortes, A.F., Joseph, D.D. & Lundgren, T.S. 1987 Nonlinear mechanics of fluidization of beds of spherical particles. J. Fluid Mech. 177, 467–483.CrossRefGoogle Scholar
Frank, X. & Li, H.Z. 2006 Negative wake behind a sphere rising in viscoelastic fluids: a lattice Boltzmann investigation. Phys. Rev. E 74 (5), 1–9.CrossRefGoogle ScholarPubMed
Garcia-Villalba, M., Kidanemariam, A.G. & Uhlmann, M. 2012 DNS of vertical plane channel flow with finite-size particles: voronoi analysis, acceleration statistics and particle-conditioned averaging. Intl J. Multiphase Flow 46, 54–74.CrossRefGoogle Scholar
Glowinski, R., Pan, T.W., Hesla, T.I., Joseph, D.D. & Périaux, J. 2001 A fictitious domain approach to the direct numerical simulation of incompressible viscous flow past moving rigid bodies: application to particulate flow. J. Comput. Phys. 169 (2), 363–426.CrossRefGoogle Scholar
Gore, R.A & Crowe, C.T. 1989 Effect of particle size on modulating turbulent intensity. Intl J. Multiphase Flow 15 (2), 279–285.CrossRefGoogle Scholar
Goyal, N. & Derksen, J.J. 2012 Direct simulations of spherical particles sedimenting in viscoelastic fluids. J. Non-Newtonian Fluid Mech. 183-184, 1–13.CrossRefGoogle Scholar
Hameduddin, I., Gayme, D.F. & Zaki, T.A. 2019 Perturbative expansions of the conformation tensor in viscoelastic flows. J. Fluid Mech. 858, 377–406.CrossRefGoogle Scholar
Hameduddin, I., Meneveau, C., Zaki, T.A. & Gayme, D.F. 2018 Geometric decomposition of the conformation tensor in viscoelastic turbulence. J. Fluid Mech. 842, 395–427.CrossRefGoogle Scholar
Hameduddin, I. & Zaki, T.A. 2019 The mean conformation tensor in viscoelastic turbulence. J. Fluid Mech. 865, 363–380.CrossRefGoogle Scholar
Hetsroni, G. & Rozenblit, R. 1994 Heat transfer to a liquid-solid mixture in a flume. Intl J. Multiphase Flow 20 (4), 671–689.CrossRefGoogle Scholar
Huisman, S.G., Barois, T., Bourgoin, M., Chouippe, A., Doychev, T., Huck, P., Morales, C.E.B., Uhlmann, M. & Volk, R. 2016 Columnar structure formation of a dilute suspension of settling spherical particles in a quiescent fluid. Phys. Rev. Fluids 1 (7), 074204.CrossRefGoogle Scholar
Joseph, D.D., Liu, Y.J., Poletto, M. & Feng, J. 1994 Aggregation and dispersion of spheres falling in viscoelastic liquids. J. Non-Newtonian Fluid Mech. 54, 45–86.CrossRefGoogle Scholar
Kaftori, D., Hetsroni, G. & Banerjee, S. 1995 Particle behavior in the turbulent boundary layer. I. Motion, deposition, and entrainment. Phys. Fluids 7 (5), 1095–1106.CrossRefGoogle Scholar
Kajishima, T. 2004 Influence of particle rotation on the interaction between particle clusters and particle-induced turbulence. Intl J. Heat Fluid Flow 25 (5), 721–728.CrossRefGoogle Scholar
Kajishima, T., Takiguchi, S., Hamasaki, H. & Miyake, Y. 2001 Turbulence structure of particle-laden flow in a vertical plane channel due to vortex shedding. JSME Intl J. B 44 (4), 526–535.CrossRefGoogle Scholar
Kemiha, M., Frank, X., Poncin, S. & Li, H.Z. 2006 Origin of the negative wake behind a bubble rising in non-Newtonian fluids. Chem. Engng Sci. 61 (12), 4041–4047.CrossRefGoogle Scholar
Kidanemariam, A.G., Chan-Braun, C., Doychev, T. & Uhlmann, M. 2013 Direct numerical simulation of horizontal open channel flow with finite-size, heavy particles at low solid volume fraction. New J. Phys. 15, 025031.CrossRefGoogle Scholar
Kulick, J.D., Fessler, J.R. & Eaton, J.K. 1994 Particle response and turbulence modification in fully developed channel flow. J. Fluid Mech. 277, 109–134.CrossRefGoogle Scholar
Lee, S.J. & Zaki, T.A. 2017 Simulations of natural transition in viscoelastic channel flow. J. Fluid Mech. 820, 232–262.CrossRefGoogle Scholar
Leighton, D & Acrivos, A. 1985 The lift on a small sphere touching a plane in the presence of a simple shear flow. Z. Angew. Math. Phys. 36 (1), 174–178.CrossRefGoogle Scholar
Lu, J. & Tryggvason, G. 2013 Dynamics of nearly spherical bubbles in a turbulent channel upflow. J. Fluid Mech. 732, 166–189.CrossRefGoogle Scholar
Magnus, G. 1853 Ueber die abweichung der geschosse, und: ueber eine auffallende erscheinung bei rotirenden körpern. Ann. Phys. 164 (1), 1–29.CrossRefGoogle Scholar
Marchioli, C., Picciotto, M. & Soldati, A. 2007 Influence of gravity and lift on particle velocity statistics and transfer rates in turbulent vertical channel flow. Intl J. Multiphase Flow 33 (3), 227–251.CrossRefGoogle Scholar
Marchioli, C. & Soldati, A. 2002 Mechanisms for particle transfer and segregation in a turbulent boundary layer. J. Fluid Mech. 468, 283–315.CrossRefGoogle Scholar
Mei, R. 1992 An approximate expression for the shear lift force on a spherical particle at finite Reynolds number. Intl J. Multiphase Flow 18 (1), 145–147.CrossRefGoogle Scholar
Monchaux, R., Bourgoin, M. & Cartellier, A. 2010 Preferential concentration of heavy particles: a Voronoï analysis. Phys. Fluids 22 (10), 103304.CrossRefGoogle Scholar
Mora, S., Talini, L. & Allain, C. 2005 Structuring sedimentation in a shear-thinning fluid. Phys. Rev. Lett. 95 (8), 088301.CrossRefGoogle Scholar
Naso, A. & Prosperetti, A. 2010 The interaction between a solid particle and a turbulent flow. New J. Phys. 12, 033040.CrossRefGoogle Scholar
Nicolaou, L., Jung, S.Y. & Zaki, T.A. 2015 A robust direct-forcing immersed boundary method with enhanced stability for moving body problems in curvilinear coordinates. Comput. Fluids 119, 101–114.CrossRefGoogle Scholar
Okabe, A., Boots, B., Sugihara, K. & Chiu, S.N. 2000 Spatial tessellations: Concepts and applications of voronoi diagrams. In Wiley Series in Probability and Statistics, 2nd edn (ed. V. Barnett et al.). Wiley.CrossRefGoogle Scholar
Page, J. & Zaki, T.A. 2014 Streak evolution in viscoelastic Couette flow. J. Fluid Mech. 742, 520–521.CrossRefGoogle Scholar
Page, J. & Zaki, T.A. 2015 The dynamics of spanwise vorticity perturbations in homogeneous viscoelastic shear flow. J. Fluid Mech. 777, 327–363.CrossRefGoogle Scholar
Page, J. & Zaki, T.A. 2016 Viscoelastic shear flow over a wavy surface. J. Fluid Mech. 801, 392–429.CrossRefGoogle Scholar
Reeks, M.W. 1983 The transport of discrete particles in inhomogeneous turbulence. J. Aerosol Sci. 14 (6), 729–739.CrossRefGoogle Scholar
Rosenfeld, M., Kwak, D. & Vinokur, M. 1991 A fractional step solution method for the unsteady incompressible navier-stokes equations in generalized coordinate systems. J. Comput. Phys. 94 (1), 102–137.CrossRefGoogle Scholar
Saffman, P.G.T. 1965 The lift on a small sphere in a slow shear flow. J. Fluid Mech. 22 (2), 385–400.CrossRefGoogle Scholar
Samanta, D., Dubief, Y., Holzner, M., Schäfer, C., Morozov, A.N., Wagner, C. & Hof, B. 2013 Elasto-inertial turbulence. Proc. Natl Acad. Sci. USA 110 (26), 10557–10562.CrossRefGoogle ScholarPubMed
Shao, X., Wu, T. & Yu, Z. 2012 Fully resolved numerical simulation of particle-laden turbulent flow in a horizontal channel at a low Reynolds number. J. Fluid Mech. 693, 319–344.CrossRefGoogle Scholar
Tanaka, M. 2017 Effect of gravity on the development of homogeneous shear turbulence laden with finite-size particles. J. Turbul. 18 (12), 1144–1179.CrossRefGoogle Scholar
Toms, B.A. 1948 Some observations on the flow of linear polymer solutions through straight tubes at large Reynolds numbers. In Proceedings of the 1st International Congress on Rheology, pp. 135–141. North-Holland.Google Scholar
Tsuji, Y., Morikawa, Y. & Shiomi, H. 1984 LDV measurements of an air-solid two-phase flow in a vertical pipe. J. Fluid Mech. 139, 417–434.CrossRefGoogle Scholar
Uhlmann, M. 2008 Interface-resolved direct numerical simulation of vertical particulate channel flow in the turbulent regime. Phys. Fluids 20 (5), 053305.CrossRefGoogle Scholar
Uhlmann, M. & Doychev, T. 2014 Sedimentation of a dilute suspension of rigid spheres at intermediate Galileo numbers: the effect of clustering upon the particle motion. J. Fluid Mech. 752 (2), 310–348.CrossRefGoogle Scholar
Virk, P.S., Mickley, H.S. & Smith, K.A. 1970 The ultimate asymptote and mean flow structure in Toms’ phenomenon. Trans. ASME J. Appl. Mech. 2 (37), 488–493.CrossRefGoogle Scholar
Vreman, A.W. & Kuerten, J.G.M. 2018 Turbulent channel flow past a moving array of spheres. J. Fluid Mech. 856, 580–632.CrossRefGoogle Scholar
Wang, G., Fong, K.O., Coletti, F., Capecelatro, J. & Richter, D.H. 2019 a Inertial particle velocity and distribution in vertical turbulent channel flow: a numerical and experimental comparison. Intl J. Multiphase Flow 120, 103105.CrossRefGoogle Scholar
Wang, M., Wang, Q. & Zaki, T.A. 2019 b Discrete adjoint of fractional-step incompressible Navier–Stokes solver in curvilinear coordinates and application to data assimilation. J. Comput. Phys. 396, 427–450.CrossRefGoogle Scholar
Wells, M.R. & Stock, D.E. 1983 The effects of crossing trajectories on the dispersion of particles in a turbulent flow. J. Fluid Mech. 136, 31–62.CrossRefGoogle Scholar
White, C.M. & Mungal, M.G. 2008 Mechanics and prediction of turbulent drag reduction with polymer additives. Annu. Rev. Fluid Mech. 40 (1), 235–256.CrossRefGoogle Scholar
Wu, J. & Manasseh, R. 1998 Dynamics of dual-particles settling under gravity. Intl J. Multiphase Flow 24 (8), 1343–1358.CrossRefGoogle Scholar
Yin, X. & Koch, D.L. 2007 Hindered settling velocity and microstructure in suspensions of solid spheres with moderate Reynolds numbers. Phys. Fluids 19 (9), 093302.CrossRefGoogle Scholar
You, J. & Zaki, T.A. 2019 Conditional statistics and flow structures in turbulent boundary layers buffeted by free-stream disturbances. J. Fluid Mech. 866, 526–566.CrossRefGoogle Scholar
Young, J. & Leeming, A. 1997 A theory of particle deposition in turbulent pipe flow. J. Fluid Mech. 340, 129–159.CrossRefGoogle Scholar
Zeng, L., Balachandar, S. & Najjar, F.M. 2010 Wake response of a stationary finite-sized particle in a turbulent channel flow. Intl J. Multiphase Flow 36 (5), 406–422.CrossRefGoogle Scholar
Zeng, L., Najjar, F., Balachandar, S. & Fischer, P. 2009 Forces on a finite-sized particle located close to a wall in a linear shear flow. Phys. Fluids 21 (3), 033302.CrossRefGoogle Scholar
Zenit, R. & Feng, J.J. 2018 Hydrodynamic interactions among bubbles, drops, and particles in non-Newtonian liquids. Annu. Rev. Fluid Mech. 50 (1), 505–534.CrossRefGoogle Scholar