Hostname: page-component-586b7cd67f-2brh9 Total loading time: 0 Render date: 2024-12-01T01:14:23.277Z Has data issue: false hasContentIssue false
  • English
  • Français

Vortex generation due to internal solitary wave propagation past a sidewall constriction

Published online by Cambridge University Press:  03 March 2021

David Deepwell Open the ORCID record for David Deepwell [Opens in a new window] ,
Cameron Clarry ,
Christopher Subich Open the ORCID record for Christopher Subich [Opens in a new window]  and
Marek Stastna
David Deepwell*
Affiliation:
Department of Physics, University of Alberta, Edmonton, AlbertaT6G 2E1, Canada
Cameron Clarry
Affiliation:
Department of Physics, University of Toronto, Toronto, OntarioM5S 1A1, Canada
Christopher Subich
Affiliation:
Meteorological Research Division, Environment & Climate Change Canada, Dorval, QuebecH9P 1J3, Canada
Marek Stastna
Affiliation:
Department of Applied Mathematics, University of Waterloo, Waterloo, OntarioN2L 3G1, Canada
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

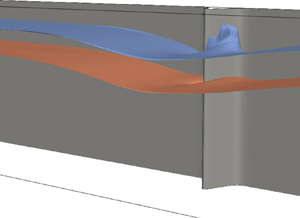
Internal solitary waves in the coastal ocean propagate in a complex environment, with variations in the background currents and stratification and with topography along both the bottom (e.g. sills) and sides (e.g. headlands). We present direct numerical simulations of internal solitary wave propagation past an isolated sidewall constriction on laboratory scales. We find that the wave-induced currents generate separation regions which develop into vortices above and below the wave-deformed pycnocline. These vortices yield horizontal tracer exchange between the near-wall region and channel interior and vertical transport sufficient to lift pycnocline fluid near to the surface. Quantitatively, the height of the vertical transport was found to be proportional to the square of the vertical vorticity. Increases in the wave amplitude and the aspect ratio of the constriction (height to width) led to stronger vortices, greater lateral and vertical transport and enhanced density overturning. We compare and contrast these findings with the literature on isolated bottom topography, focusing on the inherently three-dimensional nature (vorticity aligned perpendicular to isopycnals) of the instability in the sidewall case as opposed to typical instabilities caused by internal solitary waves.

JFM classification

Geophysical and Geological Flows: Internal waves Vortex Flows: Vortex dynamics Geophysical and Geological Flows: Stratified flows
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 913 , 25 April 2021 , A47
Check for updates
Copyright
© The Author(s), 2021. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Aghsaee, P., Boegman, L., Diamessis, P.J. & Lamb, K.G. 2012 Boundary-layer-separation-driven vortex shedding beneath internal solitary waves of depression. J.Fluid Mech. 690, 321–344.CrossRefGoogle Scholar
Aghsaee, P., Boegman, L. & Lamb, K.G. 2010 Breaking of shoaling internal solitary waves. J.Fluid Mech. 659, 289–317.CrossRefGoogle Scholar
Arthur, R.S. & Fringer, O.B. 2014 The dynamics of breaking internal solitary waves on slopes. J.Fluid Mech. 761 (2), 360–398.CrossRefGoogle Scholar
Arthur, R.S. & Fringer, O.B. 2016 Transport by breaking internal gravity waves on slopes. J.Fluid Mech. 789, 93–126.CrossRefGoogle Scholar
Basak, S. & Sarkar, S. 2006 Dynamics of a stratified shear layer with horizontal shear. J.Fluid Mech. 568, 19–54.CrossRefGoogle Scholar
Beckers, M., Verzicco, R., Clercx, H.J.H. & Van Heijst, G.J.F. 2001 Dynamics of pancake-like vortices in a stratified fluid: experiments, model and numerical simulations. J.Fluid Mech. 433, 1–27.CrossRefGoogle Scholar
Billant, P. & Bonnici, J. 2020 Evolution of a vortex in a strongly stratified shear flow. Part 2. Numerical simulations. J.Fluid Mech. 893, A18.CrossRefGoogle Scholar
Billant, P. & Chomaz, J.-M. 2000 a Experimental evidence for a new instability of a vertical columnar vortex pair in a strongly stratified fluid. J.Fluid Mech. 418, 167–188.CrossRefGoogle Scholar
Billant, P. & Chomaz, J.-M. 2000 b Theoretical analysis of the zigzag instability of a vertical columnar vortex pair in a strongly stratified fluid. J.Fluid Mech. 419, 29–63.CrossRefGoogle Scholar
Boegman, L., Ivey, G.N. & Imberger, J. 2005 The degeneration of internal waves in lakes with sloping topography. Limnol. Oceanogr. 50 (5), 1620–1637.CrossRefGoogle Scholar
Boegman, L. & Stastna, M. 2019 Sediment resuspension and transport by internal solitary waves. Annu. Rev. Fluid Mech. 51 (1), 129–154.CrossRefGoogle Scholar
Bogucki, D., Dickey, T. & Redekopp, L.G. 1997 Sediment resuspension and mixing by resonantly generated internal solitary waves. J.Phys. Oceanogr. 27 (7), 1181–1196.2.0.CO;2>CrossRefGoogle Scholar
Bogucki, D.J. & Redekopp, L.G. 1999 A mechanism for sediment resuspension by internal solitary waves. Geophys. Res. Lett. 26 (9), 1317–1320.CrossRefGoogle Scholar
Bonnier, M., Eiff, O. & Bonneton, P. 2000 On the density structure of far-wake vortices in a stratified fluid. Dyn. Atmos. Oceans 31 (1–4), 117–137.CrossRefGoogle Scholar
Boulanger, N., Meunier, P. & Le Dizés, S. 2007 Structure of a stratified tilted vortex. J.Fluid Mech. 583, 443–458.CrossRefGoogle Scholar
Boulanger, N., Meunier, P. & Le Dizès, S. 2008 Tilt-induced instability of a stratified vortex. J.Fluid Mech. 596, 1–20.CrossRefGoogle Scholar
Bourgault, D., Janes, D.C. & Galbraith, P.S. 2011 Observations of a large-amplitude internal wave train and its reflection off a steep slope. J.Phys. Oceanogr. 41 (3), 586–600.CrossRefGoogle Scholar
Bourgault, D., Morsilli, M., Richards, C., Neumeier, U. & Kelley, D.E. 2014 Sediment resuspension and nepheloid layers induced by long internal solitary waves shoaling orthogonally on uniform slopes. Cont. Shelf Res. 72, 21–33.CrossRefGoogle Scholar
Boyd, J.P. 2000 Chebyshev and Fourier Spectral Methods, 2nd edn. Dover Publications.Google Scholar
Canals, M., Pawlak, G. & MacCready, P. 2009 Tilted baroclinic tidal vortices. J.Phys. Oceanogr. 39 (2), 333–350.CrossRefGoogle Scholar
Carr, M., Davies, P.A. & Shivaram, P. 2008 Experimental evidence of internal solitary wave-induced global instability in shallow water benthic boundary layers. Phys. Fluids 20 (6), 066603.CrossRefGoogle Scholar
Deepwell, D., Stastna, M., Carr, M. & Davies, P.A. 2017 Interaction of a mode-2 internal solitary wave with narrow isolated topography. Phys. Fluids 29 (7), 076601.CrossRefGoogle Scholar
Diamessis, P.J. & Redekopp, L.G. 2006 Numerical investigation of solitary internal wave-induced global instability in shallow water benthic boundary layers. J.Phys. Oceanogr. 36 (5), 784–812.CrossRefGoogle Scholar
Dorostkar, A., Boegman, L. & Pollard, A. 2017 Three-dimensional simulation of high-frequency nonlinear internal wave dynamics in Cayuga Lake. J.Geophys. Res. 122 (3), 2183–2204.CrossRefGoogle Scholar
Dunphy, M., Subich, C. & Stastna, M. 2011 Spectral methods for internal waves: indistinguishable density profiles and double-humped solitary waves. Nonlinear Process. Geophys. 18 (3), 351–358.CrossRefGoogle Scholar
Edwards, K.A., MacCready, P., Moum, J.N., Pawlak, G., Klymak, J.M. & Perlin, A. 2004 Form drag and mixing due to tidal flow past a sharp point. J.Phys. Oceanogr. 34 (6), 1297–1312.2.0.CO;2>CrossRefGoogle Scholar
Farmer, D. & Smith, J.D. 1978 Nonlinear internal waves in a fjord. In Hydrodynamics of Estuaries and Fjords (ed. Jacques C.J. Nihoul), Elsevier Oceanography Series, vol. 23, pp. 465–493. Elsevier.CrossRefGoogle Scholar
Grimshaw, R.H.J. & Smyth, N. 1986 Resonant flow of a stratified fluid over topography. J.Fluid Mech. 169 (-1), 429.CrossRefGoogle Scholar
Harnanan, S., Soontiens, N. & Stastna, M. 2015 Internal wave boundary layer interaction: a novel instability over broad topography. Phys. Fluids 27 (1), 16605.CrossRefGoogle Scholar
Harnanan, S., Stastna, M. & Soontiens, N. 2017 The effects of near-bottom stratification on internal wave induced instabilities in the boundary layer. Phys. Fluids 29 (1), 016602.CrossRefGoogle Scholar
Helfrich, K.R. 1992 Internal solitary wave breaking and run-up on a uniform slope. J.Fluid Mech. 243 (-1), 133.CrossRefGoogle Scholar
Helfrich, K.R. & Melville, W.K. 2006 Long nonlinear internal waves. Annu. Rev. Fluid Mech. 38 (1), 395–425.CrossRefGoogle Scholar
Imasato, N., Fujio, S., Zhang, Q., Awaji, T. & Akitomo, K. 1994 Three-dimensional numerical experiments on tidal exchange through a narrow strait in a homogeneous and a stratified sea. J.Oceanogr. 50 (2), 119–139.CrossRefGoogle Scholar
Kundu, P.K., Cohen, I.M. & Dowling, D.R. 2012 Fluid Mechanics, 5th edn. Academic Press.Google Scholar
Lamb, K.G. 2004 On boundary-layer separation and internal wave generation at the Knight Inlet sill. Proc. R. Soc. Lond. A 460 (2048), 2305–2337.CrossRefGoogle Scholar
Lamb, K.G. 2014 Internal wave breaking and dissipation mechanisms on the continental slope/shelf. Annu. Rev. Fluid Mech. 46 (1), 231–254.CrossRefGoogle Scholar
Lelong, M.P. & Sundermeyer, M.A. 2005 Geostrophic adjustment of an isolated diapycnal mixing event and its implications for small-scale lateral dispersion. J.Phys. Oceanogr. 35 (12), 2352–2367.CrossRefGoogle Scholar
MacCready, P. & Pawlak, G. 2001 Stratified flow along a corrugated slope: separation drag and wave drag. J.Phys. Oceanogr. 31 (10), 2824–2839.2.0.CO;2>CrossRefGoogle Scholar
Masunaga, E., Arthur, R.S., Fringer, O.B. & Yamazaki, H. 2017 Sediment resuspension and the generation of intermediate nepheloid layers by shoaling internal bores. J.Mar. Syst. 170, 31–41.CrossRefGoogle Scholar
Michallet, H. & Ivey, G.N. 1999 Experiments on mixing due to internal solitary waves breaking on uniform slopes. J.Geophys. Res. 104 (C6), 13467–13477.CrossRefGoogle Scholar
Olsthoorn, J. & Stastna, M. 2014 Numerical investigation of internal wave-induced sediment motion: resuspension versus entrainment. Geophys. Res. Lett. 41 (8), 2876–2882.CrossRefGoogle Scholar
Preusse, M. & Peeters, F. 2014 Internal solitary waves in upper lake constance. Hydrobiologia 731 (1), 65–80.CrossRefGoogle Scholar
Quaresma, L.S., Vitorino, J., Oliveira, A. & da Silva, J. 2007 Evidence of sediment resuspension by nonlinear internal waves on the western Portuguese mid-shelf. Mar. Geol. 246 (2–4), 123–143.CrossRefGoogle Scholar
Reeder, D.B., Ma, B.B. & Yang, Y.J. 2011 Very large subaqueous sand dunes on the upper continental slope in the South China Sea generated by episodic, shoaling deep-water internal solitary waves. Mar. Geol. 279 (1–4), 12–18.CrossRefGoogle Scholar
Sakai, T., Diamessis, P.J. & Jacobs, G.B. 2020 Self-sustained instability, transition, and turbulence induced by a long separation bubble in the footprint of an internal solitary wave. I. Flow topology. Phys. Rev. Fluids 5 (10), 103801.CrossRefGoogle Scholar
Signell, R.P. & Geyer, W.R. 1991 Transient eddy formation around headlands. J.Geophys. Res. 96 (C2), 2561–2575.CrossRefGoogle Scholar
Stastna, M. 2011 Resonant generation of internal waves by short length scale topography. Phys. Fluids 23 (11), 116601.CrossRefGoogle Scholar
Stastna, M. & Lamb, K.G. 2008 Sediment resuspension mechanisms associated with internal waves in coastal waters. J.Geophys. Res. 113, C10016.CrossRefGoogle Scholar
Subich, C.J., Lamb, K.G. & Stastna, M. 2013 Simulation of the Navier–Stokes equations in three dimensions with a spectral collocation method. Intl J. Numer. Meth. Fluids 73 (2), 103–129.CrossRefGoogle Scholar
Sveen, J.K., Guo, Y., Davies, P.A. & Grue, J. 2002 On the breaking of internal solitary waves at a ridge. J.Fluid Mech. 469, 161–188.CrossRefGoogle Scholar
Talipova, T., Terletska, K., Maderich, V., Brovchenko, I., Jung, K.T., Pelinovsky, E. & Grimshaw, R. 2013 Internal solitary wave transformation over a bottom step: loss of energy. Phys. Fluids 25 (3), 32110.CrossRefGoogle Scholar
Van Haren, H. & Gostiaux, L. 2012 Energy release through internal wave breaking. Oceanography 25 (2), 124–131.CrossRefGoogle Scholar
Vlasenko, V.I. & Hutter, K. 2001 Generation of second mode solitary waves by the interaction of a first mode soliton with a sill. Nonlinear Process. Geophys. 8 (4/5), 223–239.CrossRefGoogle Scholar
Wang, C. & Pawlowicz, R. 2017 Internal wave generation from tidal flow exiting a constricted opening. J.Geophys. Res. 122 (1), 110–125.CrossRefGoogle Scholar
White, L. & Wolanski, E. 2008 Flow separation and vertical motions in a tidal flow interacting with a shallow-water island. Estuar. Coast. Shelf Sci. 77 (3), 457–466.CrossRefGoogle Scholar
Wiegand, R.C. & Carmack, E.C. 1986 The climatology of internal waves in a deep temperate lake. J.Geophys. Res. 91 (C3), 3951.CrossRefGoogle Scholar
Winters, K.B., Lombard, P.N., Riley, J.J. & D'Asaro, E.A. 1995 Available potential energy and mixing in density-stratified fluids. J.Fluid Mech. 289 (-1), 115.CrossRefGoogle Scholar
Xu, C., Stastna, M. & Deepwell, D. 2019 Spontaneous instability in internal solitary-like waves. Phys. Rev. Fluids 4 (1), 014805.CrossRefGoogle Scholar
Xu, C., Subich, C. & Stastna, M. 2016 Numerical simulations of shoaling internal solitary waves of elevation. Phys. Fluids 28 (7), 76601.CrossRefGoogle Scholar

Deepwell et al. supplementary movie

Time evolution of isopycnals for the base case. Successive frames are 0.5 s apart.

Download Deepwell et al. supplementary movie(Video)
Video 235.5 KB