Hostname: page-component-586b7cd67f-vdxz6 Total loading time: 0 Render date: 2024-11-28T19:12:03.411Z Has data issue: false hasContentIssue false
  • English
  • Français

Viscous resuspension of non-Brownian particles: determination of the concentration profiles and particle normal stresses

Published online by Cambridge University Press:  25 January 2021

Enzo d'Ambrosio ,
Frédéric Blanc  and
Elisabeth Lemaire Open the ORCID record for Elisabeth Lemaire [Opens in a new window]
Enzo d'Ambrosio
Affiliation:
Université Côte d'Azur, CNRS, InPhyNi-UMR 7010, 06108Nice CEDEX 2, France
Frédéric Blanc
Affiliation:
Université Côte d'Azur, CNRS, InPhyNi-UMR 7010, 06108Nice CEDEX 2, France
Elisabeth Lemaire*
Affiliation:
Université Côte d'Azur, CNRS, InPhyNi-UMR 7010, 06108Nice CEDEX 2, France
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

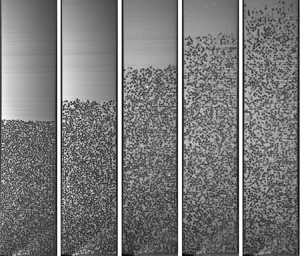
We perform local measurements of both the velocity and the particle volume fraction to study viscous resuspension in non-Brownian suspensions for Shields numbers ranging from $10^{-3}$ to $1$. With this aim, a suspension of polymethacrylate spherical particles dispersed in a lighter Newtonian fluid (Triton X100) is sheared in a vertical Couette cell where both velocity and particle density mappings are performed. We show that the radial profiles of the velocity and of the particle volume fraction are inconsistent in the framework of local rheology of a Newtonian material and that these discrepancies disappear for a neutrally buoyant suspension. The vertical concentration profiles are used to deduce the third particle normal stress, $\varSigma _{33}^p$, by solving the Cauchy equation. The value of $\varSigma _{33}^p$ is shown not to vary linearly with shear rate but rather through a power law with an exponent close to $0.7$, irrespective of the value of the particle volume fraction, in accordance with the recent results of Saint-Michel et al. (Phys. Fluids, vol. 31, 2019, 103301). Finally, we compare our results with the results of previous studies where $\alpha _3=\varSigma _{33}^p/\eta _0\dot {\gamma }$ (with $\eta_0$ the viscosity of the suspending liquid and $\dot{\gamma}$ the shear rate) was deduced from the macroscopic measurement of the height of the resuspended layer. The agreement is satisfactory.

JFM classification

Granular media: Granular media Complex Fluids: Suspensions Multiphase and Particle-laden Flows: Particle/fluid flow
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 911 , 25 March 2021 , A22
Check for updates
Copyright
© The Author(s), 2021. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Abbott, J.R., Tetlow, N., Graham, A.L., Altobelli, S.A., Fukushima, E., Mondy, L.A. & Stephens, T.S. 1991 Experimental observations of particle migration in concentrated suspensions: Couette flow. J. Rheol. 35 (5), 773–795.CrossRefGoogle Scholar
Acrivos, A., Fan, X. & Mauri, R. 1994 On the measurement of the relative viscosity of suspensions. J. Rheol. 38 (5), 1285–1296.CrossRefGoogle Scholar
Acrivos, A., Mauri, R. & Fan, X. 1993 Shear-induced resuspension in a Couette device. Intl J. Multiphase Flow 19 (5), 797–802.CrossRefGoogle Scholar
Ahuja, A. & Singh, A. 2009 Slip velocity of concentrated suspensions in Couette flow. J. Rheol. 53 (6), 1461–1485.CrossRefGoogle Scholar
Blaj, O. 2012 Comment coule une pâte granulaire? Etudes des composantes primaire et secondaire et des fluctuations de l’écoulement. PhD theses, Université Sciences et Technologies – Bordeaux I.Google Scholar
Blaj, O., Merzeau, P., Snabre, P. & Pouligny, B. 2011 An automated single-particle tracker: application to characterization of non-azimuthal motion in Couette flows at low Reynolds number. Exp. Fluids 50 (6), 1559–1570.CrossRefGoogle Scholar
Blanc, F., Lemaire, E., Meunier, A. & Peters, F. 2013 Microstructure in sheared non-Brownian concentrated suspensions. J. Rheol. 57 (1), 273–292.CrossRefGoogle Scholar
Blanc, F., Peters, F. & Lemaire, E. 2011 Local transient rheological behavior of concentrated suspensions. J. Rheol. 55 (4), 835–854.CrossRefGoogle Scholar
Boyer, F., Guazzelli, É. & Pouliquen, O. 2011 Unifying suspension and granular rheology. Phys. Rev. Lett. 107 (18), 188301.CrossRefGoogle ScholarPubMed
Butler, J.E. & Bonnecaze, R.T. 1999 Imaging of particle shear migration with electrical impedance tomography. Phys. Fluids 11 (8), 1982–1994.CrossRefGoogle Scholar
Chatté, G., Comtet, J., Niguès, A., Bocquet, L., Siria, A., Ducouret, G., Lequeux, F., Lenoir, N., Ovarlez, G. & Colin, A. 2018 Shear thinning in non-Brownian suspensions. Soft Matt. 14 (6), 879–893.CrossRefGoogle ScholarPubMed
Chow, A.W., Iwayima, J.H., Sinton, S.W. & Leighton, D.T. 1995 Particle migration of non-Brownian, concentrated suspensions in a truncated cone-and-plate. In Society of Rheology Meeting, Sacramento, CA, vol. 103.Google Scholar
Chow, A.W., Sinton, S.W., Iwamiya, J.H. & Stephens, T.S. 1994 Shear-induced particle migration in Couette and parallel-plate viscometers: NMR imaging and stress measurements. Phys. Fluids 6 (8), 2561–2576.CrossRefGoogle Scholar
Colbourne, A.A., Blythe, T.W., Barua, R., Lovett, S., Mitchell, J., Sederman, A.J. & Gladden, L.F. 2018 Validation of a low field Rheo-NMR instrument and application to shear-induced migration of suspended non-colloidal particles in Couette flow. J. Magn. Reson. 286, 30–35.CrossRefGoogle ScholarPubMed
Dai, S.-C., Bertevas, E., Qi, F. & Tanner, R.I. 2013 Viscometric functions for noncolloidal sphere suspensions with Newtonian matrices. J. Rheol. 57 (2), 493–510.CrossRefGoogle Scholar
Dbouk, T., Lobry, L. & Lemaire, E. 2013 Normal stresses in concentrated non-Brownian suspensions. J. Fluid Mech. 715, 239–272.CrossRefGoogle Scholar
Deboeuf, A., Gauthier, G., Martin, J., Yurkovetsky, Y. & Morris, J.F. 2009 Particle pressure in a sheared suspension: a bridge from osmosis to granular dilatancy. Phys. Rev. Lett. 102 (10), 108301.CrossRefGoogle Scholar
Deboeuf, S., Lenoir, N., Hautemayou, D., Bornert, M., Blanc, F. & Ovarlez, G. 2018 Imaging non-Brownian particle suspensions with x-ray tomography: application to the microstructure of newtonian and viscoplastic suspensions. J. Rheol. 62 (2), 643–663.CrossRefGoogle Scholar
Gadala-Maria, F.A. 1979 The rheology of concentrated suspensions. PhD thesis, Stanford University.Google Scholar
Gallier, S., Lemaire, E., Lobry, L. & Peters, F. 2016 Effect of confinement in wall-bounded non-colloidal suspensions. J. Fluid Mech. 799, 100–127.CrossRefGoogle Scholar
Gallier, S., Lemaire, E., Peters, F. & Lobry, L. 2014 Rheology of sheared suspensions of rough frictional particles. J. Fluid Mech. 757, 514–549.CrossRefGoogle Scholar
Gholami, M., Rashedi, A., Lenoir, N., Hautemayou, D., Ovarlez, G. & Hormozi, S. 2018 Time-resolved 2D concentration maps in flowing suspensions using x-ray. J. Rheol. 62 (4), 955–974.CrossRefGoogle Scholar
Graham, A.L., Altobelli, S.A., Fukushima, E., Mondy, L.A. & Stephens, T.S. 1991 Note: NMR imaging of shear-induced diffusion and structure in concentrated suspensions undergoing Couette flow. J. Rheol. 35 (1), 191–201.CrossRefGoogle Scholar
Guazzelli, É. & Pouliquen, O. 2018 Rheology of dense granular suspensions. J. Fluid Mech. 852, P1.CrossRefGoogle Scholar
Hampton, R.E., Mammoli, A.A., Graham, A.L., Tetlow, N. & Altobelli, S.A. 1997 Migration of particles undergoing pressure-driven flow in a circular conduit. J. Rheol. 41 (3), 621–640.CrossRefGoogle Scholar
Huang, N., Ovarlez, G., Bertrand, F., Rodts, S., Coussot, P. & Bonn, D. 2005 Flow of wet granular materials. Phys. Rev. Lett. 94 (2), 028301.CrossRefGoogle ScholarPubMed
Jana, S.C., Kapoor, B. & Acrivos, A. 1995 Apparent wall slip velocity coefficients in concentrated suspensions of noncolloidal particles. J. Rheol. 39 (6), 1123–1132.CrossRefGoogle Scholar
Koh, C.J., Hookham, P. & Leal, L.G. 1994 An experimental investigation of concentrated suspension flows in a rectangular channel. J. Fluid Mech. 266, 1–32.CrossRefGoogle Scholar
Korhonen, M., Mohtaschemi, M., Puisto, A., Illa, X. & Alava, M.J. 2015 Apparent wall slip in non-Brownian hard-sphere suspensions. Eur. Phys. J. E 38 (5), 46.CrossRefGoogle ScholarPubMed
Leighton, D. & Acrivos, A. 1986 Viscous resuspension. Chem. Engng Sci. 41 (6), 1377–1384.CrossRefGoogle Scholar
Lhuillier, D. 2009 Migration of rigid particles in non-Brownian viscous suspensions. Phys. Fluids 21 (2), 023302.CrossRefGoogle Scholar
Lobry, L., Lemaire, E., Blanc, F., Gallier, S. & Peters, F. 2019 Shear thinning in non-Brownian suspensions explained by variable friction between particles. J. Fluid Mech. 860, 682–710.CrossRefGoogle Scholar
Manneville, S., Bécu, L. & Colin, A. 2004 High-frequency ultrasonic speckle velocimetry in sheared complex fluids. Eur. Phys. J. Appl. Phys. 28 (3), 361–373.CrossRefGoogle Scholar
Metzger, B., Rahli, O. & Yin, X. 2013 Heat transfer across sheared suspensions: role of the shear-induced diffusion. J. Fluid Mech. 724, 527–552.CrossRefGoogle Scholar
Meunier, P. & Leweke, T. 2003 Analysis and optimization of the error caused by high velocity gradients in PIV. Exp. Fluids 35, 408–421.CrossRefGoogle Scholar
Mills, P. & Snabre, P. 1995 Rheology and structure of concentrated suspensions of hard spheres. Shear induced particle migration. J. Phys. II 5 (10), 1597–1608.Google Scholar
Morris, J.F. & Brady, J.F. 1998 Pressure-driven flow of a suspension: buoyancy effects. Intl J. Multiphase Flow 24 (1), 105–130.CrossRefGoogle Scholar
Morris, J.F. & Boulay, F. 1999 Curvilinear flows of noncolloidal suspensions: the role of normal stresses. J. Rheol. 43 (5), 1213–1237.CrossRefGoogle Scholar
Nott, P.R. & Brady, J.F. 1994 Pressure-driven flow of suspensions: simulation and theory. J. Fluid Mech. 275, 157–199.CrossRefGoogle Scholar
Nott, P.R., Guazzelli, E. & Pouliquen, O. 2011 The suspension balance model revisited. Phys. Fluids 23 (4), 043304.CrossRefGoogle Scholar
Ovarlez, G., Bertrand, F. & Rodts, S. 2006 Local determination of the constitutive law of a dense suspension of noncolloidal particles through magnetic resonance imaging. J. Rheol. 50 (3), 259–292.CrossRefGoogle Scholar
Ovarlez, G. & Guazzelli, E. 2013 Migration induite par cisaillement dans les suspensions. In Congrès français de mécanique, AFM, Courbevoie, France.Google Scholar
Phillips, R.J., Armstrong, R.C., Brown, R.A., Graham, A.L. & Abbott, J.R. 1992 A constitutive equation for concentrated suspensions that accounts for shear-induced particle migration. Phys. Fluids A 4 (1), 30–40.CrossRefGoogle Scholar
Ramachandran, A. & Leighton, D.T. 2008 The influence of secondary flows induced by normal stress differences on the shear-induced migration of particles in concentrated suspensions. J. Fluid Mech. 603, 207–243.CrossRefGoogle Scholar
Saint-Michel, B., Manneville, S., Meeker, S., Ovarlez, G. & Bodiguel, H. 2019 X-ray radiography of viscous resuspension. Phys. Fluids 31 (10), 103301.CrossRefGoogle Scholar
Sarabian, M., Firouznia, M., Metzger, B. & Hormozi, S. 2019 Fully developed and transient concentration profiles of particulate suspensions sheared in a cylindrical couette cell. J. Fluid Mech. 862, 659–671.CrossRefGoogle Scholar
Segre, G. & Silberberg, A. 1962 Behaviour of macroscopic rigid spheres in Poiseuille flow. Part 2. Experimental results and interpretation. J. Fluid Mech. 14 (1), 136–157.CrossRefGoogle Scholar
Snook, B., Butler, J.E. & Guazzelli, É. 2016 Dynamics of shear-induced migration of spherical particles in oscillatory pipe flow. J. Fluid Mech. 786, 128–153.CrossRefGoogle Scholar
Souzy, M., Pham, P. & Metzger, B. 2016 Taylor's experiment in a periodically sheared particulate suspension. Phys. Rev. Fluids 1 (4), 042001.CrossRefGoogle Scholar
Souzy, M., Yin, X., Villermaux, E., Abid, C. & Metzger, B. 2015 Super-diffusion in sheared suspensions. Phys. Fluids 27 (4), 041705.CrossRefGoogle Scholar
Suzuki, M., Shinmura, T., Iimura, K. & Hirota, M. 2008 Study of the wall effect on particle packing structure using x-ray micro computed tomography. Adv. Powder Technol. 19 (2), 183–195.CrossRefGoogle Scholar
Tanner, R.I., Ness, C., Mahmud, A., Dai, S. & Moon, J. 2018 A bootstrap mechanism for non-colloidal suspension viscosity. Rheol. Acta 57 (10), 635–643.CrossRefGoogle Scholar
Vázquez-Quesada, A., Mahmud, A., Dai, S., Ellero, M. & Tanner, R.I. 2017 Investigating the causes of shear-thinning in non-colloidal suspensions: experiments and simulations. J. Non-Newtonian Fluid Mech. 248, 1–7.CrossRefGoogle Scholar
Vázquez-Quesada, A., Tanner, R.I. & Ellero, M. 2016 Shear thinning of noncolloidal suspensions. Phys. Rev. Lett. 117 (10), 108001.CrossRefGoogle ScholarPubMed
Vincent, L. & Soille, P. 1991 Watersheds in digital spaces: an efficient algorithm based on immersion simulations. IEEE Trans. Pattern Anal. Mach. Intell. (6), 583–598.CrossRefGoogle Scholar
Westerweel, J. 1997 Fundamentals of digital particle image velocimetry. Meas. Sci. Technol. 8 (12), 1379.CrossRefGoogle Scholar
Yeo, K. & Maxey, M.R. 2010 Ordering transition of non-Brownian suspensions in confined steady shear flow. Phys. Rev. E 81 (5), 051502.CrossRefGoogle ScholarPubMed
Zarraga, I.E., Hill, D.A. & Leighton, D.T. Jr. 2000 The characterization of the total stress of concentrated suspensions of noncolloidal spheres in Newtonian fluids. J. Rheol. 44 (2), 185–220.CrossRefGoogle Scholar
Supplementary material: File

d'Ambrosio et al. supplementary material

d'Ambrosio et al. supplementary material

Download d'Ambrosio et al. supplementary material(File)
File 2.2 MB