Hostname: page-component-586b7cd67f-vdxz6 Total loading time: 0 Render date: 2024-11-29T03:49:32.808Z Has data issue: false hasContentIssue false
  • English
  • Français

The instability nature of the Vogel–Escudier flow

Published online by Cambridge University Press:  09 February 2015

Miguel A. Herrada ,
Vladimir N. Shtern  and
M. M. Torregrosa
Miguel A. Herrada*
Affiliation:
E.S.I., Universidad de Sevilla, Camino de los Descubrimientos s/n 41092, Spain
Vladimir N. Shtern
Affiliation:
Shtern Research and Consulting, Houston, TX 77096, USA
M. M. Torregrosa
Affiliation:
E.S.I., Universidad de Sevilla, Camino de los Descubrimientos s/n 41092, Spain
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

The instability of the steady axisymmetric flow in a sealed elongated cylinder, driven by a rotating end disk, is studied with the help of numerical simulations. It is argued that this instability is of the shear-layer type, being caused by the presence of an inflection point in the radial distribution of axial velocity of the base circulatory flow. The disturbance kinetic energy is localized in both the radial and axial directions, reaching its peak near the rotating disk, where the magnitude of base-flow axial velocity is close to its maximum. 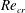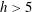The critical Reynolds number, $\mathit{Re}_{cr}$ , is found to be nearly $h$ -independent for $h>5$ ; 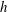$h$ is the cylinder length-to-radius ratio. It is shown that the sidewall co-rotation suppresses the instability. As the co-rotation increases, the centrifugal instability becomes the most dangerous, i.e. determines 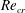$\mathit{Re}_{cr}$ . Physical explanations are given for the stabilizing effect of the co-rotation, which is stronger (weaker) for the shear-layer (centrifugal) instability.

JFM classification

Vortex Flows: Vortex breakdown Vortex Flows: Vortex Flows Vortex Flows: Vortex instability
Type
Papers
Information
Journal of Fluid Mechanics , Volume 766 , 10 March 2015 , pp. 590 - 610
Check for updates
Copyright
© 2015 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Benjamin, T. B. 1962 Theory of vortex breakdown phenomenon. J. Fluid Mech. 14, 593–629.Google Scholar
Bödewadt, U. T. 1940 Die Drehströmung über festem Grund. Z. Angew. Math. Mech. 20, 241–253.Google Scholar
Chandrasekhar, S. 1961 Hydrodynamic and Hydromagnetic Stability. Dover.Google Scholar
Escudier, M. P. 1984 Observation of the flow produced in a cylindrical container by a rotating endwall. Exp. Fluids 2, 189–196.Google Scholar
Escudier, M. P. 1988 Vortex breakdown: observations and explanations. Prog. Aerosp. Sci. 25, 189–229.CrossRefGoogle Scholar
Gelfgat, A. Yu., Bar-Yoseph, P. Z. & Solan, A. 1996 Stability of confined swirling flow with and without vortex breakdown. J. Fluid Mech. 311, 1–36.CrossRefGoogle Scholar
Gelfgat, A. Yu., Bar-Yoseph, P. Z. & Solan, A. 2001 Three-dimensional instabilities of axisymmetric flow in a rotating lid-cylinder enclosure. J. Fluid Mech. 438, 363–377.CrossRefGoogle Scholar
Hall, M. G. 1972 Vortex breakdown. Annu. Rev. Fluid Mech. 4, 125–218.Google Scholar
Herrada, M. A., Pérez-Saborid, M. & Barrero, A. 2004 Nonparallel local spatial stability analysis of pipe entrance swirling flows. Phys. Fluids 16, 2147–2153.Google Scholar
Hills, C. P. 2001 Eddies induced in cylindrical containers by a rotating end wall. Phys. Fluids 13, 2279–2286.Google Scholar
Husain, H., Shtern, V. & Hussain, F. 2003 Control of vortex breakdown by addition of near-axis swirl. Phys. Fluids 15, 271–279.CrossRefGoogle Scholar
Iwatsu, R. 2005 Vortex breakdown flows in cylindrical geometry. Notes Numer. Fluid Mech. Multidiscip. Des. 90, 141–151.Google Scholar
von Kármán, T. 1921 Über laminare und turbulent reibung. Z. Angew. Math. Mech. 1, 233–252.Google Scholar
Kulikov, D. V., Mikkelsen, R., Naumov, I. V. & Okulov, V. L. 2014 Diagnostics of bubble-mode vortex breakdown in swirling flow in a large-aspect-ratio cylinder. Tech. Phys. Lett. 40 (2), 181–184.Google Scholar
Lambourne, N. C. & Brayer, D. W.1961 The bursting of leading edge vortices – some observations and discussion of the phenomenon. Aero. Res. Counc. R & M 3282.Google Scholar
Leibovich, S. 1984 Vortex stability and breakdown: survey and extention. AIAA J. 22, 1192–1206.Google Scholar
Lopez, J. M. 1990 Axisymmetric vortex breakdown. Part 1. Confined swirling flow. J. Fluid Mech. 221, 533–552.CrossRefGoogle Scholar
Lopez, J. M. 2006 Rotating and modulated rotating waves in transitions of an enclosed swirling flow. J. Fluid Mech. 553, 323–346.CrossRefGoogle Scholar
Mununga, L., Lo Jacono, D., Sørensen, J. N., Leweke, T., Thompson, M. C. & Hourigan, K. 2014 Control of confined vortex breakdown with partial rotating lids. J. Fluid Mech. 738, 5–33.Google Scholar
Olendraru, C., Sellier, A., Rossi, M. & Huerre, P. 1996 Absolute/convective instability of the Batchelor vortex. C. R. Acad. Sci. Paris 11b, 153–159.Google Scholar
Shankar, P. N. 1998 Three-dimensional Stokes flow in a cylindrical container. Phys. Fluids 10, 540–549.Google Scholar
Shtern, V. 2012 Counterflows. Cambridge University Press.Google Scholar
Shtern, V. & Borissov, A. 2010a Counter-flow driven by swirl decay. Phys. Fluids 22, 063601.Google Scholar
Shtern, V. & Borissov, A. 2010b Nature of counterflow and circulation in vortex separators. Phys. Fluids 22, 083601.Google Scholar
Shtern, V. N., Torregrosa, M. M. & Herrada, M. A. 2011a Development of a swirling double counterflow. Phys. Rev. E 83, 056322.Google Scholar
Shtern, V. N., Torregrosa, M. M. & Herrada, M. A. 2011b Development of colliding counterflows. Phys. Rev. E 84, 046306.Google Scholar
Shtern, V. N., Torregrosa, M. M. & Herrada, M. A. 2012 Effect of swirl decay on vortex breakdown in a confined steady axisymmetric flow. Phys. Fluids 24, 043601.Google Scholar
Sorensen, J. N., Gelfgat, A. Y., Naumov, I. V. & Mikkelsen, R. 2009 Experimental and numerical results on the three-dimensional instabilities in a rotating disk-tall cylinder flow. Phys. Fluids 21, 054102.Google Scholar
Sorensen, J. N., Naumov, I. V. & Mikkelsen, R. 2006 Experimental investigation in three-dimensional flow instabilities in a rotating lid-driven cavity. Exp. Fluids 41, 425–440.Google Scholar
Sorensen, J. N., Naumov, I. V. & Okulov, V. L. 2011 Multiple helical modes of vortex breakdown. J. Fluid Mech. 683, 430–441.CrossRefGoogle Scholar
Trigub, V. N. 1985 The problem of breakdown of a vortex line. Z. Angew. Math. Mech. 95, 166–171.Google Scholar
Vogel, H. U.1968 Experimentelle Ergebnisse über die laminare Strömung in einem Zylindrischen Gehäuse mit darin rotierender Scheibe. Max-Planck-Institut für Strömungsforschung, Göttigen, Bericht 6.Google Scholar