Hostname: page-component-78c5997874-v9fdk Total loading time: 0 Render date: 2024-11-07T17:14:05.252Z Has data issue: false hasContentIssue false
  • English
  • Français

Inertial and dimensional effects on the instability of a thin film

Published online by Cambridge University Press:  16 December 2015

Alejandro G. González ,
Javier A. Diez  and
Mathieu Sellier
Alejandro G. González*
Affiliation:
Instituto de Física Arroyo Seco (CIFICEN-CONICET), Universidad Nacional del Centro de la Provincia de Buenos Aires, Pinto 399, 7000, Tandil, Argentina
Javier A. Diez
Affiliation:
Instituto de Física Arroyo Seco (CIFICEN-CONICET), Universidad Nacional del Centro de la Provincia de Buenos Aires, Pinto 399, 7000, Tandil, Argentina
Mathieu Sellier
Affiliation:
Mechanical Engineering Department, University of Canterbury, Christchurch 8140, New Zealand
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

We consider here the effects of inertia on the instability of a flat liquid film under the effects of capillary and intermolecular forces (van der Waals interaction). Firstly, we perform a linear stability analysis within the long-wave approximation, which shows that the inclusion of inertia does not produce new regions of instability other than the one previously known from the usual lubrication case. The wavelength, 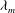${\it\lambda}_{m}$, corresponding to the maximum growth, 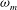${\it\omega}_{m}$ and the critical (marginal) wavelength do not change. The most affected feature of the instability under an increase of the Laplace number is the noticeable decrease of the growth rates of the unstable modes. In order to put in evidence the effects of the bidimensional aspects of the flow (neglected in the long-wave approximation), we also calculate the dispersion relation of the instability from the linearized version of the complete Navier–Stokes (N–S) equations. Unlike the long-wave approximation, the bidimensional model shows that 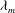${\it\lambda}_{m}$ can vary significantly with inertia when the aspect ratio of the film is not sufficiently small. We also perform numerical simulations of the nonlinear N–S equations and analyse to which extent the linear predictions can be applied depending on both the amount of inertia involved and the aspect ratio of the film.

JFM classification

Interfacial Flows (free surface): Interfacial Flows (free surface) Low-Reynolds-number flows: Lubrication theory Micro-/Nano-fluid dynamics: Micro-/Nano-fluid dynamics
Type
Papers
Information
Journal of Fluid Mechanics , Volume 787 , 25 January 2016 , pp. 449 - 473
Check for updates
Copyright
© 2015 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Bischof, J., Scherer, D., Herminghaus, S. & Leiderer, P. 1996 Dewetting modes of thin metallic films: nucleation of holes and spinodal dewetting. Phys. Rev. Lett. 77, 1536–1539.Google Scholar
Cahn, J. E. 1965 Phase separation by spinodal decomposition in isotropic systems. J. Chem. Phys. 42, 93–99.Google Scholar
Colinet, P., Kaya, H., Rossomme, S. & Scheid, B. 2007 Some advances in lubrication-type theories. Eur. Phys. J. Special Topics 146, 377–389.Google Scholar
Craster, R. V. & Matar, O. K. 2009 Dynamics and stability of thin liquid films. Rev. Mod. Phys. 81, 1131–1198.CrossRefGoogle Scholar
Diez, J. & Kondic, L. 2007 On the breakup of fluid films of finite and infinite extent. Phys. Fluids 19, 072107.Google Scholar
Eggers, J. 1997 Nonlinear dynamics and breakup of free-surface flows. Rev. Mod. Phys. 69, 865–929.Google Scholar
Fowlkes, J. D., Roberts, N. A., Wu, Y., Diez, J. A., González, A. G., Hartnett, C., Mahady, K., Afkhami, S., Kondic, L. & Rack, P. D. 2014 Hierarchical nanoparticle ensembles synthesized by liquid phase directed self-assembly. Nano Lett. 41, 774–782.Google Scholar
González, A. G., Diez, J. A., Wu, Y., Fowlkes, J. D., Rack, P. D. & Kondic, L. 2013 Instability of liquid Cu films on a $\text{SiO}_{2}$ substrate. Langmuir 29, 9378–9387.Google Scholar
Hocking, L. M. & Davis, S. H. 2002 Inertial effects in time-dependent motion of thin films and drops. J. Fluid Mech. 467, 1–17.Google Scholar
Hori, Y. 2006 Hydrodynamic Lubrication. Springer.Google Scholar
Israelachvili, J. N. 1992 Intermolecular and Surface Forces, 2nd edn. Academic.Google Scholar
Jacobs, K. & Herminghaus, S. 1998 Thin liquid polymer films rupture via defects. Langmuir 41, 965–969.Google Scholar
Kargupta, K., Sharma, A. & Khanna, R. 2004 Instability, dynamics, and morphology of thin slipping films. Langmuir 20, 244–253.Google Scholar
Lopez, P. G., Miksis, M. J. & Bankoff, S. G. 1997 Inertial effects on contact line instability in the coating of a dry inclined plane. Phys. Fluids 9, 2177–2183.Google Scholar
Mitlin, V. S. 1993 Dewetting of solid surface: analogy with spinodal decomposition. J. Colloid Interface Sci. 156, 491–497.CrossRefGoogle Scholar
Nguyen, T. D., Fuentes-Cabrera, M., Fowlkes, J. D., Diez, J. A., González, A. G., Kondic, L. & Rack, P. D. 2012 Competition between collapse and breakup in nanometer-sized thin rings using molecular dynamics and continuum modeling. Langmuir 28, 13960–13967.Google Scholar
Oron, A., Davis, S. H. & Bankoff, S. G. 1997 Long-scale evolution of thin liquid films. Rev. Mod. Phys. 69, 931–980.Google Scholar
Schwartz, L. W. 1998 Hysteretic effects in droplet motions on heterogenous substrates: direct numerical simulations. Langmuir 14, 3440–3453.Google Scholar
Schwartz, L. W. & Eley, R. R. 1998 Simulation of droplet motion of low-energy and heterogenous surfaces. J. Colloid Interface Sci. 202, 173–188.CrossRefGoogle Scholar
Seemann, R., Herminghaus, S., Neto, C., Schlagowski, S., Podzimek, D., Konrad, R., Mantz, H. & Jacobs, K. 2005 Dynamics and structure formation in thin polymer melt films. J. Phys.: Condens. Matter 17, S267–S290.Google Scholar
Szeri, A. Z. 2011 Fluid Film Lubrication. Cambridge University Press.Google Scholar
Thiele, U. 2003 Open questions and promising new fields in dewetting. Eur. Phys. J. E 12, 409–416.Google Scholar
Thiele, U., Mertig, M. & Pompe, W. 1998 Dewetting of an evaporating thin liquid film: heterogeneous nucleation and surface instability. Phys. Rev. Lett. 80, 2869–2872.CrossRefGoogle Scholar
Thiele, U., Velarde, M. G. & Neuffer, K. 2001 Dewetting: film rupture by nucleation in the spinodal regime. Phys. Rev. Lett. 87, 016104.Google Scholar
Ubal, S., Grassia, P., Campana, D. M., Giavedoni, M. D. & Saita, F. A. 2014 The influence of inertia and contact angle on the instability of partially wetting liquid strips: a numerical analysis study. Phys. Fluids 26, 032106.Google Scholar
Xie, R., Karim, A., Douglas, J. F., Han, C. C. & Weiss, R. A. 1998 Spinodal dewetting of thin polymer films. Phys. Rev. Lett. 81, 1251–1254.CrossRefGoogle Scholar
Zhang, W. Z. & Lister, J. R. 1999 Similarity solutions of van der Waals rupture of a thin film on a solid substrate. Phys. Fluids 11, 2454–2462.Google Scholar