Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Watanabe, Tomoaki
Riley, James J.
Nagata, Koji
Onishi, Ryo
and
Matsuda, Keigo
2018.
A localized turbulent mixing layer in a uniformly stratified environment.
Journal of Fluid Mechanics,
Vol. 849,
Issue. ,
p.
245.
van Reeuwijk, Maarten
Holzner, Markus
and
Caulfield, C. P.
2019.
Mixing and entrainment are suppressed in inclined gravity currents.
Journal of Fluid Mechanics,
Vol. 873,
Issue. ,
p.
786.
Neamtu-Halic, Marius M.
Krug, Dominik
Haller, George
and
Holzner, Markus
2019.
Lagrangian coherent structures and entrainment near the turbulent/non-turbulent interface of a gravity current.
Journal of Fluid Mechanics,
Vol. 877,
Issue. ,
p.
824.
Charrondière, Claudine
Brun, Christophe
Sicart, Jean-Emmanuel
Cohard, Jean-Martial
Biron, Romain
and
Blein, Sébastien
2020.
Buoyancy Effects in the Turbulence Kinetic Energy Budget and Reynolds Stress Budget for a Katabatic Jet over a Steep Alpine Slope.
Boundary-Layer Meteorology,
Vol. 177,
Issue. 1,
p.
97.
Kohan, Khashayar F.
and
Gaskin, Susan
2020.
The effect of the geometric features of the turbulent/non-turbulent interface on the entrainment of a passive scalar into a jet.
Physics of Fluids,
Vol. 32,
Issue. 9,
Neamtu-Halic, Marius M.
Krug, Dominik
Mollicone, Jean-Paul
van Reeuwijk, Maarten
Haller, George
and
Holzner, Markus
2020.
Connecting the time evolution of the turbulence interface to coherent structures.
Journal of Fluid Mechanics,
Vol. 898,
Issue. ,
Gauding, M.
Bode, M.
Brahami, Y.
Varea, É.
and
Danaila, L.
2021.
Self-similarity of turbulent jet flows with internal and external intermittency.
Journal of Fluid Mechanics,
Vol. 919,
Issue. ,
El-Nabulsi, Rami Ahmad
2021.
Thermal transport equations in porous media from product-like fractal measure.
Journal of Thermal Stresses,
Vol. 44,
Issue. 7,
p.
899.
Sadeghi, H.
Oberlack, M.
and
Gauding, M.
2021.
New symmetry-induced scaling laws of passive scalar transport in turbulent plane jets.
Journal of Fluid Mechanics,
Vol. 919,
Issue. ,
Abreu, Hugo
Pinho, Fernando T.
and
da Silva, Carlos B.
2022.
Turbulent entrainment in viscoelastic fluids.
Journal of Fluid Mechanics,
Vol. 934,
Issue. ,
Kohan, Khashayar F.
and
Gaskin, Susan J.
2022.
On the scalar turbulent/turbulent interface of axisymmetric jets.
Journal of Fluid Mechanics,
Vol. 950,
Issue. ,
Gauding, M.
Thiesset, F.
Varea, E.
and
Danaila, L.
2022.
Structure of iso-scalar sets.
Journal of Fluid Mechanics,
Vol. 942,
Issue. ,
Brizzolara, Stefano
Mollicone, Jean-Paul
van Reeuwijk, Maarten
and
Holzner, Markus
2023.
Entrainment at multi-scales in shear-dominated and Rayleigh–Taylor turbulence.
European Journal of Mechanics - B/Fluids,
Vol. 101,
Issue. ,
p.
294.
Thiesset, F.
and
Federrath, C.
2023.
Structure of iso-density sets in supersonic isothermal turbulence.
Astronomy & Astrophysics,
Vol. 676,
Issue. ,
p.
A12.
Kumar, D.
Chand, A. K. B.
and
Massopust, P. R.
2024.
Fractal Multiquadric Interpolation Functions.
SIAM Journal on Numerical Analysis,
Vol. 62,
Issue. 5,
p.
2349.
Watanabe, Tomoaki
2024.
Efficient enhancement of turbulent entrainment by small-scale shear instability.
Journal of Fluid Mechanics,
Vol. 988,
Issue. ,
Tao, Shancong
Xie, Yuanliang
Shi, Xiaotian
and
Zhou, Yi
2024.
Detection of the irrotational boundary using machine learning methods.
Physics of Fluids,
Vol. 36,
Issue. 7,
 $A_{\unicode[STIX]{x1D702}}$ of the turbulent/non-turbulent interface (TNTI) in gravity currents decreases with increasing stratification, significantly reducing the entrainment rate. Here, we consider the multiscale properties of this effect using direct numerical simulations of temporal gravity currents with different gradient Richardson numbers
$A_{\unicode[STIX]{x1D702}}$ of the turbulent/non-turbulent interface (TNTI) in gravity currents decreases with increasing stratification, significantly reducing the entrainment rate. Here, we consider the multiscale properties of this effect using direct numerical simulations of temporal gravity currents with different gradient Richardson numbers 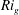 $Ri_{g}$. Our results indicate that the reduction of
$Ri_{g}$. Our results indicate that the reduction of 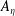 $A_{\unicode[STIX]{x1D702}}$ is caused by a decrease of the fractal scaling exponent
$A_{\unicode[STIX]{x1D702}}$ is caused by a decrease of the fractal scaling exponent 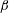 $\unicode[STIX]{x1D6FD}$, while the scaling range remains largely unaffected. We further find that convolutions of the TNTI are characterized by different length scales in the streamwise and wall-normal directions, namely the integral scale
$\unicode[STIX]{x1D6FD}$, while the scaling range remains largely unaffected. We further find that convolutions of the TNTI are characterized by different length scales in the streamwise and wall-normal directions, namely the integral scale 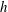 $h$ and the shear scale
$h$ and the shear scale  $l_{Sk}=k^{1/2}/S$ (formed using the mean shear
$l_{Sk}=k^{1/2}/S$ (formed using the mean shear 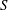 $S$ and the turbulent kinetic energy
$S$ and the turbulent kinetic energy 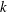 $k$) respectively. By recognizing that the anisotropy implied by the different scaling relations increases with increasing
$k$) respectively. By recognizing that the anisotropy implied by the different scaling relations increases with increasing  $Ri_{g}$, we are able to model the
$Ri_{g}$, we are able to model the 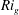 $Ri_{g}$ dependence of
$Ri_{g}$ dependence of 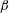 $\unicode[STIX]{x1D6FD}$ in good agreement with the data.
$\unicode[STIX]{x1D6FD}$ in good agreement with the data.


