Hostname: page-component-586b7cd67f-2brh9 Total loading time: 0 Render date: 2024-12-01T01:20:15.280Z Has data issue: false hasContentIssue false
  • English
  • Français

A finite-size correction model for two-fluid large-eddy simulation of particle-laden boundary layer flow

Published online by Cambridge University Press:  26 February 2021

Antoine Mathieu Open the ORCID record for Antoine Mathieu [Opens in a new window] ,
Julien Chauchat Open the ORCID record for Julien Chauchat [Opens in a new window] ,
Cyrille Bonamy ,
Guillaume Balarac  and
Tian-Jian Hsu
Antoine Mathieu*
Affiliation:
LEGI, University of Grenoble Alpes, G-INP, CNRS, 38000Grenoble, France
Julien Chauchat
Affiliation:
LEGI, University of Grenoble Alpes, G-INP, CNRS, 38000Grenoble, France
Cyrille Bonamy
Affiliation:
LEGI, University of Grenoble Alpes, G-INP, CNRS, 38000Grenoble, France
Guillaume Balarac
Affiliation:
LEGI, University of Grenoble Alpes, G-INP, CNRS, 38000Grenoble, France Institut Universitaire de France (IUF), 75005Paris, France
Tian-Jian Hsu
Affiliation:
Center for Applied Coastal Research, University of Delaware, Newark, DE19716, USA
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

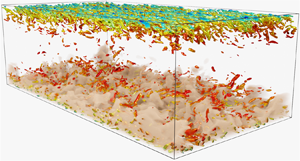
In this paper the capabilities of the turbulence-resolving Eulerian–Eulerian two-phase flow model to predict the suspension of mono-dispersed finite-sized solid particles in a boundary layer flow are investigated. For heavier-than-fluid particles, having settling velocity of the order of the bed friction velocity, the two-fluid model significantly under-estimates the turbulent dispersion of particles. It is hypothesized that finite-size effects are important and a correction model for the drag law is proposed. This model is based on the assumption that the turbulent flow scales larger than the particle diameter will contribute to the resolved relative velocity between the two phases, whereas eddies smaller than the particle diameter will have two effects: (i) they will reduce the particle response time by adding a sub-particle scale eddy viscosity to the drag coefficient, and (ii) they will contribute to increase the production of granular temperature. Integrating finite-size effects allows us to quantitatively predict the concentration profile for heavier-than-fluid particles without any tuning parameter. The proposed modification of the two-fluid model extends its range of applicability to tackle particles having a size belonging to the inertial range of turbulence and allows us to envision more complex applications in terms of flow forcing conditions, i.e. sheet flow, wave-driven transport, turbidity currents and/or flow geometries, i.e. ripples, dunes, scour.

JFM classification

Geophysical and Geological Flows: Sediment transport Multiphase and Particle-laden Flows: Particle/fluid flow
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 913 , 25 April 2021 , A26
Check for updates
Copyright
© The Author(s), 2021. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Agrawal, K., Loezos, P.N., Syamlal, M. & Sundaresan, S. 2001 The role of meso-scale structures in rapid gas-solid flows. J. Fluid Mech. 445, 151–185.CrossRefGoogle Scholar
Arshad, S., Gonzalez-Juez, E., Dasgupta, A., Menon, S. & Oevermann, M. 2019 Subgrid reaction-diffusion closure for large eddy simulations using the linear-eddy model. Flow Turbul. Combust. 13, 361–376.Google Scholar
Balachandar, S. 2009 A scaling analysis for point-particle approaches to turbulent multiphase flows. Intl J. Multiphase Flow 35, 801–810.CrossRefGoogle Scholar
Balachandar, S. & Eaton, J.K. 2010 Turbulent dispersed multiphase flow. Annu. Rev. Fluid Mech. 42 (1), 111–133.CrossRefGoogle Scholar
Berzi, D. & Fraccarollo, L. 2016 Intense sediment transport: collisional to turbulent suspension. Phys. Fluids 28 (2), 023302.CrossRefGoogle Scholar
Calzavarini, E., Volk, R., Bourgoin, M., Lévêque, E., Pinton, J.-F. & Toschi, F. 2009 Acceleration statistics of finite-sized particles in turbulent flow: the role of faxén forces. J. Fluid Mech. 630, 179–189.CrossRefGoogle Scholar
Carnahan, N.F. & Starling, K.E. 1969 Equation of state for nonattracting rigid spheres. J. Chem. Phys. 51 (2), 635–636.CrossRefGoogle Scholar
Chatzimichailidis, A., Argyropoulos, C., Assael, M. & Kakosimos, K. 2019 Qualitative and quantitative investigation of multiple large eddy simulation aspects for pollutant dispersion in street canyons using openfoam. Atmosphere 10, 17.CrossRefGoogle Scholar
Chauchat, J., Cheng, Z., Nagel, T., Bonamy, C. & Hsu, T.-J. 2017 Sedfoam-2.0: a 3-d two-phase flow numerical model for sediment transport. Geosci. Model Develop. 10 (12), 4367–4392.CrossRefGoogle Scholar
Cheng, Z., Hsu, T.-J. & Calantoni, J. 2017 Sedfoam: a multi-dimensional Eulerian two-phase model for sediment transport and its application to momentary bed failure. Coast. Engng 119, 32–50.CrossRefGoogle Scholar
Cheng, Z., Hsu, T.-J. & Chauchat, J. 2018 An Eulerian two-phase model for steady sheet flow using large-eddy simulation methodology. Adv. Water Resour. 111, 205–223.CrossRefGoogle Scholar
Ferry, J. & Balachandar, S. 2001 A fast Eulerian method for disperse two-phase flow. Intl J. Multiphase Flow 27 (7), 1199–1226.CrossRefGoogle Scholar
Ferry, J. & Balachandar, S. 2005 Equilibrium Eulerian approach for predicting the thermal field of a dispersion of small particles. Intl J. Heat Mass Transfer 48 (3), 681–689.CrossRefGoogle Scholar
Février, P., Simonin, O. & Squires, K.D. 2005 Partitioning of particle velocities in gas-solid turbulent flows into a continuous field and a spatially uncorrelated random distribution: theoretical formalism and numerical study. J. Fluid Mech. 533, 1–46.CrossRefGoogle Scholar
Finn, J.R. & Li, M. 2016 Regimes of sediment-turbulence interaction and guidelines for simulating the multiphase bottom boundary layer. Intl J. Multiphase Flow 85, 278–283.CrossRefGoogle Scholar
Fox, R.O. 2014 On multiphase turbulence models for collisional fluid-particle flows. J. Fluid Mech. 742, 368–424.CrossRefGoogle Scholar
Germano, M., Piomelli, U., Moin, P. & Cabot, W.H. 1991 A dynamic subgrid-scale eddy viscosity model. Phys. Fluids A 3 (7), 1760–1765.CrossRefGoogle Scholar
Gidaspow, D. 1986 Hydrodynamics of fluidization and heat transfer: supercomputer modeling. Appl. Mech. Rev. 39 (1), 1–23.CrossRefGoogle Scholar
Gidaspow, D. 1994 Multiphase Flow and Fluidization. Academic Press.Google Scholar
Gorokhovski, M. & Zamansky, R. 2018 Modeling the effects of small turbulent scales on the drag force for particles below and above the Kolmogorov scale. Phys. Rev. Fluids 3, 034602.CrossRefGoogle Scholar
Heynderickx, G., Das, A., De Wilde, J. & Marin, G. 2004 Effect of clustering on gas-solid drag in dilute two-phase flow. Ind. Engng Chem. Res. 43, 4635–4646.CrossRefGoogle Scholar
Homann, H. & Bec, J. 2010 Finite-size effects in the dynamics of neutrally buoyant particles in turbulent flow. J. Fluid Mech. 651, 81–91.CrossRefGoogle Scholar
Hsu, T.-J., Jenkins, J.T. & Liu, P.L.-F. 2004 On two-phase sediment transport: sheet flow of massive particles. Proc. R. Soc. Lond. A 460 (2048), 2223–2250.CrossRefGoogle Scholar
Igci, Y., Andrews, I.V., Arthur, T., Sundaresan, S., Pannala, S. & O'Brien, T. 2008 Filtered two-fluid models for fluidized gas-particle suspensions. AIChE J. 54 (6), 1431–1448.CrossRefGoogle Scholar
Jasak, H. & Uroić, T. 2020 Practical Computational Fluid Dynamics with the Finite Volume Method, pp. 103–161. Springer International Publishing.Google Scholar
Kaftori, D., Hetsroni, G. & Banerjee, S. 1995 Particle behavior in the turbulent boundary layer. II. Velocity and distribution profiles. Phys. Fluids 7 (5), 1107–1121.CrossRefGoogle Scholar
Kidanemariam, A.G., Chan-Braun, C., Doychev, T. & Uhlmann, M. 2013 Dns of horizontal open channel flow with finite-size, heavy particles at low solid volume fraction. New J. Phys. 15 (2), 25–31.CrossRefGoogle Scholar
Kiger, K. & Pan, C. 2002 Suspension and turbulence modification effects of solid particulates on a horizontal turbulent channel flow. J. Turbul. 3, 27–29.CrossRefGoogle Scholar
Lilly, D.K. 1992 A proposed modification of the Germano subgrid-scale closure method. Phys. Fluids A 4 (3), 633–635.CrossRefGoogle Scholar
Lyn, D.A. 2008 Turbulence Models for Sediment Transport Engineering, chap. 16. ASCE.CrossRefGoogle Scholar
Mathieu, A., Chauchat, J., Bonamy, C. & Nagel, T. 2019 Two-phase flow simulation of tunnel and lee-wake erosion of scour below a submarine pipeline. Water 11 (8), 1–17.CrossRefGoogle Scholar
Maxey, M.R. & Riley, J.J. 1983 Equation of motion for a small rigid sphere in a nonuniform flow. Phys. Fluids 26 (4), 883–889.CrossRefGoogle Scholar
Meneveau, C., Lund, T.S. & Cabot, W.H. 1996 A lagrangian dynamic subgrid-scale model of turbulence. J. Fluid Mech. 319, 353–385.CrossRefGoogle Scholar
Montecchia, M., Brethouwer, G., Wallin, S., Johansson, A.V. & Knacke, T. 2019 Improving LES with openfoam by minimising numerical dissipation and use of explicit algebraic SGS stress model. J. Turbul. 20 (11–12), 697–722.CrossRefGoogle Scholar
Moser, R.D., Kim, J. & Mansour, N.N. 1999 Direct numerical simulation of turbulent channel flow up to $Re_\tau =590$. Phys. Fluids 11 (4), 943–945.CrossRefGoogle Scholar
Muste, M., Yu, K., Fujita, I. & Ettema, R. 2005 Two-phase versus mixed-flow perspective on suspended sediment transport in turbulent channel flows. Water Resour. Res. 41 (10), 1–22.CrossRefGoogle Scholar
Nagel, T., Chauchat, J., Bonamy, C., Liu, X., Cheng, Z. & Hsu, T.-J. 2020 Three-dimensional scour simulations with a two-phase flow model. Adv. Water Resour. 138, 103544.CrossRefGoogle Scholar
Niño, Y. & Garcia, M.H. 1996 Experiments on particle-turbulence interactions in the near-wall region of an open channel flow: implications for sediment transport. J. Fluid Mech. 326, 285–319.CrossRefGoogle Scholar
O'Brien, T. & Syamlal, M. 1993 Particle cluster effects in the numerical simulation of a circulating fluidized bed. In Proceedings of the Fourth International Conference on Circulating Fluidized Beds, pp. 430–435.Google Scholar
Ozel, A., Fede, P. & Simonin, O. 2013 Development of filtered euler-euler two-phase model for circulating fluidised bed: high resolution simulation, formulation and a priori analyses. Intl J. Multiphase Flow 55, 43–63.CrossRefGoogle Scholar
Qureshi, N.M., Bourgoin, M., Baudet, C., Cartellier, A. & Gagne, Y. 2007 Turbulent transport of material particles: an experimental study of finite size effects. Phys. Rev. Lett. 99, 184502.CrossRefGoogle ScholarPubMed
Revil-Baudard, T., Chauchat, J., Hurther, D. & Barraud, P.-A. 2015 Investigation of sheet-flow processes based on novel acoustic high-resolution velocity and concentration measurements. J. Fluid Mech. 769, 723–724.CrossRefGoogle Scholar
Ries, F., Li, Y., Nishad, K., Dressler, L., Ziefuss, M., Mehdizadeh, A., Hasse, C. & Sadiki, A. 2021 A wall-adapted anisotropic heat flux model for large eddy simulations of complex turbulent thermal flows. Flow Turbul. Combust. 106, 733–752.CrossRefGoogle Scholar
Righetti, M. & Romano, G.P. 2004 Particle-fluid interactions in a plane near-wall turbulent flow. J. Fluid Mech. 505, 93–121.CrossRefGoogle Scholar
van Rijn, L.C. 1984 Sediment transport. Part 2. Suspended load transport. J. Hydraul. Engng 110 (11), 1613–1641.CrossRefGoogle Scholar
Rouse, H. 1938 Experiments on the mechanics of sediment suspension. In ICTAM, pp. 550–554.Google Scholar
Rusche, H. 2002 Computational fluid dynamics of dispersed two-phase flows at high phase fractions. PhD thesis, Imperial College London.Google Scholar
Schiller, L. & Naumann, A.Z. 1933 Über die grundlegenden Berechnungen bei der Schwerkraftaufbereitung. Ver. Deut. Ing. 77, 318–320.Google Scholar
Voth, G.A., La Porta, A., Crawford, A.M., Alexander, J. & Bodenschatz, E. 2002 Measurement of particle accelerations in fully developed turbulence. J. Fluid Mech. 469, 121–160.CrossRefGoogle Scholar
Vowinckel, B., Kempe, T. & Fröhlich, J. 2014 Fluid-particle interaction in turbulent open channel flow with fully-resolved mobile beds. Adv. Water Resour. 72, 32–44.CrossRefGoogle Scholar
Vowinckel, B., Nikora, V., Kempe, T. & Fröhlich, J. 2017 Momentum balance in flows over mobile granular beds: application of double-averaging methodology to DNS data. J. Hydraul. Res. 55 (2), 190–207.CrossRefGoogle Scholar
Wang, J., van der Hoef, M.A. & Kuipers, J.A.M. 2009 Why the two-fluid model fails to predict the bed expansion characteristics of geldart a particles in gas-fluidized beds: a tentative answer. Chem. Engng Sci. 64 (3), 622–625.CrossRefGoogle Scholar
Xu, H. & Bodenschatz, E. 2008 Motion of inertial particles with size larger than Kolmogorov scale in turbulent flows. Physica D 237 (14), 2095–2100.CrossRefGoogle Scholar
Yoshizawa, A. & Horiuti, K. 1985 A statistically-derived subgrid-scale kinetic energy model for the large-eddy simulation of turbulent flows. J. Phys. Soc. Japan 54 (8), 2834–2839.CrossRefGoogle Scholar