Hostname: page-component-586b7cd67f-r5fsc Total loading time: 0 Render date: 2024-12-01T03:49:38.708Z Has data issue: false hasContentIssue false
  • English
  • Français

Finite Reynolds number effect on the scaling range behaviour of turbulent longitudinal velocity structure functions

Published online by Cambridge University Press:  05 May 2017

S. L. Tang Open the ORCID record for S. L. Tang [Opens in a new window] ,
R. A. Antonia ,
L. Djenidi Open the ORCID record for L. Djenidi [Opens in a new window] ,
L. Danaila  and
Y. Zhou
S. L. Tang
Affiliation:
Institute for Turbulence-Noise-Vibration Interaction and Control, Shenzhen Graduate School, Harbin Institute of Technology, Shenzhen, 518055, PR China Digital Engineering Laboratory of Offshore Equipment, Shenzhen, 518055, PR China
R. A. Antonia*
Affiliation:
School of Engineering, University of Newcastle, NSW 2308, Australia
L. Djenidi
Affiliation:
School of Engineering, University of Newcastle, NSW 2308, Australia
L. Danaila
Affiliation:
CORIA CNRS UMR 6614, Université de Rouen Normandie, 76801 Saint Etienne du Rouvray, France
Y. Zhou
Affiliation:
Institute for Turbulence-Noise-Vibration Interaction and Control, Shenzhen Graduate School, Harbin Institute of Technology, Shenzhen, 518055, PR China Digital Engineering Laboratory of Offshore Equipment, Shenzhen, 518055, PR China
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

The effect of large-scale forcing on the second- and third-order longitudinal velocity structure functions, evaluated at the Taylor microscale 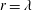$r=\unicode[STIX]{x1D706}$, is assessed in various turbulent flows at small to moderate values of the Taylor microscale Reynolds number 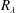$R_{\unicode[STIX]{x1D706}}$. It is found that the contribution of the large-scale terms to the scale by scale energy budget differs from flow to flow. For a fixed 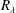$R_{\unicode[STIX]{x1D706}}$, this contribution is largest on the centreline of a fully developed channel flow but smallest for stationary forced periodic box turbulence. For decaying-type flows, the contribution lies between the previous two cases. Because of the difference in the large-scale term between flows, the third-order longitudinal velocity structure function at 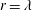$r=\unicode[STIX]{x1D706}$ differs from flow to flow at small to moderate 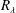$R_{\unicode[STIX]{x1D706}}$. The effect on the second-order velocity structure functions appears to be negligible. More importantly, the effect of 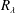$R_{\unicode[STIX]{x1D706}}$ on the scaling range exponent of the longitudinal velocity structure function is assessed using measurements of the streamwise velocity fluctuation $u$, with $R_{\unicode[STIX]{x1D706}}$ in the range 500–1100, on the axis of a plane jet. It is found that the magnitude of the exponent increases as 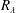$R_{\unicode[STIX]{x1D706}}$ increases and the rate of increase depends on the order $n$. The trend of published structure function data on the axes of an axisymmetric jet and a two-dimensional wake confirms this dependence. For a fixed $R_{\unicode[STIX]{x1D706}}$, the exponent can vary from flow to flow and for a given flow, the larger 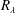$R_{\unicode[STIX]{x1D706}}$ is, the closer the exponent is to the value predicted by Kolmogorov (Dokl. Akad. Nauk SSSR, vol. 30, 1941a, pp. 299–303) (hereafter K41). The major conclusion is that the finite Reynolds number effect, which depends on the flow, needs to be properly accounted for before determining whether corrections to K41, arising from the intermittency of the energy dissipation rate, are needed. We further point out that it is imprudent, if not incorrect, to associate the finite Reynolds number effect with a consequence of the modified similarity hypothesis introduced by Kolmogorov (J. Fluid Mech., vol. 13, 1962, pp. 82–85) (K62); we contend that this association has misled the vast majority of post K62 investigations of the consequences of K62.

JFM classification

Turbulent Flows: Isotropic turbulence Turbulent Flows: Turbulence theory Turbulent Flows: Turbulent Flows
Type
Papers
Information
Journal of Fluid Mechanics , Volume 820 , 10 June 2017 , pp. 341 - 369
Check for updates
Copyright
© 2017 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Anselmet, F., Gagne, Y., Hopfinger, E. J. & Antonia, R. A. 1984 High-order velocity structure functions in turbulent shear flows. J. Fluid Mech. 140, 63–89.CrossRefGoogle Scholar
Antonia, R. A. & Burattini, P. 2006 Approach to the 4/5 law in homogeneous isotropic turbulence. J. Fluid Mech. 550, 175–184.Google Scholar
Antonia, R. A., Djenidi, L. & Danaila, L. 2014 Collapse of the turbulent dissipation range on kolmogorov scales. Phys. Fluids 26, 045105.Google Scholar
Antonia, R. A. & Pearson, B. R. 2000 Reynolds number dependence of velocity structure functions in a turbulent pipe flow. Flow Turbul. Combust. 64, 95–117.Google Scholar
Antonia, R. A., Pearson, B. R. & Zhou, T. 2000a Reynolds number dependence of second-order velocity structure functions. Phys. Fluids 12, 3000–3006.CrossRefGoogle Scholar
Antonia, R. A., Pearson, B. R. & Zhou, T. 2002a Reynolds number dependence of second-order velocity structure functions. Phys. Fluids 12, 3000–3006.Google Scholar
Antonia, R. A., Satyaprakash, B. R. & Chambers, A. J. 1982a Reynolds number dependence of velocity structure functions in turbulent shear flows. Phys. Fluids 25, 29–37.CrossRefGoogle Scholar
Antonia, R. A., Satyaprakash, B. R. & Hussain, A. K. M. F. 1982b Statistics of fine-scale velocity in turbulent plane and circular jets. J. Fluid Mech. 119, 55–89.Google Scholar
Antonia, R. A., Smalley, R. J., Zhou, T., Anselmet, F. & Danaila, L. 2003 Similarity of energy structure functions in decaying homogeneous isotropic turbulence. J. Fluid Mech. 487, 245–269.Google Scholar
Antonia, R. A., Tang, S. L., Djenidi, L. & Danaila, L. 2015 Boundedness of the velocity derivative skewness in various turbulent flows. J. Fluid Mech. 781, 727–744.CrossRefGoogle Scholar
Antonia, R. A., Zhou, T., Danaila, L. & Anselmet, F. 2000b Streamwise inhomogeneity of decaying grid turbulence. Phys. Fluids 12, 3086–3089.CrossRefGoogle Scholar
Antonia, R. A., Zhou, T. & Romano, G. P. 2002b Small-scale turbulence characteristics of two-dimensional bluff body wakes. J. Fluid Mech. 459, 67–92.Google Scholar
Batchelor, G. K. 1951 Pressure fluctuations in isotropic turbulence. Proc. Camb. Phil. Soc. 47, 533–559.Google Scholar
Belin, F., Maurer, J., Tabeling, P. & Willaime, H. 1997 Velocity gradient distributions in fully developed turbulence: experimental study. Phys. Fluids 9, 3843–3850.CrossRefGoogle Scholar
Benzi, R., Ciliberto, S., Tripiccione, R., Baudet, C., Massaioli, F. & Succi, S. 1993 Extended self-similarity in turbulent flows. Phys. Rev. E 48, 29–32.Google Scholar
Bos, W. J. T., Chevillard, L., Scott, J. F. & Rubinstein, R. 2012 Reynolds number effect on the velocity increment skewness in isotropic turbulence. Phys. Fluids 24, 015108.CrossRefGoogle Scholar
Boschung, J., Hennig, F., Gauding, M., Pitsch, H. & Peters, N. 2016 Generalised higher-order Kolmogorov scales. J. Fluid Mech. 794, 233–251.CrossRefGoogle Scholar
Burattini, P., Antonia, R. A. & Danaila, L. 2005a Scale-by-scale energy budget on the axis of a turbulent round jet. J. Turbul. 6, N19.CrossRefGoogle Scholar
Burattini, P., Antonia, R. A. & Danaila, L. 2005b Similarity in the far field of a turbulent round jet. Phys. Fluids 17, 025101.CrossRefGoogle Scholar
Cleve, J., Greiner, M., Pearson, B. R. & Sreenivasan, K. R. 2004 Intermittency exponent of the turbulent energy cascade. Phys. Rev. E 69, 066316.Google ScholarPubMed
Danaila, L., Anselmet, F. & Antonia, R. A. 2002 An overview of the effect of large-scale inhomogeneities on small-scale turbulence. Phys. Fluids 14, 2475–2482.Google Scholar
Danaila, L., Anselmet, F., Zhou, T. & Antonia, R. A. 1999 A generalization of Yaglom’s equation which accounts for the large-scale forcing in heated decaying turbulence. J. Fluid Mech. 391, 359–372.Google Scholar
Danaila, L., Anselmet, F., Zhou, T. & Antonia, R. A. 2001 Turbulent energy scale-budget equations in a fully developed channel flow. J. Fluid Mech. 430, 87–109.Google Scholar
Danaila, L., Antonia, R. A. & Burattini, P. 2004 Progress in studying small-scale turbulence using ‘exact’ two-point equations. New J. Phys. 6, 2–23.Google Scholar
Djenidi, L. & Antonia, R. A. 2015 A general self-preservation analysis for decaying homogenous isotropic turbulence. J. Fluid Mech. 773, 345–365.CrossRefGoogle Scholar
Frisch, U. 1995 Turbulence: The Legacy of A. N. Kolmogorov. Cambridge University Press.Google Scholar
Frisch, U., Sulem, P. L. & Nelkin, M. 1978 A simple dynamical model of intermittent fully developed turbulence. J. Fluid Mech. 87, 719–736.Google Scholar
Fukayama, D., Oyamada, T., Nakano, T., Gotoh, T. & Yamamoto, K. 2000 Longitudinal structure functions in decaying and forced turbulence. J. Phys. Soc. Japan 69, 701–715.Google Scholar
Gotoh, T., Fukayama, D. & Nakano, T. 2002 Velocity field statistics in homogeneous steady turbulence obtained using a high-resolution direct numerical simulation. Phys. Fluids 14, 1065–1081.CrossRefGoogle Scholar
Gotoh, T. & Nakano, T. 2003 Role of pressure in turbulence. J. Stat. Phys. 113 (5), 855–874.Google Scholar
Gotoh, T. & Watanabe, T. 2015 Power and nonpower laws of passive scalar moments convected by isotropic turbulence. Phys. Rev. Lett. 115 (11), 114502.CrossRefGoogle ScholarPubMed
Hao, Z., Zhou, T., Zhou, Y. & Mi, J. 2008 Reynolds number dependence of the inertial range scaling of energy dissipation rate and enstrophy in a cylinder wake. Exp. Fluids 44, 279–289.Google Scholar
Hearst, R. J. & Lavoie, P. 2014 Decay of turbulence generated by a square-fractal-element grid. J. Fluid Mech. 741, 567–584.Google Scholar
Hill, R. J. 2001 Equations relating structure functions of all orders. J. Fluid Mech. 434, 379–388.Google Scholar
Hill, R. J. & Wilczak, J. M. 1995 Pressure structure functions and spectra for locally isotropic turbulence. J. Fluid Mech. 296, 247–269.CrossRefGoogle Scholar
Ishihara, T., Morishita, K., Yokokawa, M., Uno, A. & Kaneda, Y. 2016 Energy spectrum in high-resolution direct numerical simulations of turbulence. Phys. Rev. Fluids 1 (8), 082403.CrossRefGoogle Scholar
Kolmogorov, A. N. 1941a Local structure of turbulence in an incompressible fluid for very large Reynolds numbers. Dokl. Akad. Nauk SSSR 30, 299–303.Google Scholar
Kolmogorov, A. N. 1941b Dissipation of energy in the locally isotropic turbulence. Dokl. Akad. Nauk SSSR 32, 19–21.Google Scholar
Kolmogorov, A. N. 1962 A refinement of previous hypotheses concerning the local structure of turbulence in a viscous incompressible fluid at high Reynolds number. J. Fluid Mech. 13, 82–85.Google Scholar
Kurien, S. & Sreenivasan, K. R. 2000 Anisotropic scaling contributions to high-order structure functions in high-Reynolds-number turbulence. Phys. Rev. E 62, 2206–2212.Google ScholarPubMed
Lindborg, E. 1999 Correction to the four-fifths law due to variations of the dissipation. Phys. Fluids 11, 510.Google Scholar
L’vov, V. S. & Procaccia, I. 1995 ‘Intermittency’ in hydrodynamic turbulence as intermediate asymptotics to Kolmogorov scaling. Phys. Rev. Lett. 74, 2690.Google Scholar
Maurer, J., Tabeling, P. & Zocchi, G. 1994 Statistics of turbulence between two counterrotating disks in low-temperature helium gas. Europhys. Lett. 26 (1), 31.CrossRefGoogle Scholar
Meldi, M. & Sagaut, P. 2013 Pressure statistics in self-similar freely decaying isotropic turbulence. J. Fluid Mech. 717, R2.Google Scholar
Mi, J., Xu, M. & Zhou, T. 2013 Reynolds number influence on statistical behaviors of turbulence in a circular free jet. Phys. Fluids 25, 075101.Google Scholar
Moisy, F., Tabeling, P. & Willaime, H. 1999 Kolmogorov equation in a fully developed turbulence experiment. Phys. Rev. Lett. 82 (20), 3994.Google Scholar
Monin, A. S. & Yaglom, A. M. 2007 Statistical Fluid Dynamics, vol. 2. MIT.Google Scholar
Morrison, J. F., Vallikivi, M. & Smits, A. J. 2016 The inertial subrange in turbulent pipe flow: centreline. J. Fluid Mech. 788, 602–613.CrossRefGoogle Scholar
Mydlarski, L. & Warhaft, Z. 1996 On the onset of high- Reynolds-number grid-generated wind tunnel turbulence. J. Fluid Mech. 320, 331–368.Google Scholar
Ni, R. & Xia, K. Q. 2013 Kolmogorov constants for the second-order structure function and the energy spectrum. Phys. Rev. E 87 (2), 023002.Google Scholar
Obligado, M., Dairay, T. & Vassilicos, J. C. 2016 Nonequilibrium scalings of turbulent wakes. Phys. Rev. Fluids 1 (4), 044409.Google Scholar
Pearson, B. R. & Antonia, R. A. 2001 Reynolds-number dependence of turbulent velocity and pressure increments. J. Fluid Mech. 444, 343–382.Google Scholar
Peters, N., Boschung, J., Gauding, M., Goebbert, J. H., Hill, R. J. & Pitsch, H. 2016 Higher-order dissipation in the theory of homogeneous isotropic turbulence. J. Fluid Mech. 803, 250–274.CrossRefGoogle Scholar
Pope, S. B. 2000 Turbulent Flows. Cambridge University Press.Google Scholar
Praskovsky, A. & Oncley, S. 1994 Measurements of the Kolmogorov constant and intermittency exponent at very high Reynolds numbers. Phys. Fluids 6, 2886–2888.Google Scholar
Qian, J. 1997 Inertial range and the finite Reynolds number effect of turbulence. Phys. Rev. E 55, 337–342.Google Scholar
Qian, J. 1998 Normal and anomalous scaling of turbulence. Phys. Rev. E 58, 7325.Google Scholar
Qian, J. 1999 Slow decay of the finite Reynolds number effect of turbulence. Phys. Rev. E 60, 3409.Google Scholar
Sagaut, P. & Cambon, C. 2008 Homogeneous Turbulence Dynamics. Cambridge University Press.Google Scholar
She, Z.-S. & Leveque, E. 1994 Universal scaling laws in fully developed turbulence. Phys. Rev. Lett. 72 (3), 336.CrossRefGoogle ScholarPubMed
Sreenivasan, K. & Antonia, R. A. 1997 The phenomenology of small-scale turbulence. Annu. Rev. Fluid Mech. 29, 435–472.CrossRefGoogle Scholar
Tabeling, P., Zocchi, G., Belin, F., Maurer, J. & Willaime, H. 1996 Probability density functions, skewness, and flatness in large Reynolds number turbulence. Phys. Rev. E 53, 1613–1621.Google Scholar
Tang, S. L., Antonia, R. A., Djenidi, L., Abe, H., Zhou, T., Danaila, L. & Zhou, Y. 2015a Transport equation for the mean turbulent energy dissipation rate on the centreline of a fully developed channel flow. J. Fluid Mech. 777, 151–177.CrossRefGoogle Scholar
Tang, S. L., Antonia, R. A., Djenidi, L. & Zhou, Y. 2015b Transport equation for the isotropic turbulent energy dissipation rate in the far-wake of a circular cylinder. J. Fluid Mech. 784, 109–129.CrossRefGoogle Scholar
Tchoufag, J., Sagaut, P. & Cambon, C. 2012 Spectral approach to finite Reynolds number effects on Kolmogorov’s 4/5 law in isotropic turbulence. Phys. Fluids 24 (1), 015107.CrossRefGoogle Scholar
Thiesset, F., Antonia, R. A. & Danaila, L. 2013b Scale-by-scale turbulent energy budget in the intermediate wake of two-dimensional generators. Phys. Fluids 25, 115105.Google Scholar
Thiesset, F., Antonia, R. A. & Djenidi, L. 2014 Consequences of self-preservation on the axis of a turbulent round jet. J. Fluid Mech. 748, R2.CrossRefGoogle Scholar
Thiesset, F., Danaila, L. & Antonia, R. A. 2013a Dynamical effect of the total strain induced by the coherent motion on local isotropy in a wake. J. Fluid Mech. 720, 393–423.CrossRefGoogle Scholar
Tsuji, Y. & Ishihara, T. 2003 Similarity scaling of pressure fluctuation in turbulence. Phys. Rev. E 68, 026309.Google Scholar
Valente, P. C. & Vassilicos, J. C. 2012 Universal dissipation scaling for non-equilibrium turbulence. Phys. Rev. Lett. 108, 214503.Google Scholar
Vassilicos, J. C. 2015 Dissipation in turbulent flows. Annu. Rev. Fluid Mech. 47, 95–114.CrossRefGoogle Scholar
Vedula, P. & Yeung, P. K. 1999 Similarity scaling of acceleration and pressure statistics in numerical simulations of isotropic turbulence. Phys. Fluids 11, 1208–1220.Google Scholar
Vincent, A. & Meneguzzi, M. 1991 The spatial structure and statistical properties of homogeneous turbulence. J. Fluid Mech. 225, 1–20.Google Scholar
Xu, G., Antonia, R. A. & Rajagopalan, S. 2001 Sweeping decorrelation hypothesis in a turbulent round jet. Fluid Dyn. Res. 28 (5), 311–321.Google Scholar
Zhou, T. & Antonia, R. A. 2000 Reynolds number dependence of the small-scale structure of grid turbulence. J. Fluid Mech. 406, 81–107.Google Scholar
Zhou, T., Antonia, R. A. & Chua, L. P. 2005 Flow and Reynolds number dependencies of one-dimensional vorticity fluctuations. J. Turbul. 6, N28.Google Scholar