Hostname: page-component-78c5997874-94fs2 Total loading time: 0 Render date: 2024-11-03T00:59:22.158Z Has data issue: false hasContentIssue false
  • English
  • Français

Comparison between passive scalar and velocity fields in a turbulent cylinder wake

Published online by Cambridge University Press:  26 January 2017

J. G. Chen ,
T. M. Zhou ,
R. A. Antonia  and
Y. Zhou
J. G. Chen
Affiliation:
Institute for Turbulence–Noise–Vibration Interactions and Control, Shenzhen Graduate School, Harbin Institute of Technology, Shenzhen 518055, China
T. M. Zhou
Affiliation:
School of Civil, Environmental and Mining Engineering, The University of Western Australia, 35 Stirling Highway, Crawley, WA 6009, Australia
R. A. Antonia
Affiliation:
School of Engineering, University of Newcastle, NSW 2308, Australia
Y. Zhou*
Affiliation:
Institute for Turbulence–Noise–Vibration Interactions and Control, Shenzhen Graduate School, Harbin Institute of Technology, Shenzhen 518055, China
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

This work compares the enstrophy with the scalar dissipation rate, as well as the passive scalar variance with the turbulent kinetic energy, in the presence of coherent Kármán vortices in the intermediate wake of a circular cylinder. Measurements are made at $x/d=10$, 20 and 40, where $x$ is the streamwise distance from the cylinder axis and 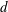$d$ is the cylinder diameter, with a Reynolds number of 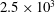$2.5\times 10^{3}$ based on the cylinder diameter and the free-stream velocity. A probe consisting of eight hot wires (four X-wires) and four cold wires is used to measure simultaneously the three components of the fluctuating velocity and vorticity vectors, as well as the fluctuating temperature gradient vector at nominally the same point in the plane of the mean shear. It is found that the enstrophy and scalar dissipation spectra collapse approximately at all wavenumbers except around the Kármán vortex street wavenumber for 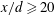$x/d\geqslant 20$. The spectral similarity between the streamwise velocity fluctuation $u$ and the passive scalar 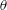$\unicode[STIX]{x1D703}$ is better than that between the velocity fluctuation vector 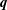$\boldsymbol{q}$ and $\unicode[STIX]{x1D703}$. This is closely related to the highly organized lateral velocity fluctuation $v$ in this flow. The present observations are fully consistent with the expectation that small scales of the velocity and temperature fields are more likely to exhibit a close relationship than scales associated with the bulk of the turbulent energy or scalar variance. The variation across the wake of the time scale ratio between scalar and velocity fields is significantly smaller than that of the turbulent Prandtl number.

JFM classification

Mixing: Turbulent mixing Wakes/Jets: Wakes
Type
Papers
Information
Journal of Fluid Mechanics , Volume 813 , 25 February 2017 , pp. 667 - 694
Check for updates
Copyright
© 2017 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Abe, H. & Antonia, R. A. 2009 Near-wall similarity between velocity and scalar fluctuations in a turbulent channel flow. Phys. Fluids 21, 25109.Google Scholar
Abe, H., Antonia, R. A. & Kawamura, H. 2009 Correlation between small-scale velocity and scalar fluctuations in a turbulent channel flow. J. Fluid Mech. 627, 1–32.Google Scholar
Antonia, R. A., Abe, H. & Kawamura, H. 2009 Analogy between velocity and scalar fields in a turbulent channel flow. J. Fluid Mech. 628, 241–268.Google Scholar
Antonia, R. A. & Browne, L. 1986 Anisotropy of the temperature dissipation in a turbulent wake. J. Fluid Mech. 163, 393–403.Google Scholar
Antonia, R. A., Browne, L. W. B., Bisset, D. K. & Fulachier, L. 1987 A description of the organized motion in the turbulent far wake of a cylinder at low Reynolds number. J. Fluid Mech. 184, 423–444.Google Scholar
Antonia, R. A. & Burattini, P. 2006 Approach to the 4/5 law in homogeneous isotropic turbulence. J. Fluid Mech. 550, 175–184.Google Scholar
Antonia, R. A., Djenidi, L. & Danaila, L. 2014 Collapse of the turbulent dissipative range on Kolmogorov scales. Phys. Fluids 26, 45105.Google Scholar
Antonia, R. A. & Kim, J. 1991 Similarity between turbulent kinetic energy and temperature spectra in the near-wall region. Phys. Fluids A 3, 989–991.Google Scholar
Antonia, R. A., Kim, J. & Browne, L. 1991 Some characteristics of small-scale turbulence in a turbulent duct flow. J. Fluid Mech. 233, 369–388.Google Scholar
Antonia, R. A. & Mi, J. 1993 Corrections for velocity and temperature derivatives in turbulent flows. Exp. Fluids 14, 203–208.Google Scholar
Antonia, R. A. & Mi, J. 1998 Approach towards self-preservation of turbulent cylinder and screen wakes. Exp. Therm. Fluid Sci. 17, 277–284.Google Scholar
Antonia, R. A., Ould-Rouis, M., Anselmet, F. & Zhu, Y. 1997 Analogy between predictions of Kolmogorov and Yaglom. J. Fluid Mech. 332, 395–409.Google Scholar
Antonia, R. A. & Rajagopalan, S. 1990 Performance of lateral vorticity probe in a turbulent wake. Exp. Fluids 9, 119–120.Google Scholar
Antonia, R. A., Zhou, Y. & Matsumura, M. 1993 Spectral characteristics of momentum and heat transfer in the turbulent wake of a circular cylinder. Exp. Therm. Fluid Sci. 6, 371–375.Google Scholar
Béguier, C., Dekeyser, I. & Launder, B. E. 1978 Ratio of scalar and velocity dissipation time scales in shear flow turbulence. Phys. Fluids 21, 307–310.Google Scholar
Berajeklian, A. & Mydlarski, L. 2011 Simultaneous velocity–temperature measurements in the heated wake of a cylinder with implications for the modeling of turbulent passive scalars. Phys. Fluids 23, 55107.Google Scholar
Bisset, D. K., Antonia, R. A. & Browne, L. 1990 Spatial organization of large structures in the turbulent far wake of a cylinder. J. Fluid Mech. 218, 439–461.CrossRefGoogle Scholar
Chassaing, P., Antonia, R. A., Anselmet, F., Joly, L. & Sarkar, S. 2002 Variable Density Fluid Turbulence. Springer.Google Scholar
Chen, J. G., Zhou, Y., Zhou, T. M. & Antonia, R. A. 2016 Three-dimensional vorticity, momentum and heat transport in a turbulent cylinder wake. J. Fluid Mech. 809, 135–167.Google Scholar
Chua, L. P. & Antonia, R. A. 1991 Spectral analogy between temperature variance and turbulent energy in a circular jet. Intl Commun. Heat Mass Transfer 18, 569–579.Google Scholar
Corrsin, S. 1953 Remarks on turbulent heat transfer: an account of some features of the phenomenon in fully turbulent regions. In Proceedings of the Iowa Thermodynamics Symposium, pp. 5–30. State University of Iowa.Google Scholar
Fulachier, L. & Antonia, R. A. 1983 Spectral relationships between velocity and temperature fluctuations in turbulent shear flows. Phys. Fluids 26, 2105–2108.Google Scholar
Fulachier, L. & Antonia, R. A. 1984 Spectral analogy between temperature and velocity fluctuations in several turbulent flows. Intl J. Heat Mass Transfer 27, 987–997.Google Scholar
Fulachier, L. & Dumas, R. 1971 Spectral distributions of thermal fluctuations in a turbulent boundary layer. AGARD-CP Turbulent Shear Flow 93, 4.Google Scholar
Fulachier, L. & Dumas, R. 1976 Spectral analogy between temperature and velocity fluctuations in a turbulent boundary layer. J. Fluid Mech. 77, 257–277.Google Scholar
Hussain, A. & Hayakawa, M. 1987 Eduction of large-scale organized structures in a turbulent plane wake. J. Fluid Mech. 180, 193–229.Google Scholar
Kolmogorov, A. N. 1941 The local structure of turbulence in incompressible viscous fluid for very large Reynolds numbers. Dokl. Akad. Nauk SSSR 30, 301–305.Google Scholar
Lefeuvre, N., Thiesset, F., Djenidi, L. & Antonia, R. A. 2014 Statistics of the turbulent kinetic energy dissipation rate and its surrogates in a square cylinder wake flow. Phys. Fluids 26, 95104.Google Scholar
Marasli, B., Nguyen, P. & Wallace, J. M. 1993 A calibration technique for multiple-sensor hot-wire probes and its application to vorticity measurements in the wake of a circular cylinder. Exp. Fluids 15, 209–218.CrossRefGoogle Scholar
Matsumura, M. & Antonia, R. A. 1993 Momentum and heat transport in the turbulent intermediate wake of a circular cylinder. J. Fluid Mech. 250, 651–668.Google Scholar
Mi, J. & Antonia, R. A. 1994 Temperature distribution within vortices in the wake of a cylinder. Intl J. Heat Mass Transfer 37, 1048–1050.Google Scholar
Newman, G. R., Launder, B. E. & Lumley, J. L. 1981 Modelling the behaviour of homogeneous scalar turbulence. J. Fluid Mech. 111, 217–232.Google Scholar
Obukhov, A. M. 1949 Structure of the temperature field in turbulent flows. Izv. Akad. Nauk SSSR Geogr. Geophys. 13, 58–62.Google Scholar
Pope, S. B. 2001 Turbulent Flows. Cambridge University Press.Google Scholar
Reynolds, A. J. 1976 The variation of turbulent Prandtl and Schmidt numbers in wakes and jets. Intl J. Heat Mass Transfer 19, 757–764.CrossRefGoogle Scholar
Shraiman, B. I. & Siggia, E. D. 2000 Scalar turbulence. Nature 405, 639–646.Google Scholar
Sreenivasan, K. R. 1991 On local isotropy of passive scalars in turbulent shear flows. Proc. R. Soc. Lond. A 434, 165–182.Google Scholar
Tennekes, H. & Lumley, J. L. 1972 A First Course in Turbulence. MIT Press.Google Scholar
Thiesset, F., Danaila, L. & Antonia, R. A. 2013 Dynamical effect of the total strain induced by the coherent motion on local isotropy in a wake. J. Fluid Mech. 720, 393–423.Google Scholar
Thiesset, F., Danaila, L. & Antonia, R. A. 2014 Dynamical interactions between the coherent motion and small scales in a cylinder wake. J. Fluid Mech. 749, 201–226.Google Scholar
Tomkins, C. D. & Adrian, R. J. 2003 Spanwise structure and scale growth in turbulent boundary layers. J. Fluid Mech. 490, 37–74.Google Scholar
Wallace, J. M. & Foss, J. F. 1995 The measurement of vorticity in turbulent flows. Annu. Rev. Fluid Mech. 27, 469–514.Google Scholar
Warhaft, Z. 2000 Passive scalars in turbulent flows. Annu. Rev. Fluid Mech. 32, 203–240.Google Scholar
Warhaft, Z. & Lumley, J. L. 1978 An experimental study of the decay of temperature fluctuations in grid-generated turbulence. J. Fluid Mech. 88, 659–684.Google Scholar
Watanabe, T. & Gotoh, T. 2004 Statistics of a passive scalar in homogeneous turbulence. New J. Phys. 6, 40.Google Scholar
Yeh, T. T. & Atta, C. W. 1973 Spectral transfer of scalar and velocity fields in heated-grid turbulence. J. Fluid Mech. 58, 233–261.Google Scholar
Yiu, M. W., Zhou, Y., Zhou, T. & Cheng, L. 2004 Reynolds number effects on three-dimensional vorticity in a turbulent wake. AIAA J. 42, 1009–1016.Google Scholar
Zhou, Y. & Antonia, R. A. 1992 Convection velocity measurements in a cylinder wake. Exp. Fluids 13, 63–70.Google Scholar
Zhu, Y. & Antonia, R. A. 1996 Spatial resolution of a 4-X-wire vorticity probe. Meas. Sci. Technol. 7, 1492.Google Scholar
Zhou, T., Antonia, R. A. & Chua, L. 2002a Performance of a probe for measuring turbulent energy and temperature dissipation rates. Exp. Fluids 33, 334–345.Google Scholar
Zhou, T., Antonia, R. A., Danaila, L. & Anselmet, F. 2000a Transport equations for the mean energy and temperature dissipation rates in grid turbulence. Exp. Fluids 28, 143–151.Google Scholar
Zhou, T., Razali, S. M., Zhou, Y., Chua, L. P. & Cheng, L. 2009 Dependence of the wake on inclination of a stationary cylinder. Exp. Fluids 46, 1125–1138.Google Scholar
Zhou, Y., So, R. M. C., Liu, M. H. & Zhang, H. J. 2000b Complex turbulent wakes generated by two and three side-by-side cylinders. Intl J. Heat Fluid Flow 21, 125–133.Google Scholar
Zhou, Y. & Yiu, M. W. 2006 Flow structure, momentum and heat transport in a two-tandem-cylinder wake. J. Fluid Mech. 548, 17–48.Google Scholar
Zhou, Y., Zhang, H. J. & Yiu, M. W. 2002b The turbulent wake of two side-by-side circular cylinders. J. Fluid Mech. 458, 303–332.Google Scholar
Zhou, T., Zhou, Y., Yiu, M. W. & Chua, L. P. 2003 Three-dimensional vorticity in a turbulent cylinder wake. Exp. Fluids 35, 459–471.Google Scholar