Published online by Cambridge University Press: 22 March 2006
We study Hamilton-Jacobi equations related to the boundary (or internal) control of semilinear parabolic equations, including the case of a control acting in a nonlinear boundary condition, or the case of a nonlinearity of Burgers' type in 2D. To deal with a control acting in a boundary condition a fractional power $(-A)^\beta$ 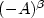 – where (A,D(A)) is an unbounded operator in a Hilbert space X – is contained in the Hamiltonian functional appearing in the Hamilton-Jacobi equation. This situation has already been studied in the literature. But, due to the nonlinear term in the state equation, the same fractional power $(-A)^\beta$
– where (A,D(A)) is an unbounded operator in a Hilbert space X – is contained in the Hamiltonian functional appearing in the Hamilton-Jacobi equation. This situation has already been studied in the literature. But, due to the nonlinear term in the state equation, the same fractional power $(-A)^\beta$ 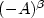 appears in another nonlinear term whose behavior is different from the one of the Hamiltonian functional. We also consider cost functionals which are not bounded in bounded subsets in X, but only in bounded subsets in a space $Y\hookrightarrow X$
appears in another nonlinear term whose behavior is different from the one of the Hamiltonian functional. We also consider cost functionals which are not bounded in bounded subsets in X, but only in bounded subsets in a space $Y\hookrightarrow X$ 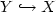 . To treat these new difficulties, we show that the value function of control problems we consider is equal in bounded sets in Y to the unique viscosity solution of some Hamilton-Jacobi-Bellman equation. We look for viscosity solutions in classes of functions which are Hölder continuous with respect to the time variable.
. To treat these new difficulties, we show that the value function of control problems we consider is equal in bounded sets in Y to the unique viscosity solution of some Hamilton-Jacobi-Bellman equation. We look for viscosity solutions in classes of functions which are Hölder continuous with respect to the time variable.